Revisiting the Gravitational Energy of the Schwarzschild Spacetime with a New Approach to the Calculations

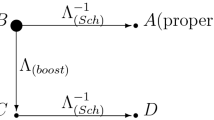
Abstract

Recently, a new method to simplify calculations in teleparallel theories has been put forward. In this article, this method is improved and used to analyze the gravitational energy–momentum tensor (density) of the Schwarzschild solution in a frame that is arbitrarily accelerated along the z-direction. It is shown that, for a special type of frame, one cannot make the gravitational energy density vanish along the observer’s worldline, regardless of the observer’s acceleration. The role played by the frame and its relation to the observers’ worldlines are investigated. It is shown that, for the aforementioned special frames, the results for the gravitational energy and angular momenta are consistent with what we would expect.

Appendix

1.1 Applying the Hybrid Machinery to Eqs. (42)–(45)

In this appendix we give the torsion tensor, the superpotential, the torsion scalar, and the quantity $\Sigma ^{\lambda \mu \nu }T_{\lambda \mu \alpha }$ in terms of the tetrad given by Eq. (24) with $\{\hat{t}_\lambda ,\hat{s}_\lambda ,\hat{\phi }_\lambda ,\hat{z}_\lambda \}$ given by Eqs. (42)–(45).

By inverting Eqs. (42)–(45), we get

$$\begin{aligned} \delta _\lambda ^0=\frac{1}{A}\left(f\hat{t}_\lambda -g\hat{z}_\lambda\right ), \end{aligned}$$

(57)

$$\begin{aligned} \delta _\lambda ^1=\frac{1}{B}\left(g\cos \theta \hat{t}_\lambda -\sin \theta \hat{s}_\lambda -f\cos \theta \hat{z}_\lambda\right ), \end{aligned}$$

(58)

$$\begin{aligned} \delta _\lambda ^2=\frac{1}{\rho B}\left(-g\sin \theta \hat{t}_\lambda -\cos \theta \hat{s}_\lambda +f\sin \theta \hat{z}_\lambda\right), \end{aligned}$$

(59)

$$\begin{aligned} \delta _\lambda ^3=-\frac{\hat{\phi }_\lambda ,}{\rho B\sin \theta }. \end{aligned}$$

(60)

We can simplify the calculations by using the following definitions:

$$\begin{aligned} h_1\equiv A{\partial }{_z}f+B{\partial }{_t}g,\ h_2\equiv h_1\cos \theta + f{\partial }{_\rho }A, \\ h_3\equiv A{\partial }{_z}g+B{\partial }{_t}\kern 0.14emf,\ h_4\equiv h_3\cos \theta +g{\partial }{_\rho }A, \\ h_5\equiv \frac{{\partial }{_\rho }A}{AB},\ h_6\equiv \frac{{\partial }{_\rho }B}{B^2}, \\ h_7\equiv \left(\kern 0.14emf^2h_5-g^2h_6\right)\sin \theta ,\ h_8\equiv \frac{h_1}{AB}+fh_5\cos \theta , \\ h_9\equiv (h_5-h_6)fg\sin \theta ,\ h_{10}\equiv \frac{h_3}{AB}+gh_5\cos \theta , \\ h_{11}\equiv (\kern 0.14emf^2h_6-g^2h_5)\sin \theta ,\ h_{12}\equiv h_6g\cos \theta , \\ h_{13}\equiv h_6\sin \theta ,\ h_{14}\equiv h_6\kern 0.14emf\cos \theta , \\ h_{15}\equiv h_{11}+h_{13},\ h_{16}\equiv h_{10}+h_{12}, \\ h_{17}\equiv h_7+h_{11},\ h_{18}\equiv h_8+h_{14}, \\ h_{19}\equiv h_7+h_{13},\ f\partial _{\mu }\kern 0.14emf=g\partial _\mu g. \end{aligned}$$

(61)

Substituting Eqs. (42)–(45) and (60) into Eqs. (28)–(31), we obtain

$$\begin{aligned} T^{(0)}{_{\mu\nu}}=&-2h_2\delta ^0_{[\mu }\delta ^1_{\nu ]}+2h_1\rho \sin \theta \delta ^0_{[\mu }\delta ^2_{\nu ]} \\&+2g\rho ({\partial }{_\rho }B)\sin \theta \delta ^1_{[\mu }\delta ^2_{\nu ]}, \end{aligned}$$

(62)

$$\begin{aligned} T^{\hat{s}}{_{\mu\nu}}=&-2\rho ({\partial }{_\rho }B)\cos \theta \delta ^1_{[\mu }\delta ^2_{\nu ]}, \end{aligned}$$

(63)

$$\begin{aligned} T^{\hat{\phi}}{_{\mu\nu}}=&-2{\partial }{_\rho }(\rho B)\sin \theta \delta ^1_{[\mu }\delta ^3_{\nu ]}-2\rho B\cos \theta \delta ^2_{[\mu }\delta ^3_{\nu ]} \\&+2\delta ^3_{[\mu }\hat{s}_{\nu ]}, \end{aligned}$$

(64)

$$\begin{aligned} T^{\hat{z}}{_{\mu\nu}}=&-2h_4\delta ^0_{[\mu }\delta ^1_{\nu ]}+2h_3\rho \sin \theta \delta ^0_{[\mu }\delta ^2_{\nu ]} \\&+2({\partial }{_\rho }B)f\rho \sin \theta \delta ^1_{[\mu }\delta ^2_{\nu ]}. \end{aligned}$$

(65)

To write the torsion components in terms of $\{\hat{t}_\lambda ,\hat{s}_\lambda ,\hat{\phi }_\lambda ,\hat{z}_\lambda \}$, we use Eqs. (57)–(60). From these equations, one can check that

$$\begin{aligned} \delta ^0_{[\mu }\delta ^1_{\nu ]}=&\ \frac{-1}{AB}\bigl( f\sin \theta \hat{t}_{[\mu }\hat{s}_{\nu ]}+\cos \theta \hat{t}_{[\mu }\hat{z}_{\nu ]} \\&+g\sin \theta \hat{s}_{[\mu }\hat{z}_{\nu ]}\bigr), \end{aligned}$$

(66)

$$\begin{aligned} \delta ^0_{[\mu }\delta ^2_{\nu ]}=&\ \frac{1}{\rho AB}( -f\cos \theta \hat{t}_{[\mu }\hat{s}_{\nu ]}+\sin \theta \hat{t}_{[\mu }\hat{z}_{\nu ]} \\&-g\cos \theta \hat{s}_{[\mu }\hat{z}_{\nu ]}), \end{aligned}$$

(67)

$$\begin{aligned} \delta ^1_{[\mu }\delta ^2_{\nu ]}=&\ \frac{-1}{\rho B^2}\left(g\hat{t}_{[\mu }\hat{s}_{\nu ]}+f\hat{s}_{[\mu }\hat{z}_{\nu ]} \right), \end{aligned}$$

(68)

$$\begin{aligned} \delta ^1_{[\mu }\delta ^3_{\nu ]}=&\ \frac{1}{\rho B^2\sin \theta }( -g\cos \theta \hat{t}_{[\mu }\hat{\phi }_{\nu ]}+\sin \theta \hat{s}_{[\mu }\hat{\phi }_{\nu ]} \\&-f\cos \theta \hat{\phi }_{[\mu }\hat{z}_{\nu ]}), \end{aligned}$$

(69)

$$\begin{aligned} \delta ^2_{[\mu }\delta ^3_{\nu ]}=&\ \frac{1}{\rho ^2 B^2\sin \theta }( g\sin \theta \hat{t}_{[\mu }\hat{\phi }_{\nu ]}+\cos \theta \hat{s}_{[\mu }\hat{\phi }_{\nu ]} \\&+f\sin \theta \hat{\phi }_{[\mu }\hat{z}_{\nu ]} ), \end{aligned}$$

(70)

$$\begin{aligned} \delta ^3_{[\mu }\hat{s}_{\nu ]}=&\ \frac{\hat{s}_{[\mu }\hat{\phi }_{\nu ]}}{\rho B\sin \theta }. \end{aligned}$$

(71)

By substituting Eqs. (66)–(71) into Eqs. (62)–(65) and simplifying, we find that

$$\begin{aligned} T^{(0)}{_{\mu\nu}}=&\ 2h_7\hat{t}_{[\mu }\hat{s}_{\nu ]}+2h_8\hat{t}_{[\mu }\hat{z}_{\nu ]}+2h_9\hat{s}_{[\mu }\hat{z}_{\nu ]}, \end{aligned}$$

(72)

$$\begin{aligned} T^{\hat{s}}{_{\mu\nu}}=&\ 2h_6\cos \theta \left( g\hat{t}_{[\mu }\hat{s}_{\nu ]}+f\hat{s}_{[\mu }\hat{z}_{\nu ]} \right) , \end{aligned}$$

(73)

$$\begin{aligned} T^{\hat{\phi}}{_{\mu\nu}}=&\ 2h_6(g\cos \theta \hat{t}_{[\mu }\hat{\phi }_{\nu ]}-\sin \theta \hat{s}_{[\mu }\hat{\phi }_{\nu ]} \\&+f\cos \theta \hat{\phi }_{[\mu }\hat{z}_{\nu ]} ), \end{aligned}$$

(74)

$$\begin{aligned} T^{\hat{z}}{_{\mu\nu}}=&\ 2h_9\hat{t}_{[\mu }\hat{s}_{\nu ]}+2h_{10}\hat{t}_{[\mu }\hat{z}_{\nu ]}-2h_{11}\hat{s}_{[\mu }\hat{z}_{\nu ]}. \end{aligned}$$

(75)

Substituting these equations into Eq. (27) and manipulating the result yield

$$\begin{aligned} T^{\lambda \mu \nu }=&\ 2\left(h_7\hat{t}{^\lambda} -h_{12}\hat{s}{^\lambda} -h_9\hat{z}{^\lambda} \right)\hat{t}{^{[\mu}}\hat{s}{^{\nu ]}} \\&-2h_{12}\hat{\phi }{^\lambda} \hat{t}{^{[\mu }}\hat{\phi }{^{\nu ]}}+2\left(h_8\hat{t}{^\lambda} -h_{10}\hat{z}{^\lambda} \right)\hat{t}{^{[\mu }}\hat{z}{^{\nu ]}} \\&+2h_{13}\hat{\phi }^\lambda \hat{s}{^{[\mu }}\hat{\phi }{^{\nu ]}}+2\left(h_9\hat{t}{^\lambda} -h_{14}\hat{s}{^\lambda} +h_{11}\hat{z}{^\lambda} \right)\hat{s}{^{[\mu }}\hat{z}{^{\nu ]}} \\&-2h_{14}\hat{\phi }{^\lambda} \hat{\phi }{^{[\mu }}\hat{z}{^{\nu ]}}. \end{aligned}$$

(76)

Rearranging the terns on the right-hand side of Eq. (76), one can verify that $2T^{[\mu|\lambda|\nu]}=T^{\lambda \mu \nu }$.

From Eqs. (72)–(75), it is possible to show that

$$\begin{aligned} T^{(0)}{_{a\nu}}\hat{t}^a=h_7\hat{s}_\nu +h_8\hat{z}_\nu , \end{aligned}$$

(77)

$$\begin{aligned} T^{\hat{s}}{_{a\nu}}\hat{s}{^a}=h_{12}\hat{t}_\nu -h_{14}\hat{z}_\nu , \end{aligned}$$

(78)

$$\begin{aligned} T^{\hat{\phi}}{_{a\nu}}\hat{\phi }{^a}=h_{12}\hat{t}_\nu -h_{13}\hat{s}_\nu -h_{14}\hat{z}_\nu , \end{aligned}$$

(79)

$$\begin{aligned} T^{\hat{z}}{_{a\nu}}\hat{z}^a=h_{10}\hat{t}_\nu -h_{11}\hat{s}_\nu . \end{aligned}$$

(80)

Using these equations, Eq. (27), and the definition $T_{\nu }=T{^a}{_{a\nu}}$, we obtain

$$\begin{aligned} T^{\nu}=&-(h_{10}+2h_{12})\hat{t}{^\nu} +(h_{17}+h_{13})\hat{s}{^\nu} \\&+(h_8+2h_{14})\hat{z}{^\nu} . \end{aligned}$$

(81)

From this expression and Eq. (32), we find that

$$\begin{aligned} g^{\lambda [\nu }T^{\mu ]}=&\ \left[ -\left(h_{17}+h_{13}\right)\hat{t}{^\lambda} +\left(h_{10}+2h_{12}\right)\hat{s}{^\lambda} \right] \hat{t}{^{[\mu}}\hat{s}{^{\nu ]}} \\&+\left(h_{10}+2h_{12}\right)\hat{\phi }{^\lambda} \hat{t}{^{[\mu}}\hat{\phi }{^{\nu]}} \\&+\left[ -\left(h_8+2h_{14}\right)\hat{t}{^\lambda} +\left(h_{10}+2h_{12}\right)\hat{z}{^\lambda} \right] \hat{t}{^{[\mu}}\hat{z}{^{\nu]}} \\&-\left(h_{17}+h_{13}\right)\hat{\phi }{^\lambda} \hat{s}{^{[\mu}}\hat{\phi }{^{\nu]}} \\&+\left[ \left(h_8+2h_{14}\right)\hat{s}{^\lambda} -\left(h_{17}+h_{13}\right)\hat{z}{^\lambda} \right] \hat{s}{^{[\mu }}\hat{z}{^{\nu ]}} \\&+\left(h_8+2h_{14}\right)\hat{\phi }{^\lambda} \hat{\phi }{^{[\mu}}\hat{z}{^{\nu ]}}. \end{aligned}$$

(82)

Finally, by substituting Eqs. (82) and (76) into Eq. (2) (remember that $2T^{[\mu|\lambda|\nu]}=T^{\lambda \mu \nu }$), we arrive at

$$\begin{aligned} \Sigma ^{\lambda \mu \nu }=&\ \left( -h_{15}\hat{t}{^\lambda} +h_{16}\hat{s}{^\lambda} -h_9\hat{z}{^\lambda} \right) \hat{t}{^{[\mu}}\hat{s}^{\nu ]} +h_{16}\hat{\phi }{^\lambda} \hat{t}{^{[\mu}}\hat{\phi }{^{\nu ]}} \\&+2\left( -h_{14}\hat{t}{^\lambda} +h_{12}\hat{z}{^\lambda} \right) \hat{t}{^{[\mu}}\hat{z}{^{\nu]}}-h_{17}\hat{\phi }{^\lambda} \hat{s}{^{[\mu}}\hat{\phi }{^{\nu]}} \\&+\left( h_9\hat{t}{^\lambda} +h_{18}\hat{s}{^\lambda} -h_{19}\hat{z}{^\lambda} \right) \hat{s}{^{[\mu}}\hat{z}{^{\nu]}}+h_{18}\hat{\phi }{^\lambda} \hat{\phi }{^{[\mu}}\hat{z}{^{\nu]}}. \end{aligned}$$

(83)

To calculate the gravitational angular momentum density, we need the component $\Sigma ^{a0b}$. From Eqs. (83), (61), and Eqs. (38)–(41), we obtain

$$\begin{aligned} \Sigma ^{a0b}=&-\frac{h_6}{A}\left(f\hat{t}{^a}-g\hat{z}{^a}\right)\left [\sin \theta \hat{s}{^b}-\cos \theta \left(g\hat{t}{^b}-f\hat{z}{^b}\right) \right ] \\&+\frac{(\partial _zg)}{2ABf}\left(\hat{s}{^a}\hat{s}{^b}+\hat{\phi }{^a}\hat{\phi }{^b}\right), \end{aligned}$$

(84)

where we have used the identities $fh_{16}-gh_{18}=({\partial }{_z}g)/(fB)$, $fh_{15}+gh_9=2fh_6\sin \theta$ and $gh_{19}-fh_9=2gh_6\sin \theta$.

To evaluate $\Sigma ^{\lambda \mu \nu }T_{\lambda\mu\alpha}$, it is convenient to proceed in the following manner: Let X, Y, and Z be elements in $\{\hat{t}{^a},\hat{s},\hat{\phi },\hat{z}\}$; assume that X is different from both Y and Z. From the orthonormality condition, we see that

$$\begin{aligned} X^{[\mu }Y^{\nu ]}X_{[\mu }Z_{\alpha ]}=\frac{1}{4}(X^aX_a)Y^\nu Z_\alpha +\frac{1}{4}(Y^aZ_a)X^\nu X_\alpha . \end{aligned}$$

(85)

We can use this identity to obtain, after a lengthy calculation, the expression

$$\begin{aligned} \Sigma ^{\lambda \mu \nu }T_{\lambda \mu \alpha}=&\ \left(\frac{h_9^2}{2}+\frac{h_7h_{15}}{2}+h_8h_{14}-h_{12}^2-2h_{10}h_{12}\right)\hat{t}{^\nu} \hat{t}_\alpha \\&+\frac{1}{2}\Bigl(-2h_9^2-h_7h_{15}-h_{14}h_{18}+h_{12}h_{16} \\&-h_{13}h_{17}-h_{11}h_{19} \Bigr)\hat{s}{^\nu} \hat{s}_\alpha +\frac{1}{2}\bigl(h_{12}h_{16} \\&-h_{13}h_{17}-h_{14}h_{18}\bigr )\hat{\phi }{^\nu} \hat{\phi }_\alpha -\Biggl(\frac{h_9^2}{2}+\frac{h_{11}h_{19}}{2} \\&+h_{14}^2+2h_8h_{14}-h_{10}h_{12} \Biggr)\hat{z}{^\nu} \hat{z}_\alpha +\Biggl(\frac{h_{13}h_{16}}{2} \\&+h_9h_{14}+h_{11}h_{12}\Biggr)\hat{t}{^\nu} \hat{s}_\alpha +\frac{1}{2}\bigl(h_{12}h_{17}-h_8h_9 \\&+h_{10}h_{19}\bigr)\hat{s}^\nu \hat{t}_\alpha +\left(h_{14}h_{16}-\frac{h_9h_{13}}{2}\right)\hat{t}{^\nu} \hat{z}_\alpha \\&+\left(h_{12}h_{18}-\frac{h_9h_{13}}{2}\right)\hat{z}^\nu \hat{t}_\alpha -\frac{1}{2}\bigl(h_8h_{15}+h_{10}h_9 \\&+h_{14}h_{17}\bigr)\hat{s}^\nu \hat{z}_\alpha +\Biggl(-h_7h_{14}+h_9h_{12} \\&-\frac{h_{13}h_{18}}{2}\Biggr)\hat{z}^\nu \hat{s}_\alpha \end{aligned}$$

(86)

Contracting $\nu$ with $\alpha$ and using (61) to eliminate $h_{18}$ and $h_{16}$, we find that

$$\begin{aligned} T=&\ 2h_9^2+h_7h_{15}+4h_8h_{14}+2h_{14}^2-2h_{12}^2 \\&-4h_{10}h_{12}+h_{13}h_{17}+h_{11}h_{19}. \end{aligned}$$

(87)