Analysis of Dynamic Behavior of V-Shaped Atomic Force Microscope Cantilever in Contact and Tapping Modes by Considering Various Immersion Surroundings

Abstract

In atomic force microscope (AFM) as a powerful device for scanning the samples, a cantilever is used to sense the variations of its dynamic characteristics due to the tip–sample interaction. Theoretical models that can accurately simulate the surface-coupled dynamics of the cantilever are necessary for quantifiable and qualitative explanation and understanding of measured results. A good understanding of the dynamics of AFM cantilevers vibrating in liquid is needed for the interpretation of scanning images, selection of AFM operating conditions and evaluation of sample’s mechanical properties. In this paper, the amplitude of frequency response functions (FRF) of vertical and rotational movements for a V-shaped atomic force microscope beam immersed in various surroundings has been surveyed for the first time. For rising the precision of theoretical model, we have considered all needful details for the beam and sample. The amplitude of FRF of vertical and rotational movements and resonant frequency of beam have been surveyed by supposing cantilever thickness, breadth and length, the angle between cantilever and sample surface, tip height and normal and lateral tip–sample interaction force. In this study, carbon tetrachloride (CCL₄), methanol, acetone, water and air have been considered as surroundings. The results show that an increase in the tip–sample interaction force considerably raises the amount of resonant frequency. By increasing the liquid viscosity, the amplitude of FRF for vertical and rotational movements and resonant frequency reduce. Moreover, the amplitude of FRF of vertical and rotational movements is reduced by raising the rectangular and tapered parts lengths, but increased by raising the rectangular part breadth and cantilever thickness. The resonant frequency goes down by increasing the rectangular and tapered parts lengths and rectangular part breadth, but increases by rising the cantilever thickness. In this paper, we have tried to model the realistic dynamic behavior of V-shaped AFM beam in contact and tapping modes with the most accuracy. Results show good agreement. Based on the experimental results, the present theoretical model can forestall better the realistic behavior of tapping mode than the contact mode for V-shaped AFM cantilever. Based on the complexity of the V-shaped AFM cantilever, this study has been done for the first time in both of the contact and tapping modes.

Appendices

Appendix A: The Boundary Conditions of the Cantilever

Here, the boundary conditions of the cantilever have been brought.

Two various tip–specimen attraction regimes, attractive and repulsion, are distinguished in the normal direction of the cantilever. By considering the supposition of the Hertzian contact principle [17, 18] and applying a Taylor expansions [2],

$$ \begin{aligned} f_{n} & = \left( {k_{n} - k_{n1} \frac{w(0,t)\cos (\alpha )}{{\delta_{0} }} - k_{n2} \frac{{\left( {w(0,t)\cos (\alpha )} \right)^{2} }}{{\delta_{0}^{2} }}} \right)\left( {w(0,t)\cos (\alpha ) - H\varphi (0,t)\sin (\alpha )} \right), \\ k_{n} & = \frac{3}{2}K_{0} \delta^{1/2} ,k_{n1} = \frac{1}{4}k_{n} ,k_{n2} = \frac{1}{24}k_{n} ,K_{0} = \frac{4}{3}E^{ * } \sqrt {R_{t} } ,\frac{1}{{E^{ * } }} = \frac{{1 - \nu_{s}^{2} }}{{E_{s} }} + \frac{{1 - \nu_{t}^{2} }}{{E_{t} }}, \\ \delta_{0} & = Z_{0} - D \\ \end{aligned} $$

(A.1)

$$ \begin{aligned} & f_{t} = \left( {k_{t} - k_{t1} \left( {\frac{w(0,t)\cos (\alpha )}{{\delta_{0} }}} \right) - k_{t2} \left( {\frac{w(0,t)\cos (\alpha )}{{\delta_{0} }}} \right)^{2} } \right)\left( {w(0,t)\sin (\alpha )} \right. \\ & \quad \quad \left. +\,{ H\varphi (0,t)\cos (\alpha )} \right),k_{t} = 8G_{0} \delta_{0}^{1/2} ,k_{t1} = \frac{1}{2}k_{t} ,k_{t2} = \frac{1}{8}\,k_{t} ,G_{0} = G^{ * } \sqrt {R_{t} } , \\ & \quad \quad \;\;\frac{1}{{G^{ * } }} = \frac{{2 - \nu_{s} }}{{G_{s} }} + \frac{{2 - \nu_{t} }}{{G_{t} }}. \\ \end{aligned} $$

(A.2)

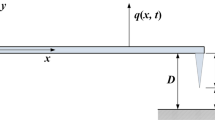
$R_{t}$ is the tip radius and $E_{t} ,E_{s} ,\,\nu_{t} \,{\text{and}}\,\nu_{s}$ are Young’s modulus and Poisson’s ratio of the tip and sample, respectively.$\delta_{0} ,D\,{\text{and}}\,Z_{0}$ are the static contact deformation or sometimes difference between intermolecular distance and equilibrium tip–sample separation, static deflection and surface offset, respectively. By considering the position of the beam, the boundary conditions can be expressed as:

$$ v( - L_{0} ,t) = 0 $$

(A.3)

$$ \psi ( - L_{0} ,t) = 0, $$

(A.4)

$$ v(0,t) = w(0,t), $$

(A.5)

$$ \psi (0,t) = \phi (0,t), $$

(A.6)

$$ I_{y0} \frac{\partial \psi (0,t)}{\partial x} - I_{y0}^{\prime } \frac{\partial \phi (0,t)}{\partial x} = 0, $$

(A.7)

$$ kGA_{0} \left( {\frac{\partial v(0,t)}{\partial x} - \psi (0,t)} \right) - kGA_{0}^{\prime } \left( {\frac{\partial w(0,t)}{\partial x} - \phi (0,t)} \right) = 0, $$

(A.8)

$$ \begin{aligned} & EI_{y0}^{\prime } \left( {1 - C_{b} } \right)\left( {1 - C_{h} } \right)^{3} \frac{{\partial \varphi (L_{1} ,t)}}{\partial x} = \\ & \quad \left( { - f_{t} \cos (\alpha ) + f_{n} \sin (\alpha )} \right)H - m_{\text{tip}} d^{2} \frac{{\partial^{2} \varphi (L_{1} ,t)}}{{\partial t^{2} }} = \left( { - \left( {k_{t} - k_{t1} \frac{{w(L_{1} ,t)\cos (\alpha )}}{{\delta_{0} }}} \right.} \right. \\ & \quad \left. { - \,k_{t2} \left( {\frac{{w(L_{1} ,t)\cos (\alpha )}}{{\delta_{0} }}} \right)^{2} } \right)\,\left( {\frac{H}{2}w(L_{1} ,t)\sin (2\alpha ) + H^{2} \varphi (L_{1} ,t)\cos^{2} (\alpha )} \right) + \left( {k_{n} - \,k_{n1} \,\frac{{w(L_{1} ,t)}}{{\delta_{0} }}\cos (\alpha )} \right. \\ & \quad \left. { - \,k_{n2} \frac{{w(L_{1} ,t)^{2} }}{{\delta_{0}^{2} }}\cos^{2} (\alpha )} \right)\,\left. {\left( {\frac{H}{2}w(L_{1} ,t)\sin (2\alpha ) - H^{2} \varphi (L_{1} ,t)\sin^{2} (\alpha )} \right)} \right) - m_{\text{tip}} d^{2} \frac{{\partial^{2} \varphi (L_{1} ,t)}}{{\partial t^{2} }}, \\ \end{aligned} $$

(A.9)

$$ \begin{aligned} & kGA_{0}^{\prime } \,\left( {1 - C_{b} } \right)\,\left( {1 - C_{h} } \right)\,\left( {\varphi (L_{1} ,t) - \frac{{\partial w(L_{1} ,t)}}{\partial x}} \right) = \\ & \quad \left( {f_{t} \,\sin \,(\alpha )\, + \,f_{n} \,\cos \,(\alpha )} \right) + m_{\text{tip}} \frac{{\partial^{2} w(L_{1} ,t)}}{{\partial t^{2} }} = \,\left( {k_{t} \,} \right. - \,k_{t1} \,\frac{{w(L_{1} ,t)\cos (\alpha )}}{{\delta_{0} }}\left. { - \,k_{t2} \left( {\frac{{w(L_{1} ,t)\cos (\alpha )}}{{\delta_{0} }}} \right)^{2} } \right) \\ & \quad \left( {w(L_{1} ,t)\sin^{2} (\alpha ) + \frac{H}{2}\varphi (L_{1} ,t)\sin \,(2\alpha )} \right) + \left( {k_{n} - \,k_{n1} \,\frac{{w(L_{1} ,t)}}{{\delta_{0} }}\cos (\alpha )\,} \right.\left. { - \,k_{n2} \frac{{w(L_{1} ,t)^{2} }}{{\delta_{0}^{2} }}\cos^{2} (\alpha )} \right) \\ & \quad \left( {w(L_{1} ,t)\cos^{2} (\alpha ) - \frac{H}{2}\varphi (L_{1} ,t)\sin \,(2\alpha )} \right) + m_{\text{tip}} \frac{{\partial^{2} w(L_{1} ,t)}}{{\partial t^{2} }}. \\ \end{aligned} $$

(A.10)

Appendix B: Modeling by Finite Element Method (FEM)

For the forces and moments, it can be said that:

$$ F_{\text{ts}} = G_{t}^{T} f_{\text{ts}}^{C} = - K_{T - S} w, $$

(B.1)

$$ f_{\text{ts}} = \{ f_{y}^{C} ,M_{z}^{C} \}^{T}, $$

(B.2)

where $G_{t}^{T}$ is the Kronecker matrix displaying the location of the nodal movements at node C in the total movement vector. $K_{T - S}$ is the matrix stiffness coefficient of interaction force. The shear force and moment at node C can be rewritten as:

$$ f_{y}^{C} = f_{t} \text{Sin} (\alpha ) + f_{n} \text{Cos} (\alpha ),M_{z}^{C} = H( - f_{t} \text{Cos} (\alpha ) + f_{n} \text{Sin} (\alpha )). $$

(B.3)

By supposing the linear tip–sample interaction force and using Eqs. (A.9) and (A.10) also [12]:

$$ \left[ \begin{aligned} f_{y} \hfill \\ M_{z} \hfill \\ \end{aligned} \right] = - [k{}_{\text{ts}}]\left[ \begin{aligned} w \hfill \\ \varphi \hfill \\ \end{aligned} \right], $$

(B.4)

$$ [k{}_{ts}] = \left[ {\begin{array}{*{20}l} {k_{n} \text{Cos}^{2} (\alpha ) + k_{t} \text{Sin}^{2} (\alpha )} \hfill & {H\text{Cos} (\alpha )\text{Sin} (\alpha )\left( {k_{t} - k_{n} } \right)} \hfill \\ {H\text{Cos} (\alpha )\text{Sin} (\alpha )\left( {k_{t} - k_{n} } \right)} \hfill & {H^{2} \left( {k_{n} \text{Sin}^{2} (\alpha ) + k_{t} \text{Cos}^{2} (\alpha )} \right)} \hfill \\ \end{array} } \right], $$

(B.5)

where $k_{n}$ and $k_{t}$ are found from Eqs. (A.1) and (A.2). By considering the harmonic excitation for holder $q_{z} (t) = h_{q} \,e^{i\,\omega \,t}$ and cantilever displacement $w(t) = W\,e^{i\,\omega \,t}$ and harmonic interaction force:

$$ \begin{aligned} & \left( {(K + K_{T - S} ) + i\omega (C + C_{a} ) - (M + M_{a} )\omega^{2} } \right)W = \\ & \quad (M + M_{a} )\omega^{2} I_{z} h_{q} - i\omega C_{a} I_{z} h_{q} - \,K_{T - S} I_{z} h_{q} , \\ \end{aligned}$$

(B.6)

now FRF vector of the beam is rewritten as:

$$ \begin{aligned} & \left. {{\text{FRF}}(\omega )} \right|_{{{\text{Liquid/Contact}}\,{\text{Mode}}}} = \frac{W}{{h_{q} }} = \\ & \quad \left( {(K + K_{T - S} ) + i\omega (C + C_{a} ) - (M + M_{a} )\omega^{2} } \right)^{ - 1} \left( {(M + M_{a} )\omega^{2} - i\omega C_{a} - K_{T - S} } \right)I_{z} \\ \end{aligned}. $$

(B.7)

When the AFM cantilever is vibrating away from the surface $F_{\text{ts}} = 0$, we have:

$$ \begin{aligned} & \left. {{\text{FRF}}(\omega )} \right|_{{{\text{Liquid/Non-Contact}}\,{\text{Mode}}}} = \frac{W}{{h_{q} }} = \\ & \quad \left( {K + i\omega (C + C_{a} ) - (M + M_{a} )\omega^{2} } \right)^{ - 1} \left( {(M + M_{a} )\omega^{2} - i\omega \,C_{a} } \right)\,I_{z}. \\ \end{aligned} $$

(B.8)

For air as the environment, the hydrodynamic damping force can be neglected, so Eqs. (B.7) and (B.8) may be written as:

$$ \begin{aligned} & \left. {{\text{FRF}}(\omega )} \right|_{{{\text{Air/Contact}}\,{\text{Mode}}}} = \frac{W}{{h_{q} }} = \\ & \quad \left( {(K + K_{T - S} ) + i\omega C - M\omega^{2} } \right)^{ - 1} \left( {M\omega^{2} - K_{T - S} } \right)I_{z} \\ \end{aligned}, $$

(B.9)

$$ \left. {{\text{FRF}}(\omega )} \right|_{{{\text{Air/Non-Contact}}\,{\text{Mode}}}} = \frac{W}{{h_{q} }} = \left( {K + i\omega \,C - M\omega^{2} } \right)^{ - 1} \left( {M\omega^{2} } \right)I_{z}. $$

(B.10)

Appendix C: Timoshenko Beam Model

Here, mass, stiffness and damping matrices are introduced using Timoshenko beam principle [19] (Fig. 14):

$$ \begin{aligned} & [k]_{e} = \\ & \frac{{2\,\overline{EI} }}{{(1 + \varphi )\,L^{3} }}\left[ {\begin{array}{*{20}c} {L^{2} (2 + A_{1} + \varphi /2)} & {L^{2} (1 - \varphi /2)} & { - L(3 + A_{1} )} & { - L(3 + A_{1} )} \\ {} & {L^{2} (2 - A_{1} + \varphi /2)} & { - L(3 - A_{1} )} & { - L(3 - A_{1} )} \\ {} & {} & 6 & 6 \\ {\text{Sym}} & {} & {} & 6 \\ \end{array} } \right], \\ \end{aligned} $$

(C.1)

$$ [m]_{e} = [m_{t} ]_{e} + [m_{r} ]_{e}, $$

(C.2)

where $[m_{t} ]_{e}$ and $[m_{\gamma } ]_{e}$ represent the mass matrix for shear inertia and rotatory inertia effects, respectively.

$$ [m_{t} ]_{e} = \left[ {\begin{array}{*{20}c} {t_{11} } & {t_{12} } & {t_{13} } & {t_{14} } \\ {} & {t_{22} } & {t_{23} } & {t_{24} } \\ {} & {} & {t_{33} } & {t_{34} } \\ {\text{Sym}} & {} & {} & {t_{44} } \\ \end{array} } \right], $$

(C.3)

$$ \begin{aligned} t_{11} & = \frac{{\rho A_{i} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{240} + \frac{\varphi }{105} + \frac{1}{168}} \right) + \frac{{\rho A_{j} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{240} + \frac{\varphi }{140} + \frac{1}{280}} \right), \\ t_{12} & = \frac{{ - \rho A_{i} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{240} + \frac{\varphi }{120} + \frac{1}{280}} \right) - \frac{{\rho A_{j} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{240} + \frac{\varphi }{120} + \frac{1}{280}} \right), \\ t_{13} & = \frac{{ - \rho A_{i} L^{2} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{40} + \frac{5\varphi }{84} + \frac{1}{28}} \right) - \frac{{\rho A_{j} L^{2} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{60} + \frac{9\varphi }{280} + \frac{1}{60}} \right), \\ t_{14} & = \frac{{\rho A_{i} L^{2} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{60} + \frac{9\varphi }{280} + \frac{1}{70}} \right) + \frac{{\rho A_{j} L^{2} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{40} + \frac{3\varphi }{70} + \frac{1}{60}} \right), \\ t_{22} & = \frac{{\rho A_{i} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{240} + \frac{\varphi }{140} + \frac{1}{280}} \right) + \frac{{\rho A_{j} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{240} + \frac{\varphi }{105} + \frac{1}{168}} \right), \\ t_{23} & = \frac{{\rho A_{i} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{40} + \frac{3\varphi }{70} + \frac{1}{60}} \right) + \frac{{\rho A_{j} L^{3} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{60} + \frac{9\varphi }{280} + \frac{1}{70}} \right), \\ t_{24} & = \frac{{ - \rho A_{i} L^{2} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{60} + \frac{9\varphi }{280} + \frac{1}{60}} \right) - \frac{{\rho A_{j} L^{2} }}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{40} + \frac{5\varphi }{84} + \frac{1}{28}} \right), \\ t_{33} & = \frac{{\rho A_{i} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{4} + \frac{8\varphi }{15} + \frac{1}{7}} \right) + \frac{{\rho A_{j} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} + \frac{\varphi }{6} + \frac{3}{35}} \right), \\ t_{34} & = \frac{{ - \rho A_{i} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} + \frac{3\varphi }{20} + \frac{9}{140}} \right) - \frac{{\rho A_{j} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} + \frac{3\varphi }{20} + \frac{9}{140}} \right), \\ t_{44} & = \frac{{\rho A_{i} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} + \frac{\varphi }{6} + \frac{3}{35}} \right) + \frac{{\rho A_{j} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{4} + \frac{8\varphi }{15} + \frac{2}{7}} \right), \\ \end{aligned} $$

(C.4)

$$ [m_{r} ]_{e} = \left[ {\begin{array}{*{20}c} {r_{11} } & {r_{12} } & {r_{13} } & {r_{14} } \\ {} & {r_{22} } & {r_{23} } & {r_{24} } \\ {} & {} & {r_{33} } & {r_{34} } \\ {\text{Sym}} & {} & {} & {r_{44} } \\ \end{array} } \right], $$

(C.5)

$$ \begin{aligned} r_{11} & = \frac{{\rho I_{i} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{4} + \frac{\varphi }{5} + \frac{1}{10}} \right) + \frac{{\rho I_{j} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} - \frac{\varphi }{30} + \frac{1}{30}} \right), \\ r_{12} & = \frac{{\rho I_{i} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} - \frac{\varphi }{12} - \frac{1}{60}} \right) + \frac{{\rho I_{j} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} - \frac{\varphi }{12} - \frac{1}{60}} \right), \\ r_{22} & = \frac{{\rho I_{i} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{12} - \frac{\varphi }{30} + \frac{1}{30}} \right) + \frac{{\rho I_{j} L}}{{(1 + \varphi )^{2} }}\left( {\frac{{\varphi^{2} }}{4} + \frac{\varphi }{5} + \frac{1}{10}} \right), \\ r_{13} & = r_{14} = \frac{{\rho I_{i} }}{{(1 + \varphi )^{2} }}\left( {\frac{3\varphi }{10}} \right) + \frac{{\rho I_{j} }}{{(1 + \varphi )^{2} }}\left( {\frac{\varphi }{5} - \frac{1}{10}} \right), \\ r_{23} & = r_{24} = \frac{{\rho I_{i} }}{{(1 + \varphi )^{2} }}\left( {\frac{\varphi }{5} - \frac{1}{10}} \right) + \frac{{\rho I_{j} }}{{(1 + \varphi )^{2} }}\left( {\frac{3\varphi }{10}} \right), \\ r_{33} & = r_{34} = r_{44} = \frac{{\rho I_{i} }}{{L(1 + \varphi )^{2} }}\left( {\frac{3}{5}} \right) + \frac{{\rho I_{j} }}{{L(1 + \varphi )^{2} }}\left( {\frac{3}{5}} \right), \\ \end{aligned} $$

(C.6)

where $\varphi = \frac{12\,EI}{{kGA\,L^{2} }},\,A_{i} = \frac{{EI_{i} - EI_{j} }}{{EI_{i} + EI_{j} }},\,\,\,\overline{EI} = (EI_{i} + EI_{j} )/2.$ We employ the proportional damping matrix as [19]:

$$ C = \phi^{ - T} C_{\text{diagonl}} \phi^{ - 1} ,C_{\text{diagonl}} = {\text{diag}}\left( {\frac{{\omega_{1} }}{{Q_{1} }},\frac{{\omega_{2} }}{{Q_{2} }}, \ldots ,\frac{{\omega_{n} }}{{Q_{n} }}} \right),\;\phi = [\phi_{1} ,\phi_{2} , \ldots ,\phi_{n} ]. $$

(C.7)

Fig. 14

Tapered element