Periodic inventory model with controllable lead time and back order discount for decaying items

Abstract

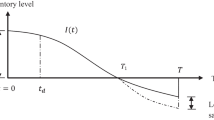
This study investigates the periodic inventory model for decaying goods that includes price discounts for backorders and considers that only a small portion of shortages are backlogged. The effect of managing lead time as a determining factor in the periodic inventory model. The present model develops a stochastic inventory model which together optimizes the backorder price discount, review period and the lead time for decaying items. During the protection interval, the demand has been looked at in two different ways: when demand distribution is known or not. The backorder ratio is influenced by price discount, and the suggested model assumes a constant deterioration rate. Numerical results show that a controlled lead time can yield significant savings. As far as the author knows, this is the first study to examine periodically deteriorating items with controlled lead times due to backorder discounts. On the other hand, as the products deteriorate at a faster pace, the overall annual cost rises. This research can help with the development of strategies for efficient inventory management of perishable commodities with backorder price reductions.

Appendices

Appendix 1

Proof of $TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)$ is concave in $L \in \left( {\Gamma_{i} ,\Gamma_{i - 1} } \right)$ in for set $\tau ,\mu_{x}$.

$$\frac{{\partial TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{\partial \Gamma } = \frac{1}{T}\left[ \begin{gathered} \sigma \psi \left( {F_{s} } \right)\left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \alpha_{0} \mu_{x} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right\}\frac{{\left( {\tau + \Gamma } \right)^{{ - \frac{1}{2}}} }}{2} \hfill \\ + h_{c} \left\{ {\frac{{\left( {\tau + \Gamma } \right)^{{ - \frac{1}{2}}} }}{2} + \left( {1 - \frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right)\sigma \frac{{\left( {\tau + \Gamma } \right)^{{ - \frac{1}{2}}} }}{2}\psi \left( {F_{s} } \right)} \right\} \hfill \\ \end{gathered} \right]$$

(19)

$$\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \Gamma^{2} }} = \frac{ - 1}{{4\tau }}\left[ \begin{gathered} \sigma \psi \left( {F_{s} } \right)\left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \alpha_{0} \mu_{x} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right\}\left( {\tau + \Gamma } \right)^{{ - \frac{3}{2}}} \hfill \\ - \frac{{h_{c} }}{4}\left\{ {\left( {\tau + \Gamma } \right)^{{ - \frac{3}{2}}} + \left( {1 - \frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right)\sigma \frac{{\left( {\tau + \Gamma } \right)^{{ - \frac{3}{2}}} }}{2}\psi \left( {F_{s} } \right)} \right\} \hfill \\ \end{gathered} \right] < 0$$

(20)

Therefore, TEC is concave $\Gamma \in \left( {\Gamma_{i} ,\Gamma_{i - 1} } \right)$ in for set $\tau$ and $\mu_{x}$.

Appendix 2

For a value of $\Gamma \in \left( {\Gamma_{i} ,\Gamma_{i - 1} } \right)$, find the Hassian Matrix H as

$$H = \left[ {\begin{array}{*{20}c} {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau^{2} }}} & {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau \mu_{x} }}} \\ {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau \mu_{x} }}} & {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \mu_{x}^{2} }}} \\ \end{array} } \right]$$

∴, Ist principal minor of $H$ is given below.

$$\left| H \right| = \frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau^{2} }}$$

$$TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right) = \left[ \begin{gathered} \frac{{\sigma \sqrt {\left( {\tau + \Gamma } \right)} \psi \left( {F_{s} } \right)\left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \alpha_{0} \mu_{x} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right\}}}{\tau } \hfill \\ + h_{c} \left\{ {\frac{\lambda T}{2} + F_{s} \sigma \sqrt {\left( {\tau + \Gamma } \right)} + \left( {1 - \frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right)\sigma \sqrt {\left( {\tau + \Gamma } \right)} \psi \left( {F_{s} } \right)} \right\} \hfill \\ + \frac{{K + \sum\limits_{j = 1}^{i} {c_{j} } }}{\tau } + \,\,\frac{1}{\tau }\left[ {\frac{\lambda + \theta Z}{\theta }\left( {\theta \tau - 1} \right) - \lambda \tau } \right] \hfill \\ \end{gathered} \right]$$

(21)

$$\frac{{\partial TEC^{w} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{\partial \tau } = \left[ \begin{gathered} - \frac{{\sigma \sqrt {\left( {\tau + \Gamma } \right)} \psi \left( {F_{s} } \right)\left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }} - \alpha_{0} \mu_{x} } \right\}}}{{\tau^{2} }} \hfill \\ + \frac{{\frac{\sigma }{2}\frac{1}{{\sqrt {\left( {\tau + \Gamma } \right)} }}\psi \left( {F_{s} } \right)\left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }} - \alpha_{0} \mu_{x} } \right\}}}{\tau } \hfill \\ + h_{c} \left\{ {\frac{\lambda \tau }{2} + \frac{{F_{s} \sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 1}{2}}} }}{2} + \left( {1 - \frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right)\sigma \frac{{\left( {\tau + \Gamma } \right)^{{ - \frac{1}{2}}} }}{2}\psi \left( {F_{s} } \right)} \right\} \hfill \\ \,\, - \frac{{K + \sum\limits_{j = 1}^{i} {c_{j} } }}{{\tau^{2} }} + \frac{\lambda + \theta Z}{\theta }\left[ {\frac{{e^{\theta T} \left( {\theta T - 1} \right)}}{\tau }} \right] + \frac{\lambda + \theta Z}{{\theta \tau^{2} }} \hfill \\ \end{gathered} \right]$$

(22)

Equation (22) can be written as

$$\begin{gathered} \frac{{K + \sum\limits_{j = 1}^{i} {c_{j} } }}{{\tau^{2} }} + \frac{{\sigma \sqrt {\left( {\tau + \Gamma } \right)} \psi \left( {F_{s} } \right)N\left( {\mu_{x} } \right)}}{{\tau^{2} }} = \left[ \begin{gathered} \frac{{\frac{\sigma }{2}\frac{{N\left( {\mu_{x} } \right)}}{{\sqrt {\left( {\tau + \Gamma } \right)} }}\psi \left( {F_{s} } \right)}}{\tau } + \,\frac{\lambda + \theta Z}{\theta }\left[ {\frac{{e^{\theta \tau } \left( {\theta \tau - 1} \right)}}{\tau }} \right] + \frac{\lambda + \theta Z}{{\theta \tau^{2} }} \hfill \\ + h_{c} \left\{ {\frac{\lambda \tau }{2} + \frac{{F_{s} \sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 1}{2}}} }}{2} + \left( {1 - \frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}} \right)\sigma \frac{{\left( {\tau + \Gamma } \right)^{{ - \frac{1}{2}}} }}{2}\psi \left( {F_{s} } \right)} \right\} \hfill \\ \end{gathered} \right]\;\;{\text{Where}} \hfill \\ N\left( {\mu_{x} } \right) = \left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }} - \alpha_{0} \mu_{x} } \right\} \hfill \\ \end{gathered}$$

(23)

$$\begin{gathered} \frac{{\partial^{2} TEC^{w} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau^{2} }} = \left[ \begin{gathered} \frac{{\sigma \left( {3\tau + 4\Gamma } \right)\psi \left( {F_{s} } \right)N\left( {\mu_{x} } \right)}}{{2\tau^{3} \sqrt {\left( {\tau + \Gamma } \right)} }} + \frac{{\sigma \left( {3\tau + 2\Gamma } \right)N\left( {\mu_{x} } \right)\psi \left( {F_{s} } \right)}}{{4\sqrt {\left( {\tau + \Gamma } \right)} }} \hfill \\ - h_{c} \frac{{F_{s} \sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} }}{4} - h_{c} \frac{{\psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} }}{4} + \frac{1}{4}\frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}\psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} \hfill \\ \,\, + \frac{{2\left( {K + \sum\limits_{j = 1}^{i} {c_{j} } } \right)}}{{\tau^{3} }} + \frac{\lambda + \theta Z}{\theta }\left[ {\theta^{2} e^{\theta \tau } - \frac{{e^{\theta \tau } \left( {\theta \tau - 1} \right)}}{{\tau^{2} }} - \frac{2}{{\tau^{3} }}} \right] \hfill \\ \end{gathered} \right]\;\;{\text{Where}} \hfill \\ N\left( {\mu_{x} } \right) = \left\{ {\frac{{\alpha_{0} \mu_{x}^{2} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} \mu_{x} }}{{\mu_{0} }} - \alpha_{0} \mu_{x} } \right\} \hfill \\ \end{gathered}$$

(24)

$$\frac{{\partial^{2} TEC^{w} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau^{2} }} = \psi \left( T \right) + \frac{{h_{c} \sigma }}{4}\left[ {F_{s} \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} + \psi \left( {F_{s} } \right)\left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} } \right]$$

where,

$$\psi \left( \tau \right) = \left[ \begin{gathered} \frac{{\sigma \left( {3\tau + 4\Gamma } \right)\psi \left( {F_{s} } \right)N\left( {\mu_{x} } \right)}}{{2\tau^{3} \sqrt {\left( {\tau + L} \right)} }} + \frac{{\sigma \left( {3\tau + 2\Gamma } \right)N\left( {\mu_{x} } \right)\psi \left( {F_{s} } \right)}}{{4\sqrt {\left( {\tau + \Gamma } \right)} }} \hfill \\ + \frac{{\sigma \left( {3\tau + 2\Gamma } \right)\psi \left( {F_{s} } \right)N\left( {\mu_{x} } \right)}}{{4\sqrt {\left( {\tau + L} \right)} }} + \frac{1}{4}\frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}\psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} \hfill \\ \,\, + \frac{{2\left( {K + \sum\limits_{j = 1}^{i} {c_{j} } } \right)}}{{\tau^{3} }} + \frac{\lambda + \theta Z}{\theta }\left[ {\theta^{2} e^{\theta \tau } - \frac{{e^{\theta \tau } \left( {\theta \tau - 1} \right)}}{{\tau^{2} }} - \frac{2}{{\tau^{3} }}} \right] \hfill \\ \end{gathered} \right]$$

(25)

Now

$$\left| {H_{11} } \right| = \psi \left( \tau \right) - \frac{{h_{c} \sigma }}{4}\left[ {F_{s} \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} + \psi \left( {F_{s} } \right)\left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} } \right]$$

$$\sigma \left( \tau \right) = \left[ \begin{gathered} \,\,\frac{{\left( {K + \sum\limits_{j = 1}^{i} {c_{j} } } \right)}}{{\tau^{2} }} + \frac{{\sigma \sqrt {\left( {\tau + \Gamma } \right)} \psi \left( {F_{s} } \right)N\left( {\mu_{x} } \right)}}{{\tau^{2} }} \hfill \\ - \frac{{\frac{\sigma }{2}\frac{1}{{\sqrt {\left( {\tau + \Gamma } \right)} }}N\left( {\mu_{x} } \right)\psi \left( {F_{s} } \right)}}{\tau } + \frac{\lambda + \theta Z}{\theta }\left[ {\theta^{2} e^{\theta \tau } - \frac{{e^{\theta \tau } \left( {\theta \tau - 1} \right)}}{{\tau^{2} }} - \frac{2}{{\tau^{3} }}} \right] \hfill \\ \end{gathered} \right]$$

Then

$$\sigma \left( \tau \right) > \frac{{h_{c} \sigma }}{4}\left[ {F_{s} \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} + \psi \left( {F_{s} } \right)\left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} } \right]$$

(26)

So from above we have

$$\left| {H_{11} } \right| > \psi \left( \tau \right) - \sigma \left( \tau \right) > 0$$

This implies $\mu_{x} < \left( {s_{c} + \mu_{o} } \right)$(therefore, $\mu_{x} < \mu_{o}$ and $s > 0$ which holds.

Therefore, $\left| {H_{11} } \right| > 0$

$$\frac{{\partial TEC^{w} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{\partial \tau } = \left[ \begin{gathered} - \frac{{\sigma \sqrt {\left( {\tau + \Gamma } \right)} \psi \left( {F_{s} } \right)\left\{ {\frac{{2\alpha_{0} \mu_{x} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} }}{{\mu_{0} }} - \alpha_{0} } \right\}}}{{\tau^{2} }} \hfill \\ + \frac{{\frac{\sigma }{2}\frac{1}{{\sqrt {\left( {\tau + \Gamma } \right)} }}\psi \left( {F_{s} } \right)\left\{ {\frac{{2\alpha_{0} \mu_{x} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} }}{{\mu_{0} }} - \alpha_{0} } \right\}}}{\tau } \hfill \\ - \left\{ {\frac{{h_{c} \alpha_{0} \psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 1}{2}}} }}{{\mu_{0} }}} \right\} \hfill \\ \end{gathered} \right]$$

(27)

$$\frac{{\partial TEC^{w} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \mu_{x} }} = \left[ {\frac{{\left\{ {\frac{{2\alpha_{0} \mu_{x} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} }}{{\mu_{0} }} - \alpha_{0} } \right\}}}{\tau } - \left\{ {\frac{{h_{c} \alpha_{0} \psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{\frac{1}{2}} }}{{\mu_{0} }}} \right\}} \right]$$

(28)

$$\frac{{\partial^{2} TEC^{w} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \mu_{x}^{2} }} = \frac{{2\alpha_{0} }}{{\mu_{o} }}$$

(29)

Similarly.

The 2nd minor principal of $H$ to be as follows

$$\left| {H_{22} } \right| = \left[ {\begin{array}{*{20}c} {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau^{2} }}} & {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau \mu_{x} }}} \\ {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau \mu_{x} }}} & {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \mu_{x}^{2} }}} \\ \end{array} } \right]$$

$$\left| {H_{22} } \right| = \frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \tau^{2} }} \times \frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \mu_{x}^{2} }} - \left( {\frac{{\partial^{2} TEC^{W} \left( {\tau ,\mu_{x} ,\Gamma } \right)}}{{\partial \mu_{x} \partial \tau }}} \right)^{2}$$

$$\begin{gathered} = \left[ \begin{gathered} \frac{{\sigma \left( {3\tau + 4\Gamma } \right)\psi \left( {F_{s} } \right)N\left( {\mu_{x} } \right)}}{{2\tau^{3} \sqrt {\left( {\tau + \Gamma } \right)} }} + \frac{{\sigma \left( {3\tau + 2L} \right)N\left( {\mu_{x} } \right)\psi \left( {F_{s} } \right)}}{{4\sqrt {\left( {\tau + \Gamma } \right)} }} \hfill \\ - \frac{{h_{c} \sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} F_{s} }}{4} - \frac{{h_{c} \sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} \psi \left( {F_{s} } \right)}}{4} + \frac{1}{4}\frac{{\alpha_{0} \mu_{x} }}{{\mu_{0} }}\psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{{\frac{ - 3}{2}}} \hfill \\ \,\, + \frac{{2\left( {K + \sum\limits_{j = 1}^{i} {c_{j} } } \right)}}{{\tau^{3} }} + \frac{\lambda + \theta Z}{\theta }\left[ {\theta^{2} e^{\theta \tau } - \frac{{e^{\theta \tau } \left( {\theta \tau - 1} \right)}}{{\tau^{2} }} - \frac{2}{{\tau^{3} }}} \right] \hfill \\ \end{gathered} \right] \hfill \\ \times \frac{{2\alpha_{0} }}{{\mu_{o} }} - \left\{ \begin{gathered} - \frac{{\left\{ {\frac{{2\alpha_{0} \mu_{x} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} }}{{\mu_{0} }} - \alpha_{0} } \right\}\alpha_{0} \psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{\frac{1}{2}} }}{{\tau^{2} }} \hfill \\ + \frac{{\left\{ {\frac{{2\alpha_{0} \mu_{x} }}{{\mu_{0} }} + s_{c} + \mu_{0} - \frac{{s_{c} \alpha_{0} }}{{\mu_{0} }} - \alpha_{0} } \right\}\frac{\sigma }{2}\psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{\frac{1}{2}} }}{{\tau^{2} }} - \frac{{h_{c} \alpha_{0} \psi \left( {F_{s} } \right)\sigma \left( {\tau + \Gamma } \right)^{\frac{1}{2}} }}{{2\mu_{0} }} \hfill \\ \end{gathered} \right\}^{2} > 0 \hfill \\ \end{gathered}$$

(30)

For

$$\mu_{x} < \left( {s_{c} + \mu_{o} } \right)$$

Hence proved.