Two echelon economic lot sizing problems with geometric shipment policy backorder price discount and optimal investment to reduce ordering cost

Abstract

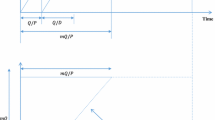
This article presents a single-vendor and a single-buyer joint economic lot size (JELS) production-distribution inventory model with the prime aim on; the effect of the investment on ordering cost reduction, back order price discount and reduction on lead time. The produced items are delivered to the buyer by adopting a geometric shipment policy. Two continuous review models are developed by assuming that the lead time demand follows a normal distribution and distribution-free. Two types of investments are incorporated to reduce the ordering cost. They are (i) logarithmic investment function and (ii) power investment function. The minimax distribution free approach is adopted in the distribution-free model to find the optimal values of the decision variables by minimizing the expected annual total cost of the system. Numerical examples are given to validate the proposed models. Sensitivity analysis is also performed to analyze the behavior of the key parameters on lot size, ordering cost, backorder price discount, the number of shipments from the vendor to the buyer in one production run and the expected annual total cost of the proposed models.

Appendices

Appendix 1

Similar to the results proved in Lin [30] the convexity of the cost function is discussed.

Observation 1

$EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is concave in $L \in [L_{i}, L_{i-1}]$ for a fixed $n, q_{1}, k, A_{b}, \pi _{x}$.

$$\begin{aligned} \frac{\partial EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial L}&= \frac{nD(\lambda -1)}{q_{1}(\lambda ^{n} -1)}\Bigl [ G(\pi _{x})\sigma \psi (k)\frac{1}{2\sqrt{L}}-c_{i} \Bigr ]\nonumber \\&\quad +\frac{h_{b}}{2\sqrt{L}}\Bigl [k\sigma +\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr )\sigma \psi (k) \Bigr ] \end{aligned}$$

(30)

$$\begin{aligned} \frac{\partial ^{2}EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial L^{2}}&=-\frac{1}{4{L^{\frac{3}{2}}}}\Bigl [\frac{nD(\lambda -1)}{q_{1}(\lambda ^{n} -1)} G(\pi _{x})\sigma \psi (k)\nonumber \\&\quad +h_{b}\Bigl [k\sigma +\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr )\sigma \psi (k) \Bigr ]\Bigr ]<0 \end{aligned}$$

(31)

Thus for a fixed $(n, q_{1}, k, A_{b}, \pi _{x})$, $EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is concave in $L \in [L_{i}, L_{i-1}]$. Hence the minimum value of $EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ exists at the end points of the interval $[L_{i}, L_{i-1}]$.

Observation 2

$EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in n for a fixed $k, q_{1}, L, A_{b}, \pi _{x}$.

$$\begin{aligned}&\text{ For, } \ \ \frac{\partial ^2}{\partial n^{2}}EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)=(h_b-h_v)\frac{\lambda ^nq_1(\ln \lambda )^2}{2(\lambda +1)}\\&\quad +h_v\frac{\lambda ^nq_1(\ln \lambda )^2(P-D)}{2P(\lambda -1)}\\&\quad +\frac{2A_vD\lambda ^n\ln \lambda (\lambda -1)}{nq_1(\lambda ^n-1)^2}+\frac{YD\lambda ^n\ln \lambda (\lambda -1)(n\ln \lambda (\lambda ^n+1)-2)}{q_1(\lambda ^n-1)^3}>0, \end{aligned}$$

where $Y = \Bigl (A_{b}+\frac{A_{v}}{n}\Bigr ) +G(\pi _x)\sigma \sqrt{L}\psi (k)+R(L)+T_{r}$

Observation 3

$EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in $q_{1}$ for a fixed $n, k, L, A_{b}, \pi _{x}$.

$$\begin{aligned}&\frac{\partial EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial q_{1}} = -\frac{1}{q_{1}^{2}}\Bigl [\Bigl (A_{b}+\frac{A_{v}}{n}\Bigr )\nonumber \\&\quad +G(\pi _{x})\sigma \sqrt{L}\psi (k)+T_{r}+R(L)\Bigr ]\frac{nD(\lambda - 1)}{\lambda ^{n}-1}\nonumber \\&\quad +h_{b}\Bigl [\frac{(\lambda ^{n}+1)}{2(\lambda +1)}\Bigr ]+h_{v}\Bigl [\frac{D}{P}+\frac{(P-D)(\lambda ^{n}-1)}{2P(\lambda -1)}-\frac{(\lambda ^{n}+1)}{2(\lambda +1)}\Bigr ] \end{aligned}$$

(32)

$$\begin{aligned}&\frac{\partial ^{2}EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial q_{1}^{2}}=\frac{2}{q_{1}^{3}}\frac{nD(\lambda - 1)}{(\lambda ^{n}-1)}\Bigl [\Bigl (A_{b}+\frac{A_{v}}{n}\Bigr )\nonumber \\&\quad +G(\pi _{x})\sigma \sqrt{L}\psi (k) +T_{r}+R(L)\Bigr ] > 0 \end{aligned}$$

(33)

Observation 4

$EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in k for a fixed $n, q_{1}, L, A_{b}, \pi _{x}$.

$$\begin{aligned}&\frac{\partial EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial k} = \sigma \sqrt{L}\Bigl [\Bigl (\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}G(\pi _{x})\nonumber \\&\quad +h_{b}\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr ) \Bigr )\Bigl (F(k)-1\Bigr )+h_{b}\Bigr ] \end{aligned}$$

(34)

$$\begin{aligned}&\frac{\partial ^{2}EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial k^{2}}=\sigma \sqrt{L}\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}G(\pi _{x})\phi (k)\nonumber \\&\quad +h_{b}\sigma \sqrt{L}\phi (k)\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr ) > 0 \end{aligned}$$

(35)

Observation 5

$EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in $\pi _{x}$ for a fixed $n, q_{1}, L, A_{b}, k$.

$$\begin{aligned}&\text{ For, }~~ \frac{\partial EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial \pi _{x}} \nonumber \\&\quad = \beta _{0}\sigma \sqrt{L}\psi (k)\Bigl [-\frac{h_{b}}{\pi _{0}} +\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}\Bigl (2\frac{\pi _{x}}{\pi _{0}}-1\Bigr )\Bigr ] \end{aligned}$$

(36)

$$\begin{aligned}&\text{ and }~~\frac{\partial ^{2}EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial \pi _{x}^{2}}=\sigma \sqrt{L}\psi (k)\frac{2nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}\frac{\beta _{0}}{\pi _{0}} > 0 \end{aligned}$$

(37)

Observation 6

$EATCI_{N}^{L}(n, q_{1}, k, \pi _{x}, \pi _{x}, L)$ is convex in $A_{b}$ for a fixed $n, q_{1}, L, \pi _{x}, k$.

$$\begin{aligned}&\text{ For, }~~ \frac{\partial EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial A_{b}}=-\frac{\tau }{\delta A_{b}}+\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)} \end{aligned}$$

(38)

$$\begin{aligned}&\text{ and }~~\frac{\partial ^{2}EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial A_{b}^{2}}=\frac{\tau }{\delta A_{b}^{2} } > 0 \end{aligned}$$

(39)

Observation 7

$EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is concave in $L \in [L_{i}, L_{i-1}]$ for a fixed $n, q_{1}, k, A_{b}, \pi _{x}$.

$$\begin{aligned}&\text{ For, }~~\frac{\partial EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial L} = \frac{nD(\lambda -1)}{q_{1}(\lambda ^{n} -1)}\Bigl [ G(\pi _{x})\sigma K\frac{1}{2\sqrt{L}}-c_{i} \Bigr ]\nonumber \\&\quad +\frac{h_{b}}{2\sqrt{L}}\Bigl [k\sigma +\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr )\sigma K \Bigr ] \end{aligned}$$

(40)

$$\begin{aligned}&\frac{\partial ^{2}EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial L^{2}} =-\frac{1}{4{L^{\frac{3}{2}}}}\Bigl [\frac{nD(\lambda -1)}{q_{1}(\lambda ^{n} -1)} G(\pi _{x})\sigma K\nonumber \\&\quad +h_{b}\Bigl [k\sigma +\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr )\sigma K \Bigr ]\Bigr ]<0 \end{aligned}$$

(41)

Thus for a fixed $(n, q_{1}, k, A_{b}, \pi _{x})$, $EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is concave in $L \in [L_{i}, L_{i-1}]$. Hence the minimum value of $EATCI_{N}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ exists at the end points of the interval $[L_{i}, L_{i-1}]$.

Observation 8

$EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in n for a fixed $k, q_{1}, L, A_{b}, \pi _{x}$,.

$$\begin{aligned}&\text{ For, } \ \ \frac{\partial ^2}{\partial n^{2}}EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)=(h_b-h_v)\frac{\lambda ^nq_1(\ln \lambda )^2}{2(\lambda +1)}\\&\quad +h_v\frac{\lambda ^nq_1(\ln \lambda )^2(P-D)}{2P(\lambda -1)}\\&\quad +\frac{2A_vD\lambda ^n\ln \lambda (\lambda -1)}{nq_1(\lambda ^n-1)^2}+\frac{YD\lambda ^n\ln \lambda (\lambda -1)(n\ln \lambda (\lambda ^n+1)-2)}{q_1(\lambda ^n-1)^3}>0, \end{aligned}$$

where $Y = \Bigl (A_{b}+\frac{A_{v}}{n}\Bigr ) +G(\pi _x)\sigma \sqrt{L}K+R(L)+T_{r}$

Observation 9

$EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in $q_{1}$ for a fixed $n, k, L, A_{b}, \pi _{x}$,.

$$\begin{aligned}&\text{ For, }~~ \frac{\partial EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial q_{1}} = -\frac{1}{q_{1}^{2}}\Bigl [\Bigl (A_{b}+\frac{A_{v}}{n}\Bigr )\nonumber \\&\quad +G(\pi _{x})\sigma \sqrt{L}K+T_{r}+R(L)\Bigr ]\frac{nD(\lambda - 1)}{(\lambda ^{n}-1)}\nonumber \\&\quad +h_{b}\Bigl [\frac{(\lambda ^{n}+1)}{2(\lambda +1)}\Bigr ]+h_{v}\Bigl [\frac{D}{P}+\frac{(P-D)(\lambda ^{n}-1)}{2P(\lambda -1)}-\frac{(\lambda ^{n}+1)}{2(\lambda +1)}\Bigr ] \end{aligned}$$

(42)

$$\begin{aligned}&\text{ and }~~\frac{\partial ^{2}EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial q_{1}^{2}}=\frac{2}{q_{1}^{3}}\frac{nD(\lambda - 1)}{(\lambda ^{n}-1)}\Bigl [\Bigl (A_{b}+\frac{A_{v}}{n}\Bigr )\nonumber \\&\quad +G(\pi _{x})\sigma \sqrt{L}K+T_{r}+R(L)\Bigr ] > 0 \end{aligned}$$

(43)

Observation 10

$EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in k for a fixed $n, q_{1}, L, A_{b}, \pi _{x}$.

$$\begin{aligned}&\frac{\partial EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial k} = \frac{\sigma \sqrt{L}}{2}\left[ \left( \frac{G(\pi _x)Dn(\lambda -1)}{q_1(\lambda ^n-1)}+h_b\left( 1-\frac{\beta _0\pi _x}{\pi _0}\right) \right) \right. \nonumber \\&\quad \left. \times \left( \frac{k}{\sqrt{1+k^2}}-1\right) +2h_b\right] \end{aligned}$$

(44)

$$\begin{aligned}&\frac{\partial ^{2}EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial k^{2}}=\frac{\sigma \sqrt{L}}{2(1+k^{2})^{\frac{3}{2}}} \Bigl [\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}G(\pi _{x})+h_{b}\Bigl (1-\frac{\beta _{0}\pi _{x}}{\pi _{0}}\Bigr )\Bigr ] > 0 \end{aligned}$$

(45)

Observation 11

$EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)$ is convex in $\pi _{x}$ for a fixed $n, q_{1}, L, A_{b}, k$.

$$\begin{aligned} \text{ For, }~~ \frac{\partial EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial \pi _{x}}&= \beta _{0}\sigma \sqrt{L}K\Bigl [-\frac{h_{b}}{\pi _{0}} +\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}\Bigl (2\frac{\pi _{x}}{\pi _{0}}-1\Bigr )\Bigr ] \end{aligned}$$

(46)

$$\begin{aligned} \text{ and }~~\frac{\partial ^{2}EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial \pi _{x}^{2}}&=\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)}\frac{2\beta _{0}}{\pi _{0}}\sigma \sqrt{L}K > 0 \end{aligned}$$

(47)

Observation 12

$EATCI_{F}^{L}(n, q_{1}, k, \pi _{x}, \pi _{x}, L)$ is convex in $A_{b}$ for a fixed $n, q_{1}, L, \pi _{x}, k$.

$$\begin{aligned} \text{ For, }~~ \frac{\partial EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial A_{b}}&=-\frac{\tau }{\delta A_{b}}+\frac{nD(\lambda - 1)}{q_{1}(\lambda ^{n}-1)} \end{aligned}$$

(48)

$$\begin{aligned} \text{ and }~~\frac{\partial ^{2}EATCI_{F}^{L}(n, q_{1}, k, A_{b}, \pi _{x}, L)}{\partial A_{b}^{2}}&=\frac{\tau }{\delta A_{b}^{2} } > 0 \end{aligned}$$

(49)

Appendix 2

As the total cost function of the integrated system is highly non-linear, the convexity of the function for a given L is checked in the distribution free case when logarithmic investment function is adopted. For this case, the Hessian matrix H is given below. The leading principal minors $H_{55}, H_{44}, H_{33}, H_{22}, H_{11}$ are positive and thus the hessian matrix is positive definite. Thus the solution obtained is globally optimum.

$$\begin{aligned} H&= \left( \begin{array}{ccccc} \frac{\partial ^2EATCI_F^L}{\partial n^2}&{} \frac{\partial ^2EATCI_F^L}{\partial n\partial q_1}&{} \frac{\partial ^2EATCI_F^L}{\partial n\partial k}&{} \frac{\partial ^2EATCI_F^L}{\partial n\partial A_b}&{} \frac{\partial ^2EATCI_F^L}{\partial n\partial \pi _x}\\ \frac{\partial ^2EATCI_F^L}{\partial q_1 \partial n}&{} \frac{\partial ^2EATCI_F^L}{\partial q_1^2}&{} \frac{\partial ^2EATCI_F^L}{\partial q_1\partial k}&{} \frac{\partial ^2EATCI_F^L}{\partial q_1\partial A_b}&{} \frac{\partial ^2EATCI_F^L}{\partial q_1\partial \pi _x}\\ \frac{\partial ^2EATCI_F^L}{\partial k \partial n}&{} \frac{\partial ^2EATCI_F^L}{\partial k\partial q_1}&{} \frac{\partial ^2EATCI_F^L}{\partial k^2}&{} \frac{\partial ^2EATCI_F^L}{\partial k\partial A_b}&{} \frac{\partial ^2EATCI_F^L}{\partial k\partial \pi _x}\\ \frac{\partial ^2EATCI_F^L}{\partial A_b \partial n}&{} \frac{\partial ^2EATCI_F^L}{\partial A_b\partial q_1}&{} \frac{\partial ^2EATCI_F^L}{\partial A_b\partial k}&{} \frac{\partial ^2EATCI_F^L}{\partial A_b^2}&{} \frac{\partial ^2EATCI_F^L}{\partial A_b \partial \pi _x}\\ \frac{\partial ^2EATCI_F^L}{\partial \pi _x \partial n}&{} \frac{\partial ^2EATCI_F^L}{\partial \pi _x\partial q_1}&{} \frac{\partial ^2EATCI_F^L}{\partial \pi _x\partial k }&{} \frac{\partial ^2EATCI_F^L}{\partial \pi _x\partial A_b}&{} \frac{\partial ^2EATCI_F^L}{\partial \pi _x^2}\\ \end{array}\right) \\ H_{55}&=\left| \begin{array}{rrrrr} 1684.7&{} 31.1922&{} 65.3789&{} -0.4597&{} -0.0139\\ 31.1922&{} 0.6862&{} 3.5557&{} -0.0250&{} -0.000758\\ 65.3789&{} 3.5557&{} 309.3898&{} 0&{} -0.000532\\ -0.4597&{} -0.0250&{} 0&{} 1.1952&{} 0\\ -0.0139&{} -0.000758&{} -0.0000532&{} 0&{} 0.0097\\ \end{array}\right| = 542.30; \\ H_{44}&=\left| \begin{array}{rrrr} 1684.7&{} 31.1922&{} 65.3789&{} -0.4597\\ 31.1922&{} 0.6862&{} 3.5557&{} -0.0250\\ 65.3789&{} 3.5557&{} 309.3898&{} 0\\ -0.4597&{}-0.0250&{} 0&{} 1.1952 \end{array}\right| =55924;\\ H_{33}&=\left| \begin{array}{rrr} 1684.7&{} 31.1922&{} 65.3789\\ 31.1922&{} 0.6862&{} 3.5557\\ 65.3789&{} 3.5557&{} 309.3898 \end{array}\right| =46915;\\ H_{22}&=\left| \begin{array}{rr} 1684.7&{} 31.1922\\ 31.1922&{} 0.6862 \end{array}\right| =183.09;\\ H_{11}&= 1684.7. \end{aligned}$$

Fig. 1

Inventory pattern of the buyer

Fig. 2

Inventory pattern of the vendor

Fig. 3

Inventory pattern of the system

Fig. 4

Expected total cost vs. number of shipments

Fig. 5

Impact of the parameters on the expected total cost

Table 1 Summary of a few related literature

Table 2 Lead time components with data

Table 3 Summarized lead time data

Table 4 Optimal solution for normal distribution case (logarithmic investment)

Table 5 Optimal solution for normal distribution case (power investment)

Table 6 Optimal solution for distribution free case (logarithmic investment)

Table 7 Optimal solution for distribution free case (power investment)

Table 8 Impact of the parameter $\lambda$ on the optimal policy

Table 9 Impact of the parameter $\beta _{0}$ on the optimal policy