Introduction

National height systems are referred to an official vertical datum and adopt different height coordinates. A complete review of all the systems in use, as well as the definition of the different height coordinates can be found, for instance, in Jekeli (2000); Meyer et al. (2006); Sansó et al. (2019).

Properly defined altimetric coordinates cannot be derived from spirit levelling observations only, but additional gravity observations at the levelling benchmarks are required. In Italy, the official height system is defined through a high-precision levelling network established and maintained by the Istituto Geografico Militare (IGM). The datum is conventionally fixed at the Genova tide gauge for the peninsula, at the Catania and Cagliari tide gauges for Sicily and Sardinia islands, respectively.

To encompass European prescriptions for the establishment of the first version of the United European Levelling Network (UELN), in the 1970 s IGM performed gravity measurements on some of the existing levelling lines and estimated the geopotential numbers of the involved levelling benchmarks. However, most of the current benchmark heights are still derived from the least squares adjustment of pure levelling measurements.

Further developments in the establishment of a unified European and international height datum, like those achieved by the European Vertical Network (EUVN) (Idhe et al. , year) and finally with the European Vertical Reference System (EVRS) project (Sacher et al. , 2006), made the computation of updated and properly defined height coordinates for the whole Italian levelling network an essential task for IGM. Based on the results of a preliminary test in the western Italian Alps (Barzaghi et al. (2014),(Betti et al. , 2015)), it was decided to derive the needed gravity values, currently observed only on a subset of the Italian levelling lines, from the Italian gravity database (IGD) used for the computation of the local Geoid ITALGEO05 (Barzaghi et al. , 2007), consisting in a 20 arcseconds spacing grid. The predicted values, in fact, were proven to be sufficiently accurate for this kind of application.

In this paper, the definition of orthometric and normal heights and their relationship with levelling increments is given in section 2; the results obtained on 38 levelling closed loops of the Italian network is presented in section 3. Beside the IGD data set, the global gravity models EGM2008 and XGM2019e were used to derive gravity values for the levelling corrections. The results of the comparison between the three sets of predicted values and the available observed values are reported in same section. The conclusions can be found in section 3.

Orthometric and normal gravity corrections to spirit leveling observations

The height of a point on the Earth surface can be expressed in different ways. In this work, we have considered orthometric and normal heights. The orthometric height is the length of the curved plumbline between the point and the equipotential surface of the gravity field chosen as reference, the Geoid. The orthometric height of two benchmarks is linked to their levelling difference through the following equation, depending on the value of the gravity along the levelling line (Heiskanen and Moritz , 1967; Sansó and Sideris , 2013):

$$\begin{aligned}{} & {} \Delta _{AB}L=H_B-H_A- \int _{ l _{AB}}\frac{g-\gamma _0}{\gamma _0}\delta L+ \nonumber \\{} & {} -\frac{\overline{g}_A-\gamma _0}{\gamma _0}H_A+ \frac{\overline{g}_B-\gamma _0}{\gamma _0}H_B= \nonumber \\{} & {} =H_B-H_A+OC_1+OC_2+OC_3 \end{aligned}$$
(1)

where \(\Delta _{AB}L\) is the observed levelling difference between the benchmarks A and B, H is the benchmark orthometric height, \(\gamma _0\) is a conventional value for the normal gravity that we have set equal to 980.6294 gal, \(\overline{g}\) is the average gravity value along the vertical through the considered benchmark from the geoid and the Earth surface. \(\overline{g}\), in gal, assuming an approximated constant Earth crust density, is given by the Prey’s reduction formula as a function of the orthometric height H (in km):

$$\begin{aligned} \overline{g}= g(H=0)+0.0424 H \end{aligned}$$
(2)

The normal height is defined with respect to the normal gravity field, i.e., the gravity field that would be generated by the actual mass of the Earth, if confined in a well-defined reference ellipsoid (Heiskanen and Moritz , 1967; Sansó and Sideris , 2013). For any point P on the Earth surface, a point Q can be found, on the normal to the reference ellipsoid through P, such that its normal gravity potential, U(Q), equals that of the actual gravity potential of P, W(P). The normal height of P is the distance of Q from the the reference ellipsoid along the normal. The normal height of two benchmarks is linked to their levelling difference through the following equation, depending on the value of the gravity along the levelling line (Betti et al. , 2015)

$$\begin{aligned}{} & {} \Delta _{AB}L=h^*_B-h^*_A+ \nonumber \\{} & {} +\int _{ l _{AB}}h^*\frac{\partial \gamma }{\partial \varphi }\frac{1}{\gamma }d\varphi -\int _{ l _{AB}}\frac{\Delta g}{\gamma }dh= \nonumber \\{} & {} = h^*_B-h^*_A+NC_1+NC_2 \end{aligned}$$
(3)

where \(\Delta _{AB}L\) is the observed levelling difference between the benchmarks A and B, \(h^*\) is the normal height, \(\gamma \) is the normal gravity, \(\Delta g\) is the gravity anomaly and \(\varphi \) is the latitude along the line. The classical formulation of Eq. 3 can be found in (Heiskanen and Moritz , 1967). Contrary to the orthometric corrections, the evaluation of normal corrections do not require any hypothesis on the Earth crust density.

In order to compute the orthometric OC and normal NC correction terms, we started from the prediction of the gravity value g on all the levelling benchmarks. This was done by applying the \(remove-compute-restore\) technique to the Italian gravity database, recently checked for outliers and reference system inconsistencies. The applied technique, detailed in (Barzaghi et al. , 2014) consists in the removal of the low-frequency gravity field component (computed from the Wenzel Gravity geopotential model, GPM98CR to d/o 720, used for the last official Italgeo05 Italian Geoid) and of the high resolution gravity field component (Residual Terrain Correction, computed with GRAVSOFT package (Tscherning et al. , 1992)) to get a residual field (remove step). The residuals are then predicted on the benchmarks by using the fast collocation approach (compute step, Bottoni and Barzaghi (1993)). The removed components are evaluated on the benchmark position and added back to the predicted residual value (restore step). On the same benchmarks, the values of the normal gravity were computed by adding to the rigorous value on the ellipsoid \(\gamma (\varphi ,h=0)\)(cf Heiskanen and Moritz (1967) eq. 2–78), due to Somigliana (1929), the two terms of the series expansion of \(\gamma \) as a function of height (cf. Heiskanen and Moritz (1967) eq. 2–123).

Fig. 1
figure 1

The 38 closed lines of the Italian levelling network considered in the present work

Full size image
Fig. 2
figure 2

Misclosure errors of the considered 38 closed loops: in red the values obtained by summing the pure levelling increments, in black the error after applying normal corrections with gravity values predicted from IGD, in blue and green the same corrections computed from EGM2008 and XGM2019e, respectively. In yellow the tolerance values are shown. For the sake or readability, the pointwise error values are connected with piecewise linear functions

Full size image
Fig. 3
figure 3

Misclosure errors of the considered 38 closed loops: in red the values obtained by summing the pure levelling increments, in black the error after applying orthometric corrections with gravity values predicted from IGD, in blue and green the same corrections computed from EGM2008 and XGM2019e, respectively. In yellow the tolerance values are shown. For the sake or readability, the pointwise error values are connected with piecewise linear functions

Full size image
Table 1 Statistics of the misclosure errors of the considered 38 closed loops
Full size table
Fig. 4
figure 4

RMS [mgal] of the differences between EGM2008 values and independent observed gravity values for every loop section

Full size image

Note that, in the evaluation of the corrections, approximated values of height, \(\tilde{H}\) (those obtained from the least squares adjustment of the levelling difference of each closed line), were used instead of the, unknown, ellipsoidal h, normal \(h^*\) and orthometric H ones. An approximate value of the height up to 10 m does not have any significant impact on the evaluation of such correcting terms as it can be verified by propagating this uncertainty to that of the gravity corrections along a line. Assuming a 10 m standard deviation on the knowledge of height (\(\sigma _H\)=10 m), a 5 mgal standard deviation on the knowledge of the predicted gravity (\(\sigma _g\)=5 mgal), a 1 mm uncertainty on the measured leveling increments at 100 m distance, along a typical line with 100 increments, and with a difference in height among the initial and final benchmarks of 350 m, one derives a standard deviation on the gravimetric correction in the order of 4 mm against an estimated correction in the order of 10 cm. In the propagation of the errors, the main contribution comes from the gravity prediction error, which, anyway, is much smaller than the computed corrections.

Fig. 5
figure 5

RMS [mgal] of the differences between the IGD independent observed gravity values for every loop section

Full size image

For any levelling difference \(\Delta _{AB}L\) between the benchmarks A and B, the following approximations to the integrals in Eq. 1 were therefore introduced to get the orthometric corrections:

$$\begin{aligned}{} & {} OC_1(AB) \approx \left[ \frac{1}{\gamma _0}\frac{g_A+g_B}{2} -1\right] \Delta _{AB}L\nonumber \\{} & {} OC_2(AB)=\frac{\overline{g}_A-\gamma _0}{\gamma _0}H_A\approx \frac{\overline{g}_A-\gamma _0}{\gamma _0}\tilde{H}_A\\{} & {} OC_3(AB)=\frac{\overline{g}_B-\gamma _0}{\gamma _0}H_B\approx \frac{\overline{g}_B-\gamma _0}{\gamma _0}\tilde{H}_B\nonumber \end{aligned}$$
(4)

Analogously, the integrals in Eq. 3 for the evaluation of the normal correction terms were approximated as follows:

$$\begin{aligned} NC_1\left( AB\right)= & {} \int _{l_{AB}}h^*\frac{\partial \gamma }{\partial \varphi }\frac{1}{\gamma }d\varphi \nonumber \\\approx & {} \frac{1}{2}\left( {\left. \frac{\tilde{H}_{A}}{\gamma _A}\frac{\partial \gamma }{\partial \varphi }\right| }_A+{\left. \frac{\tilde{H}_{B}}{\gamma _B}\frac{\partial \gamma }{\partial \varphi }\right| }_B\right) \left( \varphi _B-\varphi _A\right) \nonumber \\ NC_2\left( AB\right)= & {} \int _{l_{AB}}\frac{\triangle _g}{\gamma }dh\approx \frac{1}{2}\left( \frac{\triangle _{g_A}}{\gamma _A}+\frac{\triangle _{g_B}}{\gamma _B}\right) \;\triangle _{AB}L \end{aligned}$$
(5)

where \(\displaystyle {\frac{\partial \gamma }{\partial \varphi }}\) is obtained by deriving eq. 2–116 (cf. Heiskanen and Moritz (1967))

$$\begin{aligned} \frac{\partial \gamma }{\partial \varphi } \!=\! \gamma _a (-5,\!302457\cdot 10^{-3}\sin (2\varphi )-1,16478\cdot 10^{-5}\! \sin (4 \varphi )) \end{aligned}$$
(6)

\(\gamma _a\) being the normal gravity modulus at the equator. All the numerical coefficients are computed accounting for the GRS80 reference ellipsoid.

Results and discussion

Thirty-eight closed loops of the Italian levelling network, shown in Fig. 1, were considered.

Gravity values were predicted at each benchmark starting from three different gravity data sets: the Italian gravity database and the global gravity models EGM2008 and XGM2019e. From the three predicted gravity values, three different sets of both normal and orthometric corrections were computed and applied to each leveling increment. The sum of the leveling increments of each loop, i.e., the loop misclosure error, before and after applying the gravimetric corrections, was eventually evaluated. We expect that, by accounting for the gravity effects, this error, due to observation white noise only, would be smaller than a tolerance level equal to:

$$\begin{aligned} t [m]=2.5\cdot 10^{-3}\sqrt{L[km]}. \end{aligned}$$
(7)

The misclosure errors are reported in Figs. 2 and 3 for the normal case and for the orthometric case, respectively. For the sake of readability, the pointwise error values are connected with piecewise linear functions. In both figures, the red lines connect the misclosure errors before applying any gravimetric correction; the black, blue and green lines connect the errors after correcting the leveling increments for the gravity effects, by exploiting IGD, EGM2008 and XGM2019e gravity values, respectively.

Fig. 6
figure 6

Free-air anomaly comparison along one section of the loop 1

Full size image

As it can be seen in Figs. 2 and  3, all the misclosure errors obtained from the pure leveling increments are within the tolerance level, except the loop \(8\_1\). After applying the gravimetric corrections from IGD, all loops are within the prescribed tolerance level. This does not apply to all loops corrected with either the EGM2008 or the XGM2019e values. In Table 1 the statistics of the misclosure errors represented in 2 and in 3 are reported. The average of the misclosure errors of all the considered lines is significantly equal to 0 with standard deviations all in the order of 2 cm.

A comparison between EGM2008, XGM2019e and IGD predictions against available independent observed gravity data along the leveling lines showed a much better agreement between the IGD predictions and the observed values. The RMS values of the differences between observed and EGM2008 gravity values, computed on every loop section where observed values were available, \(RMS_{EGM2008}\), are shown in Fig. 4. Equivalent results, not shown here, were obtained with XGM2019e. The RMS values of the differences between the observed and the IGD predictions, \(RMS_{IGD}\), are shown in Fig. 5.

The range of variability of \(RMS_{EGM2008}\) and of \(RMS_{XGM2019e}\) is from 10 to 100 mgal, much larger than that of \(RMS_{IGD}\), which is from 5 to 30 mgal. The largest differences are localised in the alpine regions (cfr. loops from 1 to 7 and loop \(8\_1\) in Fig. 1).

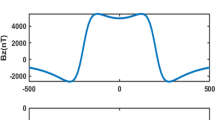
An example of the free-air gravity anomalies obtained from IGD, EGM2008 and XGM2019e along one loop sector, compared with the corresponding observed values is reported in Fig. 6.

While the IGD predicted gravity values are in good agreement with the observed values, the EGM2008 and XGM2019e values are similar to one-another but far from the observed values. The RMS of the differences for this specific sector are reported in Table 2.

Table 2 RMS of the differences of IGD, EGM2008 and XGM2019e with respect to the available independent observed gravity values of one section of loop 1
Full size table

Conclusions

This paper reports the procedure applied to correct the observations of the Italian reference leveling network for the gravity effects. The assessment performed on the misclosure errors of the 38 loops after applying the gravity corrections confirms that the IGD predicted gravity can be effectively used for this task. As expected, gravity corrections reduce misclosure errors larger than the tolerance level due to strong height/gravity variations, as for the loop \(8\_1\), located around the Ivrea body (Barzaghi et al. , 2014). EGM2008 and XGM2019e gravity values proved to be less efficient in this application: the misclosure error of the gravity corrected leveling increments becomes larger than the tolerance level in three cases. A comparison between predicted and available observed gravity values was performed to further investigate the reasons of such result. A good agreement was found with the IGD predicted gravity values, while the differences of both EGM2008 and XGM2019e have large RMS values in the North-eastern alpine region, where the gravity corrections become essential because of the large height variations, and close to the Ivrea body where the corrections are not negligible due to the high values of the gravity field.

The procedure presented in the paper will be applied to the new lines in southern Italy and Sardinia as soon as they are available. This will allow for a global least squares adjustment to eventually obtain the Italian reference normal and orthometric height systems.