Group velocity dispersion analysis in northern Peninsular Malaysia

Abstract

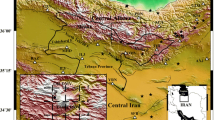
The crustal structure beneath three seismic stations over Malaysia has been investigated with the application of the group velocity dispersion analysis of the northern Sumatra earthquake data which occurred on 06 April 2010. Eighteen crustal layer models are constructed to assess the structure. Group velocity dispersions have been computed for the recorded earthquake data using a graphical method and modified Haskell matrix method for the models. Both dispersions have been presented for the interpretation of crustal layers. Findings have shown four major crustal layers having thicknesses of 2.5–4.0, 2.0–5.5, 5.0–8.0, and 8.5–9.0 km, while in Terengganu, it has shown three layers. Density, shear, and compressional wave velocities used in models have suggested that the crustal structure of the northern part of Peninsular Malaysia is crystalline. Major crustal minerals are of quartz, plagioclase, and mica. Most layers seem to have upward directions toward Perak from Kedah and Terengganu.

Appendices

Appendix 1

Haskell and half-space matrices

Notation employed: ω= angular frequency; c= phase velocity; k = ω/c= angular wave number.

For layer m: ρ _m = density; d _m = thickness; α _m = compressional wave velocity; β _m = shear wave velocity.

$$ {\gamma}_m=2{\left({\beta}_m/c\right)}^2 $$

(8)

$$ {r}_{\alpha m}=+{\left[{c}^2/{\alpha}_m^2-1\right]}^{1/2}\to c>{\alpha}_m $$

(9)

$$ {r}_{\beta m}=+{\left[{c}^2/{\beta}_m^2-1\right]}^{1/2}\to c>{\beta}_m $$

(10)

$$ {r}_{\alpha m}=-i{\left[{c}^2/{\alpha}_m^2\right]}^{1/2}\to c<{\alpha}_m $$

(11)

$$ {r}_{\beta m}=-i{\left[1-{c}^2/{\beta}_m^2\;\right]}^{1/2}\to c<{\beta}_m $$

(12)

$$ {P}_m=k{r}_{\alpha m}\;{d}_m $$

(13)

$$ {Q}_m=kr{\beta}_m\;{d}_m $$

(14)

The Haskell matrix components for layer m are:

$$ {A}_{11}^m={A}_{44}^m = {\gamma}_m\;CP-\left({\gamma}_m-1\;\right)CQ $$

(15)

$$ {A}_{12}^m={A}_{34}^m=i\left[\left({\gamma}_m-1\right)\;{r}_{\alpha m}^{-1}\;SP+{\gamma}_m\;{r}_{\beta m}\;SQ\right] $$

(16)

$$ {A}_{13}^m={A}_{24}^m = -{\left({\rho}_m{c}^2\right)}^{-1}\left(CP-CQ\right) $$

(17)

$$ {A}_{14}^m=i{\left({\rho}_m{c}^2\right)}^{-1}\left({r}_{\alpha m}^{-1}SP+{r}_{\beta m}SQ\right) $$

(18)

$$ {A}_{21}^m={A}_{43}^m=-i\left[{\gamma}_m\;{r}_{\alpha m}SP+\left({\gamma}_m-1\right)\;{r}_{\beta m}^{-1}SQ\right] $$

(19)

$$ {A}_{22}^m={A}_{33}^m=-\left({\lambda}_m-1\right)CP+{\lambda}_mCQ $$

(20)

$$ {A}_{23}^m=i{\left({\rho}_m{c}^2\right)}^{-1}\left({r}_{\alpha m}SP+{r}_{\beta m}^{-1}SQ\right) $$

(21)

$$ {A}_{31}^m={A}_{42}^m={\rho}_m{c}^2{\gamma}_m\;\left({\gamma}_m-1\right)\;\left(CP-CQ\right) $$

(22)

$$ {A}_{32}^m=i{\rho}_m{c}^2\left[{\left({\gamma}_m-1\right)}^2{r}_{\alpha m}^{-1}SP+{\gamma}_m^2{r}_{\beta m}SQ\right] $$

(23)

$$ {A}_{41}^m=i{\rho}_m{c}^2\left[{\gamma}_m^2\;{r}_{\alpha m}SP+{\left({\gamma}_m-1\right)}^2{r}_{\beta m}^{-1}SQ\right] $$

(24)

where

$$ \begin{array}{l}SP= \sin \left({P}_m\right)\kern0.5em SQ= \sin \left({Q}_m\right)\\ {}CP= \cos \left({P}_m\right)\kern0.5em \mathrm{and}\kern0.5em CQ= \cos \left({Q}_m\right)\end{array} $$

The half-space matrix is

$$ {\widehat{E}}^n=\left[\begin{array}{cccc}\hfill -2{\left({\beta}_n/{\alpha}_n\right)}^2\hfill & \hfill -{c}^2\left({\gamma}_n-1\right)\hfill & \hfill {\alpha}_n^2{r}_{\alpha n}/{\left({\rho}_n\;{\alpha}_n^2\right)}^{-1}\hfill & \hfill -{\left({\rho}_n{\alpha}_n^2{r}_{\alpha n}\right)}^{-1}\hfill \\ {}\hfill -\left({\gamma}_n-1/{\gamma}_n{r}_{\beta n}\right)\hfill & \hfill 1\hfill & \hfill {\left({\rho}_n{c}^2{\gamma}_n{r}_{\beta n}\right)}^{-1}\kern0.5em \hfill & \hfill {\left({\rho}_n{c}^2{\gamma}_n\right)}^{-1}\hfill \end{array}\right] $$

(25)

Appendix 2

Example matrix operation

The 6 × 6 ${B}_{pq}^m$ for the mth layer is derived from the second-order sub-determinants ${A}^m\;\Big|{\;}_{pq}^{ij}$ of the Haskell matrix by the following convention:

$$ \begin{array}{cc}\hfill ij(kl)\hfill & \hfill p(q)\hfill \\ {}\hfill 12\hfill & \hfill 1\hfill \\ {}\hfill 13\hfill & \hfill 2\hfill \\ {}\hfill 14\hfill & \hfill 3\hfill \\ {}\hfill 23\hfill & \hfill 4\hfill \\ {}\hfill 24\hfill & \hfill 5\hfill \\ {}\hfill 34\hfill & \hfill 6\hfill \end{array} $$

Example: Let us consider a 4 × 4 matrix,

$$ {A}^m=\left[\begin{array}{cccc}\hfill {a}_{11}\hfill & \hfill {a}_{12}\hfill & \hfill {a}_{13}\hfill & \hfill {a}_{14}\hfill \\ {}\hfill {a}_{21}\hfill & \hfill {a}_{22}\hfill & \hfill {a}_{23}\hfill & \hfill {a}_{24}\hfill \\ {}\hfill {a}_{31}\hfill & \hfill {a}_{32}\hfill & \hfill {a}_{33}\hfill & \hfill {a}_{34}\hfill \\ {}\hfill {a}_{41}\hfill & \hfill {a}_{42}\hfill & \hfill {a}_{43}\hfill & \hfill {a}_{44}\hfill \end{array}\right] $$

According to the convention, second-order sub-determinant matrix elements are:

$$ \begin{array}{l}{A}^m\;\Big|{}_{12}^{12}={A}_{11}^m{A}_{22}^m-{A}_{12}^m{A}_{21}^m={a}_{11}{a}_{22}-{a}_{12}{a}_{21}={B}_{11}^m\\ {}{A}^m\;\Big|{}_{13}^{12}={A}_{11}^m{A}_{23}^m-{A}_{13}^m{A}_{21}^m={a}_{11}{a}_{23}-{a}_{13}{a}_{21}={B}_{12}^m\\ {}\cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \cdot \\ {}{A}^m\;\Big|{}_{34}^{34}={A}_{33}^m{A}_{44}^m-{A}_{34}^m{A}_{43}^m={a}_{33}{a}_{44}-{a}_{34}{a}_{43}={B}_{66}^m\end{array} $$

Therefore, the 6 × 6 matrix,

$$ {B}^m=\left[\begin{array}{cccccc}\hfill {B}_{11}\hfill & \hfill {B}_{12}\hfill & \hfill {B}_{13}\hfill & \hfill {B}_{14}\hfill & \hfill {B}_{15}\hfill & \hfill {B}_{16}\hfill \\ {}\hfill {B}_{21}\hfill & \hfill {B}_{22}\hfill & \hfill {B}_{23}\hfill & \hfill {B}_{24}\hfill & \hfill {B}_{25}\hfill & \hfill {B}_{26}\hfill \\ {}\hfill {B}_{31}\hfill & \hfill {B}_{32}\hfill & \hfill {B}_{33}\hfill & \hfill {B}_{34}\hfill & \hfill {B}_{35}\hfill & \hfill {B}_{36}\hfill \\ {}\hfill {B}_{41}\hfill & \hfill {B}_{42}\hfill & \hfill {B}_{43}\hfill & \hfill {B}_{44}\hfill & \hfill {B}_{45}\hfill & \hfill {B}_{46}\hfill \\ {}\hfill {B}_{51}\hfill & \hfill {B}_{52}\hfill & \hfill {B}_{53}\hfill & \hfill {B}_{54}\hfill & \hfill {B}_{55}\hfill & \hfill {B}_{56}\hfill \\ {}\hfill {B}_{61}\hfill & \hfill {B}_{62}\hfill & \hfill {B}_{63}\hfill & \hfill {B}_{64}\hfill & \hfill {B}_{65}\hfill & \hfill {B}_{66}\hfill \end{array}\right] $$

Appendix 3

Model error analysis

The standard error of estimate (SE), mean residual (MR), average absolute residual (AR), weighted root mean square error (RMS), and the percent of signal power fit (SPF) are (Elenean et al. 2009):

$$ SE=\sqrt{\frac{{\displaystyle \sum_{i=1}^N{\left(ob{s}_i-\mathrm{mean}\right)}^2}}{N-1}} $$

(26)

$$ MR=\frac{{\displaystyle \sum_{i=1}^N\left(ob{s}_i-pre{d}_i\right)}}{N} $$

(27)

$$ AR=\frac{1}{N}\;\left|{\displaystyle \sum_{i=1}^N\frac{\left(ob{s}_i-pre{d}_i\right)}{SE}}\right| $$

(28)

$$ RMS=\sqrt{\frac{{\displaystyle \sum_{i=1}^N\frac{{\left(ob{s}_i-pre{d}_i\right)}^2}{\mathrm{mean}}}}{N\left(N-1\right)}} $$

(29)

$$ SPF=\left(1-\frac{{{\displaystyle \sum_{i=1}^n\left(ob{s}_i-pre{d}_i\right)}}^2}{{{\displaystyle \sum_{i=1}^N\left(ob{s}_i\right)}}^2}\right)\times 100 $$

(30)

where obs is the observed group velocity at each period, mean is the mean of the observed group velocities, N is the number of observations at each period, and pred is the predicted group velocity of the current model. Estimated errors of the models are shown in the following tables.

Table 5 Data fit criteria for KUM station

Table 6 Data fit criteria for IPM station

Table 7 Data fit criteria for KTM station

Appendix 4

Crustal abundance properties

Table 8 Average crustal abundance, density, and seismic velocities of major crustal minerals (after Anderson 2007)