Skip to main content
SpringerLink
Log in

A Topological Classification of Interval Mappings with Finitely Many Discontinuous Points

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

Abstract

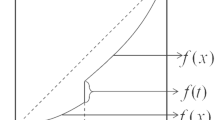
Topological conjugacy plays an important role in the study of dynamical systems and functional equations. In this paper, a topological classification for monotone functions with finitely many discontinuous points is considered. By introducing the definition of symbolic vector for discontinuous functions, we present necessary and sufficient conditions to determine the conjugate relations between these functions. Moreover, the explicit expressions for those conjugacies are also given. Finally, as an application, our results are applied to the study of classification of generalized Lorenz maps.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  1. Baron, K., Jarczyk, W.: Recent results on functional equations in a single variable, perspectives and open problems. Aequ. Math. 60, 1–48 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  2. Belitskii, G., Tkachenko, V.: One-Dimensional Functional Equations, Operator Theory: Advances and Applications, vol. 144. Birkhäuser Verlag, Basel (2003)

    Book  MATH  Google Scholar 

  3. Block, L., Hart, D.: The bifurcation of periodic orbits of one-dimensional maps. Ergod. Theory Dynam. Syst. 2, 125–129 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  4. Block, L., Coven, E.M.: Topological conjugacy and transitivity for a class of piecewise monotone maps of the interval. Trans. Am. Math. Soc. 300, 297–306 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  5. Chow, S.N., Li, C., Wang, D.: Normal Forms and Bifurcation of Planar Vector Fields. Cambridge University Press, Cambridge (1994)

    Book  MATH  Google Scholar 

  6. Collet, P., Eckmann, J.P., Lanford, O.E.: Universal properties of maps on a interval. Commun. Math. Phys. 76, 211–254 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  7. Cui, H., Ding, Y.: Renormalization and conjugacy of piecewise linear Lorenz maps. Adv. Math. 271, 235–272 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  8. Damanik, D., Killip, R.: Almost everywhere positivity of the Lyapunov exponent for the doubling map. Commun. Math. Phys. 257, 287–290 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  9. Ding, Y.: Renormalization and \(\alpha \)-limit set for expanding Lorenz map. Discrete Dyn. Syst. 29, 979–999 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  10. Fort, M.K., Jr.: The embedding of homeomorphisms in flows. Proc. Am. Math. Soc. 6, 960–967 (1955)

    Article  MathSciNet  MATH  Google Scholar 

  11. Gerard, O., Enric, F., Carles, B.: Bifurcations and chaos in converters. Discontinuous vector fields and singular Poincaré maps. Nonlinearity 13, 1095–1121 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  12. Glandinning, P.: Topological conjugation of Lorenz maps by \(\beta \)-transformation. Math. Proc. Camb. Philos. Soc. 107, 401–413 (1990)

    Article  MathSciNet  Google Scholar 

  13. Glandinning, P., Sparrow, C.: Prime and renormalizable kneading invariants and the dynamics of expanding Lorenz maps. Phys. D 62, 22–50 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  14. Hartman, P.: Ordinary Differential Equations. Wiley, New York (1964)

    MATH  Google Scholar 

  15. Hubbard, J.H., Sparrow, C.T.: The classification of topologically expansive Lorenz maps. Commun. Pure Appl. Math. XLII I, 431–443 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  16. Jiang, Y.: On Ulam–von Neumann transformations. Commun. Math. Phys. 172, 449–459 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  17. Karlin, S., McGregor, J.: Embedding iterates of analytic functions with two fixed points into continuous groups. Trans. Am. Math. Soc. 132, 137–145 (1968)

    Article  MathSciNet  MATH  Google Scholar 

  18. Katok, A., Hasselblatt, B.: Introduction to the Modern Theory of Dynamical Systems. Cambridge University Press, Cambridge (1995)

    Book  MATH  Google Scholar 

  19. Kuczma, M.: Functional Equations in a Single Variable. Polish Scientific Publishers, Warszawa (1968)

    MATH  Google Scholar 

  20. Kuczma, M., Choczewski, B., Ger, R.: Iterative Functional Equations. Encyclopedia of Mathematics and its Applications, Cambridge University Press, Cambridge (1990)

    Book  MATH  Google Scholar 

  21. Labarca, R., Moreira, C.: Essential dynamics for Lorenz maps on the real line and the Lexicographical World. Ann. Inst. Henri Poincaré 23, 683–694 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  22. Leśniak, Z., Shi, Y.: Topological conjugacy of piecewise monotonic functions of nonmonotonicity height \(\ge 1\). J. Math. Anal. Appl. 423, 1792–1803 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  23. Li, L., Zhang, W.: Conjugacy between piecewise monotonic functions and their iterative roots. Sci. China Ser. A 59, 367–378 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  24. Li, S., Shen, W.: Smooth conjugacy between \(S\)-unimodal maps. Nonlinearity 19, 1629–1634 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  25. Li, T., Yorke, J.A.: Period three implies chaos. Am. Math. Month. 82, 985–992 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  26. Li, W.: Normal Form Theory and Its Application. Science Press, Beijing (2000). (in Chinese)

  27. Liu, J., Shi, Y.: Conjugacy problem of strictly monotone maps with only one jump discontinuity. Results Math. 19, 75–90 (2020)

    MathSciNet  MATH  Google Scholar 

  28. Llibre, J.: Structure of the Set of Periods for the Lorenz Maps. In: Dynamical System and Bifurcation Theory. Wiley, New York (1987)

  29. Palmore, J.: Chaos, cycles, and Schwarzian derivatives for families of asymmetric maps. Appl. Anal. 57, 235–242 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  30. Parry, W.: Symbolic dynamics and transformations of the unit interval. Trans. Am. Math. Soc. 122, 368–378 (1966)

    Article  MathSciNet  MATH  Google Scholar 

  31. Puph, C.: On a theorem of P. Hartman. Am. J. Math. 91, 363–367 (1962)

    MathSciNet  Google Scholar 

  32. Sarkovskii, A.N.: Coexistence of cycles of a continuous map of a line into itself. Ukr. Math. J. 16, 61–71 (1964)

    Google Scholar 

  33. Shi, Y., Tang, Y.: On conjugacies between asymmetric Bernoulli shifts. J. Math. Anal. Appl. 434, 209–221 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  34. Walters, P.: An Introduction to Ergodic Theory. Graduate Texts in Mathematics, vol. 79. Springer, New York (1982)

    Book  MATH  Google Scholar 

  35. Wiggins, S.: Global Bifurcations and Chaos: Analytical Methods. Applied Mathematical Sciences, Springer, New York (1988)

    Book  MATH  Google Scholar 

Download references

Acknowledgements

The authors are very grateful to the reviewers for their carefully checking and helpful suggestions.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Hanshan Normal University, Chaozhou, 521041, Guangdong, People’s Republic of China

    Jinghua Liu

  2. College of Data Science, Jiaxing University, Jiaxing, 314001, Zhejiang, People’s Republic of China

    Lin Li

Authors
  1. Jinghua Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lin Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors reviewed the manuscript.

Corresponding author

Correspondence to Lin Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This work is supported by the National Science Foundation of China (#12026207, #11701476) and Natural Science Foundation of Guangdong Province (#2022A1515010964).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, J., Li, L. A Topological Classification of Interval Mappings with Finitely Many Discontinuous Points. Qual. Theory Dyn. Syst. 21, 157 (2022). https://doi.org/10.1007/s12346-022-00686-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12346-022-00686-8

Keywords

Mathematics Subject Classification

Search

Navigation