Skip to main content
SpringerLink
Log in

The unit-Gompertz distribution revisited: properties and characterizations

  • Published:
Rendiconti del Circolo Matematico di Palermo Series 2 Aims and scope Submit manuscript

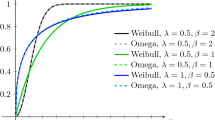
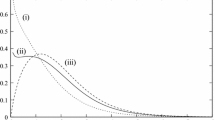
Abstract

In a recent paper, the unit-Gompertz (UG) distribution has been introduced and some of its properties have been studied. In a follow up paper, some of the subtle errors in the original paper have been corrected and some other interesting properties of this new distribution have been studied. In the present work, some more important properties are investigated. Moreover, to the best of our knowledge, no characterization results on this distribution have appeared in the literature. These are addressed in the present paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Ahsanullah, M.: Characterizations of Univariate Distributions. Atlantis Press, Paris (2017)

    Book  Google Scholar 

  2. Akbari, M.: Characterization and goodness-of-fit test of Pareto and some related distributions based on near-order statistics. J. Probab. Stat. 2020, 4262574 (2020). https://doi.org/10.1155/2020/4262574

    Article  MathSciNet  Google Scholar 

  3. Anis, M.Z., De, D.: An expository note on the unit-Gompertz distribution with applications. Statistica (Bologna) 80(4), 469–490 (2020)

    Google Scholar 

  4. Arshad, M., Azhad, Q.J., Gupta, N., Pathak, A.K.: Bayesian inference of unit-Gompertz distribution based on dual generalized order statistics. Commun. Stat.—Simul. Comput. 52(8), 3657–3675 (2023). https://doi.org/10.1080/03610918.2021.1943441

    Article  MathSciNet  Google Scholar 

  5. Baringhaus, L., Henze, N.: Tests of fit for exponentiality based on a characterization via the mean residual life function. Stat. Pap. 41, 225–236 (2000)

    Article  MathSciNet  Google Scholar 

  6. Buono, F., Longobardi, M., Szymkowiak, M.: On generalized reversed aging intensity functions. Ricer. Math. 71, 85–108 (2021). https://doi.org/10.1007/s11587-021-00560-w

    Article  MathSciNet  Google Scholar 

  7. Cheng, D.W., Zhu, Y.: Optimal order of servers in a tandem queue with general blocking. Queue. Syst. 14, 427–437 (1993)

    Article  MathSciNet  Google Scholar 

  8. Cover, T., Thomas, J.A.: Elements of Information Theory, 2nd edn. Wiley, Hoboken (2006)

    Google Scholar 

  9. Dumonceaux, R., Antle, C.E.: Discrimination between the log-normal and the Weibull distributions. Technometrics 15(4), 923–926 (1973)

    Article  Google Scholar 

  10. Glänzel, W.: A characterization theorem based on truncated moments and its application to some distribution families. In: Bauer, P., Konecny, F., Wertz, W. (eds.) Mathematical Statistics and Probability Theory. Springer, Dordrecht (1987). https://doi.org/10.1007/978-94-009-3965-3_8

    Chapter  Google Scholar 

  11. Gupta, R.D., Gupta, R.C., Sankaran, P.G.: Some characterization results based on factorization of the (reversed) hazard rate function. Commun. Stat.—Theory Methods 33, 3009–3031 (2004)

    Article  MathSciNet  Google Scholar 

  12. Hamity, V.H., Barraco, D.E.: Generalized nonextensive thermodynamics applied to the cosmical background radiation in Robertson–Walker universe. Phys. Rev. Lett. 76, 4664–4666 (1996)

    Article  Google Scholar 

  13. Hosking, J.R.: L-Moments: analysis and estimation of distributions using linear combinations of order statistics. J. R. Stat. Soc. Ser. B (Methodol.) 52, 105–124 (1990). https://doi.org/10.1111/rssb.1990.52.issue-1

    Article  MathSciNet  Google Scholar 

  14. Jha, M.K., Dey, S., Tripathi, Y.M.: Reliability estimation in a multicomponent stress-strength based on unit-Gompertz distribution. Int. J. Qual. Reliab. Manag. 37, 428–450 (2019)

    Article  Google Scholar 

  15. Jha, M.K., Dey, S., Alotaibi, R.M., Tripathi, Y.M.: Reliability estimation of a multicomponent stress-strength model for unit Gompertz distribution under progressive Type II censoring. Qual. Reliab. Eng. Int. 36, 965–987 (2020)

    Article  Google Scholar 

  16. Jiang, R., Ji, P., Xiao, X.: Aging properties of unimodal failure rate models. Reliab. Eng. Syst. Saf. 79, 113–116 (2003)

    Article  Google Scholar 

  17. Jiang, R., Cao, Y., Faqun, Q.: An aging-intensity-function-based parameter estimation method on heavily censored data. Qual. Reliab. Eng. Int. 39(8), 3484–3501 (2022)

    Article  Google Scholar 

  18. Kapur, J.N.: Generalized entropy of order \( \alpha \) and type \( \beta \). Math. Semin. 4, 79–84 (1967)

    Google Scholar 

  19. Kijima, M.: Hazard rate and reversed hazard rate monotonicities in continuous-time Markov chains. J. Appl. Probab. 35, 545–556 (1998)

    Article  MathSciNet  Google Scholar 

  20. Kumar, D., Dey, S., Ormoz, E., MirMostafaee, S.M.T.K.: Inference for the unit-Gompertz model based on record values and inter-record times with an application. Rendicont. Circolo Mat. Palermo. 69, 1295–1319 (2020). https://doi.org/10.1007/s12215-019-00471-8

    Article  MathSciNet  Google Scholar 

  21. Lagakos, W., Barraj, L.M., Gruttola, V.: Nonparametric analysis of truncated survival data, with application to AIDS. Biometrika 75(3), 515–523 (1988)

    Article  MathSciNet  Google Scholar 

  22. Lariviere, M.A., Porteus, E.L.: Selling to the newsvendor: an analysis of price-only contracts. Manuf. Serv. Oper. Manag. 3(4), 293–305 (2001)

    Article  Google Scholar 

  23. Marchetti, C.E., Mudholkar, G.S.: Characterization theorems and goodness-of-fit tests. In: Huber-Carol, C., Balakrishnan, N., Nikulin, M.S., Mesbah, M. (eds.) Goodness-of-Fit Tests and Model Validity. Statistics for Industry and Technology. Birkhäuser, Boston (2002). https://doi.org/10.1007/978-1-4612-0103-8_10

    Chapter  Google Scholar 

  24. Mathai, A.M., Haubold, H.J.: Pathway model, superstatistics, Tsallis statistics and a generalized measure of entropy. Phys. A 375, 119–122 (2007)

    Article  MathSciNet  Google Scholar 

  25. Mazucheli, J., Menezes, A.F., Dey, S.: Unit-Gompertz distribution with applications. Statistica (Bologna) 79(1), 25–43 (2019)

    Google Scholar 

  26. Mills, J.P.: Table of the ratio: area to bounding ordinate, for any portion of normal curve. Biometrika 18(3), 395–400 (1926)

    Article  Google Scholar 

  27. Nikitin, Y.: Test based on characterizations, and their efficiencies: a survey. Acta Comment. Univer. Tartu. Math. 21, 3–24 (2017)

    MathSciNet  Google Scholar 

  28. Pavía, J.M., Veres-Ferrer, E.J., Foix-Escura, G.: Credit card incidents and control systems. Int. J. Inf. Manage. 32(6), 501–503 (2012)

    Article  Google Scholar 

  29. Quesenberry, C., Hales, C.: Concentration bands for uniformity plots. J. Stat. Comput. Simul. 11(1), 41–53 (1980)

    Article  Google Scholar 

  30. Razmkhah, M., Morabbi, H., Ahmadi, J.: Comparing two sampling schemes based on entropy of record statistics. Stat. Pap. 53, 95–106 (2012)

    Article  MathSciNet  Google Scholar 

  31. Shannon, C.E.: A mathematical theory of communication. Bell Syst. Tech. J. 27, 379–423 (1948)

    Article  MathSciNet  Google Scholar 

  32. Szymkowiak, M.: Generalized aging intensity functions. Reliab. Eng. Syst. Saf. 178, 198–208 (2018)

    Article  Google Scholar 

  33. Szymkowiak, M.: Measures of ageing tendency. J. Appl. Probab. 56, 358–83 (2019)

    Article  MathSciNet  Google Scholar 

  34. Tong, S., Bezerianos, A., Paul, J., Zhu, Y., Thakor, N.: Nonextensive entropy measure of EEG following brain injury from cardiac arrest. Physica A 305, 619–628 (2002)

    Article  Google Scholar 

  35. Townsend, J.T., Wenger, M.J.: A theory of interactive parallel processing: new capacity measures and predictions for a response time inequality series. Psychol. Rev. 111, 1003–1035 (2004)

    Article  Google Scholar 

  36. Tsallis, C.: Possible generalization of Boltzmann–Gibbs statistics. J. Stat. Phys. 52(1), 479–487 (1988)

    Article  MathSciNet  Google Scholar 

  37. Upadhyay, P., Chhabra, J.K.: Kapur’s entropy based optimal multilevel image segmentation using Crow Search Algorithm. Appl. Soft Comput. 97, 105522 (2020). https://doi.org/10.1016/j.asoc.2019.105522

    Article  Google Scholar 

  38. Varma, R.S.: Generalization of Rényi’s entropy of order \( \alpha \). J. Math. Sci. 1, 34–48 (1966)

    MathSciNet  Google Scholar 

  39. Veres-Ferrer, E.J., Pavía, J.M.: Properties of the elasticity of a continuous random variable A special look at its behavior and speed of change. Commun. Stat.—Theory Methods 46(6), 3054–3069 (2017). https://doi.org/10.1080/03610926.2015.1053943

    Article  MathSciNet  Google Scholar 

  40. Veres-Ferrer, E.J., Pavía, J.M.: On the relationship between the reversed hazard rate and elasticity. Stat. Pap. 55, 275–284 (2014)

    Article  MathSciNet  Google Scholar 

  41. Veres-Ferrer, E.J., Pavía, J.M.: Elasticity function of a discrete random variable and its properties. Commun. Stat.—Theory Methods 46(17), 8631–8646 (2017). https://doi.org/10.1080/03610926.2016.1186190

    Article  MathSciNet  Google Scholar 

  42. Xu, X., Hopp, W.J.: Price trends in a dynamic pricing model with heterogeneous customers: a martingale perspective. Oper. Res. 57(5), 1298–1302 (2009)

    Article  MathSciNet  Google Scholar 

  43. Yu, M., Zhanfang, C., Hongbiao, Z.: Research of automatic medical image segmentation algorithm based on Tsallis entropy and improved PCNN. In: IEEE Proceedings on ICMA, pp. 1004–1008 (2009)

  44. Zhang, Y., Wu, L.: Optimal multi-level thresholding based on maximum Tsallis entropy via an artificial bee colony approach. Entropy 13, 841–859 (2011)

    Article  Google Scholar 

  45. Zhang, D., Jia, X., Ding, H., Ye, D., Thakor, N.V.: Application of Tsallis entropy to EEG: quantifying the presence of burst suppression after asphyxial cardiac arrest in rats. IEEE Trans. Biomed. Eng. 57(4), 867–874 (2010)

    Article  Google Scholar 

Download references

Acknowledgements

Thanks are due to the learned reviewer whose suggestions lead to an improvement in the presentation.

Author information

Authors and Affiliations

  1. SQC & OR Unit, Indian Statistical Institute, 203, B. T. Road, Calcutta, 700108, India

    M. Z. Anis & Kuntal Bera

Authors
  1. M. Z. Anis
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kuntal Bera
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Z. Anis.

Ethics declarations

Conflict of interest

There is no Conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Anis, M.Z., Bera, K. The unit-Gompertz distribution revisited: properties and characterizations. Rend. Circ. Mat. Palermo, II. Ser (2024). https://doi.org/10.1007/s12215-024-01021-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12215-024-01021-7

Keywords

Mathematics Subject Classification

Search

Navigation