Stochastic SIRS epidemic model with perturbation on immunity decay rate

Abstract

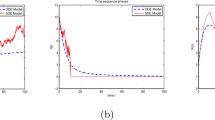
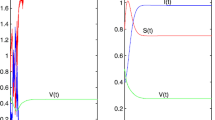
This study introduces a novel stochastic variant for the Susceptible-Infected-Recovered-Susceptible (SIRS) system, focusing on perturbations involving the immunity decay rate. We determine a critical threshold value of the reproduction number, denoted as ${\mathcal {R}}_0$, which plays a pivotal role in understanding the system dynamics. Through rigorous mathematical derivations, we have shown that a unique solution exists for the system under consideration. Additionally, we leverage the powerful analytical tool of a stochastic Lyapunov function to evaluate the extinction and persistence of the infection, providing valuable insights into the system behavior under different conditions. Our analysis reveals that if ${\mathcal {R}}_0 < 1$, the disease will eventually vanish from the population, whereas if ${\mathcal {R}}_0 > 1$, an outbreak will ensue. To reinforce our findings, we provide computer simulations as supplementary evidence.

Appendix A: Proof of Theorem 1

The dynamics of the population size N(t) can be described by

$$\begin{aligned} d N(t) = [\mu _1 - \mu _1 N(t) - \mu _2 I_d(t)] d t. \end{aligned}$$

(49)

It follows from (49) and (5) that

$$\begin{aligned} d N(t) \ge \left[ \mu _1- (\mu _1+\mu _2) N(t)\right] d t, \quad \text{ for } \text{ all }\quad t \le \tau _{2}. \end{aligned}$$

Integrating and multiplying both sides by $\exp {\left[ \left( \mu _1+\mu _2\right) t\right] }$, yields that

$$\begin{aligned} N(t)-\dfrac{\mu _1}{\mu _1+\mu _2} \ge \left( N(0)-\dfrac{\mu _1}{\mu _1+\mu _2}\right) \exp {\left[ -\left( \mu _1+\mu _2\right) t\right] }, \quad \text{ for } \text{ all }\quad t \le \tau _{2}. \end{aligned}$$

For $\omega \in \left( \tau _{2}<\infty \right)$ and $t=\tau _{2}$, $N(\tau _{2})=\mu _1/\left( \mu _1+\mu _2\right)$, we have

$$\begin{aligned} 0 \ge \left( N(0) -\dfrac{\mu _1}{\mu _1+\mu _2}\right) \exp {\left[ \left( \mu _1+\mu _2\right) \tau _{2}\right] }>0. \end{aligned}$$

That is a contradiction. The solution $Z(t)=(S(t), I_d(t), R_w(t))$ starting from $Z(0) \in \Omega$ is proven to be global, positive, and verifies $N(t)>\mu _1/\left( \mu _1+\mu _2\right)$. Using (49), one obtains $d N(t) \le \left[ \mu _1-\mu _1 N(t)\right] d t$. Multiplying the two sides by $\exp {(\mu _1 t)}$ and integrating yields $N(t)-1 \le \left[ N(0)-1 \right] \exp {(-\mu _1 t)}$, which implies, that if $Z(0) \in \Omega$, then $N(t) \le 1$. Thus, the domain $\Omega$ is positively invariant.