Skip to main content
SpringerLink
Log in

A new numerical method for solving semilinear fractional differential equation

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

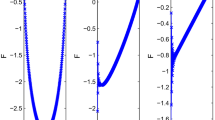
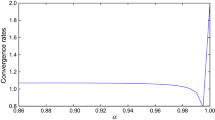
Abstract

The fractional differential equation has been used to describe many phenomenons in almost all applied sciences, such as fluid flow in porous materials, anomalous diffusion transport, acoustic wave propagation in viscoelastic materials, and others. In some cases, it is complicated to find the analytical solution of a fractional differential equation, so there are some special techniques to approximate the solution. In this paper, a new collocation method for a class of semilinear fractional differential equations has been constructed. The convergence properties of the proposed methods and the computational complexity are treated. Some numerical experiments are presented to validate the theoretical results. The proposed numerical method provides an alternative way to study some physical phenomena by fractional differential equations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Baskonus, H.M., Bulut, H.: On the numerical solutions of some fractional ordinary differential equations by fractional Adams-Bashforth-Moulton method. Open Math. 13(1), 547–556 (2015)

    Article  MathSciNet  Google Scholar 

  2. Cao, W., Zeng, F., Zhang, Z., Karniadakis, G.E.: Implicit-explicit difference schemes for nonlinear fractional differential equations with nonsmooth solutions. SIAM J. Sci. Comput. 38(5), 3070–3093 (2016)

    Article  MathSciNet  Google Scholar 

  3. Cao, W., Zhang, Z., Karniadakis, G.E.: Time-splitting schemes for fractional differential equations I: smooth solutions. SIAM J. Sci. Comput. 37(4), A1752–A1776 (2015)

    Article  MathSciNet  Google Scholar 

  4. Deng, J., Ma, L.: Existence and uniqueness of solutions of initial value problems for nonlinear fractional differential equations. Appl. Math. Lett. 23(6), 676–680 (2010)

    Article  MathSciNet  Google Scholar 

  5. Diethelm, K.: The Analysis of Fractional Differential Equations: an Application-Oriented Exposition Using Differential Operators of Caputo Type. Springer, Berlin (2010)

    Book  Google Scholar 

  6. Diethelm, K., Ford, N.J., Freed, A.D.: Detailed error analysis for a fractional Adams method. Numer. Algorithms 36(1), 31–52 (2004)

    Article  MathSciNet  Google Scholar 

  7. Gao, W., Baskonus, H.M., Shi, L.: New investigation of bats-hosts-reservoir-people coronavirus model and application to 2019-nCoV system. Adv. Differ. Equ. 2020(1), 1–11 (2020)

    Article  MathSciNet  Google Scholar 

  8. Gao, W., Günerhan, H., Baskonus, H.M.: Analytical and approximate solutions of an epidemic system of HIV/AIDS transmission. Alex. Eng. J. 59(5), 3197–3211 (2020)

    Article  Google Scholar 

  9. Gao, W., Veeresha, P., Prakasha, D.G., Baskonus, H.M.: New numerical simulation for fractional Benney-Lin equation arising in falling film problems using two novel techniques. Numer. Meth. Part Differ. Equ. 37(1), 210–243 (2021)

    Article  MathSciNet  Google Scholar 

  10. Garrappa, R.: On some explicit Adams multistep methods for fractional differential equations. J. Comput. Appl. Math. 229(2), 392–399 (2009)

    Article  MathSciNet  Google Scholar 

  11. Garrappa, R.: A family of Adams exponential integrators for fractional linear systems. Comput. Math. Appl. 66(5), 717–727 (2013)

    Article  MathSciNet  Google Scholar 

  12. Garrappa, R.: Numerical evaluation of two and three parameter Mittag-Leffler functions. SIAM J. Numer. Anal. 53(3), 1350–1369 (2015)

    Article  MathSciNet  Google Scholar 

  13. Garrappa, R.: Trapezoidal methods for fractional differential equations: Theoretical and computational aspects. Math. Comput. Simul. 110, 96–112 (2015)

    Article  MathSciNet  Google Scholar 

  14. Garrappa, R.: Neglecting nonlocality leads to unreliable numerical methods for fractional differential equations. Commun. Nonlinear Sci. Numer. Simul. 70, 302–306 (2019)

    Article  MathSciNet  Google Scholar 

  15. Garrappa, R., Popolizio, M.: On accurate product integration rules for linear fractional differential equations. J. Comput. Appl. Math. 235(5), 1085–1097 (2011)

    Article  MathSciNet  Google Scholar 

  16. Garrappa, R., Popolizio, M.: Exponential quadrature rules for linear fractional differential equations. Mediterr. J. Math. 12(1), 219–244 (2015)

    Article  MathSciNet  Google Scholar 

  17. Gorenflo, R., Loutchko, J., Luchko, Y.: Computation of the mittag-leffler function \({E}_{\alpha , \beta } (z)\) and its derivative. Fract. Calc. Appl. Anal. 5(4), 491–518 (2002)

    MathSciNet  MATH  Google Scholar 

  18. Goyal, M., Baskonus, H.M., Prakash, A.: Regarding new positive, bounded and convergent numerical solution of nonlinear time fractional HIV/AIDS transmission model. Chaos Solitons Fractals 139, 110096 (2020)

    Article  MathSciNet  Google Scholar 

  19. Hochbruck, M., Ostermann, A.: Exponential integrators. Acta Numer. 19, 209–286 (2010)

    Article  MathSciNet  Google Scholar 

  20. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam (2006)

    MATH  Google Scholar 

  21. Li, C., Chen, A., Ye, J.: Numerical approaches to fractional calculus and fractional ordinary differential equation. J. Comput. Phys. 230(9), 3352–3368 (2011)

    Article  MathSciNet  Google Scholar 

  22. Li, Y., Cao, Y., Fan, Y.: Generalized Mittag-Leffler quadrature methods for fractional differential equations. Comput. Appl. Math. 39(3), 1–16 (2020)

    MathSciNet  MATH  Google Scholar 

  23. Li, Y., Zhang, Y.: An efficient numerical method for nonlinear fractional differential equations based on the generalized Mittag-Leffler functions and Lagrange polynomials. Math. Meth. Appl. Sci. 44, 1–16 (2020)

    MathSciNet  Google Scholar 

  24. Lorenzo, C.F., Hartley, T.T.: Generalized functions for the fractional calculus (1999)

  25. Miller, K.S., Ross, B.: An Introduction to the Fractional Calculus and Fractional Differential Equations. Wiley, New York (1993)

    MATH  Google Scholar 

  26. Ostermann, A., Thalhammer, M., Wright, W.: A class of explicit exponential general linear methods. BIT 46(2), 409–431 (2006)

    Article  MathSciNet  Google Scholar 

  27. Podlubny, I.: Fractional Differential Equations. Academic press, New York (1999)

    MATH  Google Scholar 

  28. Prakash, A., Kumar, A., Baskonus, H.M., Kumar, A.: Numerical analysis of nonlinear fractional Klein-Fock-Gordon equation arising in quantum field theory via Caputo-Fabrizio fractional operator. Math. Sci. 80, 1–13 (2021)

    MathSciNet  Google Scholar 

  29. Zhao, J., Li, Y., Xu, Y.: A kind of product integration scheme for solving fractional ordinary differential equations. Appl. Numer. Math. 136, 279–292 (2019)

    Article  MathSciNet  Google Scholar 

  30. Zhao, L., Deng, W.: Jacobian-predictor-corrector approach for fractional differential equations. Adv. Comput. Math. 40(1), 137–165 (2014)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work is supported by the Fundamental Research Funds for the Central Universities (Grant Number: 2572020BC06).

Author information

Authors and Affiliations

  1. College of Science, Heilongjiang Bayi Agricultural University, Daqing, 163319, China

    Yufen Wei

  2. Institute of Cold Regions Science and Engineering, Northeast Forestry University, Harbin, 150040, China

    Ying Guo & Yu Li

  3. Department of Mathematics, Northeast Forestry University, Harbin, 150040, China

    Yu Li

Authors
  1. Yufen Wei
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ying Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yu Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yu Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wei, Y., Guo, Y. & Li, Y. A new numerical method for solving semilinear fractional differential equation. J. Appl. Math. Comput. 68, 1289–1311 (2022). https://doi.org/10.1007/s12190-021-01566-1

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-021-01566-1

Keywords

Search

Navigation