An epidemic dynamics model with limited isolation capacity

Abstract

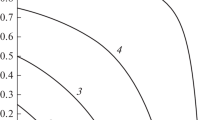
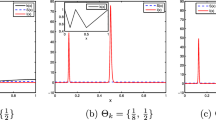
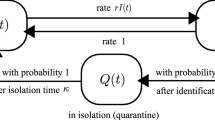
We consider a modified SIR model with a four-dimensional system of ordinary differential equations to consider the influence of a limited isolation capacity on the final epidemic size defined as the total number of individuals who experienced the disease at the end of an epidemic season. We derive the necessary and sufficient condition that the isolation reaches the capacity in a finite time on the way of the epidemic process, and show that the final epidemic size is monotonically decreasing in terms of the isolation capacity. We find further that the final epidemic size could have a discontinuous change at the critical value of isolation capacity below which the isolation reaches the capacity in a finite time. Our results imply that the breakdown of isolation with a limited capacity would cause a drastic increase of the epidemic size. Insufficient capacity of the isolation could lead to an unexpectedly severe epidemic situation, while such a severity would be avoidable with the sufficient isolation capacity.

Appendices

Appendix A Meaning of parameter $\varvec{\beta }$ for $\varvec{\mathscr {R}_0}$

In our model (1), the increase of infective population size in a sufficiently short interval $[t, t+\varDelta t]$ is given by

$$\begin{aligned}&\beta \dfrac{I(t)}{N-Q(t)}S(t)\varDelta t +\mathrm o(\varDelta t) \\&\quad = \beta \dfrac{I(t)}{S(t)+I(t)+R(t)}S(t)\varDelta t +\mathrm o(\varDelta t). \end{aligned}$$

Hence the expected number of new cases produced by an infective individual in $[t, t+\varDelta t]$ becomes

$$\begin{aligned} \beta \dfrac{S(t)}{S(t)+I(t)+R(t)}\varDelta t +\mathrm o(\varDelta t). \end{aligned}$$

(A1)

Since the basic reproduction number is defined as such an expected number of new cases when the infective individual contacts only susceptibles, it is regarded as the epidemiological index in an ideal situation for the production of new cases by the infective. In this sense, we can consider the supremum of the expected number of new cases in the ideal situation for the epidemic dynamics to derive the basic reproduction number (Seno 2022). For this reason, to derive the basic reproduction number $\mathscr {R}_0$ for our model (1), it is sufficient for us to consider only the epidemic dynamics at the isolation effective phase, since the isolation incapable phase corresponds to a non-ideal situation in which the number of susceptible individuals has become small. Above formula (A1) is monotonically increasing in terms of S(t), while sufficiently large S(t) makes $I(t)+R(t)$ small because of $S+I+Q+R = 1$. Therefore the supremum of (A1) can be given for $(S, I, R) = (N, 0, 0)$, which makes it $\beta \varDelta t+\mathrm o(\varDelta t)$. This result indicates that $\beta$ in our model (1) corresponds to the supremum of the expected number of new cases produced by an infective per unit time. Remark that, in the actual epidemic dynamics even with our model (and generally with any other model), any temporal change in the other subpopulation sizes makes the expected number of new cases produced by an infective smaller, that is, the expected number of new cases per unit time temporally changes necessarily below the supremum.

Furthermore, the mathematically obtained basic reproduction number in general is given by a formula expressing the product of such a supremum of the expected number of new cases per unit time and the expected duration of infectivity for an infective. Also from this viewpoint, $\beta$ in our model can have the meaning of the supremum of the expected number of new cases produced by an infective per unit time, because the expected duration of infectivity for an infective at the isolation effective phase is given by $1/(\gamma +\sigma _0)$ for our model (1).

Appendix B Derivation of the conserved quantity at each phase

The isolation effective phase

When the isolation never reaches the capacity in a finite time due to a sufficient isolation capacity for the epidemic dynamics, system (2) always follows the isolation effective phase with $\hat{\sigma }(q)=\hat{\sigma }_{0}$. In this case, from the equations in (2), we can derive the following differential equations:

$$\begin{aligned} \frac{di}{ds}=-1+\frac{1-q}{\mathscr {R}_0 s}, \end{aligned}$$

(B2)

where we used the equality $\hat{\sigma }_0+\hat{\gamma }=1$, and

$$\begin{aligned} \frac{dq}{dr}=\frac{\ \hat{\sigma }_0}{\hat{\gamma }}. \end{aligned}$$

(B3)

From (B3), we can obtain the following relation between q and r:

$$\begin{aligned} q=\frac{\ \sigma _0}{\gamma }r, \end{aligned}$$

(B4)

where we used $q(0)=r(0)=0$. Since $s+i+q+r=1$, equation (B4) becomes

$$\begin{aligned} 1-q=\frac{s+i+\gamma /\sigma _0}{1+\gamma /\sigma _0}. \end{aligned}$$

(B5)

Substituting (B5) for (B2), we can derive the following ordinary differential equation of i in terms of s:

$$\begin{aligned} \frac{d}{ds}\left( is^{-\sigma _0/\beta }\right) =s^{-\sigma _0/\beta } \Big ( -1+\frac{1}{\beta /\sigma _0} \Big ) +\frac{s^{-1-(\sigma _0/\beta )} }{\beta /\gamma }. \end{aligned}$$

We can easily solve this ordinary differential equation, and get relation (3), making use of $i(0)=i_0$, $s(0)=s_0$, and $i_0+s_0=1$.

The isolation incapable phase

Once the isolation reaches the capacity in a finite time on the way of the epidemic process with an insufficient isolation capacity, system (2) comes to follow the isolation incapable phase. In this case, from the first and second equations of (2), we can derive the following differential equation:

$$\begin{aligned} \frac{di}{ds}=-1+\frac{1-q_{\textrm{max}}}{\mathscr {R}_0 \left( 1+\sigma _0/\gamma \right) s}. \end{aligned}$$

(B6)

We can easily solve (B6) and get the relation

$$\begin{aligned} i(\hat{t})=-s(\hat{t})+ \frac{\,\gamma \,}{\beta } (1-q_{\textrm{max}}) \ln s(\hat{t})+C \end{aligned}$$

(B7)

with an undetermined constant C. For $\hat{t}=t^{\star }$, we have

$$\begin{aligned} C=s(t^{\star })+i(t^{\star })-\frac{\,\gamma \,}{\beta } (1-q_{\textrm{max}}) \ln s(t^{\star }). \end{aligned}$$

(B8)

Making use of (B8) for (B7), we can get equation (4) that gives the conserved quantity at the isolation incapable phase.

Appendix C Proof for theorem 5.1 and Corollaries 5.1.1 and 5.1.2

From equation (3), when the isolation never reaches the capacity, we have the equation

$$\begin{aligned} s^{-}_{\infty }=F(s^{-}_{\infty }):=-\frac{\gamma }{\ \sigma _0}+\Big ( \frac{s^{-}_{\infty }}{s_0} \Big )^{\sigma _0/\beta }\Big (1+\frac{\gamma }{\ \sigma _0}\Big ), \end{aligned}$$

(C9)

since $i(\hat{t})\rightarrow 0$ as $\hat{t}\rightarrow \infty$. We have $F(0)=-\gamma /\sigma _0$, $F(s_0)=1$, and $F'(s)>0$ for $s\in (0,s_0)$. Hence F(s) is a monotonically increasing, continuous and differentiable function of $s\in (0,s_0)$, which satisfies that $F(0)<0$ and $F(s_0)>s_0$. Further we can easily find that F(s) is linear if $\sigma _0/\beta =1$, and otherwise it is alternatively convex or concave for $s\in (0,s_0)$. Therefore we find that equation (C9) has a unique root $s^{-}_{\infty } \in (0,s_0)$, and $F(s)<s$ for $s \in (0,s^{-}_{\infty })$, while $F(s)>s$ for $s \in (s^{-}_{\infty }, s_0)$.

On the other hand, from $s^{-}_{\infty }+q^{-}_{\infty }+r^{-}_{\infty }=1$ and $q^{-}_{\infty }=(\sigma _0/\gamma )r^{-}_{\infty }$ by (B4) in Appendix B, we find that $s^{-}_{\infty }=1-(1+\gamma /\sigma _0)q^{-}_{\infty }$. Making use of this relation, we find that equation (C9) is equivalent to the following equation:

$$\begin{aligned} q^{-}_{\infty }=1-\Big ( \frac{s^{-}_{\infty }}{s_0} \Big )^{\sigma _0/\beta }. \end{aligned}$$

(C10)

It must be satisfied that $q^{-}_{\infty } \le q_{\textrm{max}}$ in the case where $q(\hat{t})$ never reaches $q_{\textrm{max}}$ for any $\hat{t}>0$. Since $q(\hat{t})$ is monotonically increasing in terms of $\hat{t}$, if $q^{-}_{\infty } \le q_{\textrm{max}}$, the isolation does not reach the capacity for any $\hat{t}>0$. Therefore, if and only if $q^{-}_{\infty } \le q_{\textrm{max}}$, the isolation does not reach the capacity for any $\hat{t}>0$.

Consequently we find that, if and only if $q^{-}_{\infty }> q_{\textrm{max}}$, the isolation reaches the capacity at $\hat{t}=t^{\star }<\infty$. From (C9) and (C10), we can derive the following condition equivalent to $q^{-}_{\infty }> q_{\textrm{max}}$:

$$\begin{aligned} s^{-}_{\infty }<1-q_{\textrm{max}}\Big ( 1+\frac{\gamma }{\ \sigma _0}\Big ). \end{aligned}$$

(C11)

Since $s^{-}_{\infty }>0$, we note that this inequality holds only if $q_{\textrm{max}}<1/(1+\gamma /\sigma _0)$. Hence if $q_{\textrm{max}}\ge 1/(1+\gamma /\sigma _0)$, inequality (C11) does not hold and we necessarily have $q^{-}_{\infty } \le q_{\textrm{max}}$, so that the isolation does not reach the capacity for any $\hat{t}>0$. From the nature of the function F(s) shown in the above, condition (C11) is equivalent to the condition that $F(s)>s$ for $s=1-q_{\textrm{max}}(1+\gamma /\sigma _0)$. This leads to condition (5) in Theorem 5.1.

On the other hand, from condition (C11), we can define the critical value for the isolation capacity $q_c$ as $q_c:= (1-s_\infty ^-)/(1+\gamma /\sigma _0)$ such that condition (C11) is satisfied if and only if $q_{\textrm{max}} < q_c$, which becomes necessary and sufficient for the isolation to reach the capacity in a finite time. Substituting $s_\infty ^-=1-q_c (1+\gamma /\sigma _0)$ for the equation $F(s_\infty ^-) = s_\infty ^-$, we can get equation (6). Then the uniqueness of $q_c$ follows that of $s_\infty ^-$ shown in the above.

Appendix D Proof for corollary 5.1.3

From (6), we can easily derive the following derivative of $q_{c}$ in terms of $1/\sigma _0$:

$$\begin{aligned} \begin{aligned} \frac{\partial q_{c}}{\partial (1/\sigma _0)}=\frac{\sigma _0^{2}\gamma q_{c}+\sigma _0\beta \left\{ \sigma _0-q_{c}\left( \sigma _0+\gamma \right) \right\} \ln (1-q_{c}) }{-\sigma _0\left( \sigma _0+\gamma \right) +\beta \left\{ \sigma _0-q_{c}\left( \sigma _0+\gamma \right) \right\} /(1-q_{c}) }. \end{aligned} \end{aligned}$$

(D12)

As we can easily find from (6) that $q_{c}\rightarrow 1-s_0$ as $1/\sigma _0\rightarrow +0$, we have

$$\begin{aligned} \frac{\partial q_{c}}{\partial \left( 1/\sigma _0\right) } \bigg \vert _ {\left( 1/\sigma _0, q_{c})\rightarrow (+0, 1-s_0\right) }>0 ~~ \iff ~~ \frac{\,\beta \,}{\gamma }> \frac{s_0-1}{s_0\ln s_0}. \end{aligned}$$

(D13)

Next, to find the sign of (D12) for sufficiently large value of $1/\sigma _0$, we use the Maclaurin expansion in terms of $\sigma _0$ and get

$$\begin{aligned} \frac{\partial q_{c}}{\partial \left( 1/\sigma _0\right) } = (1-q_{c})\ln (1-q_{c})\sigma _0+ \text{ o }(\sigma _0). \end{aligned}$$

Since $(1-q_{c})\ln (1-q_{c})<0$ for $q_{c}\in (0,1)$, the sign of (D12) must be necessarily negative for sufficiently large value of $1/\sigma _0$. As a consequence, $q_{c}$ is monotonically decreasing for sufficiently large value of $1/\sigma _0$.

Since $q_{c}$ is continuous in terms of $1/\sigma _0$, $q_{c}$ is monotonically increasing for a sufficiently small value of $1/\sigma _0$ if condition (D13) is satisfied. Then, $q_{c}$ has at least one extremal maximum for a finite value of $1/\sigma _0$. It is easily seen that $(s_0-1)/(s_0\ln s_0)>1$ for any $s_0\in (0,1)$.

On the other hand, the following equation must be satisfied at the extremum that makes derivative (D12) zero:

$$\begin{aligned} 1+\dfrac{\gamma }{\ \sigma _0} = \dfrac{1}{\ q_c\, }+\dfrac{\gamma /\beta }{\ln (1-q_c)}. \end{aligned}$$

(D14)

We can easily prove that the right side of (D14) is less than 1 for any $q_c\in (0, 1)$ if $\beta /\gamma \le 1$. Since the left side of (D14) is always greater than 1, this means that equation (D14) cannot hold for $\beta /\gamma \le 1$. Hence, derivative (D12) cannot become zero if $\beta /\gamma \le 1$.

Therefore, $\beta /\gamma > 1$ is necessary for the existence of a certain value of $1/\sigma _0 > 0$ to maximize $q_c$. At the same time, this result means that, when $\beta /\gamma \le 1$, derivative (D12) cannot change the sign at any value of $1/\sigma _0$. Then from the above arguments, it must be negative, so that $q_c$ is monotonically decreasing in terms of $1/\sigma _0$ when $\beta /\gamma \le 1$.

Appendix E Derivation of the final size equation

Final size equation for $\varvec{q_{\textrm{max}}\ge q_{c}}$

By applying $\hat{t}\rightarrow \infty$ for equation (3), we get the following equation:

$$\begin{aligned} (s^{-}_{\infty })^{-\sigma _0/\beta }\Big (s^{-}_{\infty }+\frac{\gamma }{\ \sigma _0}\Big ) = \left( s_0\right) ^{-\sigma _0/\beta }\Big (1+\frac{\gamma }{\ \sigma _0}\Big ), \end{aligned}$$

(E15)

where we used $i(\hat{t})\rightarrow 0$ as $\hat{t}\rightarrow \infty$. The final epidemic size is given by $z_\infty ^-=q^{-}_{\infty }+ r^{-}_{\infty }=1-s^{-}_{\infty }$. Making use of $s^{-}_{\infty }=1-z_\infty ^-$ for (E15), we can get equation (8) which determines the final epidemic size when the isolation never reaches the capacity.

Final size equation for $\varvec{q_{\textrm{max}}<q_{c}}$

By applying $\hat{t}\rightarrow \infty$ for equation (4), we can get the following equation:

$$\begin{aligned} s^{+}_{\infty }=s(t^{\star })+i(t^{\star })+ \frac{\,\gamma \,}{\beta }(1-q_{\textrm{max}}) \ln \frac{s^{+}_{\infty }}{s(t^{\star })}, \end{aligned}$$

(E16)

where we used $i(\hat{t})\rightarrow 0$ as $\hat{t}\rightarrow \infty$. Now, from the equality $s(\hat{t})+i(\hat{t})=1-\left\{ q(\hat{t})+ r(\hat{t})\right\}$ and (B4) derived in Appendix B, we have

$$\begin{aligned} s(\hat{t})+i(\hat{t})=1-q(\hat{t})\Big (1+\frac{\gamma }{\ \sigma _0}\Big ). \end{aligned}$$

(E17)

For the continuity of the solution at $\hat{t}=t^{\star }$, we have $s(\hat{t})=s(t^{\star })$, $i(\hat{t})=i(t^{\star })$, and $q(t^{\star })=q_{\textrm{max}}$. Then equations (3) and (E17) become

$$\begin{aligned} s(t^{\star })+i(t^{\star })&=-\frac{\gamma }{\ \sigma _0}+\Big \{\frac{s(t^{\star })}{s_0}\Big \} ^{\sigma _0/\beta }\Big (1+\frac{\gamma }{\ \sigma _0}\Big ); \end{aligned}$$

(E18)

$$\begin{aligned} s(t^{\star })+i(t^{\star })&=1-q_{\textrm{max}}\Big (1+\frac{\gamma }{\ \sigma _0}\Big ). \end{aligned}$$

(E19)

We can solve parallel equations (E18) and (E19) in terms of $s(t^{\star })$ and $i(t^{\star })$,

$$\begin{aligned} s(t^\star )&= (1-q_{\textrm{max}} )^{\beta /\sigma _0}s_0; \quad i(t^\star ) =1-s(t^\star )-q_{\textrm{max}}\Big (1+\frac{\gamma }{\ \sigma _0}\Big ), \end{aligned}$$

(E20)

and then substitute them for (E16). As a result, we can get the equation

$$\begin{aligned}&\frac{\beta /\gamma }{1-q_{\textrm{max}}}\Big \{ s^{+}_{\infty }-1+q_{\textrm{max}}\Big (1+\frac{\gamma }{\ \sigma _0}\Big ) \Big \} \nonumber \\&\quad =\ln s^{+}_{\infty }-\ln s_0- \frac{\beta }{\ \sigma _0}\ln (1-q_{\textrm{max}}). \end{aligned}$$

(E21)

When the isolation reaches the capacity at any finite time, the final epidemic size is defined by $z_\infty ^+=q_{\textrm{max}}+ r^{+}_{\infty }=1-s^{+}_{\infty }$. Thus, making use of $s^{+}_{\infty }=1-z_\infty ^+$ for (E21), we can get equation (9).

Appendix F Proof for the unique existence of the final epidemic size

Unique existence of $\varvec{z_\infty ^-}$

The left side of equation (8) is a function of $z_\infty ^-$, which we denote here by $A(z_\infty ^-)$. The right side of (8) is a positive constant $B_{0}$ independent of $z_\infty ^-$. The function A(z) is continuous and differentiable for $z\in (1-s_0,1)$, satisfying that

$$\begin{aligned} A(1-s_0)&= \left( s_0\right) ^{-\sigma _0/\beta } \Big ( s_0+\frac{\gamma }{\ \sigma _0} \Big ) < B_0; \quad \lim _{z\rightarrow 1-0}A(z) = \infty > B_0. \end{aligned}$$

Hence, there exists at least one root of the equation $A(z)=B_{0}$ for $z\in (1-s_0,1)$.

We can easily find that the function A(z) is monotonically increasing or has a unique extremal minimum in $(1-s_0,1)$. When A(z) is monotonically increasing for $z\in (1-s_0,1)$, it must have a unique intersection with the horizontal line $B_{0}$ in $(1-s_0,1)$. Even when A(z) has a unique extremal minimum for $z\in (1-s_0,1)$, it has a unique intersection with the horizontal line $B_{0}$ since $A(1-s_0)<B_{0}$. Thus in both cases, the equation $A(z)=B_{0}$ has a unique root in $(1-s_0,1)$. As a result, the final epidemic size $z_\infty ^-\in (1-s_0,1)$ is uniquely determined by equation (8).

Unique existence of $\varvec{z_\infty ^+}$

To prove that the final epidemic size $z_\infty ^+$ is uniquely determined by equation (9), let us consider the existence of a root for the equation $G(s)=0$ where

$$\begin{aligned} G(s):= s-\big \{ s(t^{\star })+i(t^{\star })\big \}-\frac{\,\gamma \,}{\beta } (1-q_{\textrm{max}}) \ln \frac{s}{s(t^{\star })}. \end{aligned}$$

(F22)

From (4) in Sect. 4 and (E16) in Appendix E, the equation $G(1-z_\infty ^+)=0$ is mathematically equivalent to final size equation (9). Since $s(\hat{t})$ is monotonically decreasing as time passes, we have $s(\hat{t})<s(t^{\star })$ for $\hat{t}>t^{\star }$. Hence we consider G(s) hereafter for $s\in (0, s(t^{\star }))$. The function G(s) is continuous and differentiable for $s\in (0,s(t^{\star }))$. Moreover, it satisfies that $\displaystyle \lim _{s\rightarrow +0}G(s)=\infty >0$, and $G(s(t^{\star }))=-i(t^*)<0$. From these facts, the equation $G(s)=0$ has at least one root in $(0,s(t^{\star }))$. Further we can easily find that G(s) is monotonically decreasing or has a unique extremal minimum in $(0,s(t^{\star }))$. When G(s) is monotonically decreasing for $s\in (0,s(t^{\star }))$, the equation $G(s)=0$ has a unique root in $(0,s(t^{\star }))$. Even when G(s) has a unique extremal minimum in $(0,s(t^{\star }))$, it has a unique root in $(0,s(t^{\star }))$, because the extremum value of G must be negative since $G(s(t^{\star }))<0$. Hence in both cases, the equation $G(s)=0$ has a unique root $s = s^{+}_{\infty }\in (0,s(t^{\star }))$. Therefore, equation (9) determines a unique final epidemic size $z_\infty ^+ \in (1-s(t^{\star }),1)$. This is because $s^{+}_{\infty }=1-z_\infty ^+$ and $z_\infty ^+=1-s^{+}_{\infty }\in (1-s(t^{\star }),1)$ where $1-s(t^{\star })=i(t^{\star })+q_{\textrm{max}}(1+\gamma /\sigma _0)>q_{\textrm{max}}(1+\gamma /\sigma _0) > q_{\textrm{max}}$ from (E19), and $s(t^{\star })$ is given by (E20) in Appendix E.

Appendix G Proof for Theorem 7.1

In order to prove Theorem 7.1, we use two lemmas.

Lemma G.1

It holds that $z_\infty ^+\ge q_{c}\left( 1+\gamma /\sigma _0\right) \ge z_\infty ^-$.

Proof

The proof is given straightforward from the arguments in the proof for Theorem 5.1 and its corollaries, given in Appendix C. From (C11), the condition $q^{-}_{\infty } \le q_{\textrm{max}}$ is equivalent to

$$\begin{aligned} s^{-}_{\infty }\ge 1-q_{\textrm{max}}\Big ( 1+\frac{\gamma }{\ \sigma _0}\Big ), \end{aligned}$$

(G23)

where $s^{-}_{\infty }$ is the root of (E15), and subsequently $q^{-}_{\infty }$ is given by (C10). Thus, when and only when condition (G23) is satisfied, the isolation never reaches the capacity, so that the epidemic dynamics is always at the isolation effective phase. Inversely, when and only when condition (G23) is unsatisfied, the epidemic dynamics enters in the isolation incapable phase in a finite time.

Thus, for the value $s(t^{\star })$ at the moment when the isolation incapable phase begins, it must hold that

$$\begin{aligned} s(t^{\star })< 1-q_{\textrm{max}}\Big ( 1+\frac{\gamma }{\ \sigma _0}\Big ). \end{aligned}$$

The value $s(\hat{t})$ is monotonically decreasing in terms of time since $ds/d\hat{t}$ is negative for any $\hat{t}>0$. Hence we have $s^{+}_{\infty }<s(t^{\star })$ where $s^{+}_{\infty }$ is the root of (E21) at the isolation incapable phase. Therefore, we have

$$\begin{aligned} s^{+}_{\infty }< 1-q_{\textrm{max}}\Big ( 1+\frac{\gamma }{\ \sigma _0}\Big ). \end{aligned}$$

(G24)

Since $z_\infty ^-=1-s^{-}_{\infty }$, these arguments indicate that, when and only when the isolation never reaches the capacity, we have $z_\infty ^-\le q_{\textrm{max}} (1+{\gamma }/{\sigma _0} )$ from (G23). Since this condition must hold for any $q_{\textrm{max}} \ge q_{c}$ from Corollary 5.1.1, and since $z_\infty ^-$ is independent of $q_{\textrm{max}}$, we find that $z_\infty ^-\le q_{c} ( 1+{\gamma }/{\sigma _0} )$.

On the other hand, when the isolation reaches the capacity at a finite time with $q_{\textrm{max}}<q_{c}$, we have $z_\infty ^+> q_{\textrm{max}} ( 1+{\gamma }/{\sigma _0} )$ from (G24). Since this condition must hold for any $q_{\textrm{max}}<q_{c}$, we have $z_\infty ^+\ge q_{c} ( 1+{\gamma }/{\sigma _0} )$. $\square$

Lemma G.2

It holds that $z_\infty ^-= q_{c}\left( 1+\gamma /\sigma _0\right)$.

Proof

Substituting $z_\infty ^-= q_{c}\left( 1+\gamma /\sigma _0\right)$ for (8) and taking account of (6) in Corollary 5.1.1, we can easily find that equation (E21) holds. Since $z_\infty ^-$ is uniquely determined as the root of (8), we can result in this lemma. $\square$

Now, equation (9) can be rewritten as

$$\begin{aligned} q_{\textrm{max}}\Big (1+\frac{\gamma }{\ \sigma _0}\Big )-z_\infty ^+ =\frac{\,\gamma \,}{\beta }(1-q_{\textrm{max}}) \ln \frac{1-z_\infty ^+}{s_0(1-q_{\textrm{max}})^{\beta /\sigma _0}}. \end{aligned}$$

(G25)

Taking the limit as $q_{\textrm{max}} \rightarrow q_{c}$ for (G25), we have the following equation with respect to $z^{\dagger }_{\infty }$ from Lemma G.2 and (8):

$$\begin{aligned} H(z^{\dagger }_{\infty }):=z_\infty ^--z^{\dagger }_{\infty } -\frac{\,\gamma \,}{\beta }(1-q_{c}) \ln \frac{1-z^{\dagger }_{\infty }}{1-z_\infty ^-}=0. \end{aligned}$$

It is easily found that $H(z_\infty ^-)=0$ and $\displaystyle \lim _{z \rightarrow 1-0}H(z)=\infty$. The derivative of H(z) becomes

$$\begin{aligned} H'(z) = -1 +\frac{\,\gamma \,}{\beta }\frac{1-q_{c}}{1-z}, \end{aligned}$$

which is monotonically increasing in terms of $z\in (z_\infty ^-, 1)\subset (0, 1)$ with $H'(z)\rightarrow \infty$ as $z\rightarrow 1-0$. If $H'(z_\infty ^-) \ge 0$, then $H(z)>0$ for any $z\in (z_\infty ^-, 1)$. In this case, the root of $H(z) = 0$ in $[z_\infty ^-, 1]$ is only $z=z_\infty ^-$. In contrast, if $H'(z_\infty ^-)<0$, there exists a unique value $\eta \in (z_\infty ^-, 1)$ such that $H'(z) < 0$ for $z\in (z_\infty ^-, \eta )$ and $H'(z) > 0$ for $z\in (\eta , 1)$. This means that $H(z) < 0$ for $z\in (z_\infty ^-, \eta )$, and H(z) is monotonically increasing for $z\in (\eta , 1)$ with $\displaystyle \lim _{z \rightarrow 1-0}H(z)=\infty$. Therefore we have a unique value $\zeta \in (\eta , 1)\subset (z_\infty ^-, 1)$ such that $H(\zeta )=0$, because H(z) is continuous in $(z_\infty ^-, 1)$.

On the other hand, from (9), we can derive

$$\begin{aligned} \frac{\partial z_\infty ^+}{\partial q_{\textrm{max}}}=\frac{1+(\gamma /\beta ) \ln \left[ (1-z_\infty ^+)/\left\{ s_0 (1-q_{\textrm{max}})^{\beta /\sigma _0}\right\} \right] }{1-(\gamma /\beta )(1-q_{\textrm{max}})/ (1-z_\infty ^+)}. \end{aligned}$$

Then we have

$$\begin{aligned}&\frac{ \partial z_\infty ^+}{\partial q_{\textrm{max}}} \bigg \vert _{(q_{\textrm{max}}, z_\infty ^+)=(q_{c}, z_\infty ^-) }= \frac{1}{1-(\gamma /\beta )(1-q_{c})/(1-z_\infty ^-)}\nonumber \\&\quad =- \frac{1}{H'(z_\infty ^-)}. \end{aligned}$$

(G26)

Hence we find that, if $H'(z_\infty ^-)<0$, derivative (G26) becomes positive. Thus, if $z^{\dagger }_{\infty }=z_\infty ^+$ with $H'(z_\infty ^-)<0$, $z_\infty ^+$ must be smaller than $z_\infty ^-$ for $q_{\textrm{max}}$ less than and sufficiently near $q_{c}$ because $z_\infty ^+$ is continuous and differentiable for $q_{\textrm{max}} \in (0, q_{c})$ and derivative (G26) is positive. This is contradictory to the result of Lemma G.1. Therefore, if $H'(z_\infty ^-)<0$, $z^{\dagger }_{\infty }$ must be greater than $z_\infty ^-$.

The condition $H'(z_\infty ^-)<0$ is equivalent to the following:

$$\begin{aligned} \frac{\,\gamma \,}{\beta }<1~~ \text {and}~~ q_{c}<q_{cc}:=\frac{1-\gamma /\beta }{1-\gamma /\beta +\gamma /\sigma _0}. \end{aligned}$$

(G27)

From $q_{\textrm{max}}<q_{c}$ and (6), the second inequality of (G27) is equivalent to

$$\begin{aligned} 1-q_{cc}\Big (1+\frac{\gamma }{\ \sigma _0}\Big )<s_0\left( 1-q_{cc}\right) ^{ \beta /\sigma _0}. \end{aligned}$$

This inequality results in the second condition of (10). If $H'(z_\infty ^-)\ge 0$, $z^{\dagger }_{\infty }$ must be $z_\infty ^-$ since the equation $H(z)=0$ has the unique root $z=z_\infty ^-$ in $[z_\infty ^-,1]$ and derivative (G26) is non-positive with no contradiction. These arguments prove the theorem.

Appendix H Proof for Corollary 6.1.1

$\varvec{(1/\sigma _0)}$-dependence of $\varvec{z_\infty ^-}$

Equation (8) to determine $z_\infty ^-$ can be rewritten as

$$\begin{aligned} \ln \dfrac{1-z_\infty ^-}{s_0} = U(z_\infty ^-) := \dfrac{\beta }{\,\sigma _0}\ln \Big (1-\dfrac{z_\infty ^-}{1+\gamma /\sigma _0}\Big ). \end{aligned}$$

(H28)

According to the function U(z) for $z\in (1-s_0, 1)$, we can easily find that U(z) is monotonically decreasing in terms of $z\in (1-s_0, 1)$, and so is the left-hand function of equation (H28), $\ln \{ (1-z)/s_0\}$. As already shown in Theorem 6.1, U(z) and $\ln \{ (1-z)/s_0\}$ necessarily have a unique intersection in $(1-s_0, 1)$, which gives $z_\infty ^-$. Since $U(1-s_0) <0$ and $|U(1)|<\infty$, it is satisfied that $U(z)>\ln \{ (1-z)/s_0\}$ for $z\in (1-s_0, z_\infty ^-)$ and $U(z)<\ln \{ (1-z)/s_0\}$ for $z\in (z_\infty ^-, 1)$.

On the other hand, we can easily show that

$$\begin{aligned}&\displaystyle \lim _{1/\sigma _0\rightarrow +0}\dfrac{\partial U(z)}{\partial (1/\sigma _0)} = \beta \ln (1 -z ) < 0; \quad \lim _{1/\sigma _0\rightarrow \infty }\dfrac{\partial U(z)}{\partial (1/\sigma _0)} =0; \\&\quad \dfrac{\partial ^2 U(z)}{\partial (1/\sigma _0)^2} > 0. \end{aligned}$$

Hence ${\partial U(z)}/{\partial (1/\sigma _0)} < 0$ for $1/\sigma _0 >0$, that is, the value of U(z) is monotonically decreasing in terms of $1/\sigma _0$ for $z\in (1-s_0, 1)$. Therefore, the intersection of U(z) and $\ln \{ (1-z)/s_0\}$ monotonically decreasing in $(1-s_0, 1)$ must move toward the larger z as $1/\sigma _0$ gets larger. This means that $z_\infty ^-$ is monotonically increasing in terms of $1/\sigma _0$.

$\varvec{(1/\sigma _0)}$-dependence of $\varvec{z_\infty ^+}$

Equation (9) to determine $z_\infty ^+$ can be rewritten as

$$\begin{aligned} \dfrac{\beta K}{\,\sigma _0} = W(z_\infty ^+) := \ln \dfrac{1-z_\infty ^+}{s_0}+\dfrac{\,\beta \, }{\gamma }\dfrac{z_\infty ^+ - q_{\textrm{max}}}{1-q_{\textrm{max}}}, \end{aligned}$$

(H29)

where $K:= {q_{\textrm{max}}}/ ({1-q_{\textrm{max}}} )+\ln (1-q_{\textrm{max}} ) > 0$ for $q_{\textrm{max}}\in (0, 1)$. We can easily find that

$$\begin{aligned} W(1-s(t^\star ))&= \ln \dfrac{s(t^\star )}{s_0} +\dfrac{\,\beta \, }{\gamma } \dfrac{1-s(t^\star )-q_{\textrm{max}}}{1-q_{\textrm{max}}}\\&> \dfrac{\beta }{\,\sigma _0}\ln (1-q_{\textrm{max}} )+\dfrac{\,\beta \, }{\gamma }\dfrac{(\gamma /\sigma _0)q_{\textrm{max}}}{1-q_{\textrm{max}}} = \dfrac{\beta K}{\sigma _0} > 0, \end{aligned}$$

making use of $s(t^\star ) = (1-q_{\textrm{max}} )^{\beta /\sigma _0}s_0$ and the inequality that $1-s(t^{\star }) >q_{\textrm{max}}(1+\gamma /\sigma _0)$ which was shown at the end of Appendix F. Further it can be easily proved that W(z) is monotonically decreasing in terms of $z\in (1-s(t^\star ), 1)$ or alternatively has a unique extremal maximum in $(1-s(t^\star ), 1)$, with $\displaystyle \lim _{z\rightarrow 1-0} W(z) = -\infty$.

On the other hand, we find that condition (5) in Theorem 5.1 is mathematically equivalent to that $(\beta K/\sigma _0) < W(1-s(t^\star ))$. This is mathematically consistent with the definition of $z_\infty ^+$ which can exist only if condition (5) is satisfied when the isolation reaches the capacity in a finite time. Hence we can hereafter consider only $1/\sigma _0$ such that $(\beta K/\sigma _0) < W(1-s(t^\star ))$, since equation (9) is valid only under condition (5).

Then, from the nature of W(z) shown in the above, equation (H29) has a unique root $z_\infty ^+\in (1-s(t^\star ), 1)$, that is, a unique intersection of W(z) and the horizontal line $\beta K/\sigma _0$ in $(1-s(t^\star ), 1)$. When W(z) is monotonically decreasing in terms of $z\in (1-s(t^\star ), 1)$, the intersection moves toward the larger z as $1/\sigma _0$ gets smaller. Even when W(z) has a unique extremal maximum in $(1-s(t^\star ), 1)$, the intersection giving $z_\infty ^+\in (1-s(t^\star ), 1)$ must be on the decreasing part of curve W(z), since $W(1-s(t^\star )) > 0$ and $(\beta K/\sigma _0) < W(1-s(t^\star ))$. Hence, even in such a case, the intersection moves toward the larger z as $1/\sigma _0$ gets smaller. Consequently, these arguments prove that $z_\infty ^+$ is monotonically decreasing in terms of $1/\sigma _0$.