A comprehensive theoretical analysis of $^{12}\hbox {B}+{}^{58}\hbox {Ni}$ elastic scattering measured for the first time by using different density distributions, different nuclear potentials and different cluster approaches

Abstract

Recently, Zevallos et al [Phys. Rev. C 99, 064613 (2019)] measured, for the first time, the elastic scattering data of $^{12}\hbox {B}+{}^{58}\hbox {Ni}$ reaction at $E_{\text {Lab}} = 30.0$ and 33.0 MeV. For the first time, we show a comprehensive theoretical analysis of the experimental data of $^{12}\hbox {B}+{}^{58}\hbox {Ni}$ reaction. First, we propose alternative density distributions for the $^{12}\hbox {B}$ nucleus, and obtain the elastic scattering angular distributions of $^{12}\hbox {B} +{}^{58}\hbox {Ni}$ reaction with the help of these densities. Secondly, we calculate the elastic scattering cross-sections of $^{12}\hbox {B} + {}^{58}\hbox {Ni}$ reaction by using 13 different nuclear potentials to reveal alternative nuclear potentials. Finally, we examine cluster structures such as $\alpha +{}^{8}\hbox {Li}$ and $n+{}^{11}\hbox {B}$ of the $^{12}\hbox {B}$ nucleus by using a simple approach, and acquire elastic scattering cross-sections of $^{12}\hbox {B}+{}^{58}\hbox {Ni}$ reaction over these cluster approaches. We compare all the theoretical results with the experimental data, and discuss their similarities and differences. Also, we propose new equations of both normalisation constant and imaginary potential parameters for all the systems analysed.

Appendices

Appendix A: Density distributions

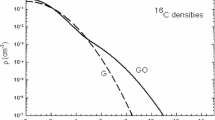
1.1 Density distributions of $^{12}\hbox {B}$ projectile

1.1.1 São Paulo density distribution

São Paulo density which has the two-parameter Fermi (2pF) shape [34] can be given by

$$\begin{aligned} \rho _{_{i}}(r)= & {} \frac{\rho _{0i}}{1+\text {exp}\left( \frac{r-R_{i}}{a_{i}}\right) }, \quad i=n,p \end{aligned}$$

(16)

$$\begin{aligned} R_{n}= & {} 1.49N^{1/3}-0.79, \quad a_{n}=0.47+0.00046N,\nonumber \\ \end{aligned}$$

(17)

$$\begin{aligned} R_{p}= & {} 1.81Z^{1/3}-1.12, \quad a_{p}=0.47-0.00083Z,\nonumber \\ \end{aligned}$$

(18)

where $R_{n(p)}$ and $a_{n(p)}$ are half-density radius and surface thickness parameter of neutron(proton), respectively. The São Paulo density is shown as SP in our study.

1.1.2 Fermi density distribution

Fermi density is in the same form as SP density distribution except for the values of $R_{n}$, $R_{p}$, $a_{n}$ and $a_{p}$. Thus, $R_{n(p)}$ and $a_{n(p)}$ values are derived from the following equations [35]:

$$\begin{aligned} R_{n}= & {} 0.953N^{1/3}+0.015Z+0.774, \nonumber \\ a_{n}= & {} 0.446+0.0072\left( \frac{N}{Z}\right) , \end{aligned}$$

(19)

$$\begin{aligned} R_{p}= & {} 1.322Z^{1/3}+0.007N+0.022, \nonumber \\ a_{p}= & {} 0.449+0.0071\left( \frac{Z}{N}\right) . \end{aligned}$$

(20)

Fermi density is shown as 2pF in our work.

1.1.3 Ngô–Ngô density distribution

Ngô–Ngô density distribution is parametrised as [36, 37]

$$\begin{aligned}&\rho _{_{i}}(r) = \frac{\rho _{0i}}{1+\text {exp}\left( \frac{r-C_{i}}{0.55}\right) }, \quad i=n,p \end{aligned}$$

(21)

$$\begin{aligned}&\rho _{_{0n}}=\frac{3}{4\pi } \frac{N}{A} \frac{1}{r_{0n}^{3}}, \quad \rho _{_{0p}}=\frac{3}{4\pi } \frac{Z}{A} \frac{1}{r_{0p}^{3}}, \end{aligned}$$

(22)

where C, the central radius, is

$$\begin{aligned} C=R\left( 1-\frac{1}{R^{2}}\right) , \,\,\,R=\frac{NR_{n}+ZR_{p}}{A}. \end{aligned}$$

(23)

The sharp radii of neutron and proton are formulated by

$$\begin{aligned} R_{n}= & {} r_{0n}A^{1/3}, \quad r_{0n}=1.1375+1.875\times 10^{-4}A, \nonumber \\\end{aligned}$$

(24)

$$\begin{aligned} R_{p}= & {} r_{0p}A^{1/3}, \quad \quad r_{0p}=1.128 \,\, \text {fm}. \end{aligned}$$

(25)

Ngô–Ngô density is shown as Ngo in our study.

1.1.4 Gupta density distribution 1

Gupta density distribution is shown as

$$\begin{aligned}&\rho _{_{i}}(r) = \frac{\rho _{0i}}{1+\text {exp}\left( \frac{r-R_{0i}}{a_{i}}\right) },\nonumber \\&\rho _{0i}=\frac{3A_{i}}{4\pi R_{0i}^{3}}\left( 1+\frac{\pi ^{2}a_{i}^{2}}{R_{0i}^{2}}\right) ^{-1}, \end{aligned}$$

(26)

where $R_{0i}$ and $a_{i}$ are taken as [38, 39]

$$\begin{aligned} R_{0i}= & {} 0.90106 + 0.10957A_{i} - 0.0013A_{i}^{2} \nonumber \\&+ 7.71458 \times 10^{-6} A_{i}^{3} - 1.62164 \times 10^{-8} A_{i}^{4}, \nonumber \\ \end{aligned}$$

(27)

$$\begin{aligned} a_{i}= & {} 0.34175+0.01234A_{i}\nonumber \\&-2.1864 \times 10^{-4}A_{i}^{2}+1.46388 \times 10^{-6} A_{i}^{3}\nonumber \\&-3.24263 \times 10^{-9}A_{i}^{4}. \end{aligned}$$

(28)

This density distribution is shown as G1 in our study.

1.1.5 Gupta density distribution 2

This density distribution is in the same form as G1 density except for the values of $R_{0i}$ and $a_{i}$ given in the following forms [40]:

$$\begin{aligned} R_{0i}= & {} 0.9543 + 0.0994A_{i} - 9.8851 \times 10^{-4} A_{i}^{2}\nonumber \\&+ 4.8399 \times 10^{-6} A_{i}^{3} - 8.4366 \times 10^{-9} A_{i}^{4}, \end{aligned}$$

(29)

$$\begin{aligned} a_{i}= & {} 0.3719 + 0.0086A_{i}-1.1898 \times 10^{-4}A_{i}^{2}\nonumber \\&+6.1678 \times 10^{-7} A_{i}^{3}-1.0721 \times 10^{-9}A_{i}^{4}. \end{aligned}$$

(30)

This density is shown as G2 in our study.

1.1.6 Wesolowski density distribution

Wesolowski [41] has showed different parameters of 2pF density parametrised by [42]

$$\begin{aligned} \rho _{0}= & {} \frac{3}{4\pi R_{0}^{3}}\left( 1+\frac{\pi ^{2}a^{2}}{R_{0}^{2}}\right) ^{-1},\,\,\, a=0.39 \,\text {fm}, \end{aligned}$$

(31)

$$\begin{aligned} R_{0}= & {} R{'}\left[ 1-\left( \frac{b}{R^{'}}\right) ^{2}+\frac{1}{3} \left( \frac{b}{R^{'}}\right) ^{6}+\cdots \right] ,\end{aligned}$$

(32)

$$\begin{aligned} R{'}= & {} \left[ 1.2-\frac{0.96}{A^{1/3}}\left( \frac{N-Z}{A}\right) \right] A^{1/3},\quad \,\,\, b=\frac{\pi }{\sqrt{3}}a. \nonumber \\ \end{aligned}$$

(33)

This density is shown as W in our work.

1.1.7 Schechter density distribution

Schechter and Canto [43] have reported another parameters of 2pF density distribution shown by

$$\begin{aligned} \rho _{0}=\frac{0.212}{1+2.66 A^{-2/3}}, \quad R_{0}=1.04A^{1/3}, \quad a=0.54 \,\, \text {fm}. \end{aligned}$$

(34)

This density is shown as S in our work.

1.1.8 Moszkowski density distribution

This density distribution proposed by Moszkowski [44] is in the form of 2pF density, and its parameters are given by

$$\begin{aligned} \rho _{0}=0.16 \,\, \text {nucl.}/\text {fm}^{3}, \quad R_{0}=1.15A^{1/3}, \quad a=0.50 \,\, \text {fm}. \end{aligned}$$

(35)

This density is shown as M in our study.

1.1.9 Density distribution of $^{58}\hbox {Ni}$ target nucleus

In the calculations, the elastic scattering of $^{12}\hbox {B}$ projectile by $^{58}\hbox {Ni}$ has been investigated. For this purpose, the density distribution of $^{58}\hbox {Ni}$ target is obtained by

$$\begin{aligned} \rho (r) = \frac{\rho _{0}}{1+\text {exp}\left( \frac{r-c}{z}\right) }, \end{aligned}$$

(36)

where $\rho _{0} = 0.172$, $c = 4.094$ and $z = 0.54$ [45].

Appendix B: Nuclear potentials

1.1 Proximity 1977 (Prox 77), Modified Proximity 1988 (Mod-Prox 88), Proximity 1995 (Prox 95), Proximity 2003 (Prox 2003), Proximity 2010 (Prox 2010) potentials

Prox 77 version of proximity potential [46, 47] is formulated as

$$\begin{aligned} V_{N}^{\mathrm {Prox}\, 77}(r)=4\pi \gamma b {\bar{R}} \Phi \left( \zeta =\frac{r-C_{1}-C_{2}}{b}\right) \, \text {MeV}, \end{aligned}$$

(37)

where

$$\begin{aligned} {\bar{R}}= & {} \frac{C_{1}C_{2}}{C_{1}+C_{2}},\nonumber \\ C_{i}= & {} R_{i} \bigg [1-\left( \frac{b}{R_{i}}\right) ^2+\cdots \bigg ], \quad b\approx 1\,\text {fm}. \end{aligned}$$

(38)

$R_{i}$, the effective radius, is given by

$$\begin{aligned} R_{i}=1.28A_{i}^{1/3}-0.76+0.8A_{i}^{-1/3} \text {fm, } \quad i=1, 2. \end{aligned}$$

(39)

$\gamma$, the surface energy coefficient, is given by

$$\begin{aligned} \gamma =\gamma _{0} \bigg [1-k_{s} \left( \frac{N-Z}{N+Z}\right) ^{2}\bigg ]. \end{aligned}$$

(40)

The universal function $\Phi (\zeta )$ is in the following form:

$$\begin{aligned} \Phi (\zeta )\!=\!\left\{ \! \begin{array}{ll} -\frac{1}{2} (\zeta -2.54)^{2}\!-\!0.0852(\zeta \!-\!2.54)^{3}, &{}\quad \! \text {for}\,\ \zeta \!\le \! 1.2511,\\ -3.437 ~\text {exp}\left( -\frac{\zeta }{0.75}\right) , &{}\quad \! \text {for}\,\ \zeta \!\ge \! 1.2511.\\ \end{array} \right. \end{aligned}$$

As a result of many studies on proximity potential, different values of $\gamma _{0}$ and $k_{s}$ have been suggested although the other parameters of the potentials are the same as Prox 77. Each new situation has been evaluated as a different proximity potential. In this respect, seven various potentials are investigated in our study, and the $\gamma _{0}$ and $k_{s}$ values of the potentials are listed in table 4.

Table 4 $\gamma _{0}$ and $k_{s}$ values of Prox 77, Mod-Prox 88, Prox 95, Prox 2003-I, Prox 2003-II, Prox 2003-III and Prox 2010 potentials.

1.2 Broglia and Winther 1991 (BW 91) potential

BW 91 potential [54] is taken as [55]

$$\begin{aligned} V_{N}^{\mathrm {BW}\,91}(r)=-\frac{V_{0}}{\left[ 1 + \text {exp} \left( \frac{r-R_{0}}{a}\right) \right] } \text {MeV}, \end{aligned}$$

(41)

where

$$\begin{aligned} V_{0}=16\pi \frac{R_{1}R_{2}}{R_{1}+R_{2}}\gamma a, \quad a=0.63 \,\text {fm} \end{aligned}$$

(42)

and

$$\begin{aligned} R_{0}= & {} R_{1}+R_{2}+0.29, \nonumber \\ R_{i}= & {} 1.233A_{i}^{1/3}-0.98A_{i}^{-1/3}, \quad i=1,2, \end{aligned}$$

(43)

with $\gamma$ as

$$\begin{aligned} \gamma= & {} \gamma _{0}\bigg [1-k_{s}\left( \frac{N_{p}-Z_{p}}{A_{p}}\right) \left( \frac{N_{t}-Z_{t}}{A_{t}}\right) \bigg ],\nonumber \\&\gamma _{0}=0.95\, \text {MeV/fm}^{2}, \, k_{s}=1.8. \end{aligned}$$

(44)

1.3 Aage Winther (AW 95) potential

AW 95 and BW 91 potentials are the same except for the following values [55, 56]:

$$\begin{aligned} a=\left[ \frac{1}{1.17(1+0.53(A_{1}^{-1/3}+A_{2}^{-1/3}))}\right] \text {fm} \end{aligned}$$

(45)

and

$$\begin{aligned} R_{0}=R_{1}+R_{2}, \quad R_{i}=1.2A_{i}^{1/3}-0.09, \quad i=1,2. \end{aligned}$$

(46)

1.4 Bass 1980 (Bass 80) potential

Bass 80 potential is formulated as [54, 55]

$$\begin{aligned} V_{N}^{\mathrm {Bass}\,80}(s)=-\frac{R_{1}R_{2}}{R_{1}+R_{2}}\phi (s=r-R_{1}-R_{2}) \,\,\, \text {MeV}, \end{aligned}$$

(47)

where

$$\begin{aligned} \phi (s)=\bigg [0.033 \,\ \text {exp}\left( \frac{s}{3.5}\right) +0.007 \,\ \text {exp}\left( \frac{s}{0.65}\right) \bigg ]^{-1} \end{aligned}$$

(48)

and

$$\begin{aligned} R_{i}= & {} R_{s}\left( 1-\frac{0.98}{R_{s}^{2}}\right) , \nonumber \\ R_{s}= & {} 1.28A_{i}^{1/3}-0.76+0.8A_{i}^{-1/3} \, \text {fm,} \quad i=1,2. \end{aligned}$$

(49)

1.5 Christensen and Winther 1976 (CW 76) potential

CW 76 potential [57] is given by [47]

$$\begin{aligned} V_{N}^{\mathrm {CW}\,76}(r)=-50 \frac{R_{1}R_{2}}{R_{1}+R_{2}} \phi (r-R_{1}-R_{2}) \, \text {MeV}, \end{aligned}$$

(50)

where

$$\begin{aligned} \phi (s)=\text {exp}\left( -\frac{r-R_{1}-R_{2}}{0.63}\right) \end{aligned}$$

(51)

and

$$\begin{aligned} R_{i}=1.233A_{i}^{1/3}-0.978A_{i}^{-1/3} \, \text {fm,} \quad i=1,2. \end{aligned}$$

(52)

1.6 Ngô 1980 (Ngo 80) potential

Ngo 80 potential is written as [37]

$$\begin{aligned}&V_{N}^{\mathrm {Ngo}\,80}(r)= {\bar{R}} \phi (r-C_{1}-C_{2}) \, \text {MeV,} \end{aligned}$$

(53)

$$\begin{aligned}&{\bar{R}}=\frac{C_{1}C_{2}}{C_{1}+C_{2}},\quad C_{i}=R_{i} \bigg [1-\left( \frac{b}{R_{i}}\right) ^2+\cdots \bigg ],\end{aligned}$$

(54)

$$\begin{aligned}&R_{i }=\frac{N R_{ni}+Z R_{pi}}{A_{i}}, \quad i=1,2,\end{aligned}$$

(55)

$$\begin{aligned}&R_{pi}=r_{0pi} A_{i}^{1/3}, \quad R_{ni}=r_{0ni} A_{i}^{1/3},\end{aligned}$$

(56)

$$\begin{aligned}&r_{0pi}=1.128 \,\ \text {fm}, \,\ r_{0ni}{=}1.1375+1.875\times 10^{-4}A_{i}\, \text {fm}.\nonumber \\ \end{aligned}$$

(57)

The universal function $\phi (s=r-C_{1}-C_{2})$ (in MeV$/$fm) is taken as

$$\begin{aligned} \Phi (s)=\left\{ \begin{array}{ll} -33+5.4 (s-s_{0})^{2}, &{}\quad \text {for } s < s_{0}, \\ -33 \,\ \text {exp}[-\frac{1}{5}(s-s_{0})^{2}], &{}\quad \text {for } s \ge s_{0}, \\ s_{0}=-1.6\, \text {fm}. \end{array} \right. \end{aligned}$$

1.7 Denisov (D) potential

D potential is formulated by [55, 58]

$$\begin{aligned} V_{N}^{\mathrm {D}}(r)= & {} -1.989843\frac{R_{1} R_{2}}{R_{1}+R_{2}} \phi (r-R_{1}- R_{2}-2.65)\nonumber \\&\times \bigg [1 + 0.003525139\left( \frac{A_{1}}{A_{2}}+ \frac{A_{2}}{A_{1}}\right) ^{3/2}\nonumber \\&-0.4113263(I_{1} + I_{2})\bigg ] \, \text {MeV}, \end{aligned}$$

(58)

where

$$\begin{aligned} I_{i}=\frac{N_{i}-Z_{i}}{A_{i}} \end{aligned}$$

(59)

and

$$\begin{aligned} R_{i}= & {} R_{ip} \left( 1-\frac{3.413817}{R_{ip}^{2}}\right) \nonumber \\&+ 1.284589\left( I_{i}-\frac{0.4A_{i}}{A_{i}+200}\right) , \end{aligned}$$

(60)

$$\begin{aligned} R_{ip}= & {} 1.24 A_{i}^{1/3} \left( 1+\frac{1.646}{A_{i}}-0.191\left( \frac{A_{i}-2Z_{i}}{A_{i}}\right) \right) ,\nonumber \\&\quad i=1,2. \end{aligned}$$

(61)

$\phi (s=r-R_{1}-R_{2}-2.65)$ is evaluated as

$$\begin{aligned}&\phi (s) \\&\;=\left\{ \begin{array}{l} 1-\dfrac{s}{0.7881663}+1.229218s^{2}- 0.2234277s^{3}\\ - 0.1038769s^{4} - \dfrac{R_{1} R_{2}}{R_{1}+R_{2}} (0.1844935s^{2}\\ \\ +0.07570101s^{3}+(I_{1}+I_{2})(0.04470645s^{2}+ \\ \\ 0.0334687s^{3})),\quad -5.65\le s\le 0,\\ \\ \left( 1-s^{2} \left( 0.05410106 \dfrac{R_{1} R_{2}}{R_{1}+R_{2}} \text {exp}\left( -\dfrac{s}{1.76058}\right) \right. \right. \\ \\ \left. \left. -0.539542(I_{1}+I_{2}) \text {exp}\left( - \dfrac{s}{2.424408}\right) \right) \right) \\ \\ \text {exp}\left( -\dfrac{s}{0.7881663}\right) ,\quad s\ge 0. \end{array} \right. \end{aligned}$$