Skip to main content
SpringerLink
Log in

Dynamics of new higher-order rational soliton solutions of the modified Korteweg–de Vries equation

  • Published:
Pramana Aims and scope Submit manuscript

Abstract

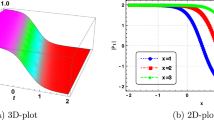
In this paper, we propose a generalised perturbation \((n, N-n)\)-fold Darboux transformation (DT) of the modified Korteweg–de Vries (mKdV) equation using the Taylor expansion and a parameter limit procedure. We apply the generalised perturbation \((1, N-1)\)-fold DT to find the new explicit higher-order rational soliton (RS) solutions in terms of determinants of the mKdV equation. These higher-order RS solutions are different from those known soliton results in terms of hyperbolic functions which are obtained from the classical iterated DT. The dynamics behaviours of the first-, second-, third-, and fourth-order RS solutions are shown graphically. The wave propagation characteristics and stability are also discussed using numerical simulations. We find that the initial constant seed solution plays an important role on the wave propagation stability of RS. Through Miura transformation, we give some complex higher-order rational solutions of the Korteweg–de Vries (KdV) equation which are different from the known results. The relevant structures also are discussed using some figures. The method used can also be extended to seek explicit rational solutions of other nonlinear integrable equations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. C H Gu (Ed.), Soliton theory and its applications (Springer, Berlin, 1995)

    MATH  Google Scholar 

  2. C H Gu, H S Hu and Z X Zhou, Darboux transformations in integrable systems: Theory and their applications to geometry (Springer, Berlin, 2005)

    Book  MATH  Google Scholar 

  3. D J Korteweg and G de Vries, Philos. Mag. 39, 422 (1895)

    Article  MathSciNet  Google Scholar 

  4. D Chudnovsky and G Chudnovsky, Proc. Natl Acad. Sci. USA 96, 12263 (1999)

    Article  ADS  MathSciNet  Google Scholar 

  5. Z D Dai, C J Wang and J Liu, Pramana – J. Phys. 83, 473 (2014)

    Article  ADS  Google Scholar 

  6. C Li, J He and K Porsezian, Phys. Rev. E 87, 012913 (2013)

    Article  ADS  Google Scholar 

  7. N Vishnu Priya and M Senthilvelan, Phys. Scr. 90, 025203 (2015)

    Article  ADS  Google Scholar 

  8. N Vishnu Priya and M Senthilvelan, Commun. Nonlinear Sci. Numer. Simulat. 20, 401 (2015)

    Article  ADS  MathSciNet  Google Scholar 

  9. Z Horii, Phys. Lett. A 306, 45 (2002)

    Article  ADS  MathSciNet  Google Scholar 

  10. L P Zhang and J K Xue, Chaos Solitons Fractals 23, 543 (2005)

    Article  ADS  Google Scholar 

  11. J Li, T Xu, X H Meng, Z C Yang, H W Zhu and B Tian, Phys. Scr. 75, 278 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  12. X Y Wen, Y T Gao and L Wang, Chaos Appl. Math. Comput. 218, 55 (2011)

    Article  Google Scholar 

  13. A M Wazwaz, Appl. Math. Comput. 182, 283 (2006)

    MathSciNet  Google Scholar 

  14. D H Peregrine, J. Austral. Math. Soc. Ser. B (Appl. Math.) 25, 16 (1983).

    Article  Google Scholar 

  15. A Chowdurya, A Ankiewicz and N Akhmediev, Eur. Phys. J. D 70, 104 (2016)

    Article  ADS  Google Scholar 

  16. X Y Wen, Y Q Yang and Z Y Yan, Phys. Rev. E 92, 012917 (2015)

    Article  ADS  MathSciNet  Google Scholar 

  17. X Y Wen and Z Y Yan, Chaos 25 123115 (2015)

    Article  ADS  MathSciNet  Google Scholar 

  18. X Y Wen and Z Y Yan, Commun. Nonlinear Sci. Numer. Simulat. 43, 311 (2017)

    Article  ADS  MathSciNet  Google Scholar 

  19. B L Guo, L L Ling and Q P Liu, Phys. Rev. E 85, 026607 (2012)

    Article  ADS  Google Scholar 

  20. Q F Xuan and D J Zhang, Commun. Theor. Phys. (Beijing, China) 50, 13 (2008)

    Article  ADS  Google Scholar 

  21. Y Y Sun and D J Zhang, Commun. Theor. Phys. (Beijing, China) 57, 923 (2012)

    Article  ADS  Google Scholar 

  22. R M Miura, J. Math. Phys. 9, 1202 (1968)

    Article  ADS  Google Scholar 

  23. V B Matveev and M A Salle, Darboux transformation and solitons (Springer-Verlag, Berlin, 1991)

    Book  MATH  Google Scholar 

  24. W Chen, H L Chen and Z D Dai, Pramana – J. Phys. 86, 713 (2016)

    Article  ADS  Google Scholar 

  25. H Gao, Pramana – J. Phys. 88, 84 (2017)

    Article  ADS  Google Scholar 

  26. Y K Liu and B Li, Pramana – J. Phys. 88, 57 (2017)

    Article  ADS  Google Scholar 

  27. E G Fan, J. Math. Phys. 42, 4327 (2001)

    Article  ADS  MathSciNet  Google Scholar 

  28. E G Fan, J. Math. Phys. 41, 7769 (2000)

    Article  ADS  MathSciNet  Google Scholar 

  29. E G Fan, Commun. Theor. Phys. (Beijing, China) 35, 651 (2001)

    Article  ADS  Google Scholar 

  30. A Ankiewicz and N Akhmediev, Nonlinear Dyn. (2017). https://doi.org/10.1007/s11071-017-3991-2

  31. L N Trefethen, Spectral methods in MATLAB (SIAM, Philadelphia, 2000)

    Book  MATH  Google Scholar 

  32. J Yang, Nonlinear waves in integrable and nonintegrable systems (SIAM, Philadelphia, 2010)

    Book  MATH  Google Scholar 

Download references

Acknowledgements

This work has been partially supported by Qin Xin Talents Cultivation Program of Beijing Information Science and Technology University (QXTCP-B201704 and QXTCP-A201702), the NSFC under Grant Nos 11375030 and 61178091, the Beijing Natural Science Foundation under Grant No. 1153004 and China Postdoctoral Science Foundation under Grant No. 2015M570161.

Author information

Authors and Affiliations

  1. School of Applied Science, Beijing Information Science and Technology University, Beijing , 100192, China

    Xiao-Yong Wen

  2. Key Laboratory of Mathematics Mechanization, Institute of Systems Science, AMSS, Chinese Academy of Sciences, Beijing , 100190, China

    Yong Chen

  3. School of Mathematical Sciences, University of Chinese Academy of Sciences, Beijing , 100049, China

    Yong Chen

Authors
  1. Xiao-Yong Wen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yong Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xiao-Yong Wen.

Appendix A

Appendix A

$$\begin{aligned} \phi ^{(1)}= & {} (1/6) \sqrt{2/c} (-114 c^2 t+12 c b_1\\&+\,12 i cd_1+3 x+4 c^2 x^3-72 c^4 x^2 t\\&+\,432 c^6 x t^2-864 c^8 t^3),\\ \psi ^{(1)}= & {} -(1/6) \sqrt{2} (15 c x-186 c^3 t+12 c^2 x^2\\&-\,144 c^4 x t+432 c^6 t^2+12 c^2 b_1\\&+12 i c^2 d_1+4 c^3 x^3-72 c^5 x^2 t+432 c^7 x t^2\\&-\,864 c^9 t^3+3)/c^{3/2},\\ \phi ^{(2)}= & {} (1/240) \sqrt{2} (-15 x-2790 c^2 t+120 c^2 x^3\\&+120 c b_1-164160 c^8 t^3-6000 c^4 x^2 t\\&+\,59040 c^6 x t^2+480 c^2 b_2+16 c^4 x^5\\&-124416 c^{14} t^5+120 i c d_1+480 i c^2 d_2\\&-34560 c^{10} x^2 t^3+480 c^3 x^2 b_1+17280 c^7 t^2 b_1\\&-480 c^6 x^4 t+103680 c^{12} x t^4+5760 c^8 x^3 t^2\\&+480 i c^3 x^2 d_1-5760 i c^5 x t d_1-5760 c^5 x t b_1\\&+\,17280 i c^7t^2 d_1)/c^{3/2}, \\ \psi ^{(2)}= & {} -(1/240)\sqrt{2} (-15+80 c^4 x^4+360 c^2 x^2\\&+103680 c^{12} t^4-69120 c^{10} x t^3+17280 c^8 x^2 t^2\\&-1920 c^6 x^3 t+960 c^3 b_1 x-5760 c^5 t b_1\\&-12000 c^4 x t+59040 c^6t^2-5760 i c^5 d_1 t\\&+\,480 i c^3 d_2-5760 i c^6 x t d_1+105 c x+600 c^2 b_1\\&-\,7350 c^3 t+280 c^3 x^3-198720 c^9 t^3+480 c^3 b_2\\&+\,16 c^5 x^5-124416 c^{15} t^5-8880 c^5 x^2t\\&+\,76320 c^7 x t^2-34560 c^{11} x^2 t^3+480 c^4 x^2 b_1\\&+\,17280 c^8 t^2 b_1-480 c^7 x^4 t+103680 c^{13} x t^4\\&+\,5760 c^9 x^3 t^2-5760 c^6 x t b_1+960 i c^3 d_1 x\\&+\,600 i c^2 d_1+17280 i c^8 t^2 d_1\\&+480 i c^4 x^2 d_1)/c^{5/2},\\ \phi ^{(3)}= & {} (1/20160) \sqrt{2} (315 x+10080 i c^2 d_2\\&-\,17915904 c^{20} t^7-32130 c^2 t+1260 c^2 x^3\\&-1260 c b_1-30270240 c^8 t^3-425880 c^4 x^2 t\\&+7554960 c^6 x t^2+1451520 i c^8t^2 d_2\\&+10080 c^2 b_2+1680 c^4 x^5-82736640 c^{14} t^5\\&-15240960 c^{10} x^2 t^3+30240 c^3 x^2 b_1\\&+4959360 c^7 t^2 b_1-104160 c^6 x^4 t\\&+57335040 c^{12} x t^4+1895040 c^8 x^3 t^2\\&- 1008000 c^5 x t b_1+64 c^6 x^7+40320 c^3 b_3\\&+\,40320 c^4 x^2 b_2+1451520 c^8 t^2 b_2+40320 c^4 b_1^2 x\\&+6720 c^5 x^4 b_1-\,241920 c^6 b_1^2 t\\&+8709120 c^{13} t^4 b_1-483840 c^{12} x^4t^3\\&+48384 c^{10} x^5 t^2-40320 c^4 d_1^2 x\\&+\,241920 c^6 d_1^2 t-2688 c^8 x^6 t+20901888 c^{18} t^6 x\\&-\,10450944 c^{16} t^5 x^2+2903040 c^{14} x^3 t^4\\&-\,483840 c^6 x t b_2-161280 c^7x^3 t b_1\\&+\,1451520 c^9 x^2 t^2 b_1\\&-\,5806080 c^{11} x t^3 b_1+30240 i c^3 x^2 d_1\\&-\,483840 i c^6 b_1 t d_1+4959360 i c^7 t^2 d_1\\&-\,5806080 i c^{11} x t^3 d_1+40320 i c^3d_3\\&+\,80640 i c^4 b_1 x d_1+8709120 i c^{13} t^4 d_1\\&+\,40320 i c^4 x^2 d_2-483840 i c^6 x t d_2\\&-\,1008000 i c^5 x t d_1-161280 i c^7 x^3 t d_1\\&+\,1451520 i c^9 x^2 t^2 d_1-1260 i c d_1\\&+\,6720 i c^5 x^4 d_1)/c^{5/2},\\ \psi ^{(3)}= & {} -(1/20160) \sqrt{2} (315+6720 i c^6 x^4 d_1\\&-\,17915904 c^{21} t^7+8709120 i c^{14} t^4 d_1+64 c^7 x^7\\&+\,448 c^6 x^6+80640 i c^4 x d_2-483840 i c^7 x^2 t d_1\\&-\,1491840 i c^6 x t d_1+8400 c^4 x^4+3780 c^2 x^2\\&+\,57335040 c^{12} t^4-30481920 c^{10} x t^3\\&+\,5685120 c^8 x^2 t^2-416640 c^6 x^3 t+60480 c^3 b_1 x\\&-\,1008000 c^5 t b_1-851760 c^4 xt+7554960 c^6 t^2\\&-\,483840 c^7 x t b_2-161280 c^8 x^3 t b_1\\&+\,1451520 c^{10} x^2 t^2 b_1-5806080 c^{12} x t^3 b_1\\&-\,483840 i c^7 b_1 t d_1+40320 c^4 b_3+60480 i c^3 d_1 x\\&+\,8820 ic^2 d_1-483840 c^6 t b_2+40320 c^5 x^2 b_2\\&+\,1451520 c^9 t^2 b_2+40320 c^5 b_1^2 x+6720 c^6 x^4 b_1\\&-241920 c^7 b_1^2 t+8709120 c^{14} t^4 b_1\\&-\,483840 c^{13} x^4 t^3+48384 c^{11}x^5 t^2\\&-\,40320 c^5 d_1^2 x+241920 c^7 d_1^2 t-2688 c^9 x^6 t\\&+\,20901888 c^{19} t^6 x-10450944 c^{17} t^5 x^2\\&+\,2903040 c^{15} x^3 t^4+241920 c^{10} x^4 t^2\\&+\,80640 c^4 x b_2+40320 ic^4 d_3+80640 i c^5 b_1 x d_1\\&+\,50400 i c^3 d_2+1451520 i c^{10} x^2 t^2 d_1\\&-40320 c^4 d_1^2+2903040 i c^9 x t^2 d_1-945 c x\\&+8820 c^2 b_1-266490 c^3 t\\&+11340 c^3 x^3-44059680 c^9 t^3\\&+\,50400 c^3 b_2+3024 c^5 x^5-93187584 c^{15} t^5\\&-\,929880 c^5 x^2 t+12514320 c^7 x t^2\\&-\,18144000 c^{11} x^2 t^3+70560 c^4 x^2 b_1\\&+\,6410880 c^8 t^2 b_1-144480 c^7 x^4t\\&+\,66044160 c^{13} x t^4+2378880 c^9 x^3 t^2\\&-\,1491840 c^6 x t b_1+20901888 c^{18} t^6\\&+40320 c^4 b_1^2-483840 c^7 x^2 t b_1\\&+2903040 c^9 x t^2 b_1-16128 c^8 x^5 t\\&-\,20901888 c^{16}t^5 x-1935360 c^{12} x^3 t^3\\&+\,8709120 c^{14} x^2 t^4+26880 c^5 x^3 b_1\\&-\,5806080 c^{11} t^3 b_1-1008000 i c^5 d_1 t\\&-\,5806080 i c^{12} x t^3 d_1-483840 i c^6 t d_2\\&+\,80640 ic^4 b_1 d_1+1451520 i c^9 t^2 d_2\\&-\,5806080 i c^{11} t^3 d_1+40320 i c^5 x^2 d_2\\&+\,70560 i c^4 x^2 d_1-483840 i c^7 x t d_2\\&+\,6410880 i c^8 t^2 d_1-161280 i c^8 x^3 td_1\\&+\,26880 i c^5 x^3 d_1)/c^{7/2}. \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wen, XY., Chen, Y. Dynamics of new higher-order rational soliton solutions of the modified Korteweg–de Vries equation. Pramana - J Phys 91, 23 (2018). https://doi.org/10.1007/s12043-018-1592-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12043-018-1592-3

Keywords

PACS Nos

Search

Navigation