Density matrix renormalization group (DMRG) for interacting spin chains and ladders

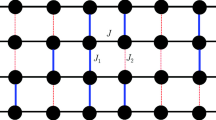
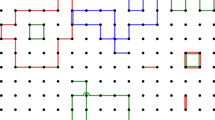
Abstract

The density matrix renormalization group (DMRG) method generates the low-energy states of linear systems of N sites with a few degrees of freedom at each site by starting with a small system and adding sites step by step while keeping the dimension of the truncated Hilbert space constant. DMRG algorithms are adapted to open chains with inversion symmetry at the central site, to cyclic chains, to weakly coupled chains, and to low-T thermodynamics. The motivation is physical properties rather than energy accuracy. The algorithms are applied to the edge states of linear Heisenberg antiferromagnets with spin $S \ge 1/2$, the quantum phases of a frustrated spin-1/2 chain with an exchange between first and second neighbors, a spin-1/2 ladder with skewed rungs, and the spin-Peierls transitions of an organic and an inorganic crystal.

Graphical abstract

The density matrix renormalization group is a numerical technique for studying the ground state properties of low-dimensional quantum many body systems. Its application to the quantum spin chain and ladder systems is reviewed.

Appendix

1.1 Implementation of the conventional DMRG algorithm

In order to understand the implementation of the DMRG method, consider an antiferromagnetic Heisenberg spin chain with Hamiltonian

$$\begin{aligned} H = \sum _{<ij>} J_{ij} (S_{i}^zS_{j}^z+\frac{1}{2}(S_{i}^+S_{j}^-+S_{i}^-S_{j}^+)) \end{aligned}$$

where $J_{ij}$ is the exchange interaction, $S_{i}^+$ and $S_{j}^-$ are the raising and lowering operators at sites i and j, respectively. For this system the DMRG method can be discussed in two parts (a) an infinite DMRG algorithm and (b) finite DMRG algorithm.

1.2 A. Infinite DMRG algorithm

• 1. First, we consider a four-site spin chain (superblock) for which the Hamiltonian can be diagonalized using the exact diagonalization method. This superblock is divided into two parts, with two sites forming a left block and the other two sites forming a right block. The Hamiltonian matrix for this superblock is constructed by using the constant $S^{z}$ basis (Figure 1).

• 2. The ground state of the superblock $|\psi _{G_4}\rangle$ is obtained by diagonalizing the Hamiltonian matrix and it is written as a linear combination of the direct product of the Fock space of the left and right blocks [{${L_{2}}$},{${R_{2}}$}] each with two sites.

  $$\begin{aligned} |\psi _{G_4}\rangle = \sum _{L_{2}} \sum _{R_{2}} C_{L_{2}R_{2}} |L_{2}\rangle |R_{2}\rangle . \end{aligned}$$

• 3. By tracing out the states of the right block, the reduced density matrix of the left block is created, and its matrix elements are given by

  $$\begin{aligned} {[}\rho _{2}]_{L_{2} L'_{2}} = \sum _{R_{2}} C_{L_{2}R_{2}} C_{L_{2}' R_{2}}. \end{aligned}$$

• 4. All the eigenvalues and the corresponding eigenvectors of the reduced density matrix are obtained by diagonalizing $\rho _{_2}$. Only ‘m’ eigenvectors of the reduced density matrix corresponding to the highest eigenvalues are stored as columns of a matrix ${\hat{O}}_{_2}$. If the Fock space of the left block is less than ‘m’ at this point, then all the density matrix eigenvectors are kept.

• 5. The Hamiltonian matrix of two sites ${\hat{H}}_{_{2}}$ is built in Fock space basis {${L_{_2}}$} and this matrix is transformed to a density matrix eigenvectors basis by a similarity transformation ${\tilde{H}} = {\hat{O}}_{_2}^+{\hat{H}}_{_2} {\hat{O}}_{_2}$.

• 6. The spin operators such as ${\hat{S}}_{_i}^z$, ${\hat{S}}_{_i}^+$, ${\hat{S}}_i^-$ at any site i in the left block of the chain are expressed as matrices in the Fock space basis of the left block and then transformed them into DMEV basis i.e. ${\tilde{S}}_i^z = {\hat{O}}_{_2}^+{\hat{S}}_i^z {\hat{O}}_{_2}$, ${\tilde{S}}_i^+ = {\hat{O}}_{_2}^+{\hat{S}}_i^+ {\hat{O}}_{_2}$ and ${\tilde{S}}_i^- = {\hat{O}}_{_2}^+{\hat{S}}_i^- {\hat{O}}_{_2}$. If the superblock has an inversion symmetry at the centre of the full system then the site operators at the right block are same as those of the corresponding sites on the left block. Otherwise we must go through steps 4 to 8 for the right block.

• 7. The system size is augmented to six by adding two sites in the middle of the superblock, where the augmented system is made up of the left block, newly added site to the left block, right block and the newly added site to the right block. The Hamiltonian corresponding to this augmented system is given by

  $$\begin{aligned} {\hat{H}}_{_6} = {\tilde{H}}_{_2} + {\tilde{H}}'_{_2} + {\tilde{S}}_{_2} \cdot {\hat{S}}_{_3} + {\hat{S}}_{_3} \cdot {\hat{S}}'_{_3} + {\hat{S}}'_3 \cdot {\tilde{S}}'_{_2}, \end{aligned}$$

• 8. The Hamiltonian matrix ${\hat{H}}_{_6}$ for the 6 site system is built in a direct product basis set $|\mu \sigma \sigma ' \mu '\rangle$, where $|\mu \rangle$ and $|\mu '\rangle$ are the density matrix eigenvectors that serve as the basis for the left and right blocks, respectively, and $|\sigma \rangle$ and $|\sigma '\rangle$ are the Fock space basis of the newly added sites 3 and $3'$. The Hamiltonian matrix corresponding to this 6 site system is

  $$\begin{aligned} \langle \mu \sigma \sigma '\mu '|{\hat{H}}_{_6}|\nu \tau \tau '\nu '\rangle= & {} \langle \mu _{_{2}}|{\tilde{H}}_{_2}^{L}|\nu _{_{2}}\rangle \delta _{\sigma \tau }\delta _{\sigma '\tau '}\delta _{\mu _{_{2}}'\nu _{_{2}}'} + \langle \mu _{_{2}}'|{\tilde{H}}_{_2}^{R}|\nu _{_{2}}'\rangle \delta _{\sigma \tau }\delta _{\sigma '\tau '}\delta _{{\mu _{_{2}} \nu _{_{2}}}} \\ {}{} & {} + \langle \sigma |{\hat{S}}_{_{3}}|\tau \rangle \cdot \langle \sigma '|{\hat{S}}_{_{3}}'|\tau '\rangle \delta _{\mu _{_{2}}'\nu _{_{2}}'} \delta _{\mu _{_{2}}\nu _{_{2}}} + \langle \mu _{_{2}}|{\tilde{S}}_{_{2}}|\nu _{_{2}}\rangle \cdot \langle \sigma |{\hat{S}}_{_{3}}|\tau \rangle \delta _{\mu _{_{2}}'\nu _{_{2}}'} \delta _{\sigma '\tau '} \\ {}{} & {} + \langle \mu _{_{2}}'|{\tilde{S}}_{_{2}}'|\nu _{_{2}}'\rangle \cdot \langle \sigma '|{\hat{S}}_{_{3}}'|\tau '\rangle \delta _{\mu _{_{2}}\nu _{_{2}}}\delta _{\sigma \tau } . \end{aligned}$$

• 9. The ground state $|\psi _{G_{6}}\rangle$ is obtained by diagonalizing the ${\hat{H}}_{6}$ matrix and the density matrix for new left block $\rho _{3}^{L}$ can be constructed as

  $$\begin{aligned} \langle \mu \sigma |\rho _{_{3}}^L|\nu \tau \rangle = \sum _{\mu _{_{2}}'\sigma '} C_{\mu _{_{2}}\sigma \sigma '\mu _{_{2}}'}C_{\nu _{_{2}}\tau \sigma '\mu _{_{2}}'}. \end{aligned}$$

• 10. By diagonalizing the density matrix $\rho _{_3}^{L}$, ‘m’ DMEVs {${\mu _{_{3}}}$} corresponding to the largest eigenvalues are obtained and stored as columns of a matrix ${\hat{O}}_{_3}$. The left block Hamiltonian $H_{3}^L$ and the spin operators of the left block are renormalized to the DMEV basis, as described in steps 5 and 6.

• 11. We repeat the steps from 7 to 9 to get the density matrix for 4 sites left block ($\rho _{_4}^{L}$) and whole process is repeated till the desired system size N is reached.

1.3 B. Finite DMRG algorithm

A well-known iterative algorithm called the finite DMRG method can increase the accuracy of the infinite DMRG method. The primary flaw in the infinite DMRG method is that the density matrices we create at each iteration of the calculation are not the density matrices of the final size system, but rather those of systems with intermediate sizes. Thus we can increase the accuracy of the eigenstates of the model Hamiltonian by building density matrices of the full system size. In the finite DMRG method, the system size remains constant, while the size of left and right blocks vary at each step of the calculation. The finite DMRG procedure begins when the infinite DMRG procedure reaches a desired system size of N sites, with the left and right blocks each having $(N/2-1)$ sites and the middle of the system has two bare sites as shown in the first step of Figure 17. The initial density matrices calculated from the infinite DMRG procedure are $\rho _{k}^{(0)\,L}$, $\rho _{k}^{(0)\,R}$ $k = 2, 3, 4 ..., (N/2-1)$, where (0) stands for the iteration number of the finite DMRG method. All of the density matrices and the site matrices are stored during the finite system iteration.

Figure 17

Schematic representation of the finite DMRG algorithm open circles indicate the bare sites. Black rectangular boxes represent the system size with N sites whereas the blue boxes represent the left and right blocks.

The procedure for the finite DMRG calculations is as follows:

• 1. Using the $|\psi _{_{G_{N}}}\rangle$ in the basis $|\mu _{_{N/2-1}}\sigma \sigma '\mu _{_{N/2-1}}'\rangle$ the density matrix $\rho _{_{N/2}}$ is constructed and $H_{_{N/2}}$ and all site operators for N/2 site are obtained in the DMEV basis of N/2 sites.

• 2. The Hamiltonian matrix of the N site system is now obtained in the basis $|\mu _{_{N/2}}\sigma \sigma '\mu _{_{N/2-2}}'\rangle$. The ground state of the N site system is obtained in direct product basis of the DMEV of $\rho _{_{N/2}}^{(0)\,L}$, $\rho _{_{N/2-2}}^{(0)\,R}$, and Fock space basis of the two bare sites using the density matrix $\rho _{_{N/2}}^{(0)\,L}$ and $\rho _{_{N/2-2}}^{(0)\,R}$ (Figure 17).

• 3. Using the ground state of the N site system, we construct the density matrix $\rho _{_{N/2+1}}^{(0)\,L}$ for the $(N/2+1)$ sites left block.

• 4. We can as before obtain the ground state of the N site system in the direct product basis of DMEVs of the new density matrix of the left block $\rho _{_{N/2+1}}^{(0)\,L}$, old density matrix of right block $\rho _{_{N/2-3}}^{(0)\,R}$ and Fock space basis of the two bare sites.

• 5. This process is repeated until the right block has a single site and the ground state of N site system is formed by the direct product basis of the DMEVs of $\rho _{_{N/2-3}}^{(0)\,L}$, Fock space basis of two bare sites and one site in the right block.

• 6. Using the ground state of the Hamiltonian in the basis $|\mu _{_{N/2-3}}^{(0)\,L}\sigma \sigma '\sigma ''\rangle$ construct the density matrix $\rho _{_{2}}^{(1)\,R}$ for the two site right block. From this obtain the ground state in the basis $|\mu _{_{N/2-4}}\sigma \sigma '\mu _{_{2}}'\rangle$ and proceed similarly to increase the block size of the right block by one more site and reducing the left block size by one less site.

• 7. In a full finite iteration, all of the density matrices in the left and right blocks are updated with the new density matrices. This means that first we sweep to the right end, then sweep to the left end, and finally sweep to the right once more, until the block on the left and right are the same size. The ground state energy and wavefunction obtained at this stage corresponds to results at the end of one finite iteration.

• 8. The entire finite iteration is repeated until desired convergence in the eigenstate are attained.