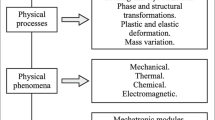
Appendix
For the sake of completeness, we state the explicit form of (6) using the error function. After using the definitions of \(q_i\) and G and integration of the spatial integrals, we get
$$\begin{aligned} \begin{aligned} u(x,t) =&\frac{3\sqrt{3}}{4\pi \sqrt{\pi }c\rho } \int _0^t Q(\tau ) \\&\times \left( f_r I_{1r}(x_1,t,\tau ) + f_f I_{1f}(x_1,t,\tau )\right) \\&\times I_2(x_2,t,\tau ) I_3(x_3,t,\tau ) d\tau , \end{aligned} \end{aligned}$$
(7)
where the functions \(I_i(x_i,\xi _i,t,\tau )\) are defined as follows.
$$\begin{aligned}&I_{1r}(x_1,t,\tau ) = \frac{1}{\sqrt{12 \alpha (t-\tau ) + \delta _{1r}(\tau )^2}} \\&\quad \times \sum _{n=0}^\infty \left\{ \exp \left[ -\frac{3(x_1-v\tau +2nL)^2}{\delta _{1r}(\tau )^2+12\alpha (t-\tau )}\right] \right. \\&\quad \times \left( {\text {erf}}\left[ \frac{12v\tau \alpha (t-\tau )+\delta _{1r}(\tau )^2(x_1+2nL)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right. \\&\qquad \left. + {\text {erf}}\left[ \frac{\delta _{1r}(\tau )^2(-x_1+v\tau -2nL)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_1+v\tau +2nL)^2}{\delta _{1r}(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12v\tau \alpha (t-\tau )+\delta _{1r}(\tau )^2(-x_1-2nL)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right. \\&\qquad \left. +{\text {erf}}\left[ \frac{\delta _{1r}(\tau )^2(x_1+v\tau +2nL)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_1+v\tau -2(n+1)L)^2}{\delta _{1r}(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12v\tau \alpha (t-\tau )+\delta _{1r}(\tau )^2(-x_1+2(n+1)L)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right. \\&\qquad \left. +{\text {erf}}\left[ \frac{\delta _{1r}(\tau )^2(x_1+v\tau -2(n+1)L)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_1-v\tau -2(n+1)L)^2}{\delta _{1r}(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12v\tau \alpha (t-\tau )+\delta _{1r}(\tau )^2(x_1-2(n+1)L)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right. \\&\qquad \left. +\left. {\text {erf}}\left[ \frac{\delta _{1r}(\tau )^2(-x_1+v\tau +2(n+1)L)}{2\delta _{1r}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1r}(\tau )^2}}\right] \right) \right\} \\&I_{1f}(x_1,t,\tau ) = \frac{1}{\sqrt{12 \alpha (t-\tau ) + \delta _{1f}(\tau )^2}} \\&\quad \times \sum _{n=0}^\infty \left\{ \exp \left[ -\frac{3(x_1-v\tau +2nL)^2}{\delta _{1f}(\tau )^2+12\alpha (t-\tau )}\right] \right. \\&\quad \times \left( {\text {erf}}\left[ \frac{12(L-v\tau )\alpha (t-\tau )+\delta _{1f}(\tau )^2(-x_1-(2n-1)L)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right. \\&\qquad \left. + {\text {erf}}\left[ \frac{\delta _{1f}(\tau )^2(x_1-v\tau +2nL)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_1+v\tau +2nL)^2}{\delta _{1f}(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12(L-v\tau )\alpha (t-\tau )+\delta _{1f}(\tau )^2(x_1+(2n+1)L)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right. \\&\qquad \left. +{\text {erf}}\left[ \frac{\delta _{1f}(\tau )^2(-x_1-v\tau -2nL)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_1+v\tau -2(n+1)L)^2}{\delta _{1f}(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12(L-v\tau )\alpha (t-\tau )+\delta _{1f}(\tau )^2(x_1-(2n+1)L)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right. \\&\qquad \left. +{\text {erf}}\left[ \frac{\delta _{1f}(\tau )^2(-x_1-v\tau +2(n+1)L)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_1-v\tau -2(n+1)L)^2}{\delta _{1f}(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12(L-v\tau )\alpha (t-\tau )+\delta _{1f}(\tau )^2(-x_1+(2n+3)L)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right. \\&\qquad \left. +\left. {\text {erf}}\left[ \frac{\delta _{1f}(\tau )^2(x_1-v\tau -2(n+1)L)}{2\delta _{1f}(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _{1f}(\tau )^2}}\right] \right) \right\} \\ \end{aligned}$$
$$\begin{aligned}&I_2(x_2,t,\tau ) = \frac{1}{\sqrt{12 \alpha (t-\tau ) + \delta _2(\tau )^2}} \\&\quad \times \sum _{n=0}^\infty \left\{ \exp \left[ -\frac{3(x_2+2nB-B_1)^2}{\delta _2(\tau )^2+12\alpha (t-\tau )}\right] \right. \\&\quad \times \left( {\text {erf}}\left[ \frac{12B_1\alpha (t-\tau )+\delta _2(\tau )^2(x_2+2nB)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right. \\&\qquad \left. + {\text {erf}}\left[ \frac{12B_2\alpha (t-\tau )+\delta _2(\tau )^2(-x_2-(2n-1)B)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_2+2nB+B_1)^2}{\delta _2(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12B_1\alpha (t-\tau )+\delta _2(\tau )^2(-x_2-2nB)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right. \\&\qquad \left. +{\text {erf}}\left[ \frac{12B_2\alpha (t-\tau )+\delta _2(\tau )^2(x_2+(2n+1)B)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_2-2(n+1)B+B_1)^2}{\delta _2(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12B_1\alpha (t-\tau )+\delta _2(\tau )^2(-x_2+2(n+1)B)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right. \\&\qquad \left. +{\text {erf}}\left[ \frac{12B_2\alpha (t-\tau )+\delta _2(\tau )^2(x_2-(2n+1)B)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_2-2(n+1)B-B_1)^2}{\delta _2(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12B_1\alpha (t-\tau )+\delta _2(\tau )^2(x_2-2(n+1)B)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right. \\&\qquad \left. +\left. {\text {erf}}\left[ \frac{12B_2\alpha (t-\tau )+\delta _2(\tau )^2(-x_2+(2n+3)B)}{2\delta _2(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _2(\tau )^2}}\right] \right) \right\} \end{aligned}$$
$$\begin{aligned}&I_3(x_3,t,\tau ) = \frac{1}{\sqrt{12 \alpha (t-\tau ) + \delta _3(\tau )^2}}\\&\quad \times \sum _{n=0}^\infty \left\{ \exp \left[ -\frac{3(x_3-2(n+1)H)^2}{\delta _3(\tau )^2+12\alpha (t-\tau )}\right] \right. \\&\quad \times \left( {\text {erf}}\left[ \frac{12H\alpha (t-\tau )+\delta _3(\tau )^2(x_3-(2n+1)H)}{2\delta _3(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _3(\tau )^2}}\right] \right. \\&\qquad \left. + {\text {erf}}\left[ \frac{12H\alpha (t-\tau )+\delta _3(\tau )^2(-x_3+(2n+3)H)}{2\delta _3(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _3(\tau )^2}}\right] \right) \\&\qquad + \exp \left[ -\frac{3(x_3+2nH)^2}{\delta _3(\tau )^2+12\alpha (t-\tau )}\right] \\&\quad \times \left( {\text {erf}}\left[ \frac{12H\alpha (t-\tau )+\delta _3(\tau )^2(-x_3-(2n-1)H)}{2\delta _3(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _3(\tau )^2}}\right] \right. \\&\qquad \left. +\left. {\text {erf}}\left[ \frac{12H\alpha (t-\tau )+\delta _3(\tau )^2(x_3+(2n+1)H)}{2\delta _3(\tau )\sqrt{\alpha (t-\tau )(12\alpha (t-\tau )+\delta _3(\tau )^2}}\right] \right) \right\} \end{aligned}$$