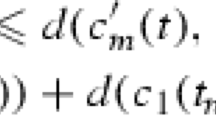Skip to main content
SpringerLink
Log in

Ergodic decompositions of geometric measures on Anosov homogeneous spaces

  • Published:
Israel Journal of Mathematics Aims and scope Submit manuscript

Abstract

Let G be a connected semisimple real algebraic group and Γ a Zariski dense Anosov subgroup of G with respect to a minimal parabolic subgroup P. Let N be the maximal horospherical subgroup of G given by the unipotent radical of P. We describe the N-ergodic decompositions of all Burger–Roblin measures as well as the A-ergodic decompositions of all Bowen–Margulis–Sullivan measures on ΓG. As a consequence, we obtain the following refinement of the main result of [17]: the space of all non-trivial N-invariant ergodic and P°-quasi-invariant Radon measures on ΓG, up to constant multiples, is homeomorphic to ℝrank G−1 × {1, …, k} where k is the number of P°-minimal subsets in ΓG.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Y. Benoist, Propriétés asymptotiques des groupes lineaires, Geometric and Functional Analysis 7 (1997), 1–47.

    Article  MathSciNet  MATH  Google Scholar 

  2. Y. Benoist, Groupes linéaires á valeurs propres positives et automorphismes des cones convexes, Comptes Rendus de l’Académie des Sciences. Série I. Mathématique 325 (1997), 471–474.

    MathSciNet  MATH  Google Scholar 

  3. Y. Benoist and J.-F. Quint, Random walks on projective spaces, Compositio Mathematica 150 (2014), 1579–1606.

    Article  MathSciNet  MATH  Google Scholar 

  4. M. Burger, Horocycle flow on geometrically finite surfaces, Duke Mathematical Journal 61 (1990), 779–803.

    Article  MathSciNet  MATH  Google Scholar 

  5. S. Choi and W. Goldman, Convex real projective structures on closed surfaces are closed, Proceedings of the American Mathematical Society 118 (1993), 657–661.

    Article  MathSciNet  MATH  Google Scholar 

  6. L. Carvajales, Growth of quadratic forms under Anosov subgroups, International Mathematics Research Notices 2023 (2023), 785–854.

    Article  MathSciNet  MATH  Google Scholar 

  7. N.-T. Dang, Topological mixing of positive diagonal flows, Israel Journal of Mathematics, to appear, https://arxiv.org/abs/2011.12900.

  8. S. G. Dani, Invariant measures and minimal sets of horospherical flows, Inventiones Mathematicae 64 (1981), 357–385.

    Article  MathSciNet  MATH  Google Scholar 

  9. S. Edwards, M. Lee and H. Oh, Anosov groups:local mixing, counting and equidistribution, Geometry & Topology 27 (2023), 513–573.

    Article  MathSciNet  MATH  Google Scholar 

  10. H. Furstenberg, The unique ergodicity of the horocycle flow, iin Recent Advances in Topological Dynamics, Lecture Notes in Mathematics, Vol. 318, Springer, Berlin–New York, 1973, pp. 95–115.

    Chapter  Google Scholar 

  11. G. Greschonig and K. Schmidt, Ergodic decomposition of quasi-invariant probability measures, Colloquium Mathematicum 84/85 (2000), 495–514.

    Article  MathSciNet  MATH  Google Scholar 

  12. O. Guichard and A. Wienhard, Anosov representations: Domains of discontinuity and applications, Inventiones Mathematicae 190 (2012), 357–438.

    Article  MathSciNet  MATH  Google Scholar 

  13. Y. Guivarch and A. Raugi, Actions of large semigroups and random walks on isometric extensions of boundaries, Annales Scientifiques de l’École Normale Supérieure. 40 (2007), 209–249.

    Article  MathSciNet  MATH  Google Scholar 

  14. M. Kapovich, B. Leeb and J. Porti, Anosov subgroups: dynamical and geometric characterizations, European Journal of Mathematics 3 (2017), 808–898.

    Article  MathSciNet  MATH  Google Scholar 

  15. F. Labourie, Anosov flows, surface groups and curves in projective space, Inventiones Mathematicae 165 (2006), 51–114.

    Article  MathSciNet  MATH  Google Scholar 

  16. O. Landesberg, M. Lee, and E. Lindenstrauss and H. Oh, Horospherical invariant measures and a rank dichotomy for Anosov groups, Journal of Modern Dynamics 19 (2023), 331–362.

    Article  MathSciNet  MATH  Google Scholar 

  17. M. Lee and H. Oh, Invariant measures for horospherical actions and Anosov groups, International Mathematics Research Notices https://doi.org/10.1093/imrn/rnac262.

  18. J.-F. Quint, Mesures de Patterson–Sullivan en rang superieur, Geometric and Functional Analysis 12 (2002), 776–809.

    Article  MathSciNet  MATH  Google Scholar 

  19. M. Ratner, On Raghunathan’s measure conjecture, Annals of Mathematics 134 (1991), 545–607.

    Article  MathSciNet  MATH  Google Scholar 

  20. T. Roblin, Fr Ergodicité et équidistribution en courbure négative, Mémoires de la Société Mathématique de France 95 (2003).

  21. A. Sambarino, Quantitative properties of convex representations, Commentarii Mathematici Helvetici 89 (2014), 443–488.

    Article  MathSciNet  MATH  Google Scholar 

  22. A. Sambarino, The orbital counting problem for hyperconvex representations, Université de Grenoble. Annales de l’Institut Fourier 65 (2015), 1755–1797.

    Article  MathSciNet  MATH  Google Scholar 

  23. K. Schmidt, Cocycles on Ergodic Transformation Groups, Macmillan Lectures in Mathematics, Vol. 1, Macmillan of India, Delhi, 1977.

    MATH  Google Scholar 

  24. W. Veech, Unique ergodicity of horospherical flows, American Journal of Mathematics 99 (1977), 827–859.

    Article  MathSciNet  MATH  Google Scholar 

  25. A. Wienhard, An invitation to higher Teichmüller theory, in Proceedings of the International Congress of Mathematicians—Rio de Janeiro 2018. Vol. II, World Scientific, Hackensack, NJ, 2018, pp. 1013–1039.

    MATH  Google Scholar 

  26. D. Winter, Mixing of frame flow for rank one locally symmetric spaces and measure classification, Israel Journal of Mathematics 210 (2015), 467–507.

    Article  MathSciNet  MATH  Google Scholar 

  27. R. Zimmer, Ergodic Theory and Semisimple Groups, Monographs in Mathematics, Vol. 81, Birkhäuser, Basel, 1984.

    Book  MATH  Google Scholar 

Download references

Acknowledgements

We would like to thank Michael Hochman for helpful conversations, especially for telling us about the reference [11]. We also thank the referee for reading the manuscript carefully and making a useful suggestion.

Author information

Authors and Affiliations

  1. Deartment of Mathematics, University of Chicago, Chicago, IL, 60637, USA

    Minju Lee

  2. Mathematics Department, Yale University, New Haven, CT, 06520, USA

    Hee Oh

Authors
  1. Minju Lee
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hee Oh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hee Oh.

Additional information

Lee and Oh respectively supported by the NSF grants DMS-1926686 (via the Institute for Advanced Study) and DMS-1900101.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lee, M., Oh, H. Ergodic decompositions of geometric measures on Anosov homogeneous spaces. Isr. J. Math. (2023). https://doi.org/10.1007/s11856-023-2560-2

Download citation

  • Received:

  • Revised:

  • Published:

  • DOI: https://doi.org/10.1007/s11856-023-2560-2

Search

Navigation