Skip to main content
SpringerLink
Log in

Application of Bernstein polynomials for solving Fredholm integro-differential-difference equations

  • Published:
Applied Mathematics-A Journal of Chinese Universities Aims and scope Submit manuscript

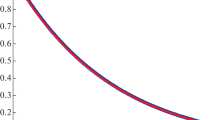
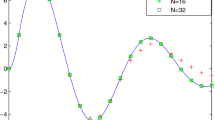
Abstract

In this paper, the Bernstein polynomials method is proposed for the numerical solution of Fredholm integro-differential-difference equation with variable coefficients and mixed conditions. This method is using a simple computational manner to obtain a quite acceptable approximate solution. The main characteristic behind this method lies in the fact that, on the one hand, the problem will be reduced to a system of algebraic equations. On the other hand, the efficiency and accuracy of the Bernstein polynomials method for solving these equations are high. The existence and uniqueness of the solution have been proved. Moreover, an estimation of the error bound for this method will be shown by preparing some theorems. Finally, some numerical experiments are presented to show the excellent behavior and high accuracy of this algorithm in comparison with some other well-known methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A Akyüz-Daşcıoglu. A Chebyshev polynomial approach for linear Fredholm-Volterra integro-differential equations in the most general form, Appl Math Comput, 2006, 181(1): 103–112.

    MathSciNet  MATH  Google Scholar 

  2. O Baghani, H Baghani. A new contraction condition and its application to weakly singular Volterra integral equations of the second kind, J Fixed Point Theory Appl, 2017, 19(4): 2601–2615.

    Article  MathSciNet  MATH  Google Scholar 

  3. S Bhattacharya, B N Mandal. Use of Bernstein polynomials in numerical solutions of Volterra integral equations, Appl Math Sci, 2008, 2(36): 1773–1787.

    MathSciNet  MATH  Google Scholar 

  4. R L Burden, J D Faires. Numerical analysis, Weber & Schmidt, Boston, 1985.

    MATH  Google Scholar 

  5. D F Han, X F Shang. Numerical solution of integro-differential equations by using CAS wavelet operational matrix of integration, Appl Math Comput, 2007, 194(2): 460–466.

    MathSciNet  MATH  Google Scholar 

  6. L M Delves, J L Mohamed. Computational methods for integral equations, Cambridge, University Press, Cambridge, 1985.

    Book  MATH  Google Scholar 

  7. R A Devore, G G Lorentz. Constructive approximation, Springer Science & Business Media, New York, 1993.

    Book  MATH  Google Scholar 

  8. B Gürbüz, M Sezer, C Güler. Laguerre collocation method for solving Fredholm integro-differential equations with functional arguments, J Appl Math, 2014, 2014, Article ID 682398, https://doi.org/10.1155/2014/682398.

  9. E Hesameddini, M Khorramizadeh, M Shahbazi. Bernstein polynomials method for solving Volterra-Fredholm integral equations, Bull Math Soc Sci Math Roumanie, 2017, 60(1): 59–68.

    MathSciNet  MATH  Google Scholar 

  10. E Hesameddini, M Shahbazi. Legendre collocation method and its convergence analysis for the numerical solutions of the conductor-like screening model for real solvents integral equation, Bull Comput Appl Math, 2017, 5(1): 33–49.

    MathSciNet  MATH  Google Scholar 

  11. E Hesameddini, M Shahbazi. Solving system of Volterra-Fredholm integral equations with Bernstein polynomials and hybrid Bernstein Block-Pulse functions, J Comput Appl Math, 2017, 315: 182–194.

    Article  MathSciNet  MATH  Google Scholar 

  12. F Hosseini Shekarabi, K Maleknejad, R Ezzati. Application of two-dimensional Bernstein polynomials for solving mixed Volterra-Fredholm integral equations, Afrika Mat, 2015, 26: 1237–1251.

    Article  MathSciNet  MATH  Google Scholar 

  13. K Ivaz, S Shahmorad, B S Mostahkam. Newton-Tau numerical solution of one dimensional nonlinear integro-differential equations, SEA bull math, 2009, 33(4): 733–740.

    MathSciNet  MATH  Google Scholar 

  14. M A Jaswon, G T Symm. Integral equation methods in potential theory and elastostatics, Academic Press, London, 1977.

    MATH  Google Scholar 

  15. S B G Karakoç, A Eryılmaz, M Başbük. The approximate solutions of Fredholm integro-differential-difference equations with variable coefficients via homotopy analysis method, Math Probl Eng, 2013, 2013, Article ID 261645, https://doi.org/10.1155/2013/261645.

  16. A Kurt, S Yalçinbaş, M Sezer. Fibonacci collocation method for solving high-order linear Fredholm integro-differential-difference equations, Int J Math Math Sci, 2013, 2013, Article ID 486013, https://doi.org/10.1155/2013/486013.

  17. K Maleknejad, Y Mahmoudi. Taylor polynomial solution of high-order nonlinear Volterra-Fredholm integro differential equations, Appl Math Comput, 2003, 145: 641–653.

    MathSciNet  MATH  Google Scholar 

  18. B N Mandal, S Bhattacharya. Numerical solution of some classes of integral equations using Bernstein polynomials, Appl Math Comput, 2007, 190: 1707–1716.

    MathSciNet  MATH  Google Scholar 

  19. S Micula. On some iterative numerical methods for a Volterra functional integral equation of the second kind, J Fixed Point Theory Appl, 2017, 19(3): 1815–1824.

    Article  MathSciNet  MATH  Google Scholar 

  20. J Saberi-Nadjafi, A Ghorbani. He’s homotopy perturbation method: An effective tool for solving nonlinear integral and integro-differential equations, Comput Math Appl, 2009, 58(11–12): 2379–2390.

    Article  MathSciNet  MATH  Google Scholar 

  21. P K Sahu, S S Sahu. Legendre spectral collocation method for Fredholm integro-differential-difference equation with variable coefficients and mixed conditions, Appl Math Comput, 2015, 268: 575–580.

    MathSciNet  MATH  Google Scholar 

  22. P K Sahu, S S Ray. Legendre wavelets operational method for the numerical solutions of nonlinear Volterra integro-differential equations system, Appl Math Comput, 2015, 256: 715–723.

    MathSciNet  MATH  Google Scholar 

  23. P K Sahu, S S Ray. Sinc-Galerkin technique for the numerical solution of fractional Volterra-Fredholm integro-differential equations with weakly singular kernels, Int J Nonlinear Sci Numer Simul, 2016, 17(6): 315–323.

    Article  MathSciNet  MATH  Google Scholar 

  24. P Schiavane, C Constanda, A Mioduchowski. Integral methods in science and engineering, Birkhaäuser, Boston, 2002.

    Google Scholar 

  25. M Sezer, M Gülsu. Polynomial solution of the most general linear Fredholm-Volterra integro differential-difference equations by means of Taylor collocation method, Appl Math Comput, 2007, 185(1): 646–657.

    MathSciNet  MATH  Google Scholar 

  26. E Tohidi. Taylor matrix method for solving linear two-dimensional Fredholm integral equations with piecewise intervals, Comp Appl Math, 2015, 34(3): 1117–1130.

    Article  MathSciNet  MATH  Google Scholar 

  27. S Yalçinbaş, T Akkaya. A numerical approach for solving linear integro-differential-difference equations with Boubaker polynomial bases, Ain Shams Eng J, 2012, 3(2): 153–161.

    Article  Google Scholar 

  28. S Yalçcinbaş, M Sezer. A Taylor collocation method for the approximate solution of general linear Fredholm Volterra integro-difference equations with mixed argument, Appl Math Comput, 2006, 175(1): 675–690.

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics Sciences, Shiraz University of Technology, P. O. Box 71555-313, Shiraz, Iran

    Esmail Hesameddini & Mehdi Shahbazi

Authors
  1. Esmail Hesameddini
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mehdi Shahbazi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Esmail Hesameddini or Mehdi Shahbazi.

Additional information

Supported by the Shiraz University of Technology, Shiraz, Iran.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hesameddini, E., Shahbazi, M. Application of Bernstein polynomials for solving Fredholm integro-differential-difference equations. Appl. Math. J. Chin. Univ. 37, 475–493 (2022). https://doi.org/10.1007/s11766-022-3620-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11766-022-3620-9

MR Subject Classification

Keywords

Search

Navigation