Mathematical Study of a Resource-Based Diffusion Model with Gilpin–Ayala Growth and Harvesting

Abstract

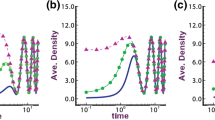
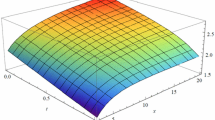
This paper focuses on a Gilpin–Ayala growth model with spatial diffusion and Neumann boundary condition to study single species population distribution. In our heterogeneous model, we assume that the diffusive spread of population is proportional to the gradient of population per unit resource, rather than the population density itself. We investigate global well-posedness of the mathematical model, determine conditions on harvesting rate for which non-trivial equilibrium states exist and examine their global stability. We also determine conditions on harvesting that leads to species extinction through global stability of the trivial solution. Additionally, for time periodic growth, resource, capacity and harvesting functions, we prove existence of time-periodic states with the same period. We also present numerical results on the nature of nonzero equilibrium states and their dependence on resource and capacity functions as well as on Gilpin–Ayala parameter $\theta$. We conclude enhanced effects of diffusion for small $\theta$ which in particular disallows existence of nontrivial states even in some cases when intrinsic growth rate exceeds harvesting at some locations in space for which a logistic model allows for a nonzero equilibrium density.

A Appendix

In this section, we present some lemmas that are already known (see, for example Pao 1992) and presented here for the sake of completeness. Consider

$$\begin{aligned} \begin{aligned} \frac{\partial v}{\partial t} +{\mathcal {L}} v&= \mu (t, x, v) \ , \quad (t, x)\in {\mathbb {A}}_T \ , \\ \frac{\partial v}{\partial n}&= 0 \ , \quad (t, x) \in S_T \ , \\ v (0, x)&= v_0 (x) \ , \quad x \in \Omega . \end{aligned} \end{aligned}$$

(68)

where ${\mathcal {L}} v$ is a uniformly elliptic operator. It will be assumed that $\mu$ is a $C^1$ function of its arguments for $(t,x, v) \in \overline{{\mathbb {Z}}} \times I$ where $I \subset {\mathbb {R}}$ is some suitable finite sub-interval that contains some upper and lower solutions as defined shortly.

Definition 1

A function $v^* \in {\mathcal {S}}_T$ is an upper solution of (68) if it satisfies

$$\begin{aligned} \begin{aligned}&\frac{\partial v^*}{\partial t} + {\mathcal {L}} v^* - \mu (t, x, v^*) \ge 0 \ , \quad (t, x)\in {\mathbb {A}}_T \ , \\&\frac{\partial v^*}{\partial n} \ge 0 \ , \quad (t, x) \in S_T \ , \\&v^* (0, x) - v_0 (x) \ge 0 \ , \quad x \in \Omega . \end{aligned} \end{aligned}$$

(69)

A function $v_* \in {\mathcal {S}}_T$ is a lower solution of (68) if $v_*$ satisfies the same conditions as in (69), except with inequalities $\ge 0$ replaced by $\le 0$. The upper-lower solution pair $(v_*, v^*)$ is said to be ordered if $v^* \ge v_*$ in ${\mathbb {A}}_T$. We denote $\langle v_*, v^* \rangle$ to be the all continuous functions $v \in C \left( \overline{{\mathbb {A}}_T} \right)$ with the property that $v_* \le v \le v^*$.

In what follows, we will assume that for an ordered set of lower and upper solutions $(v_*, v^*)$, there exists $m_* (t, x), m^* (t, x) \in C^1 \left( \overline{{\mathbb {A}}_T} \right)$ such that for $v_* \le v_2 \le v_1 \le v^*$,

$$\begin{aligned} -m_* (v_1-v_2) \le \mu \left( t, x, v_1 \right) - \mu \left( t, x, v_2 \right) \le m^* (v_1 - v_2) \end{aligned}$$

(70)

We define

$$\begin{aligned} {\mathbb {G}} (t, x, v) = m_* v + \mu \left( t, x, v \right) \ . \end{aligned}$$

(71)

The left inequality in (70) implies that ${\mathbb {G}} (t, x, v)$ is a non-decreasing function of $v \in (v_*, v^*)$.

Definition 2

Define lower $\left\{ {\underline{v}}^{(k)} \right\} _{k=1}^\infty$ and upper sequences $\left\{ {\overline{v}}^{(k)} \right\} _{k=1}^\infty$ and each of which satisfy the recursion relation

$$\begin{aligned} \begin{aligned} \frac{\partial {v}^{(k)}}{\partial t} + {\mathcal {L}} {v}^{(k)} + m_* {v}^{(k)}&= {\mathbb {G}} \left( t, x, {v}^{(k-1)} \right) \ , \quad (t, x) \in {\mathbb {A}}_T \\ \frac{\partial v^{(k)}}{\partial n}&= 0 \ , \quad (t, x) \in S_T\ , \\ {v}^{(k)} (0, x)&= v_0 (x), \quad x \in \Omega . \end{aligned} \end{aligned}$$

(72)

where $v^{(0)} = v_*$ and $v^{(0)} = v^*$ in cases of lower and upper-sequences, respectively.

Remark 5

If $v^{(k-1)} \in C^{1,2} \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}_T} \right)$, from given properties of $\mu$ and $m_*$, ${\mathbb {G}} \left( t, x, {v}^{(k-1)} (t, x) \right) \in C^1 \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}_T} \right)$, and therefore, using properties of Green’s function of the operator $\partial _t +{\mathcal {L}} + m_*$ with Neumann conditions, it follows that $v^{(k)} \in C^{1,2} \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}_T} \right)$. Therefore, since $v^0 \in C^{1,2} \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}_T} \right)$, it follows by induction that $v^{(k)} \in C^{1,2} \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}_T} \right)$ for all $k \in {\mathbb {Z}}^+$.

Theorem 7

For any $(t, x) \in {\mathbb {A}}_T$, $\left\{ {\underline{v}}_k (t, x) \right\} _{k=1}^\infty$ and $\left\{ {\overline{v}}_k (t, x) \right\} _{k=1}^\infty$ are non-decreasing and non-increasing sequences respectively satisfying following inequalities for each k

$$\begin{aligned} v_* (t, x) \le {\underline{v}}_k (t, x) \le {\overline{v}}_k (t, x) \le v^* (t, x) \end{aligned}$$

(73)

and each of the sequences ${\underline{v}}_k, {\overline{v}}_k$ converge in $C^{1, 2} \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}} \right)$ to the same solution v(t, x) of the initial value problem.

Proof

Assume the statement is true for a certain k. Now, consider

$$\begin{aligned} w = {\underline{v}}^{(k+1)}-{\underline{v}}^{(k)} \end{aligned}$$

(74)

Clearly since ${\mathbb {G}} (t, x, v)$ is a non-decreasing function of v, w satisfies

$$\begin{aligned} \frac{\partial w}{\partial t} + {\mathcal {L}} w + m_* w = {\mathbb {G}} \left( t, x, {\underline{v}}^{(k)} \right) -{\mathbb {G}} \left( t, x, {\underline{v}}^{(k-1)} \right) \end{aligned}$$

(75)

while satisfying homogeneous initial and boundary conditions. Therefore $w \ge 0$. Furthermore, from maximum principle if $w =0$ at some interior point, it must be identically zero. Therefore ${\underline{v}}^{(k+1)} (t, x) \ge {\underline{v}}^{(k)} (t, x)$, the equality holding only if they are identical. Similarly, if we define $w= {\overline{v}}^{(k)} - {\overline{v}}^{(k+1)}$, the induction statement applied to k and the fact that ${\mathbb {G}}$ is non-decreasing in v implies ${\mathbb {G}} \left( t, x, {\overline{v}}^{(k-1)} \right) \ge {\mathbb {G}} \left( t, x, {\overline{v}}^{(k)} \right) \ge 0$, it follows in this case as well, $\partial _t w + {\mathcal {L}} w + m_* w \ge 0$ while satisfying homogeneous initial and boundary condition. It follows that $w \ge 0$, with $w=0$ at some interior point only if ${\overline{v}}^{(k+1)} (t, x) = {\overline{v}}^{(k)} (t,x)$ for any $(t, x) \in {\mathbb {A}}_T$. Again taking $w = {\overline{v}}^{(k+1)} - {\underline{v}}^{(k+1)}$, it follows that

$$\begin{aligned} \partial _t w + {\mathcal {L}} w + m_* w = {\mathbb {G}} \left( t, x, {\overline{v}}^{(k)} \right) -{\mathbb {G}} \left( t, x, {\underline{v}}^{(k)} \right) \ge 0 \end{aligned}$$

(76)

and the same argument holds, implying that $w \ge 0$, and thus ${\overline{v}}^{(k+1)} (t, x) \ge {\underline{v}}^{(k+1)} (t, x)$ with equality holding at some point in ${\mathbb {A}}_T$ only if the two functions are identical. Therefore, we have proved (77). Therefore, from monotonicity of each of the sequences $\left\{ {\underline{v}}^{(k)} (t, x) \right\} _{k=1}^\infty$ and $\left\{ {\overline{v}}^{(k)} (t, x) \right\} _{k=1}^\infty$ for any $(t, x) \in {\mathbb {A}}_T$, one increasing with an upper bound, the other decreasing with a lower bound, we have pointwise convergence to a function ${\underline{v}} (t, x)$ and ${\overline{v}} (t, x)$, respectively. Now, since using Green’s function we may write the solution $v^{(k)} (t, x) \in C^{1,2} ({\mathbb {A}}_T ) \cup C \left( \overline{{\mathbb {A}}}_T \right)$ satisfying (72) may be written as an integral equation

$$\begin{aligned} v^{(k)} (t, x)&= \int _0^t \int _{\Omega } {\mathbb {G}} \left( t, x, \tau , \xi \right) {\mathbb {G}} \left( \tau , \xi , v^{(k-1)} \left( \tau , \xi \right) \right) \mathrm{d}\xi \mathrm{d}\tau \nonumber \\&\quad + \int _{\Omega } {\mathbb {G}}_0 \left( t, x, \xi \right) v_0 (\xi ) \mathrm{d}\xi \end{aligned}$$

(77)

applying dominating convergence theorem, it follows that the limiting functions, either ${\underline{v}}$ or ${\overline{v}}$ solves the integral equation

$$\begin{aligned} v (t, x) = \int _0^t \int _{\Omega } {\mathbb {G}} \left( t, x, \tau , \xi \right) {\mathbb {G}} \left( \tau , \xi , v \left( \tau , \xi \right) \right) \mathrm{d}\xi \mathrm{d}\tau + \int _{\Omega } {\mathbb {G}}_0 \left( t, x, \xi \right) v_0 (\xi ) \mathrm{d}\xi \end{aligned}$$

(78)

and therefore is in $C^{(1,2)} \left( {\mathbb {A}}_T \right) \cup C \left( \overline{{\mathbb {A}}_T } \right)$ and a solution to the nonlinear parabolic initial value problem (68). We now argue that ${\underline{v}} = {\overline{v}}$. If we define $w = {\underline{v}} - {\overline{v}}$, using (70) we note it satisfies

$$\begin{aligned} \frac{\partial w}{\partial t} + {\mathcal {L}} w -m^* w \ge 0 , \quad \frac{\partial w}{\partial n} = 0 \;\mathrm{on}\quad \partial \Omega \ , \quad \quad w (0, x) = 0 \end{aligned}$$

(79)

implying that $w \ge 0$. Since $w \le 0$ as well, it follows $w=0$. $\square$

Consider now the steady state problem

$$\begin{aligned} {\mathcal {L}} v = \mu (x, v) \ , \quad \frac{\partial v}{\partial n} = 0 ~\mathrm{on}~ \partial \Omega \end{aligned}$$

(80)

We will assume again that for $v \in J$, there exists $m_*, m^* \in C^1 ({\overline{\Omega }} )$ with the property that for $v_1 \ge v_2$

$$\begin{aligned} \mu (x, v_1) - \mu (x, v_2) \ge - m_* (v_1-v_2) \end{aligned}$$

(81)

where $m_* (x) \ge 0$ and not identically zero. From (81),

$$\begin{aligned} {\mathbb {G}} (x, v) = \mu (x, v) + m_* v \end{aligned}$$

(82)

is a non-decreasing function of v.

Definition 3

We define $v_* , v^* \in C^{2} \left( \Omega \right) \cup C^{\alpha } \left( {\overline{\Omega }} \right)$ to be positive lower and upper solutions of (80) satisfying $v^* (x) \ge v_* (x)$ for each $x \in \Omega$. Furthermore, we define lower and upper sequences $\left\{ {\underline{v}}^{(k)} \right\} _{k=1}^\infty$ and $\left\{ {\overline{v}}^{(k)} \right\} _{k=1}^\infty$ respectively so that each satisfy the recurrence relation

$$\begin{aligned} \begin{aligned}&{\mathcal {L}} {v}^{(k)} + m_* {v}^{(k)} = {\mathbb {G}} \left( x, {v}^{(k-1)} \right) ,\quad x \in \Omega \\&\frac{\partial v^{(k)}}{\partial n} = 0 \ , \quad x \in \partial \Omega . \\ \end{aligned} \end{aligned}$$

(83)

where $v^{0} = v_*$ and ${v}^*$ for lower and upper sequences, respectively.

Remark 6

Since from assumptions ${\mathbb {G}}$ is $C^1$ in v and x, it follows that that if $v^{(k-1)} \in C^2 \left( \Omega \right) \cup C^{\alpha } \left( {\overline{\Omega }} \right)$, ${v}^{(k)} \in C^{2} \left( \Omega \right) \cup C^{\alpha } \left( {\overline{\Omega }} \right)$ from theory of linear elliptic equations.

Theorem 8

For any $x \in \Omega$, $\left\{ {\underline{v}}_k (x) \right\} _{k=1}^\infty$ and $\left\{ {\overline{v}}_k (x) \right\} _{k=1}^\infty$ are non-decreasing and non-increasing sequences respectively satisfying following inequalities for each k

$$\begin{aligned} v_* (x) \le {\underline{v}}_k (x) \le {\overline{v}}_k (x) \le v^* (x) \end{aligned}$$

(84)

and each of the sequences ${\underline{v}}_k, {\overline{v}}_k$ converge in $C^{2} \left( \Omega \right) \cup C^\alpha \left( {\overline{\Omega }} \right)$ to ${\underline{v}}$ and ${\overline{v}}$, respectively, each of is a nonzero solution of (80).

Proof

Assume the statement is true for a certain k. Now, consider

$$\begin{aligned} w = {\underline{v}}^{(k+1)}-{\underline{v}}^{(k)} \end{aligned}$$

(85)

Clearly since ${\mathbb {G}} (x, v)$ is a non-decreasing function of v, w satisfies

$$\begin{aligned} {\mathcal {L}} w + m_* w = {\mathbb {G}} \left( x, {\underline{v}}^{(k)} \right) -{\mathbb {G}} \left( x, {\underline{v}}^{(k-1)} \right) \ge 0 \end{aligned}$$

(86)

while satisfying homogeneous Neumann boundary condition. Therefore $w \ge 0$ since $m_* \ge 0$ and not indentically zero. Furthermore, if $w =0$ at some interior point, it must be identically zero from maximum principle. Therefore, ${\underline{v}}^{(k+1)} (x) \ge {\underline{v}}^{(k)} (x)$, the equality holding only if they are identical. Similarly, if we define $w= {\overline{v}}^{(k)} - {\overline{v}}^{(k+1)}$, the induction statement applied to k and the fact that ${\mathbb {G}}$ is non-decreasing in v implies ${\mathbb {G}} \left( x, {\overline{v}}^{(k-1)} \right) \ge {\mathbb {G}} \left( x, {\overline{v}}^{(k)} \right) \ge 0$, it follows in this case as well, ${\mathcal {L}} w + m_* w \ge 0$ while satisfying homogeneous Neumann boundary condition. It follows that $w \ge 0$, with $w=0$ at some interior point only if ${\overline{v}}^{(k+1)} (t, x) = {\overline{v}}^{(k)} (t,x)$ for any $(t, x) \in {\mathbb {A}}_T$. Again taking $w = {\overline{v}}^{(k+1)} - {\underline{v}}^{(k+1)}$, it follows that

$$\begin{aligned} {\mathcal {L}} w + m_* w = {\mathbb {G}} \left( x, {\overline{v}}^{(k)} \right) -{\mathbb {G}} \left( x, {\underline{v}}^{(k)} \right) \ge 0 \end{aligned}$$

(87)

and the same argument holds, implying that $w \ge 0$, and thus ${\overline{v}}^{(k+1)} (x) \ge {\underline{v}}^{(k+1)} (x)$ with equality holding at some point in $\Omega$ only if the two functions are identical. Therefore, we have proved (84). Therefore, from monotonicity of each of the sequences $\left\{ {\underline{v}}^{(k)} (x) \right\} _{k=1}^\infty$ and $\left\{ {\overline{v}}^{(k)} (x) \right\} _{k=1}^\infty$ for any $x \in \Omega$, one increasing with an upper bound, the other decreasing with a lower bound, we have pointwise convergence to a function ${\underline{v}} (x)$ and ${\overline{v}} (x)$, respectively. Now, since using Green’s function we may write the solution $v^{(k)} (t, x) \in C^{2} (\Omega ) \cup C^\alpha \left( {\overline{\Omega }} \right)$ satisfying (83), may be written as an integral equation

$$\begin{aligned} v^{(k))} (x) = \int _{\Omega } {\mathbb {G}} \left( x, \xi \right) {\mathbb {G}} \left( \xi , v^{(k-1)} \left( \tau , \xi \right) \right) \mathrm{d}\xi \end{aligned}$$

(88)

applying dominating convergence theorem, it follows that the limiting functions, either ${\underline{v}}$ or ${\overline{v}}$ solves the integral equation

$$\begin{aligned} v (x) = \int _{\Omega } {\mathbb {G}} \left( x, \xi \right) {\mathbb {G}} \left( \xi , v \left( \xi \right) \right) \mathrm{d}\xi \end{aligned}$$

(89)

and therefore is in $C^{2} \left( \Omega \right) \cup C^\alpha \left( {\overline{\Omega }} \right)$ and a solution of (80). $\square$

Definition 4

Grönwall’s inequality: Let $\rho :[0,T]\rightarrow \mathbb {R+}$ be a positive differential function for which there exists a constant C so that

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}t}(\rho (t))\le C\rho (t)\ , \quad \mathrm{for all} \quad t\in [0,T] \end{aligned}$$

Then

$$\begin{aligned} \rho (t)\le e^{Ct}\rho (0) \end{aligned}$$