Propagation Length of Self-healing Slip Pulses at the Onset of Sliding: A Toy Model

Abstract

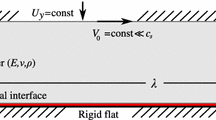
Macroscopic sliding between two solids is triggered by the propagation of a micro-slip front along the frictional interface. In certain conditions, sliding is preceded by the propagation of aborted fronts, spanning only part of the contact interface. The selection of the characteristic size spanned by those so-called precursors remains poorly understood. Here, we introduce a 1D toy model of precursors between a slider and a track in which the fronts are quasi-static self-healing slip pulses. When the slider’s thickness is large compared to the elastic correlation length and when the interfacial stiffness is small compared with the bulk stiffness, we provide an analytical solution for the length of the first precursor, $\varLambda$, and the shear stress field associated with it. These quantities are given as a function of the bulk material parameters, the frictional properties of the interface and the macroscopic loading conditions. Analytical results are in quantitative agreement with the numerical solution of the model. In contrast with previous models, our model predicts that $\varLambda$ does not depend on the frictional breaking threshold of the interface. Our results should be relevant to the various systems in which self-healing slip pulses have been observed.

Appendix: Analytical derivation of Eq. (25)

Let us introduce two dimensionless parameters

$$\begin{aligned} h \equiv \lambda _{\rm c} /H \quad {\rm and} \; q \equiv k/K, \end{aligned}$$

(26)

so that $K_{\rm L} = K/h$, $K_{\rm T} = Kh/2(1+\sigma )$, $\beta = 1/(1+b)$, $1-\beta = b/(1+b)$, $(\lambda _{\rm c} \kappa _{\rm T})^2 = hq/b$, $(\lambda _{\rm c} \kappa )^2 = q \, (1+b)/b$,

$$\begin{aligned} \varepsilon \equiv \frac{\kappa _{\rm T}^2}{\kappa ^2} = \frac{h}{1+b}, \end{aligned}$$

(27)

where $b = 2(1+\sigma )q/h$, and consider the typical system with $h, q \ll 1$. In this case $\varepsilon \ll 1$, so that $\kappa _1^2 \approx \kappa ^2 (1+\beta \varepsilon )$ and $\kappa _2^2 \approx \kappa ^2 \varepsilon \, (1-\beta ) = q \varepsilon /\lambda _{\rm c}^2$, or

$$\begin{aligned} (\lambda _{\rm c} \kappa _2)^{-1} \approx [2(1+\sigma ) + h/q]^{1/2} /h. \end{aligned}$$

(28)

In accordance with the numerics (see Fig. 2a), let us assume that in the case of $h, q \ll 1$ the displacement field in the US is given by

$$\begin{aligned} u_{\rm t} (x) \approx U_{t0} e^{-\kappa _2 x} \end{aligned}$$

(29)

and does not change during front propagation. Before nucleation of the first precursor, the solution of Eq. (7) is

$$\begin{aligned}&u(x) = A_{30} \sinh (\kappa x) + A_{40} \cosh (\kappa x) \\&- \kappa \beta U \int _{0}^{x} {\rm d}\xi \, e^{-\kappa _2 \xi } \sinh [\kappa (x - \xi )] \\&= \frac{1}{2} \left( A_{40} + A_{30} - \frac{1}{2} \, \beta U \frac{\kappa }{\kappa + \kappa _2} \right) e^{\kappa x} \\&+ \frac{1}{2} \left( A_{40} - A_{30} - \beta U \frac{\kappa }{\kappa - \kappa _2} \right) e^{-\kappa x} \\&+ \beta U \frac{\kappa ^2}{\kappa ^2 - \kappa _2^2} e^{-\kappa _2 x} . \end{aligned}$$

(30)

The right-hand-side boundary condition, $u(x) \rightarrow 0$ at $x \rightarrow \infty$, gives us

$$\begin{aligned} A_{40} + A_{30} = \frac{1}{2} \, \beta U \frac{\kappa }{\kappa + \kappa _2} \;, \end{aligned}$$

(31)

while the left-hand-side boundary condition (Eq. (10)) leads to the equation

$$\begin{aligned} (A_{40} - A_{30})(1+\lambda _{\rm c} \kappa )(\kappa + \kappa _2) = \beta U \kappa (1+a \kappa + 2 \lambda _{\rm c} \kappa _2). \end{aligned}$$

(32)

Thus, before nucleation of the first precursor, the IL displacement field is

$$\begin{aligned} u(x) = \frac{\beta U \kappa ^2}{(\kappa ^2 - \kappa _2^2)} \left( e^{-\kappa _2 x} - \frac{\kappa _2}{\kappa } \frac{(1+\lambda _{\rm c} \kappa _2)}{(1+\lambda _{\rm c} \kappa )} \, e^{-\kappa x} \right) . \end{aligned}$$

(33)

Equation (33) allows us to couple the parameters $U \equiv u_{\rm t} (0)$ and $u_{\rm c} \equiv u(0)$:

$$\begin{aligned} U = u_{\rm c} \left( 1+\frac{\kappa _2}{\kappa } \right) / (\beta \varPsi _1)\,, \end{aligned}$$

(34)

where

$$\begin{aligned} \varPsi _1 = 1+\frac{\kappa _2}{\kappa } \frac{\lambda _{\rm c} \kappa }{(1+\lambda _{\rm c} \kappa )}. \end{aligned}$$

(35)

When the displacement of the IL trailing edge reaches the threshold value $u_{\rm s}$ at some $U=U_0 = u_{\rm s} ( 1+ \kappa _2 /\kappa ) / (\beta \varPsi _1)$, the front starts to propagate. In this case the solution of Eq. (7), ahead of the propagating front, $x>s$, where $u_{\rm b} (x) =0$ so that $w(x) = \beta u_{\rm t} (x) = \beta U_0 e^{-\kappa _2 x}$, is given by

$$\begin{aligned}&\widetilde{u}(x;s) = A_3 (s) \, e^{-\kappa (x-s)} + A_4 (s) \, e^{\kappa (x-s)} \\&- \kappa \beta U_{0} \int _{s}^{x} {\rm d}\xi \, e^{-\kappa _2 \xi } \sinh [\kappa (x - \xi )] \\&= \frac{\beta U_0 \kappa ^2 e^{-\kappa _2 x}}{(\kappa ^2 - \kappa _2^2)} + A_3 (s) \, e^{-\kappa (x-s)} + A_4 (s) \, e^{\kappa (x-s)} \\&- \frac{1}{2} \, \beta U_0 \kappa \, e^{-\kappa _2 s} \left[ \frac{e^{\kappa (x-s)}}{(\kappa + \kappa _2)} + \frac{e^{-\kappa (x-s)}}{(\kappa - \kappa _2)} \right]. \end{aligned}$$

(36)

The right-hand-side boundary condition gives us the coefficient $A_4 (s)$,

$$\begin{aligned} A_4 (s) = \frac{1}{2} \, \beta U_0 \frac{\kappa \, e^{-\kappa _2 s}}{(\kappa + \kappa _2)}, \end{aligned}$$

(37)

so that Eq. (36) takes the form

$$\begin{aligned}&\widetilde{u}(x;s) = \frac{\beta U_0 \kappa ^2}{(\kappa ^2 - \kappa _2^2)} \; e^{-\kappa _2 x} \\&+ \left[ A_3 (s) - \frac{1}{2} \, \beta U_0 \frac{\kappa \, e^{-\kappa _2 s}}{(\kappa - \kappa _2)} \right] e^{-\kappa (x-s)} . \end{aligned}$$

(38)

Behind the propagating front, $x<s$, where $w(x) = \beta \, u_{\rm t} (x) + (1-\beta ) \, u_{\rm b} (x)$ and $u_{\rm b} (x) = \widetilde{u}(x+0;x) = A_3 (x) + A_4 (x)$, the solution of Eq. (7) is given by

$$\begin{aligned}&\widetilde{u}(x;s) = A_1 (s) \sinh (\kappa x) + A_2 (s) \cosh (\kappa x) \\&- \kappa \, (1-\beta ) \int _{0}^{x} {\rm d}\xi \, [A_3 (\xi ) + A_4 (\xi )] \, \sinh [\kappa (x - \xi )] \\&- \kappa \beta U_{0} \int _{0}^{x} {\rm d}\xi \, e^{-\kappa _2 \xi } \sinh [\kappa (x - \xi )] \\&= \beta U_0 {\mathcal {F}} (x) + A_1 (s) \sinh (\kappa x) + A_2 (s) \cosh (\kappa x) \\&- \kappa \, (1-\beta ) \int _{0}^{x} {\rm d}\xi \, A_3 (\xi ) \, \sinh [\kappa (x - \xi )], \end{aligned}$$

(39)

where

$$\begin{aligned}&{\mathcal {F}} (x) = \frac{\varPsi _2 \kappa ^2}{(\kappa ^2 - \kappa _2^2)} \\&\times \left( \frac{\kappa _2}{\kappa } \sinh (\kappa x) - \cosh (\kappa x) + e^{-\kappa _2 x} \right) , \end{aligned}$$

(40)

$$\begin{aligned}&\frac{{\mathcal {F}}' (x)}{\kappa } = \frac{\varPsi _2 \kappa ^2}{(\kappa ^2 - \kappa _2^2)} \\&\times \left( \frac{\kappa _2}{\kappa } \cosh (\kappa x) - \sinh (\kappa x) - \frac{\kappa _2}{\kappa } \, e^{-\kappa _2 x} \right) , \end{aligned}$$

(41)

$$\begin{aligned}&\varPsi _2 = \frac{(3-\beta ) \kappa + 2\kappa _2}{2(\kappa +\kappa _2)}, \end{aligned}$$

(42)

so that ${\mathcal {F}} (0) =0$ and ${\mathcal {F}}' (0) =0$.

The coefficients $A_{\dots } (s)$ in these equations are determined by the boundary and continuity conditions. The left-hand-side boundary condition (Eq. (10)) couples the coefficients $A_1 (s)$ and $A_2 (s)$. Using $u_{\rm b} (0)=u_{\rm s}$, $\widetilde{u}(0;s) = A_2 (s)$ and $\widetilde{u}'(0;s) = \kappa A_1 (s)$, we obtain

$$\begin{aligned}&A_2 (s) - (\lambda _{\rm c} \kappa )^{-1} A_1 (s) = \varPsi _3, \\&\varPsi _3 = \beta U_0 + (1-\beta ) \, u_{\rm s}. \end{aligned}$$

(43)

The continuity conditions (Eqs. (23) and (24)) lead to two equations

$$\begin{aligned} \kappa&\, (1-\beta ) \int _{0}^{s} {\rm d}\xi \, A_3 (\xi ) \, \sinh [\kappa (s - \xi )] + A_3 (s) \\&= A_1 (s) \sinh (\kappa s) + A_2 (s) \cosh (\kappa s) + \beta U_0 \varPsi _4 (s) \end{aligned}$$

(44)

and

$$\begin{aligned} \kappa&\, (1 -\beta ) \int _{0}^{s} {\rm d}\xi \, A_3 (\xi ) \, \cosh [\kappa (s - \xi )] - A_3 (s) \\&= A_1 (s) \cosh (\kappa s) + A_2 (s) \sinh (\kappa s) + \beta U_0 \varPsi _5 (s), \end{aligned}$$

(45)

where

$$\begin{aligned} \varPsi _4 (s)&= {\mathcal {F}} (s) - \frac{\kappa \, e^{-\kappa _2 s}}{2 (\kappa + \kappa _2)} \;, \end{aligned}$$

(46)

$$\begin{aligned} \varPsi _5 (s)&= \frac{{\mathcal {F}}' (s)}{\kappa } - \frac{\kappa \, e^{-\kappa _2 s}}{2 (\kappa + \kappa _2)} \;. \end{aligned}$$

(47)

Taking the difference and sum of Eqs. (44) and (45), we obtain two new equations:

$$\begin{aligned}&2 A_3 (s) \, e^{\kappa s} - \kappa \, (1-\beta ) \int _{0}^{s} {\rm d}\xi \, A_3 (\xi ) \, e^{\kappa \xi } \\&= A_2 (s) - A_1 (s) + \beta U_0 [\varPsi _4 (s) - \varPsi _5 (s)] \, e^{\kappa s} , \end{aligned}$$

(48)

$$\begin{aligned}&\kappa \, (1-\beta ) \int _{0}^{s} {\rm d}\xi \, A_3 (\xi ) \, e^{-\kappa \xi } \\&= A_2 (s) + A_1 (s) + \beta U_0 \left[ \varPsi _4 (s) + \varPsi _5 (s) \right] \, e^{-\kappa s} . \end{aligned}$$

(49)

Using Eq. (43), Eqs. (46) and (47) may be rewritten as

$$\begin{aligned} 2 A_3 (s) \, e^{\kappa s}&- \kappa \, (1-\beta ) \int _{0}^{s} {\rm d}\xi \, A_3 (\xi ) \, e^{\kappa \xi } = A_1 (s) \frac{(1- \lambda _{\rm c} \kappa )}{\lambda _{\rm c} \kappa } \\&+ \varPsi _3 + \beta U_0 [\varPsi _4 (s) - \varPsi _5 (s)] \, e^{\kappa s} , \end{aligned}$$

(50)

$$\begin{aligned} \kappa \, (1&-\beta ) \int _{0}^{s} {\rm d}\xi \, A_3 (\xi ) \, e^{-\kappa \xi }= A_1 (s) \frac{(1+ \lambda _{\rm c} \kappa )}{\lambda _{\rm c} \kappa } \\&+ \varPsi _3 + \beta U_0 \left[ \varPsi _4 (s) + \varPsi _5 (s) \right] \, e^{-\kappa s} . \end{aligned}$$

(51)

Combining these equations, we finally come to the integral equation for the coefficient $A_3 (s)$:

$$\begin{aligned}&A_3 (s) (1+\lambda _{\rm c} \kappa ) \, e^{\kappa s} \\&- \kappa (1-\beta ) \int _0^s {\rm d}\xi \, A_3 (\xi ) \, [\cosh (\kappa \xi ) + (\lambda _{\rm c} \kappa ) \sinh (\kappa \xi )] \\&= \lambda _{\rm c} \kappa \varPsi _3 + \beta U_0 \varPsi _2 \varPsi _6 (s), \end{aligned}$$

(52)

where

$$\begin{aligned} \varPsi _6 (s)&= \frac{\kappa \, (1+\lambda _{\rm c} \kappa )}{2(\kappa -\kappa _2)} \, e^{(\kappa -\kappa _2) s} \\&- \frac{\kappa \, (\lambda _{\rm c} \kappa ^2 + \kappa _2)}{(\kappa ^2 - \kappa _2^2)} \left[ 1+e^{-(\kappa +\kappa _2) s} \right] \end{aligned}$$

(53)

so that

$$\begin{aligned} \varPsi _6^{\prime } (s) = \left[ \frac{(1+\lambda _{\rm c} \kappa )}{2} \, e^{\kappa s} +\frac{(\lambda _{\rm c} \kappa ^2 + \kappa _2)}{(\kappa - \kappa _2)} \, e^{-\kappa s} \right] \kappa \, e^{-\kappa _2 s} . \end{aligned}$$

(54)

From Eq. (52) we find that

$$\begin{aligned} A_3 (0) = [\lambda _{\rm c} \kappa \varPsi _3 + \beta U_0 \varPsi _2 \varPsi _6 (0)]/(1+\lambda _{\rm c} \kappa ). \end{aligned}$$

(55)

Differentiating Eq. (52), we obtain a differential equation for $A_3 (s)$:

$$\begin{aligned} & \frac{1}{\kappa } \, A'_3 (s) + A_3 (s) = A_3 (s) \, \frac{(1-\beta )}{(1+\lambda _{\rm c} \kappa )} \\ &\times [\cosh (\kappa s) + (\lambda _{\rm c} \kappa ) \sinh (\kappa s)] \, e^{-\kappa s} \\ &+ \frac{\beta U_0 \varPsi _2}{\kappa \, (1+\lambda _{\rm c} \kappa )} \varPsi _6^{\prime } (s) \, e^{-\kappa s}. \end{aligned}$$

(56)

From Eq. (56) we obtain that at short distances, $s \ll \kappa ^{-1}$, $A_3 (s) \approx A_3 (0) (1+ \gamma _3 s)$, where

$$\gamma _3 = -\frac{\kappa }{1+ \lambda _{\rm c} \kappa } \left[ \beta +\lambda _{\rm c} \kappa - \frac{\beta U_0}{A_3 (0)} \varPsi _2 \frac{\varPsi _6^{\prime } (0)}{\kappa } \right].$$

(57)

From Eqs. (37) and (56) it follows that $\widetilde{u} (s+0;s) = A_3 (s) + A_4 (s) \approx A_0 \, (1+\gamma s)$ at short distances, $s \ll \kappa ^{-1}$, where

$$A_0 = A_3 (0) + A_4 (0)$$

(58)

and

$$\gamma = [ \gamma _3 A_3 (0) - \kappa _2 A_4 (0) ]/A_0,$$

(59)

while for long distances, $s \gg \kappa ^{-1}$, $\widetilde{u} (s+0;s)$ decays exponentially,

$$\begin{aligned} & \widetilde{u} (s+0;s) \approx {\mathcal {A}} \, e^{-\kappa _2 s} , \\ &{\mathcal {A}} = \beta U_0 \left[ \frac{\kappa }{2 (\kappa + \kappa _2)} + \frac{\varPsi _2}{(1+\beta -2 \kappa _2 /\kappa )} \right] . \end{aligned}$$

(60)

The function $\widetilde{u} (s+0;s)$ may be approximated as

$$\widetilde{u} (s+0;s) \approx A_0 \; \frac{(1+C)^{\alpha } \, e^{\kappa _3 s}}{(e^{\kappa _3 s} +C)^{\alpha }},$$

(61)

where

$$\alpha = 1+ \kappa _2 /\kappa _3,$$

(62)

$$C= (\kappa _2 + \gamma )/(\kappa _3 - \gamma ),$$

(63)

and comparing Eqs. (59) and (61), we obtain a nonlinear equation, which defines the value $\kappa _3$:

$$\ln \frac{\mathcal {A}}{A_0} = \left( 1+ \frac{\kappa _2}{\kappa _3} \right) \ln \frac{\kappa _2 + \kappa _3}{\kappa _3 - \gamma }.$$

(64)

Then, the IL stress ahead of the front is $\sigma _{\rm c} (s) = k \, \widetilde{u} (s+0;s) /\lambda _{\rm c}^2$, and the equation $\sigma _{\rm c} (\varLambda ) = \sigma _{\rm s}$ defines the characteristic length $\varLambda$:

$$\varLambda \approx \kappa _3^{-1} \ln y,$$

(65)

where $y$ is determined by the solution of the equation ${\mathcal {B}} y = (y+C)^{\alpha }$ with ${\mathcal {B}} = (1+C)^{\alpha } k A_0 /(\sigma _{\rm s} \lambda _{\rm c}^2)$.

Using Eq. (60), $\varLambda$ may approximately be presented as

$$\begin{aligned} \varLambda&\approx \kappa _2^{-1} \ln \left( k {\mathcal {A}}/\sigma _{\rm s} \lambda _{\rm c}^2 \right) \\ &= \frac{1}{\kappa _2} \ln \left[ \frac{2}{(1+\beta -2 \kappa _2 /\kappa ) \, \varPsi _1} \right]. \end{aligned}$$

(66)

Equation (65) corresponds to the analytical solution for $\varLambda$, whereas Eq. (66) corresponds to the approximated analytical solution provided as Eq. (25) in the main text.