Abstract
I present a generalized dynamic model of firm behavior under rate of return regulation. The modeled firm has access to multiple types of capital which are substitutes (imperfect or perfect) in production. These capital inputs are differentiated based on durability and heterogeneous marginal effects on the firm’s total cost of capital. This approach is kept general but is motivated by the stylized shape of the bond yield curve, wherein high-durability (longer lived) assets command a higher required return on investment (higher bond yield). The results indicate that a regulated firm (relative to an unregulated firm) will over or under-invest in specific assets depending on their durability and the size of the assets’ marginal effects on the cost of capital relative to the regulated rate of return. Two specific sources of distortion in the capital structure are identified. The “yield curve” effect pushes the firm further (relative to the unconstrained case) towards assets with a low marginal contribution to the cost of capital, thus reducing the firm’s average cost of capital. The “duration” effect pushes the firm towards longer lived assets as a means to inflate the steady state capital stock. This is similar to (yet distinct from) the classic Averch and Johnson (Am Econ Rev 52(5):1052–1069, 1962) result.

Similar content being viewed by others
Notes
Another common alternative to standard rate of return regulation is the use of Negotiated Settlements between producers and consumers. In practice however, settlement outcomes are often based on, or are a modification of, the existing rate of return framework they are replacing. See Fellows (2011).
The referenced reduction in average cost of capital does not imply a reduction in overall capital cost, as the total stock of capital may or may not increase as a result of this shift.
Google Scholar indicates approximately 2452 citations of the original Averch and Johnson (1962) article, over 1000 of which are in the last decade alone. I therefore limit myself to a selection of noteworthy citations.
The logic is straightforward; a regulated firm faces a revenue cap based largely on a regulated rate return applied to the firm’s and capital stock. Increasing capital inputs relative to labor inputs increases the revenue cap by a greater amount than costs and therefore leads to higher profits relative to the cost minimizing case.
For an example of this in practice, see: National Energy Board of Canada (1995).
Elaborating on the capital labor substitution issue: The model developed below can accommodate, but does not rely on, and assumption of Leontief production between Labor and capital inputs. See footnote 18 for a technical description in the context of the model presented here.
Schroeder (2010) provides an equation governing one dimensional, compressible fluid flow, derived in part from the Fanning friction equation. Using Schroeder’s generalized equation as a base, the fluid flow equation can be simplified as: \(Q = D^{2.5} \cdot \sqrt{\left( P_{inlet} ^2 - P_{outlet} ^2 \right) } \cdot \varTheta \), where \(Q\) indicates cubic feet per day, \(P_{inlet}\) is the inlet pressure (PSIA), \(P_{outlet}\) is the outlet pressure (PSIA) and \(\varTheta \) is a function of other parameters including the length of the pipeline and the specific gravity of the gas being transported. Holding the pipe outlet pressure (and other parameters) constant, the marginal rate of technical substitution between inlet pressure and diameter is calculated as: \(MRTS_{P_{inlet} , D} = 2.5 \frac{P_I ^2 - P_O ^2}{D \cdot P_I}. \) which indicates a convex isoquant for any level of output \(Q\). Whereas the associated isocost contour is unlikely to exhibit the classic linear shape, the associated optimum (or optima) would certainly be interior and exhibit marginal trade-offs.
See: Westcoast Energy Inc’s (2003) Transmission Depreciation Study for an example of practical discussion of these issues in the context of a regulated pipeline.
The example here refers to substitutions made on a single physical pipeline, but it is simple to extend the analysis to the case of building a second parallel line running through the same compressor stations. This is referred to “looping” in the industry taxonomy.
Unlike the previous two examples, the repair or replace decision is greatly impacted by the distinction between physical depreciation and financial depreciation. Repairing an asset (i.e., regular maintenance), influences the physical depreciation on an input by acting to preserve its productive capacity. However this change in physical depreciation may or may not be reflected in the financial depreciation rate determined by the GAAP. I comment further on this distinction in the model construction section below.
Averch and Johnson consider a single exogenous cost of capital defined as “... the interest cost involved in holding plant and equipment Averch and Johnson (1962), p. 1054.” This suggests that they implicitly assume their modeled firm to be entirely debt financed.
See for examle: Buranabunyut and Peoples (2012), p. 186.
It is interesting here to note that, while Dew-Becker (2012) provides strong evidence of a relationship between the yield curve and the composition of assets, he is unable to provide evidence that this relationship carries over to the composition of debt. These results are consistent with the interpretation that the change in asset composition changes the opportunity cost of capital (internalized by the firm) which is distinct from the nominal cost of capital (determined by the financing decisions).
If there is only one element in the set N, then the model collapses to a something approximating a standard AJ model (with added dynamics, which become largely irrelevant since the firm has only one feasible choice for its average depreciation rate). This special case of the model makes no useful contribution to extant literature. I direct interested readers to Caputo and Partovi (2002), who provide a generalized and comprehensive examination of a the static AJ model.
I implicitly assume that \(W\) and \(L_t\) are a single scalar and variable, however the model is robust to including a vector of differentiated labor inputs and associated wage rates in which case \(W\) and \(L_t\) would be vectors.
More specifically the function \(F(K_t,L_t)\) is assumed to produce a negative definite Hessian Matrix, with elements; \(\left\{ \frac{\partial F(K_t,L_t)}{\partial k_{i,t}} >0 \; ; \; \frac{\partial ^2 F(K_t,L_t)}{\partial k_{i,t}^2} <0 \; \right\} \forall i \in N \) and \(\frac{\partial ^2 F(K_t,L_t)}{\partial k_{i,t} \partial k_{i,t}} >0 \; \forall i \ne j \in N \)
As indicated above, the model is robust to a Leontief relationship between capital and labor. Formally, \(F(K_t,L_t)\) can take the form: \(F(K_t,L_t) = F \left( min \left\{ L_t , G(K_t) \right\} \right) \) (where \(G(K_t)\) is some function of the vector \(K_t\)) without loss of generality.
\(r_{\!f}\) is the firm’s discount rate on cash flow. Because there is no explicitly modeled risk in the net cash flow equation, \(r_{\!f}\) is assumed to be exogenous through time and is treated as a fixed short-term risk-free rate of return.
For more details see: Chiang (2000), section 8.2, pp. 210–212.
I ignore the non-negativity constraint on labor input, as it lends no useful insight into the model implications.
Despite the revenue constraint, both the firm’s output, and it’s revenues are endogenous and jointly determined by the level of capital investment. Since the firm must produce output sufficient to generate the revenues it is allowed to collect under the rate-of-return constraint, there are no explicit minimum quality or quantity constraints in Eq. (5).
Together, Eqs. (7) and (10) and Lemma 1 imply that \(q_i \ge 0\) and \(\eta _i \le 0 \; \forall i \in N\). This is intuitive as capital used in production should have a positive effect on the future profit stream whereas the liability of that capital stock should have a negative effect on the future profit stream.
Equation 15 draws from the derivatives of a revenue function \(F(\;)\), rather than directly from a production function. However, for a single product firm, the definitions are identical. \( \frac{\left( \frac{\delta F}{\delta k_i} \right) }{\left( \frac{\delta F}{\delta k_j} \right) } = \frac{\left( \frac{\delta F}{\delta Q} \right) \left( \frac{\delta Q}{\delta k_i} \right) }{ \left( \frac{\delta F}{\delta Q} \right) \left( \frac{\delta Q}{\delta k_j} \right) } = \frac{\left( \frac{\delta Q}{\delta k_i}\right) }{ \left( \frac{\delta Q}{\delta k_j} \right) } = MRTS_{i,j} \)
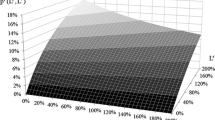
The specific values used in the table are not directly based on empirical data; however, the values are generally consistent with the range of estimated or observed corollaries found in the existing literature.
Essentially, that the revenue function produces a negative definite Hessian matrix, and that the regulatory constraint is binding (and not just binding). See Caputo and Partovi (2002).
Recall that: \(\widehat{B}_i = \frac{\delta _i}{\alpha _i}\widehat{k}_i\) and that \(\widehat{k}_i\) is a function of S. Thus the inequality referenced will produce a threshold value of S, below which the firm will choose \(\widehat{k}_i = 0 \;\; \forall i \in N\) and cease to produce in the optimal steady state.
See also, Church and Ware (2000), p. 850.
This equation is derived by setting \(det \left( A-\varLambda \imath \right) = 0\) where A is the Jacobian matrix of the \(2N\) system given above and \(\imath \) is the identity matrix.
References
Abel, A. B. (1981). Taxes, inflation and the durability of capital. Journal of Political Economy, 89(3), 548–560.
Anderson, T.S. (2005). Diablo canyon power plant: Steam generator repair/replacement cost/benefit analysis. In Proceedings of the 2005 crystal ball user conference.
Averch, H., & Johnson, L. L. (1962). Behaviour of the firm under regulatory constraint. The American Economic Review, 52(5), 1052–1069.
Auerbach, A. J. (1979). Wealth maximization and the cost of capital. The Quarterly Journal of Economics, 93(3), 433–446.
Auerbach, A. J. (1983). Taxes, corporate financial policy and the cost of capital. Journal of Economic Literature, 21, 905–940.
Awerbuch, S. (1995). Market-based IRP: It’s easy!!!. The Electricity Journal, 8(3), 50–67.
Blank, L., & Mayo, J. W. (2009). Endogenous regulatory constraints and the emergence of hybrid regulation. Review of Industrial Organization, 35(3), 233–255.
Buranabunyut, N., & Peoples, J. (2012). An empirical analysis of incentive regulation and the allocation of inputs in the US telecommunications industry. Journal of Regulatory Economics, 41(2), 181–200.
Caputo, M. R., & Partovi, M. H. (2002). Reexamination of the AJ effect. Economics Bulletin, 12(10), 1–9.
Chiang, A. C. (2000). Elements of dynamic optimization. Illinois: Waveland Press Inc.
Church, J. R., & Ware, R. (2000). Industrial organization: A strategic approach. New York: McGraw-Hill.
Cohen, D., & Hassett, K. A. (1997). Inflation, taxes, and the durability of capital. Division of Research & Statistics and Monetary Affairs, Federal Reserve Board.
Dew-Becker, I. (2012). Investment and the cost of capital in the cross-section: The term spread predicts the duration of investment. Cambridge: Harvard University.
El-Hodiri, M. A., & Takayama, A. (1973). Behavior of the firm under regulatory Constraint: Clarifications. American Economic Review, 63(1), 235–237.
Fellows, G. K. (2011). Negotiated Settlements with a cost of service backstop: The consequences for depreciation. Energy Policy, 39(3), 1505–1513.
Gibbons, J. C. (1984). The optimal durability of fixed capital when demand is uncertain. The Journal of Business, 57(3), 389–403.
Goolsbee, A. (2004). Taxes and the quality of capital. Journal of Public economics, 88(3), 519–543.
Jorgenson, D. W. (1972). Investment Behavior and the Production Function. The Bell Journal of Economic and Management Science, 3, 220–251.
Katz, M. L. (1983). A general analysis of the Averch–Johnson effect. Economics Letters, 11(3), 279–283.
Law, S. M. (2014). Assessing the Averch–Johnson–Wellisz effect for regulated utilities. International Journal of Economics and Finance, 6(8), 41–67.
Lettau, M., & Wachter, J. A. (2007). Why is long-horizon equity less risky? A duration-based explanation of the value premium. The Journal of Finance, 62(1), 55–92.
Meyer, R. A. (1979). Regulated monopoly under uncertainty. Southern Economic Journal, 45, 1121–1129.
Modigliani, F., & Miller, M. H. (1958). The cost of capital, corporation finance and the theory of investment. American Economic Review, 48(3), 261–297.
National Energy Board of Canada (1995). RH-2-94, reasons for decisions, multi-pipeline (Cost of Capital). http://publications.gc.ca/collections/Collection/NE22-1-1995-1E.
Pressman, I., & Carol, A. (1971). Behaviour of the firm under regulatory constraint: Note. American Economic Review, 61(1), 210–212.
Pressman, I., & Carol, A. (1973). Behaviour of the firm under regulatory constraint: Reply. American Economic Review, 63(1), 238.
Rogerson, W. P. (1992). Optimal depreciation schedules for regulated utilities. Journal of Regulatory Economics, 4(1), 5–33.
Sanyal, P., & Bulan, L. T. (2011). Regulatory risk, market uncertainties, and firm financing choices: Evidence from US Electricity Market Restructuring. The Quarterly Review of Economics and Finance, 51(3), 248–268.
Schroeder, D., Jr. (2010). A tutorial on pipe flow equation. PSIG Annual Meeting.
Spiegel, Y., & Spulber, D. F. (1997). Capital structure with countervailing incentives. The RAND Journal of Economics, 2(1), 1–24.
Takayama, A. (1969). Behavior of the firm under regulatory constraint. American Economic Review, 59(3), 255–260.
Westcoast Energy Inc’s (2003). Transmission depreciation study. https://www.neb-one.gc.ca/ll-eng/livelink.exe/fetch/2000/90465/92833/92844/586981/305270/310870/304770/A0J4S6_-_Tab_1_Depreciation_Study?nodeid=304786.
Acknowledgments
I offer thanks to two anonymous referees whose attention and comments greatly improved my understanding and communication of the ideas herein. I am also very grateful for the continued support and assistance of my doctoral supervisor Aidan Hollis. Additional thanks to Jeffrey Church, Robert Mansell, Gordon Sick, Irving Rosales Arredondo and Mike Ata for helpful comments and thanks to Daniel Bieber for his assistance on footnote 7. All errors are my own.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1: Dynamics of the system
I present here a sketch for the proof of dynamic stability (convergence to the steady state) for the optimal control problem described by the Hamiltonian given in Eq. (5). I begin this sketch with an illustration that the system is stable in a subspace comprised of the state variables. I then illustrate that, for each asset class (\(i \in N\)), the ratio of the liability (\(B_i\)) to the productive value (\(k_i\)) of capital is known. Finally I provide a simple phase diagram in \(k_i\), \(B_i\) space illustrating that the system is stable around the optimal steady state equilibrium.
For each element in set \(N\) (the total set of distinct asset classes) there are 3 accompanying dynamic variables: \(I_{i,t}\), \(k_{i,t}\) and \(B_{i,t}\). Consider the lower dimension \(2N\) subset of the dynamic system composed of Eqs. (3) and (4). The system can be written in the standard matrix form (\(\dot{X} = Ax + c\)):
The set of eigenvalues for this subspace can be characterized by the set of values \(\varLambda \) which satisfyFootnote 31:
Thus the set of eigenvalues for the subspace dimension is uniformly negative. The system is therefore stable in both of the state variables for each asset class via the standard stability conditions. For any given level of the control variables \(I_i\) the state variables \(k_i\) and \(B_i\) will converge to a steady state.
The ratio between \(k_i\) and \(B_i\) in the steady state is fixed and can be derived for any given level of investment. Consider a case in which investment in an asset class \(i\) is fixed through time at a value \(\bar{I}\). In this situation, \(k_i\) and \(B_i\) will each converge to a steady state where:
Thus, a feasible path to the steady state exists. Full characterization of this path implies that the firm will choose levels for the control variables \(I_{i,t}\) which will ensure that all \(k_i\) and \(B_i\) converge to the optimal steady state (\(\widehat{k}_i , \widehat{B}_i\)) following the maximization problem outlined in the main paper. At the optimal steady state \(I_i = \delta _i \widehat{k}_i = \alpha _i \widehat{B_i} \; \implies \; \dot{I}_i = 0\).
The phase diagram for the 2 dimensional subset \(k_i\), \(B_i\) of the system around the steady state (i.e. when \(I_i =\delta _i \widehat{k}_i = \alpha _i \widehat{B_i}\)) is given in Fig. 2.
Appendix 2: Mathematical appendix
Proof (Proof of Lemma 1)
Arrow’s sufficiency conditions for the existence of an equilibrium requires the Hamiltonian function to be jointly concave with respect to the state variables. Joint concavity of \(k_i\) and \(k_j\) requires that:
(which is satisfied for any input \(k_i\) and function H) and
Where \(b_1\) and \(b_2\) are the first and second principle minors of the bordered Hessian of (5). \(\frac{\partial F}{\partial k_i} >0 \;\forall i \in N\) and the set of equations given in (10) imply: \(sign \left\{ {\frac{\partial H}{\partial k_i}} \right\} \equiv sign \left\{ \frac{\partial H}{\partial k_j}\right\} \;\; \forall i,j \in N\). Thus: \(\frac{\partial H}{\partial k_i}\cdot \frac{\partial H}{\partial k_j} >0 \; \forall i,j \in N\). Imposing \( \left\{ \frac{\partial ^2 F}{\partial k_i ^2} < 0 \; ; \; \frac{\partial ^2 F}{\partial k_{i,t} \partial k_{j,t} } \ge 0 \right\} \;\forall \;i \in N \), the above relationship implies \(\lambda < 1\). A binding rate of return constraint implies \(\lambda > 0\) via the standard Karesh-Kuhn-Tucker conditions. Therefore \(\lambda \in (0,1)\). \(\square \)
Proof (Proof of Lemma 2)
From Lemma 1: \(\lambda \in (0,1)\). Thus:
-
If \(S>r_i\) : \( \widehat{\mu _i} > 0 \implies \mu _i ^* > 0\), it follows that \( \widehat{k_i} = 0 \; \implies \; k_i ^* = 0\).
-
If \(S<r_i\) : \(\mu _i ^* > 0 \implies \widehat{\mu _i} > 0 \), it follows that \(k_i ^* = 0 \; \implies \; \widehat{k_i} = 0\).
-
If \(S=r_i\) : \(\mu _i ^* > 0 \Leftrightarrow \widehat{\mu _i} > 0 \), it follows that \(\widehat{k_i} = 0 \Leftrightarrow k_i ^* = 0\).
\(\square \)
Proof (Proof of Lemma 3)
Via the definition of \(M\): \(k_i >0 \;\;\forall i \in M\). Imposing the condition \( \left( \frac{\partial F}{\partial k_i} \ge 0 \; \;i \in M \right) \) Eq. (13) implies: \(\lambda \le \left( \frac{r_i +\alpha _i}{S+\alpha _i} \right) \; \forall i \in M\) if and only if \(\lambda \in (0,1)\). From Lemma 1: \(\lambda \;\in (0,1)\). Define \(\overline{\lambda }\) as the largest value that satisfies \( \left( \overline{\lambda } \le \frac{r_i +\alpha _i}{S+\alpha _i} \right) \; \forall i \in M\). Then \(\lambda \in (0,\overline{\lambda })\). \(\square \)
1.1 2.1
Proof (Proof of Proposition 1)
Subtracting Eq. (14) from Eq. (13) forms a set of equalities given by:
(Recall that \(\mu _i =0 \;\; \forall i \in M\).) Taking a linear approximation of the change on the left hand side of the above set of equalities, the set can be given in matrix notation as: \(\mathcal {H} K_{\varDelta } = \mathcal {C} \) where \(\mathcal {H}\) is the \(MxM\) Hessian matrix associated with the capital inputs \(i \in M\) of the revenue function (\(F(K,L)\)), \(K_{\varDelta }\) is an \(Mx1\) vector composed of elements \(\widehat{k_i} - k_i ^*\) and \(\mathcal {C}\) is a vector of values \((r_i - S) \left( \frac{\lambda }{1- \lambda } \right) \). As a Hessian matrix, \(\mathcal {H}\) is a symmetric Hermitian matrix. The function \(F(K,L)\) is assumed jointly concave in all capital inputs such that \(\mathcal {H}\) is a negative definite matrix and \(x^T \mathcal {H} x <0\) for any real vector x. It follows that: \(K_{\varDelta }^T \mathcal {H} K_{\varDelta } = K_{\varDelta }^T \mathcal {C} < 0 \)
Note that the row and column orderings in the Hessian matrix \(\mathcal {H}\) are arbitrary so long as symmetry is maintained. As a negative definite matrix, all upper left symmetric sub-matrices of \(\mathcal {H}\) are themselves negative definite. Therefore the above equality holds for any sub-matrix of \(\mathcal {H}\) and the inequality set implies that:
If an equilibrium exists, then via Lemma 1: \(\lambda \in (0,1)\), thus:
To this point the proof is established for capital inputs \(i \in M\). For the set \( \left\{ i \in N | i \notin M \right\} \); following directly from Lemma 2 and the definition of the set \(N\):
Thus the set of inequalities holds for all \(i \in N\):
\(\square \)
1.2 2.2
Proof (Proof of Proposition 2)
From Lemma 3, if an equilibrium exists: \(\lambda \in (0, \overline{\lambda })\).
Substituting Eqs. (15) and (16) for the left and right hand side of the above inequality respectively, the inequality can be rewritten as: \( \left\{ \widehat{\left( \frac{\partial k_j}{\partial k_i} \right) } > \left( \frac{\partial k_j}{\partial k_i} \right) ^* \right\} \forall i \ne j \in M \) (recall that \(\widehat{\mu _i} = \mu _i ^* = 0 \) if and only if \(i \in M\)). Imposing declining marginal revenue product for both inputs:
Following directly from Lemma 2 and the definition of the set \(N\):
although the fraction \(\frac{k_j^*}{k_i^*}\) is undefined \(\forall \left\{ i,j \in N | i,j \notin M \right\} \) Therefore, \(S>r_i>r_j\) and \(\alpha _i = \alpha _j \; \implies \;\left\{ \frac{k_j^*}{k_i^*} < \frac{\widehat{k_j}}{\widehat{k_i}} \right\} \forall i \ne j \in N\) if and only if \(k_i ^* + k_j ^* >0\) . \(\square \)
1.3 2.3
Proof (Proof of Proposition 3)
From Lemma 3, if an equilibrium exists \(\lambda \in (0, \overline{\lambda })\):
Substituting Eqs. (15) and (16) for the left and right hand side of the above inequality respectively, the inequality can be rewritten as: \(\left\{ \widehat{\left( \frac{\partial k_j}{\partial k_i} \right) } < \left( \frac{\partial k_j}{\partial k_i} \right) ^* \right\} \forall i \ne j \in M\). Imposing declining marginal revenue product for both inputs:
From Lemma 2 if \(S=r_i\) then \(i \in M\) by definition. By extension, the only set of elements \(i\) that satisfies \(S=r_i\) and \(i \in N | i \notin M\) is the null set. Therefore: \(r = r_i = r_j\) and \( \alpha _i < \alpha _j \; \implies \; \; \left\{ \frac{\widehat{k_i}}{\widehat{k_j}} > \frac{k_i^*}{k_j^*} \right\} \forall i \ne j \in N\). \(\square \)
1.4 2.4
Proof (Proof of Proposition 4)
From Lemma 1, if an equilibrium exists \(\lambda \in (0, \overline{\lambda })\):
Substituting Eqs. (15) and (16) for the left and right hand side of the above inequality respectively, the inequality can be rewritten as:\( \left\{ \widehat{\left( \frac{\partial k_j}{\partial k_i} \right) }> \left( \frac{\partial k_j}{\partial k_i} \right) ^* \right\} \forall i \ne j \in M\). Imposing declining marginal revenue product for both inputs:
The alternative effect; \((r_j + \alpha _j) - (r_i + \alpha _i) < (r_i \alpha _j - r_j \alpha _i) / S \;\; \implies \;\; \frac{\widehat{k_i}}{\widehat{k_j}} > \frac{k_i^*}{k_j^*} \;\; \forall i \ne j \in M\) follows from a parallel proof wherein the direction of the inequities is reversed. The null effect; \((r_j + \alpha _j) - (r_i + \alpha _i) = (r_i \alpha _j - r_j \alpha _i) / S \;\; \implies \;\; \frac{\widehat{k_i}}{\widehat{k_j}} = \frac{k_i^*}{k_j^*} \;\; \forall i \ne j \in M\) follows from a parallel proof wherein an equality is substituted for the inequalities. Via Lemma 2, using the same logic as in the preceding proofs, these results can be generalized to elements \(i \ne j \in N\) unless \(k_i ^* = k_j ^* = 0\). \(\square \)
Rights and permissions
About this article
Cite this article
Fellows, G.K. The capital structure of a firm under rate of return regulation: durability and the yield curve. J Regul Econ 47, 273–299 (2015). https://doi.org/10.1007/s11149-015-9271-5
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11149-015-9271-5