Lattice surgery-based Surface Code architecture using remote logical CNOT operation

Abstract

The lattice surgery approach allows for an efficient implementation of universal quantum gate sets with topological quantum error correcting codes that achieve a high threshold and are composed of only the nearest gate operations and low-weight stabilizers. Here, we propose two types of lattice surgery-based logical qubit architectures using the logical remote-controlled-not operation and circuit mapping method. Our architectures enhanced the qubit efficiency, and when combined with our qubit initialization and routing process, they reduced the running time and quantum volume of several quantum circuits by removing time-expensive logical SWAP operations and enabling fast logical CNOT operations. The quantum volume was compared between three cases, one in which the magic state distillation technique was not applied, one in which the multiple magic state distillation circuits are used to reduce the circuit execution time, and the other in which one magic state distillation circuit are used to reduce the number of qubit used.

Appendix A: Logical CNOT operation in r-arch

Fig. 16

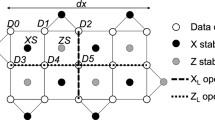
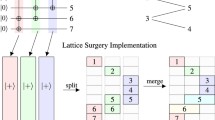
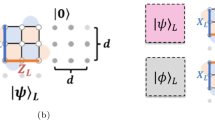
CNOT operation for the control and target logical qubits in the same row. a Control qubit $\vert C \rangle _L$, the target qubit $\vert T \rangle _L$, and two intermediate qubits $\vert INT_1 \rangle _L$ and $\vert INT_2 \rangle _L$ are prepared. Intermediate qubits are in $\vert + \rangle _L$ states. Merge $\vert C \rangle _L$ and $\vert INT_1 \rangle _L$. Merge $\vert T \rangle _L$ and $\vert INT_2 \rangle _L$ at the same time. b $\vert C_1 \rangle _L$ and $\vert T_1 \rangle _L$ is the merged state. Split $\vert C_1 \rangle _L$ by X measurements on green colored qubits c $\vert C_2 \rangle _L$ and $\vert INT_1' \rangle _L$ are the split states. Merge $\vert INT_1' \rangle _L$ and $\vert T_2 \rangle _L$. d $\vert T_3 \rangle _L$ is the merged state. X measurement on green-colored qubits and Z measurements on red-colored qubits to reduce the logical qubit size. e State after the physical X and Z measurement $\vert T_4 \rangle _L$. Prepare a red physical qubit in the $\vert + \rangle$ state and perform stabilizer measurements. f The stabilizer changes to $\vert T_5 \rangle _L$. Perform X measurements on green-colored qubits to shrink the qubit size g State after CNOT operation. Steps (d)–(f) steps can be performed over d cycles (Color figure online)

In Sect. 3.2, we briefly explain the CNOT operation when the control and target qubits are in the same row. This appendix exhibits how this CNOT operation is performed. The logical states in Fig. 16 are transformed as follows.

The control logical qubit is denoted as $\vert C \rangle _L = \alpha \vert 0 \rangle _L+\beta \vert 1 \rangle _L$, the target logical qubit as $\vert T \rangle _L = a\vert 0 \rangle _L+b \vert 1 \rangle _L$, and the two intermediate logical qubits as $\vert INT_1 \rangle _L$ and $\vert INT_2 \rangle _L$. Intermediate qubits were prepared in $\vert + \rangle _L$ states (Fig. 16a).

From Eq. 2, the post-merged states in Fig. 16b are

$$\begin{aligned} \begin{aligned} \vert C_2 \rangle _L&= {{\alpha +\beta } \over \sqrt{2}} \vert + \rangle _L+(-1)^{m_1}{{\alpha -\beta } \over \sqrt{2}} \vert - \rangle _L&= X_{L_1}^{m_1}(\alpha \vert 0 \rangle _L + \beta \vert 1 \rangle _L)\\ \vert T_2 \rangle _L&= {{a+b} \over \sqrt{2}}\vert + \rangle _L+(-1)^{m_2}{{a-b} \over \sqrt{2}}\vert - \rangle _L&=X_{L_2}^{m_2}(a \vert 0 \rangle _L + b \vert 1 \rangle _L) \end{aligned} \end{aligned}$$

where $X_{L_1} (X_{L_2})$ is equal to $X_C X_{I_1} (X_T X_{I_2})$ and $m_1$ and $m_2$ are the merge measurement outcomes.

From Eq. 3, the state remained after $M_x$ on green qubits in Fig. 16c becomes

$$\begin{aligned} \begin{aligned} \vert C_3 \; INT_1' \rangle _L = X_{L_1}^{m_1}(\alpha \vert 00 \rangle _L + \beta \vert 11 \rangle _L) \end{aligned} \end{aligned}$$

In Fig. 16d, the post z-boundary merging state is

$$\begin{aligned} \begin{aligned} \vert C_3 \; T_4 \rangle _L&= X_{L_1}^{m_1}X_{L_2}^{m_2}[(\alpha \vert 0 \rangle _L(a\vert 0 \rangle _L+b\vert 1 \rangle _L) + (-1)^{m_3}\beta \vert 1 \rangle _L X_{L_3} (a\vert 0 \rangle _L+b\vert 1 \rangle _L)]\\&=X_{L_1}^{m_1}X_{L_2}^{m_2}Z_{L_3}^{m_3}[(\alpha \vert 0 \rangle _L(a\vert 0 \rangle _L+b\vert 1 \rangle _L) + \beta \vert 1 \rangle _L X_{L_3}L (a\vert 0 \rangle _L+b\vert 1 \rangle _L)]\\&=X_{L_1}^{m_1}X_{L_2}^{m_2}Z_{L_3}^{m_3}[\alpha \vert 0 \rangle _L\vert T \rangle _L + \beta \vert 0 \rangle _L X_T\vert T \rangle _L] \end{aligned} \end{aligned}$$

where $Z_{L_3}(X_{L_3})$ is equal to $Z_T(X_T)$ and $m_3$ is the merge measurement outcome.

After trimming, as shown in Fig. 16e–g, the target logical qubit shrinks and goes back to its original size.