Analysis on Laakso graphs with application to the structure of transportation cost spaces

Abstract

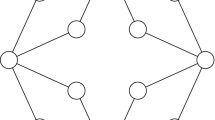
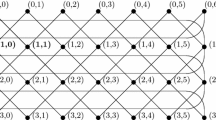
This article is a continuation of our article in Dilworth et al. (Can J Math 72:774–804, 2020). We construct orthogonal bases of the cycle and cut spaces of the Laakso graph $\mathcal {L}_n$. They are used to analyze projections from the edge space onto the cycle space and to obtain reasonably sharp estimates of the projection constant of ${\text {Lip}}_0(\mathcal {L}_n)$, the space of Lipschitz functions on $\mathcal {L}_n$. We deduce that the Banach–Mazur distance from ${\mathrm{TC}}\quad (\mathcal {L}_n)$, the transportation cost space of $\mathcal {L}_n$, to $\ell _1^N$ of the same dimension is at least $(3n-5)/8$, which is the analogue of a result from [op. cit.] for the diamond graph $D_n$. We calculate the exact projection constants of ${\text {Lip}}_0(D_{n,k})$, where $D_{n,k}$ is the diamond graph of branching k. We also provide simple examples of finite metric spaces, transportation cost spaces on which contain $\ell _\infty ^3$ and $\ell _\infty ^4$ isometrically.