The determinant representation of Ward soliton solutions and its dynamical behaviors

Abstract

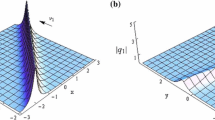
In this work, we constructed the multi-soliton solutions to the Ward equation with the help of the generalized Darboux transformation method. Importantly, we obtained the determinant representation of the Ward multi-soliton solutions with trivial and nontrivial scattering. All calculations indicate that the Darboux transformation method is an effective and straightforward way to generate the Ward solutions. These obtained determinantal solutions are particularly useful for analyzing the dynamical behaviors of the energy density functions of the Ward multi-soliton solutions. By investigating the energy density figures, we illustrated the nontrivial scattering properties of the Ward multi-soliton solutions. These results are meaningful for quantum field theory and could be utilized to provide an intuitive description of the particle interactions.

Appendices

Appendix A. Proof of Theorem 1

Proof

According to the Lax pair of the Ward equation, we can obtain that $\textbf{U}=\left[ (\lambda \partial _x-\partial _u) {\pmb \Psi }\right] {\pmb \Psi }^{-1}$. Assuming that $\textbf{U}$ is independent of $\lambda$. Set the Darboux transformation as ${\pmb \Psi }_{1}=\textbf{T}_1{\pmb \Psi }$, we hope that ${\pmb \Psi }_{1}$ satisfies the same equation as system (5). Based on the system (5), we have $\textbf{T}_1[(\lambda \partial _x-\partial _u) {\pmb \Psi }]=\textbf{T}_1\textbf{U}{\pmb \Psi }$, which can be used to derive the following formulas

$$\begin{aligned} \begin{aligned}&\textbf{T}_1[(\lambda \partial _x-\partial _u) {\pmb \Psi }]=\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}{} \textbf{T}_1{\pmb \Psi },\\&\textbf{T}_1[(\lambda \partial _x-\partial _u) {\pmb \Psi }]+[(\lambda \partial _x-\partial _u)\textbf{T}_1]{\pmb \Psi }\\&\quad =\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}{} \textbf{T}_1{\pmb \Psi }+[(\lambda \partial _x-\partial _u)\textbf{T}_1]{\pmb \Psi },\\&(\lambda \partial _x-\partial _u)\textbf{T}_1{\pmb \Psi }\\&\quad =\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}{\pmb \Psi }_1+[(\lambda \partial _x\textbf{T}_1-\partial _u\textbf{T}_1)\textbf{T}_1^{-1}]\textbf{T}_1{\pmb \Psi },\\&(\lambda \partial _x-\partial _u){\pmb \Psi }_1\\&\quad =\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}{\pmb \Psi }_1+[(\lambda \partial _x\textbf{T}_1-\partial _u\textbf{T}_1)\textbf{T}_1^{-1}]\textbf{T}_1{\pmb \Psi },\\&(\lambda \partial _x-\partial _u){\pmb \Psi }_1\\&\quad =[\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}+(\lambda \partial _x\textbf{T}_1-\partial _u\textbf{T}_1)\textbf{T}_1^{-1}]{\pmb \Psi }_1. \end{aligned} \end{aligned}$$

Take $\textbf{U}_1=\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}+(\lambda \partial _x \textbf{T}_{1}-\partial _u \textbf{T}_{1})\textbf{T}_1^{-1}$, then ${\pmb \Psi }_{1}$ and $\textbf{U}_1$ satisfy the new system (11). Next, we need to prove that $\textbf{U}_1$ is independent of $\lambda$.

According to the form of $\textbf{T}_1$ and $\textbf{T}_1^{-1}$, we know that $\textbf{U}_1$ may have singularities at $\lambda ={\lambda _1}^*$ and $\lambda ={\lambda _1}$, the residue of $\textbf{U}_1$ at $\lambda =\lambda _1$ and $\lambda ={\lambda _1}^*$ should be 0, which is equivalent to

$$\begin{aligned} \underset{\lambda =\lambda _1}{\text {Res}}{} \textbf{U}_1 =&-({\lambda _1}^*-\lambda _1)\Big [-\lambda _1({\mathbb {I}}-\textbf{P}_1)\textbf{P}_{1,x}\\&+({\mathbb {I}}-\textbf{P}_1) \textbf{P}_{1,u}+({\mathbb {I}}-\textbf{P}_1)\textbf{U}(\lambda _1)\textbf{P}_1\Big ]\\ =&({\lambda _1}^*-\lambda _1)\left( {\mathbb {I}}-\frac{ |\textbf{y}_1\rangle \langle \textbf{y}_1|}{ \langle \textbf{y}_1 \mid \textbf{y}_1 \rangle }\right) \\&\Big [\lambda _1 |\textbf{y}_1\rangle \left( \frac{ \langle \textbf{y}_1|}{ \textbf{y}_1 \mid \textbf{y}_1 \rangle }\right) _x\\&- |\textbf{y}_1\rangle \left( \frac{ \langle \textbf{y}_1|}{\textbf{y}_1 \mid \textbf{y}_1 \rangle }\right) _u\Big ] =0,\\ \underset{\lambda =\lambda _1^*}{\text {Res}}{} \textbf{U}_1 =&-({\lambda _1}-\lambda _1^*)\big [\lambda _1^*\textbf{P}_{1,x}-\textbf{P}_{1,u}\\&+\textbf{P}_1\textbf{U}(\lambda _1^*)\Big ]({\mathbb {I}}-\textbf{P}_1)\\ =&-({\lambda _1}^*-\lambda _1)\left( {\mathbb {I}}-\frac{ |\textbf{y}_1\rangle \langle \textbf{y}_1|}{ \langle \textbf{y}_1 \mid \textbf{y}_1 \rangle }\right) \\&\left[ \lambda _1^*\left( \frac{ \langle \textbf{y}_1|}{ \textbf{y}_1 \mid \textbf{y}_1 \rangle }\right) _x |\textbf{y}_1\rangle -\left( \frac{ \langle \textbf{y}_1|}{ \textbf{y}_1 \mid \textbf{y}_1 \rangle }\right) _u |\textbf{y}_1\rangle \right] \\ =&0. \end{aligned}$$

Then we consider the asymptotics as $\lambda \rightarrow \infty$. Since $\textbf{U}$ is independent of $\lambda$, we get $\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}$ is independent of $\lambda$. For $\lambda \rightarrow \infty$, by taking the limit of $(\lambda \partial _x \textbf{T}_{1}-\partial _u \textbf{T}_{1})\textbf{T}_1^{-1}$, we obtain that ${\textbf{U}}_1={\mathcal {O}}(\lambda ^{-1})$ as $\lambda \rightarrow \infty$. So $\textbf{U}_1$ is holomorphic at $\lambda \in {\mathbb {C}} \cup \lbrace \infty \rbrace$. By Liouville theorem, we can get $\textbf{U}_1$ is independent of $\lambda$. Similarly, we can prove that $\textbf{V}_1=(\lambda \partial _v \textbf{T}_{1}-\partial _x \textbf{T}_{1})\textbf{T}_1^{-1}+\textbf{T}_1\textbf{V}{} \textbf{T}_1^{-1}$ is independent of $\lambda$.

Finally, since $\textbf{U},\textbf{V} \in \textbf{u}(n)$, so $\textbf{U},\textbf{V}$ have the following symmetry,

$$\begin{aligned} \textbf{U}^{\dagger }(x,y,t;\lambda ^*)= & {} -\textbf{U}(x,y,t;\lambda ), \\ \quad \textbf{V}^{\dagger }(x,y,t;\lambda ^*)= & {} -\textbf{V}(x,y,t;\lambda ). \end{aligned}$$

We need to prove that $\textbf{U}_1$ and $\textbf{V}_1$ have the same symmetry as $\textbf{U}$ and $\textbf{V}$. For $\textbf{U}_1=\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}+(\lambda \partial _x \textbf{T}_{1}-\partial _u \textbf{T}_{1})\textbf{T}_1^{-1}$, we have

$$\begin{aligned} \begin{aligned} \textbf{U}_1^{\dagger }(\lambda ^*)&=\left[ \textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}+(\lambda \partial _x \textbf{T}_{1}-\partial _u \textbf{T}_{1})\textbf{T}_1^{-1}\right] ^{\dagger }\\&=[\textbf{T}_1^{-1}]^{\dagger }{} \textbf{U}^{\dagger }{} \textbf{T}_1^{\dagger }+(\lambda \textbf{T}_{1,x}{} \textbf{T}_1^{-1})^{\dagger }-(\textbf{T}_{1,u}{} \textbf{T}_1^{-1})^{\dagger }\\&=\textbf{T}_1\textbf{U}^{\dagger }{} \textbf{T}_1^{-1}+\lambda \textbf{T}_{1}(\textbf{T}_1^{-1})_x-\textbf{T}_{1}(\textbf{T}_1^{-1})_u\\&=-\textbf{T}_1\textbf{U}{} \textbf{T}_1^{-1}-\lambda \textbf{T}_{1,x}{} \textbf{T}_1^{-1}-\textbf{T}_{1,u}{} \textbf{T}_1^{-1}\\&=-\textbf{U}_1(\lambda ). \end{aligned} \end{aligned}$$

Then we have $\textbf{U}_1 \in \textbf{u}(n)$. Similarly, we get that $\textbf{V}_1 \in \textbf{u}(n)$. Therefore, it is proved that $\textbf{U}_1, \textbf{V}_1$ share the same form and properties with $\textbf{U}, \textbf{V}$, respectively. Hence, we verify that Darboux transformation ${\pmb \Psi }_{1}=\textbf{T}_1{\pmb \Psi }$ is a gauge transformation that can be used to construct exact solutions. $\square$

Appendix B. Proof of Theorem 2

Proof

According to the Theorem 1, we can write the n-fold Darboux matrix as:

$$\begin{aligned} \textbf{T}^{[n]}(x,y,t;\lambda )= & {} \textbf{T}_{n}(x,y,t;\lambda )\textbf{T}_{n-1}(x,y,t;\lambda )\\{} & {} \quad \cdots \textbf{T}_{2}(x,y,t;\lambda )\textbf{T}_{1}(x,y,t;\lambda ). \end{aligned}$$

It is obvious that $\textbf{T}^{[n]}(x,y,t;\lambda )$ has singularities at $\lambda ={\lambda _i}^*$, so $\textbf{T}^{[n]}(x,y,t;\lambda )$ can be rewritten as the following form,

$$\begin{aligned} \textbf{T}^{[n]}(x,y,t;\lambda )= {\mathbb {I}}+\sum \limits _{i=1}^{n}\frac{\textbf{A}_i(x,y,t)}{\lambda -\lambda _i^*}, \end{aligned}$$

where rank$(\textbf{A}_i(x,y,t))=1$ which can be derived from the Theorem 1. Then we can assume $\textbf{A}_i(x,y,t)= |\textbf{x}_i\rangle \langle \textbf{y}_i|$, where $|\textbf{x}_i\rangle$ is a column vector, $\langle \textbf{y}_i|$ is a row vector.

Considering the $\textbf{U}(n)$-real condition $\textbf{T}^{[n]}(x,y,t;\lambda )[\textbf{T}^{[n]}(x,y,t;{\lambda }^*)]^{\dagger }={\mathbb {I}}$ and its Taylor expansion at $\lambda =\lambda _j$, then we obtain

$$\begin{aligned} \left( {\mathbb {I}}+\sum \limits _{i=1}^{n}\frac{|\textbf{x}_i\rangle \langle \textbf{y}_i|}{\lambda _j-{\lambda _i}^*}\right) |\textbf{y}_j\rangle =0,\,\, |\textbf{y}_j\rangle ={\pmb \Psi }(x,y,t;\lambda _j){\textbf{q}}_{j}. \end{aligned}$$

Based on Ker$(\textbf{T}^{[n]}(x,y,t;\lambda _j))={\pmb \Psi }(x,y,t; \lambda _j)\textbf{q}_{j}$, then we get

$$\begin{aligned} |\textbf{y}_j\rangle =\sum \limits _{i=1}^{n}\frac{\langle \textbf{y}_i| \textbf{y}_j\rangle }{{\lambda _i}^*-\lambda _j}|\textbf{x}_i\rangle , j=1,2,\cdots , n, \end{aligned}$$

which can be represented as the matrix form:

$$\begin{aligned} (|\textbf{y}_1\rangle , |\textbf{y}_2\rangle , \cdots ,| \textbf{y}_n\rangle )=(|\textbf{x}_1\rangle , |\textbf{x}_2\rangle , \cdots , |\textbf{x}_n\rangle )\textbf{M}, \end{aligned}$$

where $\textbf{M}=\left( \frac{\left\langle \textbf{y}_i \mid \textbf{y}_j\right\rangle }{\lambda _i^*-\lambda _j}\right) _{1 \le i, j \le n}.$ Therefore, we have

$$\begin{aligned} \textbf{T}^{[n]}(x,y,t;\lambda )={\mathbb {I}}+\textbf{Y}{} \textbf{M}^{-1}{} \textbf{D}^{-1}{} \textbf{Y}^{\dagger }, \end{aligned}$$

where $\textbf{D}={\text {diag}}\left( \lambda -{\lambda _1}^*, \lambda -{\lambda _2}^*, \cdots , \lambda -{\lambda _n}^*\right)$, $\textbf{Y}=\left[ \left| \textbf{y}_1\right\rangle , \left| \textbf{y}_2\right\rangle , \cdots , \left| \textbf{y}_n\right\rangle \right] .$ $\square$