Skip to main content
SpringerLink
Log in

Integrability, bilinearization, exact traveling wave solutions, lump and lump-multi-kink solutions of a \(\varvec{(3 + 1)}\)-dimensional negative-order KdV–Calogero–Bogoyavlenskii–Schiff equation

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

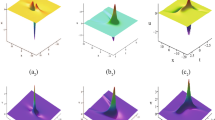
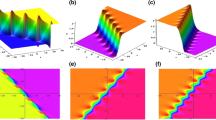
In this article, we consider a \((3+1)\)-dimensional negative-order KdV–CBS equation which represents interactions of long wave propagation dynamics with remarkable applications in the field of fluid mechanics and quantum mechanics. We investigate the integrability aspect of the considered model in the framework of Hirota bilinear differential calculus, construct infinitely many conservations laws and formulate a Lax pair. At first, we introduce the concept of Bell polynomial theory and utilize it to obtain the Hirota bilinear form. We introduce a two-field condition to determine the bilinear Bäcklund transformation. We use the Cole–Hopf transformation in bilinear Bäcklund transformation and linearize it to obtain the Lax pair formulation. The existence of infinitely many conservation laws has been checked through the Bell polynomial theory. Moreover, we derive one-kink, two-kink and three-kink soliton solution from the Hirota bilinear form. We have successfully investigated the existence of traveling wave solution for the \((3+1)\)-dimensional negative-order KDV–CBS equation and the conditions for the existence of the solution are reported. The traveling wave solutions are extracted in the form of incomplete elliptic integral of second kind and Jacobi elliptic function. Particularly, the use of long wave limit yields kink soliton solutions. Furthermore, we exhibit necessary and sufficient condition for extracting lump solutions of \((3+1)\)-dimensional nonlinear evolution equations, which have few particular types of Hirota bilinear form. The lump solutions are exploited by means of well-known test function in the Hirota bilinear form. This method reduces the number of algebraic equations to solve in deriving lump solutions of variety of NLLEs in comparison with the previously available methods in literature. Finally, two new forms of test functions are chosen and lump-multi-kink solutions have been determined.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Data availability

All data generated or analyzed during this study are included in this article.

References

  1. Munson, B. R., Okiishi, T. H., Huebsch, W. W., Rothmayer, A.P.: Fundamentals of Fluid Mechanics, Wiley, (2013)

  2. Kubokawa, A.: Growing solitary disturbance in a baroclinic boundary current. J. Phys. Oceanogr. 19, 182–192 (1989)

    ADS  Google Scholar 

  3. Luttinger, J.M., Kohn, W.: Motion of electrons and holes in perturbed periodic fields. Phys. Rev. 97, 869 (1955)

    ADS  CAS  Google Scholar 

  4. Hasegawa, A.: Plasma Instabilities and Nonlinear Effects, Springer Science and Business Media, (2012)

  5. Agrawal, G.P.: Nonlinear Fiber Optics. Academic Press, San Diego (2006)

    Google Scholar 

  6. Gardner, C.S., Greene, J.M., Kruskal, M.D., Miura, R.M.: Method for solving the Korteweg-de Vries equation. Phys. Rev. Lett. 19, 1095 (1967)

    ADS  CAS  Google Scholar 

  7. Ablowitz, M.J., Ablowitz, M.A., Clarkson, P.A.: Solitons, Nonlinear Evolution Equations and Inverse Scattering Transform. Cambridge University Press, Cambridge (1991)

    Google Scholar 

  8. Hirota, R.: The Direct Method in Soliton Theory. Cambridge University Press, Cambridge (2004)

    Google Scholar 

  9. Matveed, V.B., Salle, M.A.: Darboux Transformation and Solitons. Springer-Verlag, Berlin (1991)

    Google Scholar 

  10. Yin, Y.H., Lü, X.: Dynamic analysis on optical pulses via modified PINNs: soliton solutions, rogue waves and parameter discovery of the CQ-NLSE. Commun. Nonlinear Sci. Numer. Simul. 126, 107441 (2023)

    MathSciNet  Google Scholar 

  11. Weiss, J., Tabor, M., Carnevale, G.: The Painlevè property for partial differential equations. J. Math. Phys. 24, 522–526 (1983)

    ADS  MathSciNet  Google Scholar 

  12. Olver, P.J.: Applications of Lie groups to Differential Equations. Springer, Berlin (2000)

    Google Scholar 

  13. Yin, Y.H., Lü, X., Ma, W.X.: Bäcklund transformation, exact solutions and diverse interaction phenomena to a \((3+1)\) -dimensional nonlinear evolution equation. Nonlinear Dyn. 108, 4181–4194 (2022)

    Google Scholar 

  14. Zhang, R.F., Li, M.C., Gan, J.Y., Li, Q., Lan, Z.Z.: Novel trial functions and rogue waves of generalized breaking soliton equation via bilinear neural network method. Chaos Solit. Fractals. 154, 111692 (2022)

    MathSciNet  Google Scholar 

  15. Zhang, R.F., Li, M.C., Albishari, M., Zheng, F.C., Lan, Z.Z.: Generalized lump solutions, classical lump solutions and rogue waves of the \((2+1)\)-dimensional Caudrey-Dodd-Gibbon-Kotera-Sawada-like equation. Appl. Math. Comput. 403, 126201 (2021)

    MathSciNet  Google Scholar 

  16. Zhang, R.F., Bilige, S.: Bilinear neural network method to obtain the exact analytical solutions of nonlinear partial differential equations and its application to p-gBKP equation. Nonlinear Dyn. 95, 3041–3048 (2019)

    Google Scholar 

  17. Zhang, R.F., Li, M.C.: Bilinear residual network method for solving the exactly explicit solutions of nonlinear evolution equations. Nonlinear Dyn. 108, 521–531 (2022)

    Google Scholar 

  18. Wazwaz, A.M.: New \((3+1)\)-dimensional Painlevè integrable fifth-order equation with third-order temporal dispersion. Nonlinear Dyn. 106, 891–897 (2021)

    Google Scholar 

  19. Wazwaz, A.M.: Painlevè integrability and lump solutions for two extended \((3+1)\)- and \((2+1)\)-dimensional Kadomtsev–Petviashvili equations. Nonlinear Dyn. 111, 3623–3632 (2023)

    Google Scholar 

  20. Salah, M., Ragb, O., Wazwaz, A.M.: Efficient discrete singular convolution differential quadrature algorithm for solitary wave solutions for higher dimensions in shallow water waves. Waves Rand. Compl. Med. (2022). https://doi.org/10.1080/17455030.2022.2136420

    Article  Google Scholar 

  21. Gilson, C., Lambert, F., Nimmo, J., Willox, R.: On the combinatorics of the Hirota D-Operators. Proc. R. Soc. Lond. A. 452, 223–234 (1996)

    ADS  MathSciNet  Google Scholar 

  22. Lambert, F., Springael, J.: Construction of Bäcklund transformations with binary Bell Polynomials. J. Phys. Soc. Japan. 66, 2211–2213 (1997)

    ADS  MathSciNet  CAS  Google Scholar 

  23. Lambert, F., Springael, J.: On a direct procedure for the disclosure of Lax pairs and Bäcklund transformations. Chaos Solitons Fractals 12, 2821–2832 (2001)

    ADS  MathSciNet  Google Scholar 

  24. Fan, E.: The integrability of nonisospectral and variable-coefficient KdV equation with binary Bell polynomials. Phys. Lett. A 52, 493 (2011)

    ADS  MathSciNet  Google Scholar 

  25. Xu, G.Q., Wazwaz, A.M.: A new \((n+1)\)-dimensional generalized Kadomtsev-Petviashvili equation: integrability characteristics and localized solutions. Nonlinear Dyn. 111, 9495–9507 (2023)

    Google Scholar 

  26. Mandal, U.K., Malik, S., Kumar, S., Das, A.: A generalized \((2+1)-\)diensional Hirota bilinear equation: integrability, solitons and invariant solutions. Nonlinear Dyn. 111, 4593–4611 (2023)

    Google Scholar 

  27. Raut, S., Barman, R., Sarkar, T.: Integrability, breather, lump and quasi-periodic waves of non-autonomous Kadomtsev-Petviashvili equation based on Bell-polynomial approach. Wave Motion 119, 103125 (2023)

    MathSciNet  Google Scholar 

  28. Fun, E., Zhang, J.: Applications of the Jacobi elliptic function method to special-type nonlinear equations. Phys. Lett. A. 305, 383–392 (2002)

    ADS  MathSciNet  Google Scholar 

  29. Ali, A.T.: New generalized Jacobi elliptic function rational expansion method. J. Comput. Appl. Math. 235, 4117–4127 (2011)

    MathSciNet  Google Scholar 

  30. Adem, A.R., Muatjetjeja, B.: Conservation laws and exact solutions for a 2D Zakharov-Kuznetsov equation. Appl. Math. Lett. 48, 109–117 (2015)

    MathSciNet  Google Scholar 

  31. Wang, M., Li, X.: Extended F-expansion method and periodic wave solutions for the generalized Zakharov equations. Phys. Lett. A. 343, 48–54 (2005)

    ADS  MathSciNet  CAS  Google Scholar 

  32. Zhang, S., Xia, T.: A generalized F-expansion method and new exact solutions of Konopelchenko–Dubrovsky equations. Appl. Math. Comput. 83, 1190–1200 (2006)

    MathSciNet  Google Scholar 

  33. Lü, X., Tian, B., Zhang, H.Q., Xu, T., Li, H.: Generalized \((2+ 1)\)-dimensional Gardner model: bilinear equations, Bäcklund transformation, Lax representation and interaction mechanisms. Nonlinear Dyn. 67, 2279–2290 (2012)

    Google Scholar 

  34. Wang, M., Li, X., Zhang, J.: The (\(G^{\prime }\)/G)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A. 372, 417–423 (2008)

    ADS  MathSciNet  CAS  Google Scholar 

  35. Cheemaa, N., Younis, M.: New and more exact traveling wave solutions to integrable (2+1) dimensional Maccari system. Nonlinear Dyn. 83, 1395–1401 (2016)

    MathSciNet  Google Scholar 

  36. Fan, E.: Extended tanh-function method and its applications to nonlinear equations. Phys. Lett. A. 277, 212–218 (2000)

    ADS  MathSciNet  CAS  Google Scholar 

  37. Kumar, D., Park, C., Tamanna, N., Paul, G.C., Osman, M.S.: Dynamics of two-mode Sawada-Kotera equation: mathematical and graphical analysis of its dual-wave solutions. Results Phys. 19, 103581 (2020)

    Google Scholar 

  38. Guner, O., Bekir, A.: The exp-function method for solving nonlinear space-time fractional differential equations in mathematical physics. J. Assoc. Arab Univ. Basic Appl. Sci. 24, 277–282 (2017)

    Google Scholar 

  39. Ma, W.X.: Matrix integrable fifth-order mKdV equations and their soliton solutions. Chin. Phys. B 32, 020201 (2023)

    ADS  Google Scholar 

  40. Ma, W.X.: Sasa-Satsuma type matrix integrable hierarchies and their Riemann-Hilbert problems and soliton solutions. Phys. D Nonlinear Phenom 446, 133672 (2023)

    MathSciNet  Google Scholar 

  41. Ma, W.X.: Soliton hierarchies and soliton solutions of type \((-\lambda ^*,-\lambda )\) reduced nonlocal nonlinear Schrödinger equations of arbitrary even order. Partial. Differ. Equ. Appl. Math. 7, 100515 (2023)

    Google Scholar 

  42. Ma, W.X.: Soliton solutions to constrained nonlocal integrable nonlinear Schrödinger hierarchies of type \((-\lambda,\lambda )\). Int. J. Geom. Methods Mod. 06, 2350098 (2023)

    Google Scholar 

  43. Ma, W.X., Zhou, Y.: Lump solutions to nonlinear partial differential equations via Hirota bilinear forms. J. Differ. Equ. 264, 2633–2659 (2018)

    ADS  MathSciNet  Google Scholar 

  44. Liu, B., Zhang, X., Wang, B., Lü, X.: Rogue waves based on the coupled nonlinear Schrödinger option pricing model with external potential. Mod. Phys. Lett. B. 36, 2250057 (2022)

    ADS  CAS  Google Scholar 

  45. Ma, W.X., Yong, X.L., Lü, X.: Soliton solutions to the B-type Kadomtsev–Petviashvili equation under general dispersion relations. Wave Motion 103, 102719 (2021)

    MathSciNet  Google Scholar 

  46. Nimmo, J.C., Freeman, N.C.: A method of obtaining the N-soliton solution of the Boussinesq equation in terms of a Wronskian. Phys. Lett. A 95, 4–6 (1983)

    ADS  MathSciNet  Google Scholar 

  47. Ma, W.X., Li, C.X., He, J.: A second Wronskian formulation of the Boussinesq equation. Nonlinear Anal Theory Methods Appl. 70, 4245–4258 (2009)

    MathSciNet  Google Scholar 

  48. Ma, W.X., You, Y.: Rational solutions of the Toda lattice equation in Casoratian form. Chaos Solitons Fractals 22, 395–406 (2004)

    ADS  MathSciNet  Google Scholar 

  49. Ma, W.X., Lee, J.H.: A transformed rational function method and exact solutions to the \((3+1)\)-dimensional Jimbo–Miwa equation. Chaos Solitons Fractals 42, 1356–1363 (2009)

    ADS  MathSciNet  Google Scholar 

  50. Ma, W.X.: Lump solutions to the Kadomtsev–Petviashvili equation. Phys. Lett. A 379, 1975–1978 (2015)

    ADS  MathSciNet  CAS  Google Scholar 

  51. Yue, Y., Huang, L., Chen, Y.: N-solitons, breathers, lumps and rogue wave solutions to a \((3+1)\)-dimensional nonlinear evolution equation. Comput. Math. with Appl. 75, 2538–2548 (2018)

    MathSciNet  Google Scholar 

  52. Chen, S.J., Lü, X.: Lump and lump-multi-kink solutions in the \((3+1)\)-dimensions. Commun. Nonlinear Sci. Numer. Simul. 109, 106103 (2022)

    MathSciNet  Google Scholar 

  53. Chen, S.J., Yin, Y.H., Lü, X.: Elastic collision between one lump wave and multiple stripe waves of nonlinear evolution equations. Commun. Nonlinear Sci. Numer. Simul. (2023). https://doi.org/10.1016/j.cnsns.2023.107205

    Article  Google Scholar 

  54. Chen, S.J., Lü, X., Yin, Y.H.: Dynamic behaviors of the lump solutions and mixed solutions to a \((2+1)\)-dimensional nonlinear model. Commun. Theor. Phys. 75, 055005 (2023)

    ADS  MathSciNet  Google Scholar 

  55. Lü, X., Chen, S.J.: Interaction solutions to nonlinear partial differential equations via Hirota bilinear forms: one-lump-multi-stripe and one-lump-multi-soliton types. Nonlinear Dyn. 103, 947–977 (2021)

    Google Scholar 

  56. Korteweg, D.J., Vries, G.D.: On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Phil. Mag. 5, 422–443 (1895)

    MathSciNet  Google Scholar 

  57. Bruzon, M.S., Gandarias, M.L., Muriel, C., Ramirez, J., Saez, S., Romero, F.R.: The Calogero–Bogoyavlenskii–Schiff equation in \((2+1)\) Dimensions. Theor. Math. Phys. 137, 1367–1377 (2003)

    MathSciNet  Google Scholar 

  58. Verosky, J.M.: Negative powers of Olver recursion operators. J. Math. Phys. 32, 1733–1736 (1991)

    ADS  MathSciNet  Google Scholar 

  59. Olver, P.J.: Evolution equations possessing infinitely many symmetries. J. Math. Phys. 18, 1212–1215 (1997)

    ADS  MathSciNet  Google Scholar 

  60. Wazwaz, A.M.: A new \((2 + 1)\)-dimensional Korteweg-de Vries equation and its extension to a new \((3 + 1)\)-dimensional Kadomtsev-Petviashvili equation. Phys. Scr. 84, 035010 (2011)

    ADS  Google Scholar 

  61. Wazwaz, A.M.: Abundant solutions of various physical features for the \((2+1)\)-dimensional modified KdV–Calogero–Bogoyavlenskii–Schiff equation. Nonlinear Dyn. 89, 1727–1732 (2017)

    MathSciNet  Google Scholar 

  62. Wazwaz, A.M.: Two new Painlevè integrable KdV-Calogero-Bogoyavlenskii-Schiff (KdV-CBS) equation and new negative-order KdV-CBS equation. Nonlinear Dyn. 104, 4311–4315 (2021)

    Google Scholar 

  63. Razaa, N., Arsheda, S., Wazwaz, A.M.: Structures of interaction between lump, breather, rogue and periodic wave solutions for new \((3 +1)\)-dimensional negative order KdV–CBS model. Phys. Lett. A 458, 128589 (2023)

    MathSciNet  Google Scholar 

  64. Gandarias, M.L., Raza, N.: Conservation laws and travelling wave solutions for a negative-order KdV–CBS equation in \((3+1)\) dimensions. Symmetry 14, 1861 (2022)

  65. Singh, S., Saha Ray, S.: Painlevè integrability and analytical solutions of variable coefficients negative order KdV–Calogero–Bogoyavlenskii–Schiff equation using auto-Bäcklund transformation. Opt. Quantum Electron. 55, 195 (2023)

    Google Scholar 

Download references

Funding

The author UKM wishes to express his gratitude to the CSIR for providing financial support in the form of an SRF scholarship, as evidenced by letter number: 09/106(0198)/2019-EMR-I. The author BK wishes to express his gratitude to the UGC for providing financial support in the form of a JRF scholarship, as evidenced by letter number: F-1/UGC/Mathematics/2022/S-529. The author AD gratefully acknowledges the financial support provided under the Scheme “Fund for Improvement of S & T Infrastructure (FIST)” of the Department of Science & Technology (DST), Government of India, as evidenced by letter number: SR/FST/MS-I/2019/42 to the Department of Mathematics, University of Kalyani. The research work of AD is also funded by SERB-DST (Govt of India), file no: EEQ/2022/000719.

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Kalyani, Kalyani, 741235, India

    Uttam Kumar Mandal, Biren Karmakar & Amiya Das

  2. Department of Mathematics, Zhejiang Normal University, Jinhua, 321004, Zhejiang, China

    Wen-Xiu Ma

  3. Department of Mathematics, King Abdulaziz University, 21589, Jeddah, Saudi Arabia

    Wen-Xiu Ma

  4. Department of Mathematics and Statistics, University of South Florida, Tampa, FL, 33620-5700, USA

    Wen-Xiu Ma

  5. Material Science Innovation and Modelling, North-West University Mafikeng Campus, Private Bag X2046, Mmabatho, 2735, South Africa

    Wen-Xiu Ma

Authors
  1. Uttam Kumar Mandal
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Biren Karmakar
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Amiya Das
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Wen-Xiu Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Amiya Das or Wen-Xiu Ma.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mandal, U.K., Karmakar, B., Das, A. et al. Integrability, bilinearization, exact traveling wave solutions, lump and lump-multi-kink solutions of a \(\varvec{(3 + 1)}\)-dimensional negative-order KdV–Calogero–Bogoyavlenskii–Schiff equation. Nonlinear Dyn 112, 4727–4748 (2024). https://doi.org/10.1007/s11071-023-09028-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-023-09028-y

Keywords

Search

Navigation