Skip to main content
SpringerLink
Log in

A reduced-order model of the three-parameter fluid viscous damper with consideration of fluid compressibility and bellows volume deformation

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

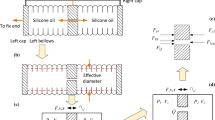
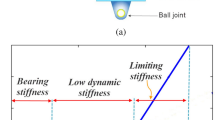
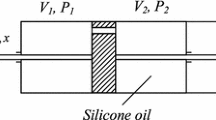
When the control moment gyroscope, momentum wheel, solar array drive mechanism, antenna drive mechanism, and refrigerator on spacecraft work, they produce micro-vibration, which seriously affects the accuracy of observation equipment. It is an effective way to suppress micro-vibration to install a vibration isolation device at the payload. In this paper, a three-parameter fluid viscous damper (TPFVD) for spacecraft micro-vibration suppression is researched. Considering the compressibility of the fluid and the volume deformation of the bellows, according to the effective area principle of bellows, the bellows is equivalent to a single tube, and a reduced-order model of the vibration isolator is established by using the approximate analytical modeling method. The nonlinearity caused by the inlet and outlet effects of the damping orifice is also considered in the model. The model error is further modified by introducing the correction coefficient of bellows volume deformation. Compared with the FEM model, the validity of the model and the modified method is verified. The results show that the models in the existing literature are applicable only when the frequency is less than 100 Hz. The calculation error of the model proposed in this paper is less than 8.20% in the frequency range of 1–300 Hz, and the maximum error of the unmodified model in the literature can reach 34.28%. In addition, the influences of damping orifice parameters, viscosity, payload mass, and nonlinear characteristics on the force transmissibility and payload displacement of the isolator are analyzed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20

Similar content being viewed by others

Data availability

Derived data supporting the findings of this study are available from the corresponding author upon reasonable request.

References

  1. Hur, G.: Isolation of micro-vibrations due to reaction wheel assembly using a source-path-receiver approach for quantitative requirements. J. Vib. Control 25(8), 1424–1435 (2019)

    Article  Google Scholar 

  2. Stabile, A.: Electromagnetic Shunt Damper for Spacecraft Micro-Vibration Mitigation. University of Surrey (United Kingdom) (2018)

  3. Davis, P., Cunningham, D., Harrell, J.: Advanced 1.5 Hz passive viscous isolation system. In: 35th Structures, Structural Dynamics, and Materials Conference (1994)

  4. Davis, L.P., Carter, D.R., Hyde, T.T.: Second-generation hybrid D-strut. In: Smart Structures and Materials 1995: Passive Damping, vol. 2445. SPIE (1995)

  5. Meng, G., et al.: Research on micro-vibration control and testing of FY-4 meteorological satellite. SCIENTIA SINICA Physica, Mechanica & Astronomica 49(2), 024508 (2018)

    Article  Google Scholar 

  6. Qin, C., et al.: Design and optimization of the micro-vibration isolation system for large space telescope. J Sound Vib. 482, 115461 (2020)

    Article  Google Scholar 

  7. Lee, D.-O., Park, G., Han, J.-H.: Experimental study on on-orbit and launch environment vibration isolation performance of a vibration damper using bellows and viscous fluid. Aerosp. Sci. Technol. 45, 1–9 (2015)

    Article  Google Scholar 

  8. Lee, D.-O., Park, G., Han, J.-H.: Hybrid isolation of micro-vibrations induced by reaction wheels. J. Sound Vib. 363, 1–17 (2016)

    Article  Google Scholar 

  9. Segel, L., Lang, H.H.: The mechanics of automotive hydraulic dampers at high stroking frequencies. In: The Dynamics of Vehicles on Roads and on Tracks, pp. 82–85. Routledge (1981)

  10. Lee, D., Taylor, D.P.: Viscous damper development and future trends. Struct. Des. Tall Build. 10(5), 311–320 (2001)

    Article  Google Scholar 

  11. Lang, H.H.: A study of the characteristics of automotive hydraulic dampers at high stroking frequencies. University of Michigan (1977)

  12. Makris, N., Constantinou, M.C.: Viscous Dampers: Testing, Modeling (1990)

  13. Makris, N., Constantinou, M.C.: Fractional-derivative Maxwell model for viscous dampers. J. Struct. Eng. 117(9), 2708–2724 (1991)

    Article  Google Scholar 

  14. San Andres Alvares, L.A.: Effect of fluid inertia on squeeze film damper force response. Diss. Texas A&M University. Libraries (1985)

  15. Tichy, J.A.: The effect of fluid inertia in squeeze film damper bearings: a heuristic and physical description. In: Turbo Expo: Power for Land, Sea, and Air, vol. 79559. American Society of Mechanical Engineers (1983)

  16. Symans, M.D., et al.: Semi-active fluid viscous dampers for seismic response control. In: First World Conference on Structural Control, vol. 3 (1994)

  17. Wilson, J.F., Davis, L.P.: Viscous damped space structure for reduced jitter. In: Proc. 58th Shock and Vibration Symp. (1987)

  18. Wang, C.X., et al.: Design and experiment of a three-parameter isolation system with optimal damping. Jixie Gongcheng Xuebao/J. Mech. Eng. 51(15), 90–96 (2015)

    Article  Google Scholar 

  19. Huang, X., et al.: Analytical stiffness model of a fluid-filled U-shaped bellows based three-parameter fluid damper for micro-vibration suppression. Aerosp. Sci. Technol. 69, 357–369 (2017)

    Article  Google Scholar 

  20. Likun, L., Gangtie, Z., Wenhu, H.: Study of liquid viscosity dampers in octo-strut platform for whole-spacecraft vibration isolation. Acta Astronaut. 58(10), 515–522 (2006)

    Article  Google Scholar 

  21. Terenzi, G.: Dynamics of SDOF systems with nonlinear viscous damping. J. Eng. Mech. 125(8), 956–963 (1999)

    Google Scholar 

  22. Goel, R.K.: Seismic response of linear and non-linear asymmetric systems with non-linear fluid viscous dampers. Earthq. Eng. Struct. Dyn. 34(7), 825–846 (2005)

    Article  Google Scholar 

  23. Bahnasy, A., Lavan, O.: Linear or nonlinear fluid viscous dampers? A seismic point of view. In: Structures Congress 2013: Bridging Your Passion with Your Profession (2013)

  24. Narkhede, D.I., Sinha, R.: Behavior of nonlinear fluid viscous dampers for control of shock vibrations. J. Sound Vib. 333(1), 80–98 (2014)

    Article  Google Scholar 

  25. Wilson, G.W., Wolke, P.J.: Performance prediction of D-Strut isolation systems.. In: Smart Structures and Materials 1997: Passive Damping and Isolation, vol. 3045. SPIE (1997)

  26. Shi, W., et al.: Modeling and dynamic properties of a four-parameter Zener model vibration damper. Shock Vib. (2016)

  27. Chen, Y., et al.: Dynamics of a passive micro-vibration damper based on a pretensioned plane cable net structure and fluid damper. J. Phys. Conf. Ser. 744(1). IOP Publishing (2016)

  28. Wang, J., Zhao, S., Wu, D.: A numerical study on the performance of nonlinear models of a micro-vibration damper. Shock Vib. (2014)

  29. Jiao, X., Zhao, Y., Ma, W.: Nonlinear dynamic characteristics of a micro-vibration fluid viscous damper. Nonlinear Dyn. 92(3), 1167–1184 (2018)

    Article  Google Scholar 

  30. Jiao, X., et al.: Research on nonlinear stiffness and damping of bellows-type fluid viscous damper. Nonlinear Dyn. 103(1), 215–237 (2021)

  31. He, L., Zheng, G.T.: Effect of viscous heating in fluid damper on the vibration isolation performance. Mech. Syst. Signal Process. 21(8), 3060–3071 (2007)

    Article  Google Scholar 

  32. Wang, J., et al.: The interior working mechanism and temperature characteristics of a fluid based micro-vibration damper. J. Sound Vib. 360, 1–16 (2016)

  33. Dennehy, C., Alvarez-Salazar, O.S.: Spacecraft micro-vibration: a survey of problems, experiences, potential solutions, and some lessons learned (2018)

  34. Expansion Joint Manufacturers Association, Inc.: Standards of the Expansion Joint Manufacturers Association, Ninth Edition (2008)

  35. Langhaar, H.L.: Steady flow in the transition length of a straight tube A55–A58 (1942)

Download references

Acknowledgements

This work was supported by the Provincial Joint Fund of Guangdong Province (No. 2020A1515110582), Natural Science Foundation of Guangdong Province (No. 2021A1515012051), and National Natural Science Funds of China (No. 62022013).

Funding

This work was funded by the Provincial Joint Fund of Guangdong Province (Grant No. 2020A1515110582), Natural Science Foundation of Guangdong Province (Grant No. 2021A1515012051), and National Natural Science Funds for Excellent Yong Scholars of China (Grant No. 62022013).

Author information

Authors and Affiliations

  1. School of Aeronautics and Astronautics, Sun Yat-Sen University, Shenzhen, 518107, China

    Xiaolei Jiao & Jinxiu Zhang

  2. Beijing Institute of Control Engineering, Beijing, 100190, China

    Wenbo Li

  3. China Aerospace Science and Technology Corporation, Beijing, 100048, China

    Jingjing Mu

Authors
  1. Xiaolei Jiao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jinxiu Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Wenbo Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jingjing Mu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wenbo Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

The expressions of the coefficient \(\lambda_{1}\) and \(\lambda_{2}\) in Eq. (18) are as follows

$$ \begin{aligned} \lambda_{1} & = \frac{{2GF_{0} }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {4\omega^{2} \left[ {m_{2} \left( {x_{3}^{2} - \omega^{2} + y_{3}^{2} } \right) + x_{3} \left( {m_{2}^{2} - \omega^{2} + n_{2}^{2} } \right)} \right]} \right\} \cdot \left( { - \omega } \right)}}{\begin{gathered} + \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$
$$ \lambda_{2} = \frac{{\left\{ {2\omega \left[ {\left( {x_{3}^{2} - \omega^{2} + y_{3}^{2} } \right)\left( {m_{2}^{2} - \omega^{2} + n_{2}^{2} } \right) - 4m_{2} x_{3} \omega^{2} } \right]} \right\} \cdot \left( {\frac{{A_{g} }}{{\rho L_{g} }}} \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } $$

The expressions of the coefficient \(\lambda_{3}\) and \(\lambda_{4}\) in Eq. (19) are as follows:

$$ \begin{aligned} \lambda_{3} & = \frac{{ - 2GF_{0} \omega }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\} \cdot \left( {\frac{{A_{g} }}{{\rho L_{g} }}} \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$
$$ \begin{aligned} \lambda_{4} & = \frac{{ - 2GF_{0} \omega }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\} \cdot \left( { - \omega } \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$

The expressions of the coefficient \(\lambda_{5}\) and \(\lambda_{6}\) in Eq. (20) are as follows:

$$ \begin{aligned} \lambda_{5} & = \frac{{2GF_{0} }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {4\omega^{2} \left[ {m_{2} \left( {x_{3}^{2} - \omega^{2} + y_{3}^{2} } \right) + x_{3} \left( {m_{2}^{2} - \omega^{2} + n_{2}^{2} } \right)} \right]} \right\} \cdot \left( { - \omega } \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$
$$ \begin{aligned} \lambda_{6} & = \frac{{2GF_{0} }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {2\omega \left[ {\left( {x_{3}^{2} - \omega^{2} + y_{3}^{2} } \right)\left( {m_{2}^{2} - \omega^{2} + n_{2}^{2} } \right) - 4m_{2} x_{3} \omega^{2} } \right]} \right\} \cdot \left( {\frac{{A_{g} }}{{\rho L_{g} }}} \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$

The expressions of the coefficient \(\lambda_{7}\) and \(\lambda_{8}\) in Eq. (21) are as follows:

$$ \begin{aligned} \lambda_{7} & = \frac{{ - 2GF_{0} \omega }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\} \cdot \left( {\frac{{A_{g} }}{{\rho L_{g} }}} \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$
$$ \begin{aligned} \lambda_{8} & = \frac{{ - 2GF_{0} \omega }}{{x_{0} }} \\ & \quad \cdot \frac{{\left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\} \cdot \left( { - \omega } \right)}}{\begin{gathered} \left\{ {2\left[ {\omega \left( {x_{1} + x_{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right) + 2m_{1} \omega \left( {x_{1} x_{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ + \left\{ {2\left[ { - 2m_{1} \omega^{2} \left( {x_{1} + x_{2} } \right) + \left( {x_{1} x_{2} - \omega^{2} } \right)\left( {m_{1}^{2} + n_{1}^{2} - \omega^{2} } \right)} \right]} \right\}^{2} \hfill \\ \end{gathered} } \\ \end{aligned} $$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jiao, X., Zhang, J., Li, W. et al. A reduced-order model of the three-parameter fluid viscous damper with consideration of fluid compressibility and bellows volume deformation. Nonlinear Dyn 111, 7027–7053 (2023). https://doi.org/10.1007/s11071-022-08211-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-08211-x

Keywords

Search

Navigation