Skip to main content
SpringerLink
Log in

Local stability and Hopf bifurcations analysis of the Muthuswamy-Chua-Ginoux system

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

A Correction to this article was published on 06 May 2022

This article has been updated

Abstract

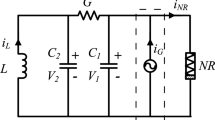
The three-dimensional Muthuswamy–Chua–Ginoux (MCG, for short) circuit system based on a thermistor is a generalization of the classical Muthuswamy–Chua circuit differential system. At present, there are only partial numerical simulations for the qualitative analysis of the MCG circuit system. In this work, we study local stability and Hopf bifurcations of the MCG circuit system depending on 8 parameters. The emerging of limit cycles under zero-Hopf bifurcation and Hopf bifurcation is investigated in detail by using the averaging method and the center manifolds theory, respectively. We provide sufficient conditions for a class of the circuit systems to have a prescribed number of limit cycles bifurcating from the zero-Hopf equilibria by making use of the third-order averaging method, as well as the methods of Gröbner basis and real solution classification from symbolic computation. Such algebraic analysis allows one to study the zero-Hopf bifurcation for any other differential system in dimension 3 or higher. After, the classical Hopf bifurcation of the circuit system is analyzed by computing the first three focus quantities near the Hopf equilibria. Some examples and numerical simulations are presented to verify the established theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Data availability

The authors declare that the manuscript has no associated data.

Change history

References

  1. Chua, L.: Memristor-the missing circuit element. IEEE Trans. Circuit Theory 18(5), 507–519 (1971)

    Article  Google Scholar 

  2. Strukov, D.B., Snider, G.S., Stewart, D.R., Williams, R.S.: The missing memristor found. Nature 453, 80–83 (2008)

    Article  Google Scholar 

  3. Deng, Y., Li, Y.: Symmetrical Hopf-induced bursting and hyperchaos control in memristor-based circuit. Chaos 31(4), 043103–13 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  4. Dong, Y., Wang, G., Iu, H.H.-C., Chen, G., Chen, L.: Coexisting hidden and self-excited attractors in a locally active memristor-based circuit. Chaos 30(10), 103123–17 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  5. Buscarino, A., Fortuna, L., Frasca, M., Gambuzza, L.V.: A chaotic circuit based on Hewlett-Packard memristor. Chaos 22(2), 023136–9 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  6. Zhao, Q., Wang, C., Zhang, X.: A universal emulator for memristor, memcapacitor, and meminductor and its chaotic circuit. Chaos 29(1), 013141–14 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  7. Pershin, Y.V., Ventra, M.: On the validity of memristor modeling in the neural network literature. Neural Netw. 121, 52–56 (2019)

    Article  Google Scholar 

  8. Rajagopal, K., Parastesh, F., Azarnoush, H., Hatef, B., Jafari, S., Berec, V.: Spiral waves in externally excited neuronal network: solvable model with a monotonically differentiable magnetic flux. Chaos 29(4), 043109–23 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  9. Chua, L.O., Kang, S.M.: Memristive devices and systems. Proc. IEEE 64(2), 209–223 (1976)

    Article  MathSciNet  Google Scholar 

  10. Ginoux, J.-M., Muthuswamy, B., Meucci, R., Euzzor, S., Garbo, A.D., Ganesan, K.: A physical memristor based muthuswamy-chua-ginoux system. Sci. Rep. 10(1), 19206–110 (2020)

    Article  Google Scholar 

  11. Jiang, J., Liang, F., Wu, W., Huang, S.: On the first Liapunov coefficient formula of 3D Lotka-Volterra equations with applications to multiplicity of limit cycles. J. Differ. Equ. 284, 183–218 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  12. Buzzi, C., Llibre, J., Santana, P.: Periodic orbits of a Hamiltonian system related with the Friedmann-Robertson-Walker system in rotating coordinates. Phys. D 413, 132673–9 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  13. Huang, J., Ruan, S., Song, J.: Bifurcations in a predator-prey system of Leslie type with generalized Holling type III functional response. J. Differ. Equ. 257(6), 1721–1752 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  14. Buzzi, C., Llibre, J., Medrado, J.: Hopf and zero-Hopf bifurcations in the Hindmarsh-Rose system. Nonlinear Dyn. 83(3), 1549–1556 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  15. Cid-Montiel, L., Llibre, J., Stoica, C.: Zero-Hopf bifurcation in a hyperchaotic Lorenz system. Nonlinear Dyn. 75(3), 561–566 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  16. Cândido, M.R., Llibre, J.: Zero-Hopf bifurcations in 3-dimensional differential systems with no equilibria. Math. Comput. Simul. 151, 54–76 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  17. Muthuswamy, B., Banerjee, S.: Introduction to Nonlinear Circuits and Networks. Springer, Berlin (2019)

    Book  Google Scholar 

  18. Buicǎ, A., Llibre, J.: Averaging methods for finding periodic orbits via brouwer degree. Bull. Sci. Math. 128(1), 7–22 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  19. Llibre, J., Novaes, D.D., Teixeira, M.A.: Higher order averaging theory for finding periodic solutions via brouwer degree. Nonlinearity 27(3), 563–583 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  20. Llibre, J., Moeckel, R., Simó, C.: Central Configuration, Periodic Oribits, and Hamiltonian Systems. Birkhäuser, Basel (2015)

    Book  Google Scholar 

  21. Browder, F.E.: Fixed point theory and nonlinear problems. Bull. Am. Math. Soc. 9(1), 1–39 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  22. Kuznetsov, Y.A.: Elements of Applied Bifurcation Theory. Springer, New York (2004)

    Book  MATH  Google Scholar 

  23. Il’yashenko, Y.S.: Finiteness Theorems for Limit Cycles. American Mathematical Society, Providence, RI (1991)

    Book  MATH  Google Scholar 

  24. Romanovski, V.G., Shafer, D.S.: The Center and Cyclicity Problems: A Computational Algebra Approach. Birkhäuser, Boston (2009)

    MATH  Google Scholar 

  25. Edneral, V.F., Mahdi, A., Romanovski, V.G., Shafer, D.S.: The center problem on a center manifold in \(\mathbb{R}^3\). Nonlinear Anal. Theory Methods Appl. 75(4), 2614–2622 (2012)

    MATH  Google Scholar 

  26. Han, M., Yu, P.: Normal Forms. Melnikov Functions and Bifurcations of Limit Cycles. Springer, London (2012)

    Book  MATH  Google Scholar 

  27. Roussarie, R.: Bifurcation of Planar Vector Fields and Hilbert’s Sixteenth Problem. Birkhäuser, Basel (1998)

    Book  MATH  Google Scholar 

  28. Liang, H.H., Torregrosa, J.: Weak-foci of high order and cyclicity. Qual. Theory Dyn. Syst. 16(2), 235–248 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  29. Shi, S.L.: A method of constructing cycles without contact around a weak focus. J. Differ. Equ. 41(3), 301–312 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  30. Euzébio, R., Llibre, J.: Zero-hopf bifurcation in a chua system. Nonlinear Anal. Real World Appl. 37, 31–40 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  31. Yang, L., Xia, B.: Real solution classification for parametric semi-algebraic systems. In: Dolzmann, A., Seidl, A., Thomas Sturm, T. (eds.) Proceedings of Algorithmic Algebra and Logic, pp. 281–289. BoD Norderstedt, Germany (2005)

    Google Scholar 

  32. Xia, B.: DISCOVERER: a tool for solving semi-algebraic systems. ACM Commun. Comput. Algebra 41(3), 102–103 (2007)

  33. Lazard, D., Rouillier, F.: Solving parametric polynomial systems. J. Symb. Comput. 42(6), 636–667 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  34. Gerhard, J., Jeffrey, D., Moroz, G.: A package for solving parametric polynomial systems. ACM Commun. Comput. Algebra 43(3/4), 61–72 (2010)

    Article  MATH  Google Scholar 

Download references

Acknowledgements

We are very grateful to the anonymous referees whose constructive comments and suggestions helped improve and clarify this paper. This research is supported by the National Natural Science Foundation of China (No.12101032, No.12131004, No.11790273 and No.11801582) and Guangdong Basic and Applied Basic Research Foundation (No.2019A1515011239).

Funding

The authors have not disclosed any funding.

Author information

Author notes

  1. Yuzhou Tian and Bo Huang contributed equally to this work.

Authors and Affiliations

  1. Department of Mathematical Sciences, Tsinghua University, Beijing, 100084, China

    Yuzhou Tian

  2. LMIB - School of Mathematical Sciences, Beihang University, Beijing, 100191, China

    Bo Huang

Authors
  1. Yuzhou Tian
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Bo Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Bo Huang.

Ethics declarations

Conflict of Interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tian, Y., Huang, B. Local stability and Hopf bifurcations analysis of the Muthuswamy-Chua-Ginoux system. Nonlinear Dyn 109, 1135–1151 (2022). https://doi.org/10.1007/s11071-022-07409-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07409-3

Keywords

Mathematics Subject Classification

Search

Navigation