Skip to main content
SpringerLink
Log in

On suppression of chaotic motion of a nonlinear MEMS oscillator

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

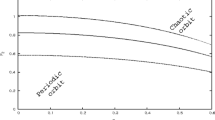
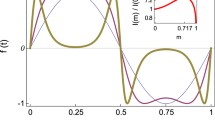
Abstract

This work investigates the behavior of the linear and nonlinear stiffness terms and damping coefficient related to the dynamics of a microelectromechanical resonator. The system is controlled by forcing it into an orbit obtained from the analytical solution of the harmonic balance method. The control techniques considered are the polynomial expansion of Chebyshev, the Picard interactive method, Lyapunov–Floquet, OLFC control, and SDRE controls. Additionally, in order to study the thermal effects, the effect of damping with fractional-order was implemented. To analyze the behavior of the system in fractional-order, the wavelet-based scale index test was carried out. In addition, the control robustness is investigated analyzing the parametric errors, and the sensitivity of the fractional derivative variation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Roukes, M.: Nanoelectromechanical systems face the future. Phys. World 14, 25 (2001)

    Google Scholar 

  2. Xie, H., Fedder, G.K.: Vertical comb-finger capacitive actuation and sensing for CMOS-MEMS. Sens. Actuator A Phys. 2, 212–221 (1995)

    Google Scholar 

  3. Younis, M.I., Nayfeh, A.H.: A study of the nonlinear response of a resonant microbeam to an electric actuation. Nonlinear Dyn. 31, 91–117 (2003)

    MATH  Google Scholar 

  4. Shaw, S.W., Balachandran, B.: A review of nonlinear dynamics of mechanical systems in year 2008. J. Syst. Des. Dyn. 2, 611–640 (2008)

    Google Scholar 

  5. Zhang, W., Baskaran, R., Turner, L.K.: Effect of cubic nonlinearity on auto-parametrically amplified resonant MEMS mass sensor. Sens. Actuator A Phys. 102, 139–150 (2002)

    Google Scholar 

  6. Tusset, A.M., Balthazar, J.M., Bassinello, D.G., Pontes Jr., B.R., Felix, J.L.P.: Statements on chaos control designs, including a fractional order dynamical system, applied to a “MEMS” comb-drive actuator. Nonlinear Dyn. 69, 1837–1857 (2012)

    MathSciNet  MATH  Google Scholar 

  7. Tusset, A.M., Janzen, F.C., Rocha, R.T., Balthazar, J.M.: On an optimal control applied in MEMS Oscillator with chaotic behavior including fractional order. Complexity 2018, 1–12 (2018)

    MATH  Google Scholar 

  8. Shao, S., Masri, K.M., Younis, M.I.: The effect of time-delayed feedback controller on an electrically actuated resonator. Nonlinear Dyn. 74, 257–270 (2013)

    MathSciNet  MATH  Google Scholar 

  9. Rhoads, J.F., Kumar, V., Shaw, S.W., Turner, K.L.: The non-linear dynamics of electromagnetically actuated microbeam resonators with purely parametric excitations. Int. J. Non-Linear Mech. 55, 79–89 (2013)

    Google Scholar 

  10. Blocher, D., Rand, R.H., Zehnder, A.T.: Analysis of laser power threshold for self oscillation in thermo-optically excited doubly supported MEMS beams. Int. J. Non-Linear Mech. 57, 10–15 (2013)

    Google Scholar 

  11. Tusset, A.M., Ribeiro, M.A., Lenz, W.B., Rocha, R.T., Balthazar, J.M.: Time delayed feedback control applied in an atomic force microscopy (AFM) model in fractional-order. J. Vib. Eng. Technol. 7, 1–9 (2019)

    Google Scholar 

  12. Jazar, G.N.: Mathematical modeling and simulation of thermal effects in flexural microcantilever resonator dynamics. J. Vib. Control 12(2), 139–163 (2006)

    MathSciNet  MATH  Google Scholar 

  13. DeMartini, B.E.: Development of nonlinear and coupled microelectromechanical oscillators for sensing applications. University of California, Santa Barbara, p. 462 (2008)

  14. Yin, Y., Sun, B., Han, F.: Self-locking avoidance and stiffness compensation of a three-axis micromachined electrostatically suspended accelerometer. Sensors 16(711), 1–116 (2016)

    Google Scholar 

  15. Guan, Y., Gao, S., Liu, H., Jin, L., Zhang, Y.: Vibration sensitivity reduction of micromachined tuning fork gyroscopes through stiffness match method with negative electrostatic spring effect. Sensors 16(1146), 1–12 (2016)

    Google Scholar 

  16. Allen, D.P., Bolívar, E., Farmer, S., Voit, W., Gregg, R.D.: Mechanical simplification of variable-stiffness actuators using dielectric elastomer transducers. Actuators 8(44), 1–19 (2019)

    Google Scholar 

  17. Benítez, R., Bolós, V.J., Ramírez, M.E.: A wavelet-based tool for studying non-periodicity. Comput. Math. Appl. 60, 634–641 (2010)

    MathSciNet  MATH  Google Scholar 

  18. Nayfeh, A.H., Mook, D.T.: Nonlinear Oscillations. Wiley, Hoboken (2008)

    MATH  Google Scholar 

  19. Nayfeh, A.H.: Perturbation Methods. Wiley, Hoboken (2008)

    Google Scholar 

  20. Nayfeh, A.H.: Introduction to Perturbation Techniques. Wiley, Hoboken (2011)

    MATH  Google Scholar 

  21. Nayfeh, A.H., Balakumar, B.: Applied Nonlinear Dynamics: Analytical, Computational, and Experimental Methods. Wiley, Hoboken (2008)

    MATH  Google Scholar 

  22. Sinha, S.C., Butcher, E.A.: Symbolic computation of fundamental solution matrices for linear time-periodic dynamical systems. J. Sound Vib. 206, 61–85 (1997)

    MATH  Google Scholar 

  23. Sinha, S.C., Joseph, P.: Control of general dynamic systems with periodically varying parameters via Lyapunov-Floquet transformation. J. Dyn. Meas. Control 116, 650–658 (1994)

    MATH  Google Scholar 

  24. David, A., Sinha, S.C.: Control of chaos in nonlinear systems with time-periodic coefficients. In: Proceedings of the American Control Conference, pp. 764–768 (2000)

  25. Peruzzi, N.J., Balthazar, J.M., Pontes, B.R., Brasil, R.M.L.R.F.: Nonlinear dynamics and control of an ideal/nonideal load transportation system with periodic coefficients. J. Comput. Nonlinear Dyn. 2, 32–39 (2007)

    Google Scholar 

  26. Peruzzi, N.J., Chavarette, F.R., Balthazar, J.M., Tusset, A.M., Perticarrari, A.L.P.M., Brasil, R.M.F.L.: The dynamic behavior of a parametrically excited time-periodic MEMS taking into account parametric errors. J. Vib. Control 22, 4101–4110 (2016)

    MathSciNet  MATH  Google Scholar 

  27. Rafikov, M., Balthazar, J.M.: On an optimal control design for Rössler system. Phys. Lett. A 333, 241–245 (2004)

    MathSciNet  MATH  Google Scholar 

  28. Pearson, J.D.: Approximation methods in optimal control. Int. J. Electron. 13, 453–469 (1962)

    Google Scholar 

  29. Wernli, A., Cook, G.: Suboptimal control for the nonlinear quadratic regulator problem. Automatica 11, 75–84 (1975)

    MathSciNet  MATH  Google Scholar 

  30. Mracek, C.P., Cloutier, J.R.: Control designs for the nonlinear benchmark problem via the state-dependent Riccati equation method. Int. J. Robust Nonlinear Control 8, 401–433 (1998)

    MathSciNet  MATH  Google Scholar 

  31. Friedland, B.: Advanced Control System Design, pp. 110–112. Prentice-Hall, Englewood Cliffs (1996)

    MATH  Google Scholar 

  32. Tusset, A.M., Bueno, A.M., Nascimento, C.B., Kaster, M.S., Balthazar, J.M.: Nonlinear state estimation and control for chaos suppression in MEMS resonator. Shock Vib. 20, 749–761 (2013)

    Google Scholar 

  33. Haghighi, H.S., Markazi, A.H.D.: Chaos prediction and control in MEMS resonators. Commun. Nonlinear Sci. Numer. Simul. 15, 3091–3099 (2010)

    Google Scholar 

  34. Sabarathinam, S., Thamilmaran, K.: Implementation of analog circuit and study of chaotic dynamics in a generalized Duffing-type MEMS resonator. Nonlinear Dyn. 87, 2345–2356 (2017)

    Google Scholar 

  35. Miandoab, E.M., Pishkenari, H.N., Yousefi-Koma, A., Tajaddodianfar, F.: Chaos prediction in MEMS-NEMS resonators. Int. J. Eng. Sci. 82, 74–83 (2014)

    Google Scholar 

  36. Miandoab, E.M., Yousefi-Koma, A., Pishkenari, H.N., Tajaddodianfar, F.: Study of nonlinear dynamics and chaos in MEMS/NEMS resonators. Commun. Nonlinear Sci. Numer. Simul. 22, 611–622 (2015)

    Google Scholar 

  37. Sinha, S.C., Henrichs, J.T., Ravindra, B.: A general approach in the design of active controllers for nonlinear systems exhibiting chaos. Int. J. Bifurc. Chaos 10, 65–178 (2000)

    MathSciNet  MATH  Google Scholar 

  38. Tusset, A.M., Piccirillo, V., Bueno, A.M., Balthazar, M.J., Danuta, S., Felix, J.L.P., Brasil, R.M.L.R.F.: Chaos control and sensitivity analysis of a double pendulum arm excited by an RLC circuit based nonlinear shaker. J. Vib. Control 22, 3621–3637 (2016)

    MathSciNet  Google Scholar 

  39. Balthazar, J.M., Bassinello, D.G., Tusset, A.M., Bueno, A.M., Pontes Jr., B.R.: Nonlinear control in an electromechanical transducer with chaotic behaviour. Meccanica 49, 1859–1867 (2014)

    MathSciNet  MATH  Google Scholar 

  40. Bechlioulis, C.P., Rovithakis, G.A.: Adaptive control with guaranteed transient and steady state tracking error bounds for strict feedback systems. Automatica 45, 532–538 (2009)

    MathSciNet  MATH  Google Scholar 

  41. Schueller, G.I.: On the treatment of uncertainties instructural mechanics and analysis. Comput. Struct. 85, 235–243 (2007)

    Google Scholar 

  42. Triguero, R.C., Murugan, S., Gallego, R., Friswell, M.I.: Robustness of optimal sensor placement under parametric uncertainty. Mech. Syst. Signal Process. 41, 268–287 (2013)

    Google Scholar 

  43. Nozaki, R., Balthazar, J.M., Tusset, A.M., Pontes, B.R., Bueno, A.M.: Nonlinear control system applied to atomic force microscope including parametric errors. Int. J. Control. Autom. Electr. Syst. 24, 223–231 (2013)

    Google Scholar 

  44. Fateme, T., Amin, F.: Size-dependent dynamic instability of double-clamped nanobeams under dispersion forces in the presence of thermal stress effects. Microsyst. Technol. 23, 3685–3699 (2017)

    Google Scholar 

  45. Jazar, R.N., Mahinfalah, M., Mahmoudian, N., Rastgaar, M.A.: Effects of nonlinearities on the steady state dynamic behavior of electric actuated microcantilever-based resonators. J. Vib. Control 15(9), 1283–1306 (2009)

    MathSciNet  MATH  Google Scholar 

  46. Younis, M.I.: Introduction to nonlinear dynamics. MEMS linear and nonlinear statics and dynamics. Microsystems, 20. Springer, Boston, MA, pp. 155–249 (2011)

  47. Wang, K., Wong, A.C., Nguyen, C.T.: VHF free-free beam high-Q micromechanical resonators. J. Microelectromech. Syst. 9, 347–360 (2000)

    Google Scholar 

  48. Tavazoei, M.S., Haeri, M.: A note on the stability of fractional order systems. Math. Comput. Simul. 79, 1566–1576 (2009)

    MathSciNet  MATH  Google Scholar 

  49. Atangana, A., Baleanu, D.: New fractional derivatives with nonlocal and non-singular kernel: theory and application to heat transfer model. Therm. Sci. 20, 763–769 (2016)

    Google Scholar 

  50. Bagley, R., Calico, R.: Fractional order state equations for the control of viscoelastically damped structures. J Guid. Control Dyn. 14, 304–11 (1991)

    Google Scholar 

  51. Yu, Y., Li, H.X., Wang, S., Yu, J.: Dynamic analysis of a fractional-order Lorenz chaotic system. Chaos Solitons Fractals 42, 1181–1189 (2009)

    MathSciNet  MATH  Google Scholar 

  52. Dorcak, L.: Numerical models for the simulation of the fractional-order control systems. arXiv preprint math/0204108 (2002)

  53. Petráš, I.: Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation. Springer, Berlin (2011)

    MATH  Google Scholar 

Download references

Acknowledgements

The authors acknowledge support from CNPQ, CAPES, FAPESP and FA, all Brazilian research funding agencies.

Author information

Authors and Affiliations

  1. Department of Electric Engineering, Federal Technological University of Paraná, UTFPR, Ponta Grossa, PR, 84016-210, Brazil

    Angelo M. Tusset, Jose M. Balthazar, Rodrigo T. Rocha, Mauricio A. Ribeiro & Wagner B. Lenz

Authors
  1. Angelo M. Tusset
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jose M. Balthazar
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Rodrigo T. Rocha
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Mauricio A. Ribeiro
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Wagner B. Lenz
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Angelo M. Tusset.

Ethics declarations

Conflict of interest

The authors declare that there is no conflict of interest regarding the publication of this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

Variables a, b, c, d,  and e for Eq. (5).

$$\begin{aligned}&-\,4a\omega ^{2}-4\alpha -9a^{2}\alpha -6b^{2}\alpha +\,4c\alpha +24a^{2}c\alpha +6b^{2}c\alpha -6c^{2}\alpha +6c^{3}\alpha +6ad\alpha \nonumber \\&\quad -\,36acd\alpha -6d^{2}\alpha +12cd^{2}\alpha +36abe\alpha -\,6e^{2}\alpha +12ce^{2}\alpha +4ak_l \nonumber \\&\quad +\,3\left( a^{3}-a^{2}d+b^{2}d-c^{2}d+2bce +\,2a\left( {b^{2}+c^{2}+d^{2}+e^{2}} \right) \right) k_{nl} =0 \end{aligned}$$
(A.1)
$$\begin{aligned}&\frac{1}{2}\left( \begin{array}{l} -8b\omega ^{2}-3b\left( {-2a\left( {-1+c} \right) +d+2cd} \right) \alpha +9b^{2}e\alpha \\ +\,\left( {2+9a^{2}-3c+3c^{2}+3d^{2}} \right) e\alpha +3e^{3}\alpha -4c\omega \mu \\ \end{array} \right) \nonumber \\&\quad +\,bk_l +\frac{3}{4}\left( 2a^{2}b+2a\left( {bd+ce} \right) +\,b\left( {b^{2}+c^{2}+2\left( {d^{2}+e^{2}} \right) } \right) \right) k_{nl} =0 \end{aligned}$$
(A.2)
$$\begin{aligned}&\begin{array}{l} \dfrac{1}{2}\left( {\begin{array}{l} -8c\omega ^{2}+4a^{3}\alpha +9c^{2}\left( {a-d} \right) \alpha -2d\alpha -9a^{2}d\alpha -3b^{2}d\alpha -3d^{3}\alpha -3be\alpha \\ -3de^{2}\alpha +3c\left( {-2a+d+2be} \right) \alpha +a\left( {2+3b^{2}+6d^{2}+6e^{2}} \right) \alpha +4b\omega \mu \\ \end{array}} \right) \\ \quad +\,ck_l +\frac{3}{4}\left( 2a^{2}c+a\left( {-2cd+2be} \right) +\,c\left( {b^{2}+c^{2}+2d^{2}+2e^{2}} \right) \right) k_{nl} =0 \\ \end{array} \end{aligned}$$
(A.3)
$$\begin{aligned}&\begin{array}{l} -\,36d\omega ^{2}+3a^{2}\alpha -3b^{2}\alpha -4c\alpha -18a^{2}c\alpha -\,6b^{2}c\alpha +3c^{2}\alpha -6c^{3}\alpha \\ \quad -\,12ad\alpha +24acd\alpha -18cd^{2}\alpha +\,12bde\alpha -6ce^{2}\alpha -12e\omega \mu +4dk_l \\ \quad +\,\left( -a^{3}+3a\left( {b^{2}-c^{2}} \right) +\,6a^{2}d+3d\left( {2b^{2}+2c^{2}+d^{2}+e^{2}} \right) \right) k_{nl} =0 \\ \end{array} \end{aligned}$$
(A.4)
$$\begin{aligned}&\begin{array}{l} \dfrac{1}{2}\left( {\begin{array}{l} 3b^{3}\alpha +b\left( {2+9a^{2}-3c+3c^{2}+3d^{2}} \right) \alpha +9be^{2}\alpha \\ -6e\left( {3\omega ^{2}+\left( {a-2ac+cd} \right) \alpha } \right) +6d\omega \mu \\ \end{array}} \right) +ek_l \\ \quad +\frac{3}{4}\left( {2abc+2a^{2}e+e\left( {2b^{2}+2c^{2}+d^{2}+e^{2}} \right) } \right) k_{nl} =0 \\ \end{array} \end{aligned}$$
(A.5)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tusset, A.M., Balthazar, J.M., Rocha, R.T. et al. On suppression of chaotic motion of a nonlinear MEMS oscillator. Nonlinear Dyn 99, 537–557 (2020). https://doi.org/10.1007/s11071-019-05421-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-019-05421-8

Keywords

Search

Navigation