Skip to main content
SpringerLink
Log in

Suppressing homoclinic chaos for a weak periodically excited non-smooth oscillator

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

A Correction to this article was published on 23 March 2022

This article has been updated

Abstract

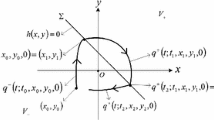
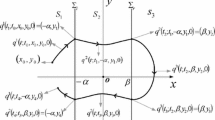
In this work, some new effective methods for suppressing homoclinic chaos in a weak periodically excited non-smooth oscillator are studied, and the main idea is to modify slightly the Melnikov function such that the zeros are eliminated. Firstly, a general form of planar piecewise-smooth oscillators is given to approximatively model many nonlinear restoring force of smooth oscillators subjected to all kinds of damping and periodic excitations. In the absence of controls, the Melnikov method for non-smooth homoclinic trajectories within the framework of a piecewise-smooth oscillator is briefly introduced without detailed derivation. This analytical tool is useful to detect the threshold of parameters for the existence of homoclinic chaos in the non-smooth oscillator. After some methods of state feedback control, self-adaptive control and parametric excitations control are, respectively, considered, sufficient criteria for suppressing homoclinic chaos are derived by employing the Melnikov function of non-smooth systems. Finally, the effectiveness of strategies for suppressing homoclinic chaos is analytically and numerically demonstrated through a specific example.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24

Similar content being viewed by others

Change history

References

  1. Budd, C.J.: Non-smooth dynamical systems and the grazing bifurcation. In: Aston, P. (ed.) Nonlinear Mathematics and its Applications. University Press, pp. 219–235 (1996)

  2. Kunze, M.: Non-smooth Dynamical Systems. Springer, Berlin (2000)

    MATH  Google Scholar 

  3. Cao, Q., Wiercigroch, M., Pavlovskaia, E.E., Grebogi, C., Thompson, J.M.T.: Archetypal oscillator for smooth and discontinuous dynamics. Phys. Rev. E 74, 046218 (2006)

    MathSciNet  MATH  Google Scholar 

  4. Bernardo, M.D., Budd, C.J., Champneys, A.R., Kowalczyk, P.: Piecewise-Smooth Dynamical Systems: Theory and Application. Springer, London (2008)

    MATH  Google Scholar 

  5. Leine, R.I., Van Campen, D.H., Van de Vrande, B.L.: Bifurcations in nonlinear discontinuous systems. Nonlinear Dyn. 23, 105–164 (2000)

    MathSciNet  MATH  Google Scholar 

  6. Feldbrugge, J., Lehners, J.L., Turok, N.: No smooth beginning for spacetime. Phys. Rev. Lett. 119, 171301 (2017)

    Google Scholar 

  7. Pei, J.S., Wright, J.P., Gay-Balmaz, F., Beck, J.L., Todd, M.D.: On choosing state variables for piecewise-smooth dynamical system simulations. Nonlinear Dyn. 95, 1–24 (2018)

    MATH  Google Scholar 

  8. Nesterov, Y.: Excessive gap technique in nonsmooth convex minimization. SIAM J. Optim. 16, 235–249 (2005)

    MathSciNet  MATH  Google Scholar 

  9. Nordmark, A.B.: Non-periodic motion caused by grazing incidence in an impact oscillator. J Sound Vib. 145, 279–297 (1991)

    Google Scholar 

  10. Popp, K., Stelter, P.: Nonlinear Oscillations of Structures Induced by Dry Friction. Nonlinear Dynamics in Engineering Systems, pp. 233–240. Springer, Berlin (1990)

    Google Scholar 

  11. Kobori, T., Takahashi, M., Nasu, T., Niwa, N., Ogasawara, K.: Seismic response controlled structure with active variable stiffness system. Earthq. Eng. Struct. Dyn. 22, 925–941 (1993)

    Google Scholar 

  12. Adler, D., Henisch, H.K., Mott, N.: The mechanism of threshold switching in amorphous alloys. Rev. Mod. Phys. 50, 209 (1978)

    Google Scholar 

  13. Mackey, M.C., Glass, L.: Oscillation and chaos in physiological control systems. Science 197, 287–289 (1977)

    MATH  Google Scholar 

  14. Hefner, A.R., Blackburn, D.L.: Simulating the dynamic electro-thermal behavior of power electronic circuits and systems. In: Proceedings 1992 IEEE Workshop on Computers in Power Electronics, pp. 143–151 (1992)

  15. Chen, G., Yu, X. (eds.): Chaos Control: Theory and Applications, vol. 292. Springer, Berlin (2003)

    MATH  Google Scholar 

  16. Ott, E., Grebogi, C., Yorke, J.A.: Controlling chaos. Phys. Rev. Lett. 64, 1196–1199 (1990)

    MathSciNet  MATH  Google Scholar 

  17. Pyragas, K.: Control of chaos via extended delay feedback. Phys. Lett. A 206, 323–330 (1995)

    MathSciNet  MATH  Google Scholar 

  18. Gritli, H., Belghith, S.: Walking dynamics of the passive compass-gait model under OGY-based control: emergence of bifurcations and chaos. Commun. Nonlinear Sci. Numer. Simul. 47, 308–327 (2017)

    MathSciNet  MATH  Google Scholar 

  19. Ling, Y., Liu, Z.R.: An improvement and proof of OGY method. Appl. Math. Mech. 19, 1–8 (1998)

    MathSciNet  MATH  Google Scholar 

  20. de Paula, A.S., Savi, M.A.: A multiparameter chaos control method based on OGY approach. Chaos Solitons Fractals 40, 1376–1390 (2009)

    MATH  Google Scholar 

  21. Parthasarathy, S., Sinha, S.: Controlling chaos in unidimensional maps using constant feedback. Phys. Rev. E 51, 6239 (1995)

    Google Scholar 

  22. Osipov, G.V., Kozlov, A.K., Shalfeev, V.D.: Impulse control of chaos in continuous systems. Phys. Lett. A 247, 119–128 (1998)

    Google Scholar 

  23. Qu, Z.L., Hu, G., Yang, G.J., Qin, G.R.: Phase effect in taming nonautonomous chaos by weak harmonic perturbations. Phys. Rev. Lett. 74, 1736C1739 (1995)

    Google Scholar 

  24. Meucci, R., Gadomski, W., Ciofini, M., Arecchi, F.T.: Experimental control of chaos by means of weak parametric perturbations. Phys. Rev. E 49, R2528 (1994)

    Google Scholar 

  25. Meucci, R., Euzzor, S., Pugliese, E., Zambrano, S., Gallas, M.R., Gallas, J.A.C.: Optimal phase-control strategy for damped-driven Duffing oscillators. Phys. Rev. Lett. 116, 044101 (2016)

    Google Scholar 

  26. Hubler, A.W.: Adaptive control of chaotic system. Helv. Phys. Acta 62, 343–346 (1989)

    Google Scholar 

  27. Braiman, Y., Goldhirsch, I.: Taming chaotic dynamics with weak periodic perturbations. Phys. Rev. Lett. 66, 2545–2548 (1991)

    MathSciNet  MATH  Google Scholar 

  28. Melnikov, V.K.: On the stability of the center for time periodic perturbations. Tans. Mosc. Math. Soc. 12, 1–57 (1963)

    MathSciNet  Google Scholar 

  29. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical System and Bifurcations of Vector Fields. Springer, New York (1983)

    MATH  Google Scholar 

  30. Wiggins, S.: Global Bifurcations and Chaos-Analytical Methods. Springer, New York (1988)

    MATH  Google Scholar 

  31. Zhang, W., Zhang, J.H., Yao, M.H.: The extended Melnikov method for non-autonomous nonlinear dynamical systems and application to multi-pulse chaotic dynamics of a buckled thin plate. Nonlinear Anal. Real World Appl. 11, 1442–1457 (2010)

    MathSciNet  MATH  Google Scholar 

  32. Yao, M.H., Zhang, W., Zu, J.W.: Multi-pulse Chaotic dynamics in non-planar motion of parametrically excited viscoelastic moving belt. J. Sound Vib. 331, 2624–2653 (2012)

    Google Scholar 

  33. Kukučka, P.: Melnikov method for discontinuous planar systems. Nonlinear Anal. Theory Methods Appl. 66, 2698–2719 (2007)

    MathSciNet  MATH  Google Scholar 

  34. Shi, L., Zou, Y., Kpper, T.: Melnikov method and detection of chaos for non-smooth systems. Acta Math. Appl. Sin. Engl. Ser. 29, 881–896 (2013)

    MathSciNet  MATH  Google Scholar 

  35. Du, Z.D., Zhang, W.N.: Melnikov method for homoclinic bifurcations in nonlinear impact oscillators. Comput. Math. Appl. 50, 445–458 (2005)

    MathSciNet  MATH  Google Scholar 

  36. Xu, W., Feng, J.Q., Rong, H.W.: Melnikov’s method for a general nonlinear vibro-impact oscillator. Nonlinear Anal. Theory Methods Appl. 71, 418–426 (2009)

    MathSciNet  MATH  Google Scholar 

  37. Granados, A., Hogan, S.J., Seara, T.M.: The Melnikov method and subharmonic orbits in a piecewise-smooth system. SIAM J. Appl. Dyn. Syst. 11, 801–830 (2012)

    MathSciNet  MATH  Google Scholar 

  38. Battelli, F., Fečkan, M.: Homoclinic trajectories in discontinuous systems. J. Dyn. Differ. Equ. 20, 337–376 (2008)

    MathSciNet  MATH  Google Scholar 

  39. Battelli, F., Fečkan, M.: Bifurcation and chaos near sliding homoclinics. J. Differ. Equ. 248, 2227–2262 (2010)

    MathSciNet  MATH  Google Scholar 

  40. Battelli, F., Fečkan, M.: Nonsmooth homoclinic orbits, Melnikov functions and chaos in discontinuous systems. Physica D 241, 1962–1975 (2012)

    MathSciNet  Google Scholar 

  41. Tian, R.L., Zhou, Y.F., Zhang, B.L., Yang, X.W.: Chaotic threshold for a class of impulsive differential system. Nonlinear Dyn. 83, 2229–2240 (2016)

    MathSciNet  MATH  Google Scholar 

  42. Li, S.B., Zhang, W., Hao, Y.X.: Melnikov-type method for a class of discontinuous planar systems and applications. Int. J. Bifurc. Chaos 24(1450022), 1–18 (2014)

    MathSciNet  Google Scholar 

  43. Li, S.B., Shen, C., Zhang, W., Hao, Y.X.: Homoclinic bifurcations and chaotic dynamics for a piecewise linear system under a periodic excitation and a viscous damping. Nonlinear Dyn. 79, 2395–2406 (2015)

    MathSciNet  MATH  Google Scholar 

  44. Li, S.B., Ma, W.S., Zhang, W., Hao, Y.X.: Melnikov method for a three-zonal planar hybrid piecewise-smooth system and application. Int. J. Bifurc. Chaos 26(1650014), 1–13 (2016)

    MathSciNet  MATH  Google Scholar 

  45. Li, S.B., Ma, W.S., Zhang, W., Hao, Y.X.: Melnikov method for a class of planar hybrid piecewise-smooth systems. Int. J. Bifurc. Chaos 26(1650030), 1–12 (2016)

    MathSciNet  MATH  Google Scholar 

  46. Li, S.B., Gong, X.J., Zhang, W., Hao, Y.X.: The Melnikov method for detecting chaotic dynamics in a planar hybrid piecewise smooth system with a switching Manifold. Nonlinear Dyn. 89, 939–953 (2017)

    MathSciNet  MATH  Google Scholar 

  47. Li, S.B., Zhao, S.B.: The analytical method of studying subharmonic periodic orbits for planar piecewise smooth systems with two switching manifolds. Int. J. Dyn. Control 7, 1–13 (2018)

    MathSciNet  Google Scholar 

  48. Lima, R., Pettini, M.: Suppression of chaos by resonant parametric perturbations. Phys. Rev. A 41, 726 (1990)

    MathSciNet  Google Scholar 

  49. Rajasekar, S.: Controlling of chaos by weak periodic perturbations in Duffing-van der Pol oscillator. Pramana 41, 295–309 (1993)

    Google Scholar 

  50. Lenci, S., Rega, G.: Optimal control of nonregular dynamics in a Duffing oscillator. Nonlinear Dyn. 33, 71C86 (2003)

    MathSciNet  MATH  Google Scholar 

  51. Andrew, Y.T.L., Liu, Z.R.: Suppressing chaos for some nonlinear oscillators. Int. J. Bifurc. Chaos 14, 1455–1465 (2004)

    MathSciNet  MATH  Google Scholar 

  52. Andrew, Y.T.L., Liu, Z.R.: Some new methods to suppress chaos for a kind of nonlinear oscillator. Int. J. Bifurc. Chaos 14, 2955–2961 (2004)

    MathSciNet  MATH  Google Scholar 

  53. Chacón, R.: Control of Homoclinic Chaos by Weak Periodic Perturbations. World Scientific, London (2005)

    MATH  Google Scholar 

  54. Yang, J., Jing, Z.: Controlling chaos in a pendulum equation with ultra-subharmonic resonances. Chaos Solitons Fractals 42, 1214–1226 (2009)

    MATH  Google Scholar 

  55. Jimenez-Triana, A., Tang, W.K.S., Chen, G., Gauthier, A.: Chaos control in Duffing system using impulsive parametric perturbations. IEEE Trans. Circuits Syst. II Express Briefs 57, 305–309 (2010)

    Google Scholar 

  56. Li, H., Liao, X., Huang, J., Chen, G., Dong, Z., Huang, T.: Diverting homoclinic chaos in a class of piecewise smooth oscillators to stable periodic orbits using small parametrical perturbations. Neurocomputing 149, 1587–1595 (2015)

    Google Scholar 

  57. Du, L., Zhao, Y.P., Lei, Y.M., Hu, J., Yue, X.: Suppression of chaos in a generalized Duffing oscillator with fractional-order deflection. Nonlinear Dyn. 92, 1921C1933 (2018)

    MATH  Google Scholar 

  58. Martínez Ovejas, P.J., Euzzor, S., Gallas, J.A.C., Meucci, R., Chacón García, R.: Identification of minimal parameters for optimal suppression of chaos in dissipative driven systems. Sci. Rep. 7, 17988 (2017)

    Google Scholar 

  59. Chacón, R., Miralles, J.J., Martínez, J.A., Balibrea, F.: Taming chaos in damped driven systems by incommensurate excitations. Commun. Nonlinear Sci. Numer. Simul. 73, 307–318 (2019)

    MathSciNet  MATH  Google Scholar 

  60. Shaw, S.W., Holmes, P.J.: A periodically forced piecewise linear oscillator. J. Sound Vib. 90, 129–155 (1983)

    MathSciNet  MATH  Google Scholar 

  61. Cao, Q.J., Wiercigroch, M., Pavlovskaia, E.E., Thompson, J.M.T., Grebogi, C.: Piecewise linear approach to an archetypal oscillator for smooth and discontinuous dynamics. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 366, 635–652 (2007)

    MathSciNet  MATH  Google Scholar 

  62. Lai, S.K., Wu, B.S., Lee, Y.Y.: Free vibration analysis of a structural system with a pair of irrational nonlinearities. Appl. Math. Model. 45, 997–1007 (2017)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors gratefully acknowledge the support of the National Natural Science Foundation of China (NNSFC) through Grant Nos. 11672326, 11802208, 11602210, U1533103.

Funding

This study was funded by the National Natural Science Foundation of China through Grant Nos. 11672326, 11802208, 11602210, U1533103.

Author information

Authors and Affiliations

  1. College of Science, Civil Aviation University of China, Tianjin, 300300, China

    Shuangbao Li & Xixi Ma

  2. School of Science, Tianjin University of Technology and Education, Tianjin, 300222, China

    Xiaoli Bian

  3. Department of Civil and Environmental Engineering, The Hong Kong Polytechnic University, Kowloon, Hong Kong, China

    Siu-Kai Lai

  4. The Hong Kong Polytechnic University Shenzhen Research Institute, Shenzhen, China

    Siu-Kai Lai

  5. College of Aeronautical Engineering, Civil Aviation University of China, Tianjin, 300300, China

    Wei Zhang

Authors
  1. Shuangbao Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xixi Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xiaoli Bian
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Siu-Kai Lai
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Wei Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Shuangbao Li or Wei Zhang.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, S., Ma, X., Bian, X. et al. Suppressing homoclinic chaos for a weak periodically excited non-smooth oscillator. Nonlinear Dyn 99, 1621–1642 (2020). https://doi.org/10.1007/s11071-019-05380-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-019-05380-0

Keywords

Search

Navigation