Skip to main content
SpringerLink
Log in

Closed-form dynamic modeling and performance analysis of an over-constrained 2PUR-PSR parallel manipulator with parasitic motions

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

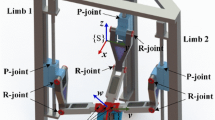
This paper presents an systematic dynamic modeling and performance analysis method of an over-constrained 2PUR-PSR parallel manipulator with parasitic motions, where P, U, S, and R represent the prismatic joint, universal joint, spherical joint, and revolute joint, respectively. The process of the deduction of the over-constrained forces/moment is given. The Newton–Euler approach and natural orthogonal complement method are adopted to establish two types of dynamic models with and without constrained forces/moments with the consideration of the over-constrained forces/moments. The dynamic manipulability ellipsoid, which measures the uniformity of changing the position and orientation of the manipulator’s moving platform, is adopted to evaluate the dynamic performance of the parallel manipulator. To show the feasibility of the proposed method, numerical simulations are conducted to investigate the dynamic models and performance of the 2PUR-PSR manipulator. The actuation forces, constrained forces/moments, and the compatible deformation are calculated. Distribution of the DME index is also obtained. The proposed modeling approach provides a fundamental basis for the structural optimization and control scheme design of the over-constrained parallel manipulator.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Kuo, Y.L., Cleghorn, W.L., Behdinan, K.: Stress-based finite element method for Euler–Bernoulli beams. Trans. Can. Soc. Mech. Eng. 30, 1–6 (2006)

    Article  Google Scholar 

  2. Dwivedy, S.K., Eberhard, P.: Dynamic analysis of flexible manipulators, a literature review. Mech. Mach. Theory 41, 749–777 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  3. Pietsch, I.T., Krefft, M., Becker, O.T., Bier, C.C., Hesselbach, J.: How to reach the dynamic limits of parallel robots? An autonomous control approach. IEEE Trans. Autom. Sci. Eng. 2, 369–380 (2005)

    Article  Google Scholar 

  4. Huang, Z., Li, Q., Ding, H.: Theory of Parallel Mechanisms. Springer, Dordrecht (2013)

    Book  Google Scholar 

  5. Lin, R., Guo, W., Gao, F.: On parasitic motion of parallel mechanisms. In: ASME 2016 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Charlotte, North Carolin, pp. 1–13 (2016)

  6. Tsai, L.W.: Robot Analysis and Design: The Mechanics of Serial and Parallel Manipulators. Wiley, New York (1999)

    Google Scholar 

  7. Dasgupta, B., Mruthyunjaya, T.S.: A Newton–Euler formulation for the inverse dynamics of the Stewart platform manipulator. Mech. Mach. Theory 33, 1135–1152 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  8. Dasgupta, B., Choudhury, P.: A general strategy based on the Newton–Euler approach for the dynamic formulation of parallel manipulators. Mech. Mach. Theory 34, 801–824 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  9. Chen, G., Yu, W., Li, Q., Wang, H.: Dynamic modeling and performance analysis of the 3-PRRU 1T2R parallel manipulator without parasitic motion. Nonlinear Dyn. 90, 339–353 (2017)

    Article  Google Scholar 

  10. Bi, Z.M., Kang, B.: An inverse dynamic model of over-constrained parallel kinematic machine based on Newton–Euler formulation. J. Dyn. Syst. Meas. Control 136, 041001 (2014)

    Article  Google Scholar 

  11. Ahmadi, M., Dehghani, M., Eghtesad, M., Khayatian, A.: Inverse dynamics of Hexa parallel robot using Lagrangian dynamics formulation. In: World Automation Congress, Hawaii, HI , pp. 1–6 (2008)

  12. Staicu, S., Zhang, D.: A novel dynamic modelling approach for parallel mechanisms analysis. Robot. Comput. Integr. Manuf. 24, 167–172 (2008)

    Article  Google Scholar 

  13. Staicu, S., Zhang, D., Rugescu, R.: Dynamic modelling of a 3-DOF parallel manipulator using recursive matrix relations. Robotica 24, 125–130 (2005)

    Article  Google Scholar 

  14. Staicu, S.: Dynamics analysis of the star parallel manipulator. Robot. Auton. Syst. 57, 1057–1064 (2009)

    Article  MATH  Google Scholar 

  15. Huang, J., Chen, Y.H., Zhong, Z.: Udwadia–Kalaba approach for parallel manipulator dynamics. J. Dyn. Syst. Meas. Control 135, 061003 (2013)

    Article  Google Scholar 

  16. Xin, G., Deng, H., Zhong, G.: Closed-form dynamics of a 3-DOF spatial parallel manipulator by combining the Lagrangian formulation with the virtual work principle. Nonlinear Dyn. 86, 1329–1347 (2016)

    Article  Google Scholar 

  17. Abdellatif, H., Heimann, B.: Computational efficient inverse dynamics of 6-DOF fully parallel manipulators by using the Lagrangian formalism. Mech. Mach. Theory 44, 192–207 (2009)

    Article  MATH  Google Scholar 

  18. Liang, D., Song, Y., Sun, T., Dong, G.: Optimum design of a novel redundantly actuated parallel manipulator with multiple actuation modes for high kinematic and dynamic performance. Nonlinear Dyn. 83, 631–658 (2016)

    Article  MathSciNet  Google Scholar 

  19. Briot, S., Arakelian, V.: On the dynamic properties of rigid-link flexible-joint parallel manipulators in the presence of type 2 singularities. J. Mech. Robot. 2, 021004–021004-6 (2010)

    Article  Google Scholar 

  20. Briot, S., Arakelian, V.: On the dynamic properties of flexible parallel manipulators in the presence of type 2 singularities. J. Mech. Robot. 3, 031009–031009-8 (2011)

    Article  Google Scholar 

  21. Kane, T.R., Levinson, D.A.: The use of Kane’s dynamical equations in robotics. Int. J. Robot. Res. 2, 3–21 (1983)

    Article  Google Scholar 

  22. Chen, Z., Kong, M., Ji, C., Liu, M.: An efficient dynamic modelling approach for high-speed planar parallel manipulator with flexible links. Proc. IMechE Part C J. Mech. Eng. Sci. 229, 663–678 (2015)

    Article  Google Scholar 

  23. Cheng, G., Shan, X.: Dynamics analysis of a parallel hip joint simulator with four degree of freedoms (3R1T). Nonlinear Dyn. 70, 2475–2486 (2012)

    Article  MathSciNet  Google Scholar 

  24. Gallardo-Alvarado, J., Rodríguez-Castro, R., Delossantos-Lara, P.J.: Kinematics and dynamics of a 4-PRUR Schönflies parallel manipulator by means of screw theory and the principle of virtual work. Mech. Mach. Theory 122, 347–360 (2018)

    Article  Google Scholar 

  25. Hu, B., Yu, J., Lu, Y.: Inverse dynamics modeling of a (3-UPU)+(3-UPS+S) serial–parallel manipulator. Robotica 34, 687–702 (2016)

    Article  Google Scholar 

  26. Li, M., Mei, J., et al.: Dynamic formulation and performance comparison of the 3-DOF modules of two reconfigurable PKM—the tricept and the trivariant. J. Mech. Des. 127, 1129–1136 (2005)

    Article  Google Scholar 

  27. Huang, T., Liu, S., Mei, J., Chetwynd, D.G.: Optimal design of a 4-DOF SCARA type parallel robot using dynamic performance indices and angular constraints. Mech. Mach. Theory 70, 246–253 (2012)

    Article  Google Scholar 

  28. Angeles, J., Lee, S.K.: The formulation of dynamical equations of holonomic mechanical systems using a natural orthogonal complement. J. Appl. Mech. 55, 243–244 (1988)

    Article  MATH  Google Scholar 

  29. Ganesh, S.S., Rao, A.B.K.: Inverse dynamics of a 3-DOF translational parallel kinematic machine. J. Mech. Sci. Technol. 29, 4583–4591 (2015)

    Article  Google Scholar 

  30. Yoshikawa, T.: Dynamic manipulability of robot manipulators. In: IEEE International Conference on Robotics and Automation, pp. 1033–1038, MO, USA, USA, St. Louis (1985)

Download references

Acknowledgements

This research was supported in part by the Natural Science Foundation of China under Grant No. 51525504, the Natural Science Foundation of Zhejiang under Grant No. LY18E050019, the Excellent Talent Cultivation Foundation under Grant No. ZSTUME02B09, and the Starting Foundation of Zhejiang Sci-Tech University under Grant No. 17022051-Y.

Author information

Authors and Affiliations

  1. Mechatronic Institute, Zhejiang Sci-Tech University, Hangzhou, 310018, Zhejiang Province, China

    Zhengsheng Chen, Lingming Xu, Weizhong Zhang & Qinchuan Li

Authors
  1. Zhengsheng Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lingming Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Weizhong Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Qinchuan Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Qinchuan Li.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$\begin{aligned}&{{\varvec{C}}}_\lambda ^O =\left( {{\begin{array}{c@{\quad }c} {\frac{{l}_3^3 {{\varvec{e}}}_3^{{\varvec{T}}} {{\varvec{R}}}_{{L,}1}^{-1} {{\varvec{L}}}_{{B}_{{1,T}} }^{{\varvec{c}}} }{3}+\frac{{l}_3^2 {{\varvec{e}}}_1^{{\varvec{T}}} {{\varvec{R}}}_{{L,}1}^{-1} {{\varvec{L}}}_{{B}_{1{,{{R}}}} }^{{\varvec{c}}} }{2}}&{} {-\frac{{l}_3^3 {{\varvec{e}}}_3^{{\varvec{T}}} {{\varvec{R}}}_{{L,}2}^{-1} {{\varvec{L}}}_{{B}_{2{,T}} }^{{\varvec{c}}} }{3}-\frac{{l}_3^2 {{\varvec{e}}}_1^{{\varvec{T}}} {{\varvec{R}}}_{{L,}2}^{-1} {{\varvec{L}}}_{{B}_{{2,{{R}}}} }^{{\varvec{c}}} }{2}} \\ {{l}_3 {{\varvec{e}}}_4^T {{\varvec{R}}}_{{L,1}}^{-1} {{\varvec{L}}}_{{B}_{{1,{{R}}}} }^{{\varvec{c}}} }&{} {{-l}_3 {{\varvec{e}}}_4^T {{\varvec{R}}}_{{L,2}}^{-1} {{\varvec{L}}}_{{B}_{{2,{{R}}}} }^{{\varvec{c}}} } \\ \end{array} }} \right) \\&{{\varvec{C}}}_{M}^{O} =\left( {{\begin{array}{c@{\quad }c} {\left[ {\frac{\left( {{l}_3 -{r}_{{L,}2,1} } \right) ^{3}}{3}+\frac{{r}_{{L,}2,1} \left( {{l}_3 -{r}_{{L,}2,1} } \right) ^{2}}{2}} \right] {{\varvec{e}}}_3^{{\varvec{T}}} {{\varvec{R}}}_{{L,}1}^{-1} {m}_{{L,}1} }&{} {\mathbf{0}_{1\times 3} } \\ {\mathbf{0}_{1\times 3} }&{} {{{\varvec{e}}}_4^{{\varvec{T}}} {{\varvec{R}}}_{{L,}1}^{-1} {{\varvec{R}}}_{{L,}1} {{\varvec{I}}}_{{L,}1}^{l} {{\varvec{R}}}_{{L,}1}^{T} } \\ \end{array} }} \right. \\&\quad \left. {{\begin{array}{c@{\quad }c} {-\left[ {\frac{\left( {{l}_3 -{r}_{{L,}2,2} } \right) ^{3}}{3}+\frac{{r}_{{L,}2,2} \left( {{l}_3 -{r}_{{L,}2,2} } \right) ^{2}}{2}} \right] {{\varvec{e}}}_3^{{\varvec{T}}} {{\varvec{R}}}_{{L,2}}^{-1} {m}_{{L,2}} }&{} {\mathbf{0}_{1\times 3} } \\ {\mathbf{0}_{1\times 3} }&{} {-{{\varvec{e}}}_4^{{\varvec{T}}} {{\varvec{R}}}_{{L,}2}^{-1} {{\varvec{R}}}_{{L,}2} {{\varvec{I}}}_{{L,}2}^{l} {{\varvec{R}}}_{{L,}2}^{T} } \\ \end{array} }} \right) \\&{{\varvec{C}}}_G^{{\varvec{O}}} =\left( {\begin{array}{c} \left[ {\frac{\left( {{l}_3 -{r}_{{L}_{2,1} } } \right) ^{3}}{3}+\frac{{r}_{{L}_{2,1} } \left( {{l}_3 -{r}_{{L}_{2,1} } } \right) ^{2}}{2}} \right] e_3^{{\varvec{T}}} {{\varvec{R}}}_{{L,}1}^{-1} {m}_{{L,}1} -\left[ {\frac{\left( {{l}_3 -{r}_{{L}_{2,2} } } \right) ^{3}}{3}+\frac{{r}_{{L}_{2,2} } \left( {{l}_3 -{r}_{{L}_{2,2} } } \right) ^{2}}{2}} \right] {{\varvec{e}}}_3^{{\varvec{T}}} {{\varvec{R}}}_{{L,}2}^{-1} {m}_{{L,}2} \\ \mathbf{0}_{1\times 3} \\ \end{array}} \right) \\&{{\varvec{C}}}_W^{{\varvec{O}}} =\left( {{\begin{array}{c@{\quad }c@{\quad }c@{\quad }c} 0&{} 0&{} 0&{} 0 \\ 0&{} {{{\varvec{e}}}_4^{{\varvec{T}}} {{\varvec{R}}}_{{L,}1}^{-1} {\tilde{{\varvec{\omega }}}}_{{L,}1} {{\varvec{R}}}_{{L,}1} {{\varvec{I}}}_{{L,}1}^{l} {{\varvec{R}}}_{{L,}1}^{T} }&{} 0&{} {-{{\varvec{e}}}_4^{{\varvec{T}}} {{\varvec{R}}}_{{L,}2}^{-1} {\tilde{{\varvec{\omega }}}}_{{L,}2} {{\varvec{R}}}_{{L,}2} {{\varvec{I}}}_{{L,}2}^{l} {{\varvec{R}}}_{{L,}2}^{T} } \\ \end{array} }} \right) \end{aligned}$$
$$\begin{aligned}&{{\varvec{C}}}_{\lambda ,1}^{{\varvec{O}}} =\left[ {{\begin{array}{c@{\quad }c@{\quad }c@{\quad }c} {{{\varvec{C}}}_{\lambda ,1}^{{\varvec{O}}} \left( {:,1} \right) }&{} {{{\varvec{C}}}_{\lambda ,1}^{{\varvec{O}}} \left( {:,5} \right) } \\ \end{array} }} \right] \\&{{\varvec{C}}}_{\lambda ,2}^{{\varvec{O}}} =\left[ {{\begin{array}{l@{\quad }l} {{{\varvec{C}}}_{\lambda ,1}^{{\varvec{O}}} \left( {:,2:4} \right) }&{} {{{\varvec{C}}}_{\lambda ,1}^{{\varvec{O}}} \left( {:,6:10} \right) } \\ \end{array} }} \right] \\&{{\varvec{C}}}_{\lambda ,1,2}^{{\varvec{O}}} =-\left( {{{\varvec{C}}}_{\lambda ,1}^{{\varvec{O}}} } \right) ^{-1}{{\varvec{C}}}_{\lambda ,2}^{{\varvec{O}}} , \quad {{\varvec{C}}}_{{M},1,2}^{O} =\left( {{{\varvec{C}}}_{\lambda ,1}^{O} } \right) ^{-1}{{\varvec{C}}}_{M}^{O} , {{\varvec{C}}}_{{W},1,2}^{O}\\&\quad =\left( {{{\varvec{C}}}_{\lambda ,1}^{O} } \right) ^{-1}{{\varvec{C}}}_{W}^{O} , {{\varvec{C}}}_{{G},1,2}^{O} =\left( {{{\varvec{C}}}_{\lambda ,1}^{O} } \right) ^{-1}{{\varvec{C}}}_{G}^{O}\\&\Delta {{\varvec{G}}}=\left( {{\begin{array}{l@{\quad }l} {{{\varvec{C}}}_\lambda }&{} {{{\varvec{w}}}_{{CS}}^{a} } \\ \end{array} }} \right) \left( {{\begin{array}{l@{\quad }l@{\quad }l@{\quad }l@{\quad }l} {\mathbf{0}_{9\times 3} }&{} {{{\varvec{C}}}_{{G},1,2}^{{{\varvec{o}}}\mathrm{T}} \left( {:,1} \right) }&{} {\mathbf{0}_3 }&{} {{{\varvec{C}}}_{{G},1,2}^{{{\varvec{o}}}\mathrm{T}} \left( {:,2} \right) }&{} {\mathbf{0}_{3\times 30} } \\ \end{array} }} \right) ^{\mathrm{T}}\\&\Delta {{\varvec{M}}}=\left( {{\begin{array}{l@{\quad }l} {{{\varvec{C}}}_\lambda }&{} {{{\varvec{w}}}_{{CS}}^{a} } \\ \end{array} }} \right) \\&\left( {{\begin{array}{c@{\quad }c@{\quad }c@{\quad }c@{\quad }c} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 18} } \\ {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{M},1,2}^{{\varvec{O}}} \left( {1,1:6} \right) }&{} {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{M},1,2}^{{\varvec{O}}} \left( {1,7:12} \right) }&{} {\mathbf{0}_{1\times 18} } \\ {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 18} } \\ {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{M},1,2}^{{\varvec{O}}} \left( {2,1:6} \right) }&{} {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{M},1,2}^{{\varvec{O}}} \left( {2,7:12} \right) }&{} {\mathbf{0}_{1\times 18} } \\ {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 18} } \\ \end{array} }} \right) ,\\&\Delta {{\varvec{C}}}_\lambda = \left( {{\begin{array}{l@{\quad }l} {{{\varvec{C}}}_\lambda }&{} {{{\varvec{w}}}_{{CS}}^{a} } \\ \end{array} }} \right) \\&\quad \left( {{\begin{array}{c@{\quad }c@{\quad }c@{\quad }c@{\quad }c} {E_9 }&{} {\mathbf{0}_{9\times 3} }&{} {\mathbf{0}_{9\times 9} }&{} {\mathbf{0}_{9\times 5} }&{} {\mathbf{0}_{9\times 16} } \\ {\mathbf{0}_{1\times 9} }&{} {{{\varvec{C}}}_{\lambda ,1,2}^{{\varvec{O}}} \left( {1,1:3} \right) }&{} {\mathbf{0}_{1\times 9} }&{} {{{\varvec{C}}}_{\lambda ,1,2}^{{\varvec{O}}} \left( {1,4:8} \right) }&{} {\mathbf{0}_{1\times 16} } \\ {\mathbf{0}_{3\times 9} }&{} {E_3 }&{} {\mathbf{0}_{3\times 9} }&{} {\mathbf{0}_{3\times 5} }&{} {\mathbf{0}_{3\times 16} } \\ {\mathbf{0}_{1\times 9} }&{} {{{\varvec{C}}}_{\lambda ,1,2}^{{\varvec{O}}} \left( {2,1:3} \right) }&{} {\mathbf{0}_{1\times 9} }&{} {{{\varvec{C}}}_{\lambda ,1,2}^{{\varvec{O}}} \left( {2,4:8} \right) }&{} {\mathbf{0}_{1\times 16} } \\ {\mathbf{0}_{30\times 2} }&{} {\mathbf{0}_{30\times 3} }&{} {\mathbf{0}_{30\times 2} }&{} {\mathbf{0}_{30\times 5} }&{} {{{\varvec{E}}}_{30} } \\ \end{array} }} \right) \,,\\&\Delta {{\varvec{W}}} =\left( {{\begin{array}{l@{\quad }l} {{{\varvec{C}}}_\lambda }&{} {{{\varvec{w}}}_{{CS}}^{a} } \\ \end{array} }} \right) \\&\quad \left( {{\begin{array}{c@{\quad }c@{\quad }c@{\quad }c@{\quad }c} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 6} }&{} {\mathbf{0}_{9\times 18} } \\ {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{W},1,2}^{{\varvec{O}}} \left( {1,1:6} \right) }&{} {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{W},1,2}^{{\varvec{O}}} \left( {1,7:12} \right) }&{} {\mathbf{0}_{1\times 18} } \\ {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 6} }&{} {\mathbf{0}_{3\times 18} } \\ {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{W},1,2}^{{\varvec{O}}} \left( {2,1:6} \right) }&{} {\mathbf{0}_{1\times 6} }&{} {{{\varvec{C}}}_{{W},1,2}^{{\varvec{O}}} \left( {2,7:12} \right) }&{} {\mathbf{0}_{1\times 18} } \\ {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 6} }&{} {\mathbf{0}_{30\times 18} } \\ \end{array} }} \right) \,, \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, Z., Xu, L., Zhang, W. et al. Closed-form dynamic modeling and performance analysis of an over-constrained 2PUR-PSR parallel manipulator with parasitic motions. Nonlinear Dyn 96, 517–534 (2019). https://doi.org/10.1007/s11071-019-04803-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-019-04803-2

Keywords

Search

Navigation