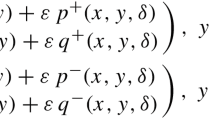Skip to main content
SpringerLink
Log in

Bifurcation of limit cycles at infinity in a class of switching systems

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

In this paper, we present a method to compute focal values and periodic constants at infinity of a class of switching systems and apply it to study a cubic system. We prove that such a cubic system can have 7 limit cycles in the sufficiently small neighborhood of infinity. Moreover, we consider a quintic switching system to obtain 14 limit cycles at infinity, while continuous quintic systems can have only 11 limit cycles in the sufficiently small neighborhood of infinity. This indicates that switching systems or discontinuous systems can exhibit more complex dynamics compared to smooth systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Li, J.: Hilbert’s 16th problem and bifurcations of planar polynomial vector fields. Int. J. Bifurc. Chaos 13, 47–106 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  2. Song, Z., Xu, J.: Stability switches and multistability coexistence in a delay-coupled neural oscillators system. J. Theor. Biol. 313, 98–114 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  3. Song, Z., Yang, K., Xu, J., Wei, Y.C.: Multiple pitchfork bifurcations and multiperiodicity coexistences in a delay-coupled neural oscillator system with inhibitory-to-inhibitory connection. Commun. Nonlinear Sci. Numer. Simul. 29, 327–345 (2015)

    Article  MathSciNet  Google Scholar 

  4. Song, Z., Xu, J., Zhen, B.: Multitype activity coexistence in an inertial two-neuron system with multiple delays. Int. J. Bifurc. Chaos 25, 1530040 (2015). (18 pages)

    Article  MathSciNet  MATH  Google Scholar 

  5. Yu, P., Lin, W.: Complex dynamics in biological systems arising from multiple limit cycle bifurcation. J. Biol. Dyn. 10, 263–285 (2016)

    Article  MathSciNet  Google Scholar 

  6. Gavrilov, L., Iliev, I.D.: Bifurcations of limit cycles from infinity in quadratic systems. Can. J. Math. 54, 1038–1064 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  7. Blows, T.R., Rousseau, C.: Bifurcation at infinity in polynomial vector fields. J. Differ. Equ. 104, 215–242 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  8. Huang, W., Liu, Y.: Bifurcations of limit cycles from infinity for a class of quintic polynomial system. Bull. Sci. Math. 128, 291–302 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  9. Zhang, Q., Liu, Y.: A cubic polynomial system with seven limit cycles at infinity. Appl. Math. Comput. 177, 319–329 (2006)

    MathSciNet  MATH  Google Scholar 

  10. Liu, Y., Chen, H.: Stability and bifurcations of limit cycles of the equator in a class of cubic polynomial systems. Comput. Math. Appl. 44, 997–1005 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  11. Sotomayor, J., Paterlini, R.: Bifurcations of polynomial vector fields in the plane, in oscillation, bifurcation and chaos. In: Atkinson F.V., Langford S.F., Mingarelli A.B. (eds.) CMS-AMS Conference Proceedings, vol. 8, pp. 665–685, Providence RI (1987)

  12. Guinez, V., Saez, E., Szanto, I.: Simultaneous Hopf bifurcations at the origin and infinity for cubic systems in dynamical systems. In: Bamon, R., Labarca, R., Palis, J. (eds.) Dynamical Systems, Pitman Research Notes in Mathematics, vol. 285, pp. 40–51. Longman Scientific & Technical, Santiago de Chile. Copublished by Wiley, New York (1990)

  13. Keith, W.L., Rand, R.H.: Dynamics of a system exhibiting the global bifurcation of a limit cycle at infinity. Int. J. Nonlinear Mech. 20, 325–338 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  14. Malaguti, L.: Soluzioni periodiche dellequazione di lienard: biforcazione dallinfinito e non unicita. Rend. Istit. Mat. Univ. Trieste 19, 12–31 (1987)

    MathSciNet  Google Scholar 

  15. Sabatini, M.: Bifurcation from infinity. Rend. Sem. Math. Univ. Padova 78, 237–253 (1987)

    MathSciNet  MATH  Google Scholar 

  16. Liu, Y.: Theory of center-focus in a class of high order singular points and infinity. Sci. China 31, 37–48 (2001)

    Google Scholar 

  17. Amelkin, V.V.: Nonlinear Oscillations in the Second Order Systems (in Russian). BGU Publ, Minsk (1982)

    Google Scholar 

  18. Coll, B., Gasull, A., Prohens, R.: Center-focus and isochronous center problems for discontinuous differential equations. Discrete Contin. Dyn. Syst. 6, 609–624 (2003)

    MathSciNet  MATH  Google Scholar 

  19. Astrom, K.J., Lee, T.H., Tan, K.K., Johansson, K.H.: Recent advances in relay feedback methods—a survey. Proceedings of IEEE Conference Systems, Manand. Cybernetics 3, 2616–2621 (1995)

  20. Bernardo, M.D., Feigin, M.I., Hogan, S.J., Homer, M.E.: Local analysis of C-bifurcations in n-dimensional piecewise-smooth dynamical systems. Chaos Solitons Fractals 11, 1881–1908 (1999)

    MathSciNet  MATH  Google Scholar 

  21. Banerjee, S., Verghese, G.: Nonlinear Phenomena in Power Electronics: Attractors, Bifurcations, Chaos, and Nonlinear Control. Wiley-IEEE Press, New York (2001)

    Book  Google Scholar 

  22. Bernardo, M.D., Kowalczyk, P., Nordmark, A.B.: Sliding bifurcations: a novel mechanism for the sudden onset of chaos in dry friction oscillators. Int. J. Bifurc. Chaos 13, 2935–2948 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  23. Dankowicz, H., Nordmark, A.B.: On the origin and bifurcations of stickC-slip oscillators. Phys. D 136, 280–302 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  24. Leine, R.I., Nijmeijer, H.: Dynamics and Bifurcations of Nonsmooth Mechanical Systems. Lecture Notes in Appl. Comput. Mech., vol. 18. Springer-Verlag, Berlin (2004)

    Book  Google Scholar 

  25. Zou, Y., Beyn, T.W.-J., Küpper, T.: Generalized Hopf bifurcation for planar Filippov systems continuous at the origin. J. Nonlinear Sci. 16, 159–177 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  26. Freire, E., Ponce, E., Ros, J.: The focus-center-limit cycle bifurcation in symmetric 3D piecewise linear systems. SIAM J. Appl. Math. 65, 1933–1951 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  27. Chen, X., Du, Z.: Limit cycles bifurcate from centers of discontinuous quadratic systems. Comput. Math. Appl. 59, 3836–3848 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  28. Tian, Y., Yu, P.: Center conditions in a switching Bautin system. J. Differ. Equ. 259, 1203–1226 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  29. Llibre, J., Lopes, B.D., Moraes, J.R.: Limit cycles for a class of continuous and discontinuous cubic polynomial differential systems. Qual. Theory Dyn. Syst. 13, 129–148 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  30. Llibre, J., Mereu, A.C.: Limit cycles for discontinuous quadratic differential systems with two zones. J. Math. Anal. Appl. 413, 763–775 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  31. Martins, R.M., Mereu, A.C.: Limit cycles in discontinuous classical Linard equations. Nonlinear Anal. RWA. 20, 67–73 (2014)

    Article  MATH  Google Scholar 

  32. Llibre, J., Ponce, E.: Hopf bifurcation from infinity for planar control systems. Publ. Matematiques 41, 181–198 (1997)

  33. Gouveia, M.R., Llibre, J., Novaes, D.D.: On limit cycles bifurcating from the infinity in discontinuous piecewise linear differential systems. Appl. Math. Comput. 271, 365–374 (2015)

    MathSciNet  Google Scholar 

  34. Andronov, A.A., Leontovich, E.A., Gordon, I.I., Maier, A.G.: Qualitative Theory of Second-Order Dynamic Systems. Wiley, New York-Toronto (1973)

    MATH  Google Scholar 

  35. Gasull, A., Torregrosa, J.: Center-focus problem for discontinuous planar differential equations. Int. J. Bifurc. Chaos 13, 1755–1765 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  36. Li, F., Yu, P., Tian, Y., Liu, Y.: Center and isochronous center conditions for switching systems associated with elementary singular points. Commun. Nonlinear Sci. Numer. Simul. 28, 81–97 (2015)

    Article  MathSciNet  Google Scholar 

  37. Chavarriga, J., Sabatini, M.: A survey of isochronous centers. Qual. Theory Dyn. Syst. 1, 1–70 (1999)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

F. Li and Y. Liu thank the support received from the National Nature Science Foundation of China (No. 11601212), and P. Yu acknowledges the support received from the Natural Science and Engineering Research Council of Canada (No. R2686A02).

Author information

Authors and Affiliations

  1. School of Science, Linyi University, Linyi, Shandong, 276005, People’s Republic of China

    Feng Li & Yuanyuan Liu

  2. Department of Applied Mathematics, Western University, London, ON, N6A 5B7, Canada

    Feng Li & Pei Yu

Authors
  1. Feng Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yuanyuan Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Pei Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Pei Yu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, F., Liu, Y. & Yu, P. Bifurcation of limit cycles at infinity in a class of switching systems. Nonlinear Dyn 88, 403–414 (2017). https://doi.org/10.1007/s11071-016-3249-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-016-3249-4

Keywords

Search

Navigation