Skip to main content
SpringerLink
Log in

Bifurcation of periodic orbits in discontinuous systems

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

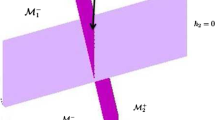
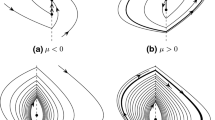
This paper treads discontinuous bifurcation in piecewise smooth systems of Filippov type. These bifurcations occur when a fixed point or a periodic orbit crosses with the border between two regions of smooth behavior. A detailed analysis of generalization Poincaré map and monodromy matrix which are related shows that subfamily of system with invariant cone-like objects is foliated by periodic orbits and determines its stability. In addition, we introduce a theoretical framework for analyzing 3D perturbed nonlinear piecewise smooth systems and give necessary conditions so that different types of bifurcations occur. The analysis identifies criteria for the existence of a novel bifurcation based on sensitively the location of the return map. Moreover, the piecewise smooth Melnikov function and sufficient conditions of the existence of the periodic orbits for nonlinear perturbed system are explicitly obtained.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Awrejcewicz, J., Lamarque, C.: Bifurcation and Chaos in Nonsmooth Mechanical Systems. World Scientific, Singapore (2004)

    MATH  Google Scholar 

  2. Awrejcewicz, J., Olejnik, P.: Regular and chaotic stick-slip dynamics in a self-excited two-degree-of-freedom system with friction. Int. J. Bifur. Chaos. 13(4), 843–861 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  3. Brogliato, B.: Nonsmooth Mechanics-Models, Dynamics and Control. Springer-Verlag, London (1999)

    MATH  Google Scholar 

  4. Carmona, V., Freire, E., Ponce, E., Torres, F.: Bifurcation of invariant cones in piecewise linear homogeneous systems. Int. J. Bifur. Chaos. 15(8), 2469–2484 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  5. di Bernardo, M., Budd, C., Champneys, A.R., Kowalczyk, P.: Piecewise-smooth Dynamical Systems: Theory and Applications. Applied Mathematics Series, vol. 163. Springer-Verlag, London (2008)

    MATH  Google Scholar 

  6. di Bernardo, M., Budd, C., Champneys, A.R., Kowalczyk, P., Nordmark, A.B., Olivar, G., Piiroinen, P.T.: Bifurcations in nonsmooth dynamical systems. SIAM Rev. 50(4), 629–701 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  7. Dieci, L., Lopez, L.: Sliding motion in Filippov differential systems: theoretical results and a computational approach. SIAM J. Numer. Anal. 47, 2023–2051 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  8. Du, Z., Li, Y., Zhang, W.: Bifurcation of periodic orbits in a class of planar Filippov systems. Nonlin. Anal. Ser. A 69, 3610–3628 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  9. Fečkan, M.: Bifurcation of periodic and chaotic solutions in discontinuous systems. Arch. Math. 34(1), 73–82 (1998)

    MathSciNet  MATH  Google Scholar 

  10. Fečkan, M., Pospíšil, M.: Bifurcation from family of periodic orbits in discontinuous autonomous systems. Differ. Equ. Dyn. Syst. 20(3), 207–234 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  11. Filippov, A.F.: Differential equations with discontinuous right-hand side. Am. Math. Soc. Transl. 2(42), 199–231 (1964)

    Article  MATH  Google Scholar 

  12. Galvanetto, U.: Bifurcations and chaos in a four-dimensional mechanical system with dry-friction. J. Sound Vibr. 204, 690–695 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  13. Galvanetto, U.: Some discontinuous bifurcations in a two block stick-slip system. J. Sound Vibr. 284, 653–669 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  14. Gouveiaa, M.R.A., Llibreb, J., Novaesc, D.D., Pessoaa, C.: Piecewise smooth dynamical systems: persistence of periodic solutions and normal forms. J. Differ. Eqs. 260, 6108–6129 (2016)

    Article  MathSciNet  Google Scholar 

  15. Guckenheimer, J., Holmes, J.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector. Springer-Verlag, New York (1983)

    Book  MATH  Google Scholar 

  16. Hosham, H.A.: Bifurcations in nonsmooth high-dimensional brake system. In: Proceedings of 2nd International Symposium Rare Attractors and Rare Phenomena in Nonlinear Dynamics RA’11 May (16), pp. 17–20. Rīga - Jūrmala, Latvia (2011)

  17. Hosham, H.A.: Cone-like invariant manifolds for nonsmooth systems. Ph.D. Thesis. Universität zu Köln (2011)

  18. Küpper, T.: Invariant cones for non-smooth systems. Math. Comput. Simul. 79, 1396–1409 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  19. Küpper, T., Hosham, H.A.: Reduction to invariant cones for non-smooth systems. Math. Comput. Simul. 81, 980–995 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  20. Küpper, T., Hosham, H.A., Dudtschenko, K.: The dynamics of bells as impacting system. J. Mech. Eng. Sci. 225(10), 2436–2443 (2011)

    Article  Google Scholar 

  21. Küpper, T., Hosham, H.A., Weiss, D.: Bifurcation for nonsmooth dynamical systems via reduction methods. In: Johann, A., Kruse, H.-P., Rupp, F., Schmitz, S. (eds.) Recent Trends in Dynamical Systems, vol. 35 of Proceedings in Mathematics and Statistics, pp. 79–105. Springer, Basel (2013)

  22. Li, S., Liu, C.: A linear estimate of the number of limit cycles for some planar piecewise smooth quadratic differential system. J. Math. Anal. Appl. 428, 1354–1367 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  23. Leine, R.I., Nijmeijer, H.: Dynamics and Bifurcations of Non- Smooth Mechanical Systems. Springer-Verlag, Berlin (2004)

    Book  MATH  Google Scholar 

  24. Leine, R.I., van Campen, D.H., van de Vrande, B.L.: Bifurcations in nonlinear discontinuous systems. Nonlinear Dyn. 23(2), 105–164 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  25. Pfeiffer, F., Glocker, C.: Multibody Dynamics with Unilateral Contacts, Wiley Series in Nonlinear Science. Wiley, Hoboken (1996)

    Book  MATH  Google Scholar 

  26. Sanjuán, A.F.: Liénard systems, limit cycles, and Melnikov theory. Phys. Rev. E 57, 340–344 (1998)

    Article  MathSciNet  Google Scholar 

  27. Shen, J., Du, Z.: Heteroclinic bifurcation in a class of planar piecewise smooth systems with multiple zones. ZAMP 67, 1–17 (2016)

  28. van de Wouw, N., Leine, R.I.: Attractivity of equlibrium sets of systems with dry friction. Int. J. Nonlin. Dyn. Chaos Engin. Syst. 35, 19–39 (2004)

    Article  MATH  Google Scholar 

  29. Weiss, D., Küpper, T., Hosham, H.A.: Invariant manifolds for nonsmooth systems. Phys. D: Nonlinear Phenom. 241(22), 1895–1902 (2012)

    Article  MathSciNet  Google Scholar 

  30. Weiss, D., Küpper, T., Hosham, H.A.: Invariant manifolds for nonsmooth systems with sliding mode. Math. Comput. Simul. 110, 15–32 (2015)

    Article  MathSciNet  Google Scholar 

  31. Wu, C., Si, G., Zhang, Y., Yang, N.: The fractional-order state-space averaging modeling of the Buck-Boost DC/DC converter in discontinuous conduction mode and the performance analysis. Nonlinear Dyn. 1(79), 689–703 (2014)

    Google Scholar 

  32. Xiong, Y.: Limit cycle bifurcations by perturbing piecewise smooth Hamiltonian systems with multiple parameters. J. Math. Anal. Appl. 421, 260–275 (2015)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, Al-Azhar University, Assiut, 71524, Egypt

    Hany A. Hosham

Authors
  1. Hany A. Hosham
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hany A. Hosham.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hosham, H.A. Bifurcation of periodic orbits in discontinuous systems. Nonlinear Dyn 87, 135–148 (2017). https://doi.org/10.1007/s11071-016-3031-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-016-3031-7

Keywords

Search

Navigation