Cable’s mode interactions under vertical support motions: boundary resonant modulation

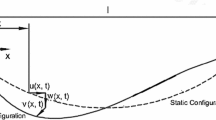
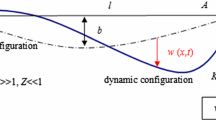
Abstract

Cable’s triad and two-to-one mode interactions excited by support motions are modeled and analyzed in a unified boundary modulation formulation. Based upon proper scaling and a boundary resonance concept, the small support motion is modeled as a nonzero boundary modulation term for cable’s reduced (slow) dynamics through attacking cable’s continuous dynamic equations directly by the multiple scale method. Boundary resonance coefficients, characterizing the boundary modulation effect, are derived analytically for both cable’s triad and two-to-one mode resonant dynamics. It is found that the boundary resonance coefficients depend on both cable’s boundary modal information and cable’s initial deformation/sag. Frequency response diagrams based on cable’s reduced models (modulation equations) are obtained, with stability and bifurcation determined. Finally, these approximate analytical results are verified by the numerical results through applying the finite-difference method directly to cable’s original partial differential equations.

Appendices

Appendix 1

Suspended cable’s linear modal analysis can be found in reference [3]. We restrict our attention to cable’s in-plane symmetric modes in this paper, and these modes are given by

$$\begin{aligned} \phi _i \left( x \right)= & {} c_i \left[ {1-\tan \left( {\frac{\omega _i }{2}} \right) \sin \omega _i x-\cos \omega _i x} \right] ,\nonumber \\&i=1,3,5\cdots \end{aligned}$$

(60)

where $c_{i}$ is the normalization constants. And the associated eigenfrequencies are determined by

$$\begin{aligned} \frac{1}{2}\omega _i -\tan \left( {\frac{1}{2}\omega _i } \right) -\frac{1}{2\lambda ^{2}}\omega _i^3 =0 \end{aligned}$$

(61)

where $\lambda ^{2}=EA/mgl(8b/l)^{3}$ is the elasto-geometric parameter. The above nonlinear transcendental equations can be solved by the Newton–Raphson method.

Appendix 2

$$\begin{aligned} \varPi _{12} \left( x \right)= & {} \alpha \left\langle {{\phi }_1' ,{\phi }_2'} \right\rangle {y}''+\alpha \left\langle {{y}',{\phi }_2' } \right\rangle {\phi }_1'' +\alpha \left\langle {{y}',{\phi }_1'} \right\rangle {\phi }_2''\nonumber \\ \end{aligned}$$

(62)

$$\begin{aligned} \varPi _{31} \left( x \right)= & {} \alpha \left\langle {{\phi }_1' ,{\phi }_3' } \right\rangle {y}''+\alpha \left\langle {{y}',{\phi }_3' } \right\rangle {\phi }_1'' +\alpha \left\langle {{y}',{\phi }_1' } \right\rangle {\phi }_3''\nonumber \\ \end{aligned}$$

(63)

$$\begin{aligned} \varPi _{32} \left( x \right)= & {} \alpha \left\langle {{\phi }_2' ,{\phi }_3' } \right\rangle {y}''+\alpha \left\langle {{y}',{\phi }_3' } \right\rangle {\phi }_2'' +\alpha \left\langle {{y}',{\phi }'_2 } \right\rangle {\phi }_3''\nonumber \\ \end{aligned}$$

(64)

$$\begin{aligned} \varPi _1 \left( x \right)= & {} \left( {\alpha /2} \right) \left\langle {{\phi }_1', {\phi }_1' } \right\rangle {y}''+\alpha \left\langle {{y}',{\phi }_1' } \right\rangle {\phi }_1'' \end{aligned}$$

(65)

$$\begin{aligned} \varPi _{21} \left( x \right)= & {} \alpha \left\langle {{\phi }_1' ,{\phi }_2' } \right\rangle {y}''+\alpha \left\langle {{y}',{\phi }_2' } \right\rangle {\phi }_1'' +\alpha \left\langle {{y}',{\phi }_1' } \right\rangle {\phi }_2''\nonumber \\ \end{aligned}$$

(66)

Appendix 3

Single-mode solutions associated with triad mode resonance and two-to-one resonance, and their stability analysis, are determined by following reference [18].

The single-mode equilibrium solutions of cable’s modulation equations are obtained through setting $a_1 =a_2 ={a}_3' ={\gamma }_2' =0$ in Eqs. (44)–(46) and Eqs. (47) and (48)

$$\begin{aligned} a_3 =\frac{1}{2\omega _3 }\frac{\varLambda _3 Z_0 }{\sqrt{\mu _3^2 +\sigma _2^2 }} \end{aligned}$$

(67)

For coupled-mode solutions, the cable’s high-frequency mode $a_{3}$ is saturated at (i.e., keeping constant if arriving at a critical value, irrespective of the excitation)

$$\begin{aligned} a_3^*=\frac{4}{\left| {\varGamma _k } \right| }\sqrt{\omega _1 \omega _2 }\sqrt{\mu _1 \mu _2 }\left[ {1+\left( {\frac{\sigma _1 +\sigma _2 }{\mu _1 +\mu _2 }} \right) ^{2}} \right] ^{\frac{1}{2}} \end{aligned}$$

(68)

In other words, $0\le a_3 \le a_3^*$. Furthermore, the single-mode equilibrium solution in Eq. (67) is stable if $a_3 <a_3^*$, otherwise unstable.