Skip to main content
SpringerLink
Log in

Internal, combinational and sub-harmonic resonances of a nonlinear asymmetrical rotating shaft

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

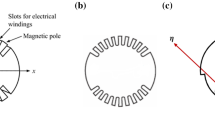
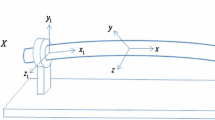
The internal, combinational and sub-harmonic resonances of a simply supported rotating asymmetrical shaft with unequal mass moments of inertia and bending stiffness in the direction of principal axes are simultaneously considered. The excitation terms are due to dynamic imbalances of shaft and shaft asymmetry. The nonlinearities are due to extensionality of shaft and large amplitudes. To analyze the nonlinear equations of motion, the method of harmonic balance is utilized. The influences of inequality between two eccentricities corresponding to the principal axes and external damping on the steady-state responses and bifurcation points of the asymmetrical rotating shaft are investigated. The numerical computations are utilized to verify the harmonic balance method results. The results of harmonic balance method are in accordance with those of numerical computations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

Abbreviations

\(A\) :

Cross-sectional area

\(c\) :

External damping coefficient

\(D_{xx} ,D_{yy} ,D_{zz} \) :

Torsional and bending stiffnesses

\(E\) :

Elasticity modulus

\(e_y ,\,e_z \) :

Eccentricity distributions with respect to \(y\) and \(z\) axes

\(G\) :

Shear modulus

\(I_{xx} , I_{yy} ,I_{zz} \) :

Polar and diametrical mass moments of inertia

\(k_x ,k_y ,k_z \) :

Shaft curvatures

\(l\) :

Length of rotating shaft

\(m\) :

Mass per unit length of the shaft

\(N_{xx} \) :

Longitudinal stiffness

\(u\) :

Longitudinal displacement

\(v, w\) :

Transverse displacements

\(\xi \) :

Strain along the neutral axis of the shaft

\(\psi , \theta \) :

Euler angles

\(\rho \) :

Mass density

\(\varOmega \) :

Rotational speed

\(\varDelta _\mathrm{I} \) :

Difference between two mass moments of inertia

\(\varDelta _\mathrm{D} \) :

Difference between two bending stiffnesses

References

  1. Ganesan, R.: Effects of bearing and shaft asymmetries on the instability of rotors operating at near-critical speeds. Mech. Mach. Theory 35(5), 737–752 (2000)

    Article  MATH  Google Scholar 

  2. Wettergren, H.L., Olsson, K.O.: Dynamic instability of a rotating asymmetric shaft with internal viscous damping supported in anisotropic bearings. J. Sound Vib. 195(1), 75–84 (1996)

  3. Verichev, N.N., Verichev, S.N., Erofeyev, V.I.: Damping lateral vibrations in rotary machinery using motor speed modulation. J. Sound Vib. 329(1), 13–20 (2010)

    Article  Google Scholar 

  4. Shahgholi, M., Khadem, S.E.: Primary and parametric resonances of asymmetrical rotating shafts with stretching nonlinearity. Mech. Mach. Theory 51, 131–144 (2012)

    Article  Google Scholar 

  5. Shahgholi, M., Khadem, S.: Stability analysis of a nonlinear rotating asymmetrical shaft near the resonances. Nonlinear Dyn. 1–15 (2012). doi:10.1007/s11071-012-0535-7

  6. Sabuncu, M., Evran, K.: The dynamic stability of a rotating pre-twisted asymmetric cross-section Timoshenko beam subjected to lateral parametric excitation. Int. J. Mech. Sci. 48(8), 878–888 (2006)

    Article  MATH  Google Scholar 

  7. Vakakis, A.: Fundamental and subharmonic resonances in a system with a ‘1-1’ internal resonance. Nonlinear Dyn. 3(2), 123–143 (1992). doi:10.1007/bf00118989

    Article  Google Scholar 

  8. Carrella, A., Friswell, M.I., Zotov, A., Ewins, D.J., Tichonov, A.: Using nonlinear springs to reduce the whirling of a rotating shaft. Mech. Syst. Signal Process. 23(7), 2228–2235 (2009)

    Article  Google Scholar 

  9. Zhang, W.-M., Meng, G.: Stability, bifurcation and chaos of a high-speed rub-impact rotor system in MEMS. Sens. Actuators A Phys. 127(1), 163–178 (2006)

    Article  MathSciNet  Google Scholar 

  10. McDonald, R.J., Namachchivaya, N.S.: Global bifurcations in periodically perturbed gyroscopic systems with application to rotating shafts. Chaos Solitons Fractals 8(4), 613–636 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  11. Badlani, M., Kleinhenz, W., Hsiao, C.C.: The effect of rotary inertia and shear deformation on the parametric stability of unsymmetric shafts. Mech. Mach. Theory 13(5), 543–553 (1978)

    Article  Google Scholar 

  12. Parkinson, A.G.: The vibration and balancing of shafts rotating in asymmetric bearings. J. Sound Vib. 2(4), 477–501 (1965)

    Article  Google Scholar 

  13. Kurnik, W.: Stability and bifurcation analysis of a nonlinear transversally loaded rotating shaft. Nonlinear Dyn. 5(1), 39–52 (1994). doi:10.1007/bf00045079

    Google Scholar 

  14. Al-Nassar, Y.N., Al-Bedoor, B.O.: On the vibration of a rotating blade on a torsionally flexible shaft. J. Sound Vib. 259(5), 1237–1242 (2003)

    Article  Google Scholar 

  15. Legrand, M., Jiang, D., Pierre, C., Shaw, S.W.: Nonlinear normal modes of a rotating shaft based on the invariant manifold method. Int J Rotating Mach. 10(4), 319–335 (2004)

  16. Rajalingham, C., Bhat, R.B., Xistris, G.D.: Influence of support flexibility and damping characteristics on the stability of rotors with stiffness anisotropy about shaft principal axes. Int. J. Mech. Sci. 34(9), 717–726 (1992)

    Article  Google Scholar 

  17. Khadem, S.E., Shahgholi, M., Hosseini, S.A.A.: Two-mode combination resonances of an in-extensional rotating shaft with large amplitude. Nonlinear Dyn. 65(3), 217–233 (2011). doi:10.1007/s11071-010-9884-2

    Article  MATH  MathSciNet  Google Scholar 

  18. Khadem, S.E., Shahgholi, M., Hosseini, S.A.A.: Primary resonances of a nonlinear in-extensional rotating shaft. Mech. Mach. Theory 45(8), 1067–1081 (2010)

  19. Luczko, J.: A geometrically non-linear model of rotating shafts with internal resonance and self-excited vibration. J. Sound Vib. 255(3), 433–456 (2002)

    Article  Google Scholar 

  20. Karpenko, E.V., Wiercigroch, M., Cartmell, M.P.: Regular and chaotic dynamics of a discontinuously nonlinear rotor system. Chaos Solitons Fractals 13(6), 1231–1242 (2002)

    Article  MATH  Google Scholar 

  21. Wang, L., Cao, D.Q., Huang, W.: Nonlinear coupled dynamics of flexible blade–rotor–bearing systems. Tribol. Int. 43(4), 759–778 (2010)

    Article  Google Scholar 

  22. Pei, Y.-C.: Stability boundaries of a spinning rotor with parametrically excited gyroscopic system. Eur. J. Mech. A. Solids 28(4), 891–896 (2009)

    Article  MATH  Google Scholar 

  23. Sinou, J.J.: Non-linear dynamics and contacts of an unbalanced flexible rotor supported on ball bearings. Mech. Mach. Theory 44(9), 1713–1732 (2009)

    Article  MATH  Google Scholar 

  24. Fan, Y.-H., Chen, S.-T., Lee, A.-C.: Active control of an asymmetrical rigid rotor supported by magnetic bearings. J. Franklin Inst. 329(6), 1153–1178 (1992)

    Article  Google Scholar 

  25. Turhan, Ö., Bulut, G.: Dynamic stability of rotating blades (beams) eccentrically clamped to a shaft with fluctuating speed. J. Sound Vib. 280(3–5), 945–964 (2005)

    Article  Google Scholar 

  26. Kamel, M., Bauomy, H.: Nonlinear behavior of a rotor–AMB system under multi-parametric excitations. Meccanica 45(1), 7–22 (2010). doi:10.1007/s11012-009-9213-3

    Article  MATH  Google Scholar 

  27. Sheu, H.-C., Chen, L.-W.: A lumped mass model for parametric instability analysis of cantilever shaft–disk systems. J. Sound Vib. 234(2), 331–348 (2000)

    Article  Google Scholar 

  28. Chang-Jian, C.-W., Chen, Co-K: Chaos of rub-impact rotor supported by bearings with nonlinear suspension. Tribol. Int. 42(3), 426–439 (2009)

    Article  Google Scholar 

  29. Shahgholi, M., Khadem, S.: Hopf bifurcation analysis of asymmetrical rotating shafts. Nonlinear Dyn.77(4), 1141–1155 (2014). doi:10.1007/s11071-014-1367-4

  30. Nayfeh, A.H., Pai, P.F.: Linear and Nonlinear Structural Mechanics. Wiley-Interscience, New York (2004)

    Book  MATH  Google Scholar 

  31. Nayfeh, A.H.: Introduction to Perturbation Techniques. Wiley-Interscience, New York (1981)

    MATH  Google Scholar 

  32. Nayfeh, A.H., Mook, D.T.: Nonlinear Oscillations. Wiley-Interscience, New York (1995)

    Book  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Tarbiat Modares University, P.O. Box 14115-177, Tehran, Iran

    Majid Shahgholi & S. E. Khadem

Authors
  1. Majid Shahgholi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. E. Khadem
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. E. Khadem.

Appendix

Appendix

$$\begin{aligned}&K_{11}^{(1n)} =\int \limits _0^1 {\phi _n ^{2}(x)} \mathrm{d}x , \\&K_{11}^{(2n)}=\frac{1}{\pi ^{2}}\int \limits _0^1 {{\phi }''_n (x)\phi _n (x)} \mathrm{d}x , \\&K_{11}^{(3n)}=\frac{1}{\pi ^{4}}\int \limits _0^1 {\phi _n ^{(IV)}(x)} \phi _n (x)\mathrm{d}x\\&\varGamma _1^{(n)}=K_{11}^{(1n)} -K_{11}^{(2n)} I , \\&\varGamma _4^{(n)}=-\frac{\alpha }{\pi ^{4}}\int \limits _0^1 {{\phi '}_n^{2}(x)\phi _n ^{\prime \prime }(x)\phi _n (x)} \mathrm{d}x ,\\&\varGamma _3^{(n)} =K_{11}^{(2n)} I_1 ,\quad \varGamma _5^{(n)} =\varDelta _\mathrm{I} K_{11}^{(2n)} \\&e_1 =\int \limits _0^1 {e_z \phi _n } (x)\mathrm{d}x , \\&e_2=\int \limits _0^1 {e_y \phi _n } (x)\mathrm{d}x ,\quad \vartheta _t =\sqrt{e_1^2 +e_2^2 } \\ \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shahgholi, M., Khadem, S.E. Internal, combinational and sub-harmonic resonances of a nonlinear asymmetrical rotating shaft. Nonlinear Dyn 79, 173–184 (2015). https://doi.org/10.1007/s11071-014-1654-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-014-1654-0

Keywords

Search

Navigation