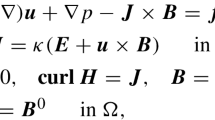Skip to main content
SpringerLink
Log in

Nonlinear transport coefficients from Grad’s 13–moment approximation

  • Published:
Meccanica Aims and scope Submit manuscript

Abstract

In this work we use Grad’s 13–moment approximation to study a stationary shock wave in dilute gases. The conservation equations valid across the shock wave profile depend on the constitutive relations for the viscous tensor and the heat flux. The Grad’s 13-moment equations are taken to obtain the set of constitutive equations and the conservation equations are closed and solved. In addition, such constitutive equations can be written in terms of nonlinear transport coefficients depending on the velocity gradient. Their structure shows that the temperature gradient and the gradient of velocity produce non-trivial contributions in the heat flux as well as in the viscous tensor. A comparison with experiments and some other models is done.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Salas MD (2007) The curious events leading to the theory of shock waves. Shock Waves 16(6):477–487

    Article  MATH  Google Scholar 

  2. Gilbarg D (1951) The existence and limit behavior of the one-dimensional shock layer. Am J Math 73:256–274

    Article  MathSciNet  MATH  Google Scholar 

  3. Taylor GI (1910) The conditions necessary for discontinuous motion in gases. Proc R Soc London Series A 84:371–377

    Article  MATH  Google Scholar 

  4. Becker R (1922) Stosswelle und detonation. Z Phys 8:321–362

    Article  Google Scholar 

  5. Becker R (1929) Impact waves and detonation, parts i and ii. Technical report 506, national advisory committee for aeronautics, Washington. From Zeitschrift für Physick, Volume VIII

  6. Thomas LH (1944) Note on Becker’s theory of the shock front. J Chem Phys 12:449–453

    Article  Google Scholar 

  7. Johnson BM (2013) Analytical shock solutions at large and small Prandtl number. J Fluid Mech 726:4

    Article  MathSciNet  Google Scholar 

  8. Johnson BM (2014) Closed-form shock solutions. J Fluid Mech 745:1

    Article  MathSciNet  Google Scholar 

  9. Myong RS (2014) Analytical solutions of shock structure thickness and asymmetry in Navier-Stokes/fourier framework. AIAA J 52:1075–1080

    Article  Google Scholar 

  10. Uribe FJ, Velasco RM (2019) Exact solutions for shock waves in dilute gases. Phys Rev E 100(2):023118

    Article  MathSciNet  Google Scholar 

  11. Grad H (1952) The profile of a steady plane shock wave. Comm Pure Appl Math  5(3):257–300

    Article  MathSciNet  MATH  Google Scholar 

  12. Holway LH (1964) Existence of kinetic theory solutions to the shock structure problem. Phys Fluids 7:911–913

    Article  MATH  Google Scholar 

  13. Gilbarg D, Paolucci D (1953) The structure of shock waves in the continuum theory of fluids. J Ration Mech Anal 2:617–642

    MathSciNet  MATH  Google Scholar 

  14. Weiss W (1995) Continuous shock structure in extended thermodynamics. Phys Rev E 52:5760–5763

    Article  Google Scholar 

  15. Anile AM, Majorana A (1981) Shock structure for heat conducting and viscous fluids. Meccanica 16:149–156

    Article  MATH  Google Scholar 

  16. Jou D, Pavon D (1991) Nonlocal and nonlinear effects in shock-waves. Phys Rev A 44:6496–6502

    Article  Google Scholar 

  17. Müller I, Ruggeri T (1998) Rational extended thermodynamics. Springer, New York

    Book  MATH  Google Scholar 

  18. Cai Z, Torrilhon M (2019) On the Holway-Weiss debate: convergence of the grad-moment-expansion in kinetic gas theory. Phys Fluids 31(12):126105

    Article  Google Scholar 

  19. Boillat G, Ruggeri T (1998) On the shock structure problem for hyperbolic system of balance laws and convex entropy. Contin Mech Thermodyn 10:285–292

    Article  MathSciNet  MATH  Google Scholar 

  20. Struchtrup H, Torrilhon M (2003) Regularization of Grad’s 13 moment equations: derivation and linear analysis. Phys Fluids 15:2668–2680

    Article  MathSciNet  MATH  Google Scholar 

  21. Timokhin MY, Struchtrup H, Kokhanchik AA, Bondar YA (2017) Different variants of R13 moment equations applied to the shock-wave structure. Phys Fluids 29:037105

    Article  Google Scholar 

  22. AlGhoul M, Eu BC (1997) Generalized hydrodynamics and shock waves. Phys Rev E 56:2981–2992

    Article  MathSciNet  Google Scholar 

  23. Myong RS (2014) On the high Mach number shock structure singularity caused by overreach of Maxwellian molecules. Phys Fluids 26(5):056102

    Article  Google Scholar 

  24. Zinner CP, Öttinger HC (2019) Numerical stability with help from entropy: solving a set of 13 moment equations for shock tube problem. J Non-Equilib Thermodyn 44(1):43–69

    Article  Google Scholar 

  25. Agrawal A, Kushwaha HM, Jadhav RS (2020) Microscale flow and heat transfer. Springer, Switzerland

    Book  Google Scholar 

  26. García-Colín LS, Velasco RM, Uribe FJ (2004) Inconsistency in the Moment’s method for solving the Bolztmann equation. J Non-Equilib Thermodyn 29:257–277

    Article  MATH  Google Scholar 

  27. Uribe FJ, García-Colín LS (1999) Nonlinear viscosity and Grad’s method. Phys Rev E 60:4052

    Article  Google Scholar 

  28. Velasco RM, Uribe FJ (2021) A study on the Holian conjecture and linear irreversible thermodynamics for shock-wave structure. Wave Motion 100:102684

    Article  MathSciNet  MATH  Google Scholar 

  29. Chapman S, Cowling TG (1970) The mathematical theory of non-uniform gases. Cambridge University Press, Cambridge

    MATH  Google Scholar 

  30. Muntz EP, Harnett NL (1969) Molecular velocity distribution function measurements in a normal shock wave. Phys Fluids 12(10):2027–2035

    Article  Google Scholar 

  31. Hurly JJ, Mehl JB (2007) \(^4\)He thermophysical properties: new Ab initio calculations. J Res Natl Stand Technol 112:75–94

    Article  Google Scholar 

  32. Greenshields CJ, Reese JM (2007) The structure of shock waves as a test of Brenner’s modifications to the Navier-Stokes equations. J Fluid Mech 580:407–429

    Article  MathSciNet  MATH  Google Scholar 

  33. Velasco RM, Uribe FJ (2019) Shock-wave structure according to a linear irreversible thermodynamic model. Phys Rev E 99(2):023114

    Article  Google Scholar 

  34. Velasco RM (2020) Uribe FJ (2020) Erratum: shock-wave structure according to a linear irreversible thermodynamic model [Phys Rev E 99: 023114 (2019)]. Phys Rev E 1:019903

    Article  Google Scholar 

  35. Uribe FJ, Velasco RM (2018) Shock-wave structure based on the Navier-Stokes-fourier equations. Phys Rev E 97:043117

    Article  MathSciNet  Google Scholar 

  36. Velasco RM, García-Colín LS, Uribe FJ (2011) Entropy production: its role in non-equilibrium thermodynamics. Entropy 13:82–116

    Article  MathSciNet  MATH  Google Scholar 

  37. Uribe FJ, Velasco RM (2016) Einstein relation for electrons in an electric field. J Stat Phys 162:242–266

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

We would like to thank the referees for their insigths and comments.

Author information

Author notes

  1. Francisco J. Uribe and Rosa M. Velasco have contributed equally to this work.

Authors and Affiliations

  1. Department of Physics, Universidad Autónoma Metropolitana, Av. San Rafael Atlixco 186, 09340, México, CDMX, México

    Francisco J. Uribe & Rosa M. Velasco

Authors
  1. Francisco J. Uribe
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Rosa M. Velasco
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Francisco J. Uribe.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Uribe, F.J., Velasco, R.M. Nonlinear transport coefficients from Grad’s 13–moment approximation. Meccanica 58, 1099–1108 (2023). https://doi.org/10.1007/s11012-022-01565-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-022-01565-x

Keywords

Search

Navigation