Equilibrium Joining Strategies in the Retrial Queue with Two Classes of Customers and Delayed Vacations

Abstract

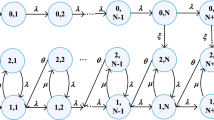
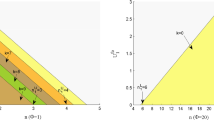
We consider a non-preemptive priority M/M/1 retrial queue with two classes of customers (low-priority and high-priority customers) and delayed vacations. When the server is unavailable, an arriving high-priority customer can wait in line, whereas an arriving low-priority customer needs to enter a virtual queue and retry later. After completing a service, the server will remain idle for a reserved idle time if it finds no high-priority customers in the system. Arrivals during the reserved idle period will be served immediately. Otherwise, if no customers arrive during this interval, the server will switch to the vacation state. By constructing a three-dimensional Markov chain, we successively obtain the stability condition of the system and some main performance measures. Then depending on a linear reward-cost structure, we derive customers’ two-dimensional equilibrium joining strategies in the fully unobservable case. Due to the complexity of the social welfare function, we explore the socially optimal joining strategies through the Particle Swarm Optimization (PSO) algorithm. Finally, we illustrate the impact of system parameters on the two types of joining strategies via numerical experiments.

Appendix

Proof of Theorem 1

Proof

We define the generator H as the following form

$$\begin{aligned} H=A+B+C=\left\{ \begin{array}{ccccccccccccccc} \eta _{4} &{} {\lambda _1} + \theta &{} \lambda _{2}&{} \alpha &{} &{}&{}&{}&{}&{}&{}&{}&{}&{}&{}\\ \mu _{1} &{} {\bar{\eta }_1} &{} &{} &{} \lambda _{2}&{} &{}&{}&{}&{}&{}&{}&{}&{}&{}\\ \mu _{2} &{} &{}{\bar{\eta }_2}&{} &{} &{}\lambda _{2} &{}&{}&{}&{}&{}&{}&{}&{}&{}\\ \beta &{} &{}0 &{}{\bar{\eta }_3}&{} &{} &{}\lambda _{2}&{} &{}&{}&{}&{}&{}&{}&{}\\ &{} &{} \mu _{1} &{} &{}{\bar{\eta }_1} &{} &{} &{}\lambda _{2}&{} &{}&{}&{}&{}&{}&{}\\ &{} &{} \mu _{2} &{} &{} &{}{\bar{\eta }_2} &{} &{}&{}\lambda _{2}&{}&{}&{}&{}&{}&{} \\ &{} &{} \beta &{} &{} &{}0&{}{\bar{\eta }_3}&{}&{}&{}\lambda _{2}&{}&{}&{}&{}&{}\\ &{} &{} &{} &{} &{}\mu _{1} &{} &{}{\bar{\eta }_1}&{}&{}&{}\lambda _{2}&{}&{}&{}&{} \\ &{} &{} &{} &{} &{}\mu _{2} &{}&{}&{}{\bar{\eta }_2}&{}&{}&{}\lambda _{2}&{}&{}\\ &{} &{} &{} &{} &{}\beta &{}&{}&{}0&{}{\bar{\eta }_3}&{}&{}&{}\lambda _{2}&{}\\ \ &{} &{} &{} &{} &{} &{}&{}&{}\vdots &{}&{}\ddots &{}&{}&{}\ddots \\ \end{array}\right\} . \end{aligned}$$

By reference to Neuts (1981) and Kapodistria and Palmowski (2017), the QBD drift condition is ${\pi Ce} < {\pi Ae}$, where ${\pi } = \left( {{\pi _0},{\pi _1},{\pi _2}, \cdots } \right)$ satisfies ${\pi H} ={0}$ and ${\pi e} = 1$, in which e is a column vector of 1’s. Then by expanding the matrix vector equation ${\pi H} = {0}$, we get the following equations

$$\begin{aligned} - \left( {{\lambda _{1} + \lambda }_{2} + \alpha + \theta } \right) \pi _{0} + \mu _{1}\pi _{1} + \mu _{2}\pi _{2} + \beta \pi _{3} = 0, \end{aligned}$$

(47)

$$\begin{aligned} ({\lambda _1} + \theta ){\pi _0} - ({\lambda _2} + {\mu _1}){\pi _1} = 0 , \end{aligned}$$

(48)

$$\begin{aligned} {\lambda _2}{\pi _0} - \left( {{\lambda _2} + {\mu _2}} \right) {\pi _2} + {\mu _1}{\pi _4} + {\mu _2}{\pi _5} + \beta {\pi _6} = 0 , \end{aligned}$$

(49)

$$\begin{aligned} \alpha {\pi _0} - \left( {{\lambda _2} + \beta } \right) {\pi _3} = 0 , \end{aligned}$$

(50)

$$\begin{aligned} \lambda _{2}\pi _{i} - \left( {\lambda _{2} + \mu _{1}} \right) \pi _{i + 3} = 0 , \end{aligned}$$

(51)

$$\begin{aligned} \lambda _{2}\pi _{i + 1} - \left( {\lambda _{2} + \mu _{2}} \right) \pi _{i + 4} + \mu _{1}\pi _{i + 6} + \mu _{2}\pi _{i + 7} + \beta \pi _{i + 8} = 0 , \end{aligned}$$

(52)

$$\begin{aligned} \lambda _{2}\pi _{i + 2} - \left( {\lambda _{2} + \beta } \right) \pi _{i + 5} = 0 , \end{aligned}$$

(53)

where $i=1,4,7\cdots$, then by iterating Eqs. 51 and 53 and combining Eqs. 48 and 50, we have

$$\begin{aligned} \pi _{i} = \frac{\lambda _{1} + \theta }{\lambda _{2} + \mu _{1}}\left( \frac{\lambda _{2}}{\lambda _{2} + \mu _{1}} \right) ^{\frac{i - 1}{3}}\pi _{0} , \end{aligned}$$

(54)

$$\begin{aligned} \pi _{i+2}=\frac{\alpha }{\lambda _2+\beta }\left( \frac{\lambda _2}{\lambda _2+\beta }\right) ^\frac{i-1}{3}\pi _0 . \end{aligned}$$

(55)

From Eqs. 54 and 55, we can derive

$$\begin{aligned} {\pi _1} + {\pi _4} + {\pi _7} + \cdots = \frac{{{\lambda _1} + \theta }}{{{\mu _1}}}{\pi _0}, \end{aligned}$$

(56)

$$\begin{aligned} {\pi _3} + {\pi _6} + {\pi _9} + \cdots = \frac{\alpha }{\beta }{\pi _0}\mathrm{{\;}}. \end{aligned}$$

(57)

Rearranging Eqs. 49 and 52 yields

$$\begin{aligned} {\left\{ \begin{array}{ll} {\lambda _2}\left( {{\pi _0} - {\pi _2}} \right) = {\mu _2}\left( {{\pi _2} - {\pi _5}} \right) - {\mu _1}{\pi _4} - \beta {\pi _6}\\ {\lambda _2}\left( {{\pi _2} - {\pi _5}} \right) = {\mu _2}\left( {{\pi _5} - {\pi _8}} \right) - {\mu _1}{\pi _7} - \beta {\pi _9}\\ {\lambda _2}\left( {{\pi _5} - {\pi _8}} \right) = {\mu _2}\left( {{\pi _8} - {\pi _{11}}} \right) - {\mu _1}{\pi _{10}} - \beta {\pi _{12}}\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\cdots \end{array}\right. } \end{aligned}$$

(58)

Then, summed up the equations in Eq. 58 sequentially in the order from top to bottom, we get

$$\begin{aligned} \pi _{2} = \rho _{2}\pi _{0} + \frac{\mu _{1}}{\mu _{2}}\left( {\pi _{4} + \pi _{7} + \cdots } \right) + \frac{\beta }{\mu _{2}}\left( {\pi _{6} + \pi _{9} + \cdots } \right) , \end{aligned}$$

(59)

$$\begin{aligned} {\pi _{i + 1}} = {\rho _2}{\pi _{i - 2}} + \frac{{{\mu _1}}}{{{\mu _2}}}\left( {{\pi _{i + 3}} + {\pi _{i + 6}} + \cdots } \right) + \frac{\beta }{{{\mu _2}}}\left( {{\pi _{i + 5}} + {\pi _{i + 8}} + \cdots } \right) , \end{aligned}$$

(60)

where ${\rho _2} = \frac{{{\lambda _2}}}{{{\mu _2}}}$ and $i=4,7\cdots$. Next, summing all the equations in Eqs. 59 and 60, then combining Eqs. 54 and 55, we have

$$\begin{aligned} \ \pi _0+\pi _2+\pi _5+\cdots =\frac{\pi _0\mu _2}{\mu _2-\lambda _2}\left( 1+\frac{\lambda _2\left( \lambda _1+\theta \right) }{\mu _1\mu _2}+\frac{\lambda _2\alpha }{\mu _2\beta }\right) , \end{aligned}$$

(61)

Notice that ${\pi e}=1$, then combining Eqs. 56, 57 and 61, we get

$$\begin{aligned} \left( {\frac{{{\lambda _1} + \theta }}{{{\mu _1}}} + \frac{\alpha }{\beta }} \right) {\pi _0} + \left( {\frac{{{\mu _2}}}{{{\mu _2} - {\lambda _2}}} + \frac{{{\lambda _2}\left( {{\lambda _1} + \theta } \right) }}{{{\mu _1}\left( {{\mu _2} - {\lambda _2}} \right) }} + \frac{{{\lambda _2}\alpha }}{{\beta \left( {{\mu _2} - {\lambda _2}} \right) }}} \right) {\pi _0} = 1. \end{aligned}$$

(62)

That is

$$\begin{aligned} {\pi _0} = \frac{{{\mu _1}\beta \left( {{\mu _2} - {\lambda _2}} \right) }}{{{\mu _1}{\mu _2}\beta + {\mu _2}\beta \left( {{\lambda _1} + \theta } \right) + {\mu _1}{\mu _2}\alpha }}. \end{aligned}$$

(63)

Then using the inequality $\pi Ce < \pi Ae$, we obtain $\frac{{{\lambda _1}}}{{{\lambda _1} + \theta }} < {\pi _0}$. Combining with Eq. 63 yields Theorem 1.