Asymptotic expansion of Toeplitz determinants of an indicator function with discrete rotational symmetry and powers of random unitary matrices

Abstract

In this short article, we propose a full large N asymptotic expansion of the probability that the $m{\text {th}}$ power of a random unitary matrix of size N has all its eigenvalues in a given arc-interval centered in 1 when N is large. This corresponds to the asymptotic expansion of a Toeplitz determinant whose symbol is the indicator function of several intervals having a discrete rotational symmetry. This solves and improves a conjecture left opened by the author in Marchal (Lett Math Phys 110:211–258, 2020). It also provides a rare example of the explicit computation of a full asymptotic expansion of a genus $g>0$ classical spectral curve, including the oscillating non-perturbative terms, using the topological recursion.

Appendix A: Topological recursion details in the one-cut case

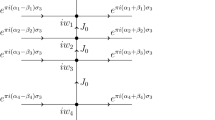
In this section, we list the intermediate steps obtained in the computation of the topological recursion of [25] applied to the classical spectral curve of genus 0 given by (2.6) that may be parametrized globally as:

$$\begin{aligned} x(z)= & {} \frac{1}{2}\tan \left( \frac{\pi \epsilon }{2}\right) \left( z+\frac{1}{z}\right) \nonumber \\ y(z)= & {} \frac{8 z^3}{\sin \left( \frac{\pi \epsilon }{2}\right) (z-1)(z+1) \left( \left( \tan ^2 \frac{\pi \epsilon }{2}\right) z^4+2\left( \tan ^2 \frac{\pi \epsilon }{2}+2\right) z^2+ \tan ^2 \frac{\pi \epsilon }{2}\right) }\nonumber \\ \end{aligned}$$

(A.1)

with the global involution $\bar{z}=\frac{1}{z}$. The parametrization implies that there are two simple branchpoints at $z=1$ and $z=-1$. The definition of the topological recursion in this particular case is presented in Appendix B of [37]. It provides the following intermediate steps:

$$\begin{aligned} \omega _2^{(0)}(z_1,z_2)= & {} \frac{dz_1 \, dz_2}{(z_1-z_2)^2}\nonumber \\ \omega _{1}^{(1)}(z)= & {} \frac{z }{2(z-1)^2(z+1)^2 \cos \frac{\pi \epsilon }{2} }\nonumber \\ \omega _{3}^{(0)}(z)= & {} 0\nonumber \\ \omega _{4}^{(0)}(z)= & {} 0\nonumber \\ \omega _{2}^{(1)}(z_1,z_2)= & {} \frac{z_1^2z_2^2+z_1^2+z_2^2+4z_1z_2+1}{4 \left( \cos \frac{\pi \epsilon }{2}\right) ^2 (z_1-1)^2(z_1+1)^2(z_2-1)^2(z_2+1)^2} \nonumber \\ \omega _1^{(2)}(z)= & {} \frac{z\left( \left( 13\tan ^2 \frac{\pi \epsilon }{2} +4\right) z^4+\left( 10\tan ^2 \frac{\pi \epsilon }{2} +28\right) z^2+13\tan ^2 \frac{\pi \epsilon }{2} +4\right) }{32 \left( \cos \frac{\pi \epsilon }{2}\right) (z+1)^4(z-1)^4}\nonumber \\ \omega _3^{(1)}(z_1,z_2,z_3)= & {} \frac{(z_1z_2+z_1z_3+z_2z_3+1)(z_1z_2z_3+z_1+z_2+z_3)}{\left( \cos \frac{\pi \epsilon }{2}\right) ^3(z_1^2-1)^2(z_2^2-1)^2(z_3^2-1)^2}\nonumber \\ \omega _1^{(3)}(z)= & {} \frac{z}{\left( \cos \frac{\pi \epsilon }{2}\right) (z^2-1)^6}\Big (\left( 413 \tan ^4 \frac{\pi \epsilon }{2}+268 \tan ^2 \frac{\pi \epsilon }{2}+8 \right) (z^8+1)\nonumber \\{} & {} +\left( 580 \tan ^4 \frac{\pi \epsilon }{2}+1880 \tan ^2 \frac{\pi \epsilon }{2}+688\right) (z^6+z^2)\nonumber \\{} & {} +\left( 1614 \tan ^4 \frac{\pi \epsilon }{2}+2904 \tan ^2 \frac{\pi \epsilon }{2}+2208\right) z^4\Big )\nonumber \\ \end{aligned}$$

(A.2)