Skip to main content
SpringerLink
Log in

Quantum Gaussian maximizers and log-Sobolev inequalities

  • Published:
Letters in Mathematical Physics Aims and scope Submit manuscript

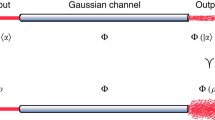
Abstract

In the present paper, we give a proof of optimality of Gaussian encodings for the ultimate communication rate of generalized noisy homodyne and heterodyne receivers under the oscillator energy constraint, without any additional “threshold condition” onto the signal power. Rather remarkably, the proof is based on various generalizations of the celebrated log-Sobolev inequality motivated by a solution of the problem of Gaussian maximizers for the capacity of quantum measurement channels.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Data availability

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

Notes

  1. The fact that the log-Sobolev inequality implies Lieb’s bound for the Wehrl entropy was observed by Carlen [1], see also [25]. Another related result [3] concerns minimization of the Wehrl entropy with a fixed entropy. We are indebted to the referee who pointed out these references.

  2. See, e.g., [10, 13], for the mathematical treatment of expressions with the unbounded operators related to the bosonic position–momentum observables.

  3. We denote by Sp the trace of \(2s\times 2s\)-matrices as distinct from the trace of operators on \({\mathcal {H}}\).

  4. We denote by \(I_{s}\) the unit \(s\times s-\)matrix.

  5. Notably, the expression (36) is of the same type as the one obtained in [7] by optimizing the information from applying sharp position measurement to noisy optimally squeezed states (A.H. is indebted to M. J. W. Hall for this observation).

  6. The Wehrl inequality proved by Lieb [23] corresponds to \(\beta _{q}=\beta _{p}=1/2\) and gives the minimal entropy \(\ln 2\pi e\) attained on the coherent states.

References

  1. Carlen, E.A.: Some integral identities and inequalities for entire functions and their application to the coherent state transform. J. Funct. Anal. 97(1), 231–249 (1991). https://doi.org/10.1016/0022-1236(91)90022-W

    Article  MathSciNet  MATH  Google Scholar 

  2. Caves, C.M., Drummond, P.D.: Quantum limits on bosonic communication rates. Rev. Mod. Phys. 66(2), 481–537 (1994). https://doi.org/10.1103/RevModPhys.66.481

    Article  ADS  Google Scholar 

  3. De Palma, G.: Uncertainty relations with quantum memory for the Wehrl entropy. Lett. Math. Phys. 108(9), 2139–2152 (2018). https://doi.org/10.1007/s11005-018-1067-y

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Giovannetti, V., Holevo, A.S., Garcia-Patron, R.: A solution of Gaussian optimizer conjecture for quantum channels. Commun. Math. Phys. 334(3), 1553–1571 (2015). https://doi.org/10.1007/s00220-014-2150-6

    Article  ADS  MathSciNet  MATH  Google Scholar 

  5. Giovannetti, V., Holevo, A.S., Mari, A.: Majorization and additivity for multimode bosonic Gaussian channels. Theor. Math. Phys. 182(2), 284–293 (2015). https://doi.org/10.1007/s11232-015-0262-6

    Article  MathSciNet  MATH  Google Scholar 

  6. Gross, L.: Logarithmic Sobolev inequalities. Am. J. Math. 97(4), 1061–1083 (1975). https://doi.org/10.2307/2373688

    Article  MathSciNet  MATH  Google Scholar 

  7. Hall, M.J.W.: Gaussian noise and quantum-optical communication. Phys. Rev. A 50, 3295–3303 (1994). https://doi.org/10.1103/PhysRevA.50.3295

    Article  ADS  Google Scholar 

  8. Hall, M.J.W.: Information exclusion principle for complementary observables. Phys. Rev. Lett. 74, 3307–3311 (1995). https://doi.org/10.1103/PhysRevLett.74.3307

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Holevo, A.: On the classical capacity of general quantum Gaussian measurement. Entropy 23(3), 377 (2021). https://doi.org/10.3390/e23030377

    Article  ADS  MathSciNet  Google Scholar 

  10. Holevo, A.S.: Probabilistic and Statistical Aspects of Quantum Theory, 2nd edition. Edizioni Della Normale, Pisa, (2011). https://doi.org/10.1007/978-88-7642-378-9

  11. Holevo, A.S.: Information capacity of a quantum observable. Probl. Inform. Transm. 48(1), 1–10 (2012). https://doi.org/10.1134/S0032946012010012

    Article  MathSciNet  MATH  Google Scholar 

  12. Holevo, A.S.: On the constrained classical capacity of infinite-dimensional covariant quantum channels. J. Math. Phys. 57(1), 015203 (2016). https://doi.org/10.1063/1.4928050

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. Holevo, A.S.: Quantum Systems, Channels, Information: A Mathematical Introduction, 2nd edition. De Gruyter, Berlin/Boston, (2019). https://doi.org/10.1515/9783110642490

  14. Holevo, A.S.: Gaussian maximizers for quantum Gaussian observables and ensembles. IEEE Trans. Inform. Theory 66(9), 5634–5641 (2020). https://doi.org/10.1109/TIT.2020.2987789

    Article  MathSciNet  MATH  Google Scholar 

  15. Holevo, A.S.: Accessible information of a general quantum Gaussian ensemble. J. Math. Phys. 62(9), 092201 (2021). https://doi.org/10.1063/5.0048112

    Article  ADS  MathSciNet  MATH  Google Scholar 

  16. Holevo, A.S.: Structure of a general quantum Gaussian observable. Proc. Steklov Inst. Math. 313, 70–77 (2021). https://doi.org/10.1134/S0081543821020085

    Article  MathSciNet  MATH  Google Scholar 

  17. Holevo, A.S.: Log-Sobolev inequality and proof of Hypothesis of the Gaussian Maximizers for the capacity of quantum noisy homodyning, (2022). Preprint at https://arxiv.org/abs/2204.10626

  18. Holevo, A.S., and Filippov, S.N.: Proof of the Gaussian maximizers conjecture for the communication capacity of noisy heterodyne measurements, (2022). Preprint at https://arxiv.org/abs/2206.02133

  19. Holevo, A.S., Kuznetsova, A.A.: Information capacity of continuous variable measurement channel. J. Phys. A: Math. Theor. 53(17), 175304 (2020). https://doi.org/10.1088/1751-8121/ab7df8

    Article  ADS  MathSciNet  MATH  Google Scholar 

  20. Holevo, A.S., Yashin, V.I.: Maximum information gain of approximate quantum position measurement. Quant. Inf. Process. 20, 97 (2021). https://doi.org/10.1007/s11128-021-03046-8

    Article  ADS  MathSciNet  MATH  Google Scholar 

  21. Horodecki, M., Shor, P.W., Ruskai, M.B.: Entanglement breaking channels. Rev. Math. Phys. 15(6), 629–641 (2003). https://doi.org/10.1142/S0129055X03001709

    Article  MathSciNet  MATH  Google Scholar 

  22. Lee, J., Ji, S.-W., Park, J., Nha, H.: Gaussian benchmark for optical communication aiming towards ultimate capacity. Phys. Rev. A 93, 050302 (2016). https://doi.org/10.1103/PhysRevA.93.050302

    Article  ADS  Google Scholar 

  23. Lieb, E.H.: Proof of an entropy conjecture of Wehrl. Commun. Math. Phys. 62, 35–41 (1978). https://doi.org/10.1007/BF01940328

    Article  ADS  MathSciNet  MATH  Google Scholar 

  24. Lieb, E.H., and Loss, M.: Analysis. Am. Math. Soc., (2001). Section 8.14

  25. Luo, S.: A simple proof of Wehrl’s conjecture on entropy. J. Phys. A: Math. Gen. 33(16), 3093 (2000). https://doi.org/10.1088/0305-4470/33/16/303

    Article  ADS  MathSciNet  MATH  Google Scholar 

  26. Mari, A., Giovannetti, V., Holevo, A.S.: Quantum state majorization at the output of bosonic Gaussian channels. Nat. Commun. 5(1), 1–5 (2014). https://doi.org/10.1038/ncomms4826

    Article  Google Scholar 

  27. Schäfer, J., Karpov, E., García-Patrón, R., Pilyavets, O.V., Cerf, N.J.: Equivalence relations for the classical capacity of single-mode Gaussian quantum channels. Phys. Rev. Lett. 111, 030503 (2013). https://doi.org/10.1103/PhysRevLett.111.030503

    Article  ADS  Google Scholar 

  28. Serafini, A.: Quantum Continuous Variables: A Primer of Theoretical Methods. CRC Press, Taylor & Francis Group, Boca Raton, (2017). https://doi.org/10.1201/9781315118727

  29. Shirokov, M.E.: The Holevo capacity of infinite dimensional channels and the additivity problem. Commun. Math. Phys. 262(1), 137–159 (2006). https://doi.org/10.1007/s00220-005-1457-8

    Article  ADS  MathSciNet  MATH  Google Scholar 

  30. Shirokov, M.E.: On properties of quantum channels related to their classical capacity. Theory Probab. Appl. 52(2), 250–276 (2008). https://doi.org/10.1137/S0040585X97982980

    Article  MathSciNet  MATH  Google Scholar 

  31. Shor, P.W.: Additivity of the classical capacity of entanglement-breaking quantum channels. J. Math. Phys. 43(9), 4334–4340 (2002). https://doi.org/10.1063/1.1498000

    Article  ADS  MathSciNet  MATH  Google Scholar 

  32. Takeoka, M., Guha, S.: Capacity of optical communication in loss and noise with general quantum Gaussian receivers. Phys. Rev. A 89, 042309 (2014). https://doi.org/10.1103/PhysRevA.89.042309

    Article  ADS  Google Scholar 

Download references

Acknowledgements

The work was supported by the grant of Russian Science Foundation 19-11-00086, https://rscf.ru/project/19-11-00086/.

Author information

Authors and Affiliations

  1. Steklov Mathematical Institute of Russian Academy of Sciences, 8 Gubkina St., Moscow, Russia, 119991

    Alexander S. Holevo & Sergey N. Filippov

Authors
  1. Alexander S. Holevo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sergey N. Filippov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexander S. Holevo.

Ethics declarations

Conflict of interest

On behalf of all authors, the corresponding author states that there is no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Holevo, A.S., Filippov, S.N. Quantum Gaussian maximizers and log-Sobolev inequalities. Lett Math Phys 113, 10 (2023). https://doi.org/10.1007/s11005-023-01634-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11005-023-01634-6

Keywords

Mathematics Subject Classification

Search

Navigation