Pure bending in non-linear micropolar elasticity

Abstract

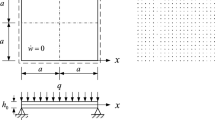
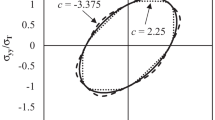
Large-deformation pure cylindrical bending of a homogeneous strip of uniform finite thickness with micropolar (Cosserats’) material constitution is analysed and related both to the large-deformation solution of classical (Cauchy’s) continuum theory and that of the small-deformation (linear) micropolar theory, known to exhibit a size effect. For a micropolar generalisation of the semi-linear material, the closed-form solution is obtained using a semi-inverse approach, which is equivalent to the solution in classical elasticity, possessing the same relationship between the curvature and the distribution of stress components and exhibiting the same softening effect relative to the linear solution. A couple stress of constant amount over the cross section develops in addition, in proportion to the curvature and the squared ratio between the characteristic bending length of the material and the thickness of the specimen and linear in Poisson’s ratio, in the same way as in the linear micropolar elasticity. The compound effect of non-linear softening and micropolar stiffening is analysed in detail with the view of providing a theoretical background for development of architected materials with internal structure for large-displacement applications. In particular, the size-effect estimate supplied by the linear analysis is shown to be in error, which builds up rapidly in highly deformed materials with relatively large characteristic bending length.

Appendix: Solution in small-deformation regime

Since $\zeta = \frac{h}{2} \frac{m_0}{\omega }$ and $\zeta = \frac{1}{2} \ln \frac{1+{\bar{p}}_0}{1-{\bar{p}}_0} = {\bar{p}}_0 + \frac{1}{3} {\bar{p}}_0^3 + \frac{1}{5} {\bar{p}}_0^5 + \mathcal{O} \left( {\bar{p}}_0^7 \right)$, in the linear case there follows $\zeta = {\bar{p}}_0$ and $\frac{m_0}{p_0} = \frac{2\omega }{{\tilde{E}}h}$. Next, the coefficient $\kappa = 6 \frac{\sinh 2 \zeta - 2 \zeta }{(2\zeta )^2\sinh 2 \zeta } \frac{\tanh \zeta }{\zeta }$ in (19) may be expanded as $\kappa = 6 \frac{2 \zeta + \frac{(2\zeta )^3}{6} + \frac{(2\zeta )^5}{120} + \mathcal{O} (\zeta ^7) - 2 \zeta }{(2\zeta )^2 \left[ 2 \zeta + \frac{(2\zeta )^3}{6} + \frac{(2\zeta )^5}{120} + \mathcal{O} (\zeta ^7) \right] } \frac{\zeta - \frac{\zeta ^3}{3} + \mathcal{O} (\zeta ^5)}{\zeta } = 1 - \frac{4\zeta ^2}{5} + \mathcal{O} (\zeta ^4)$, and thus $\lim _{\zeta \rightarrow 0} M = p_0 W \left( 1 + \frac{12\omega }{{\tilde{E}} h^2} \right) = m_0 A \left( 1+ \frac{{\tilde{E}} h^2}{12 \omega } \right)$. Conversely,

$$\begin{aligned} \zeta = \frac{1}{1+\phi } \frac{M}{{\tilde{E}} W} , \quad p_0 = \frac{1}{1+\phi } \frac{M}{W} , \quad m_0 = \frac{\phi }{1+\phi } \frac{M}{A} , \end{aligned}$$

(A.1)

with $\phi = 24 (1-n) \left( \frac{l_b}{h} \right) ^2$ introduced in (20). For $\zeta \rightarrow 0$, $\cosh \eta = 1$, $\sinh \eta = \frac{2 \zeta X^2}{h}$ and $e^{-\eta } = 1 - \frac{2 \zeta X^2}{h}$, thus the strain and stress components in (22) turn into

$$\begin{aligned} E_B^{11}= & {} - \zeta \frac{2 X^2}{h} = - \frac{1}{1+\phi } \frac{M X^2}{{\tilde{E}} I} , \nonumber \\ B^{11}= & {} - {\tilde{E}} \zeta \frac{2 X^2}{h} = - \frac{1}{1+\phi } \frac{M X^2}{I} , \nonumber \\ E_B^{22}= & {} a \zeta \frac{2 X^2}{h} = \frac{a}{1+\phi } \frac{M X^2}{{\tilde{E}} I} , B^{22} = 0 , \nonumber \\ K^{31}= & {} \frac{2 \zeta }{h} = \frac{1}{1+\phi } \frac{M}{{\tilde{E}} I} , G^{31} = 2 \frac{\omega }{h} \zeta = \frac{\phi }{1+\phi } \frac{M}{A} , \nonumber \\ B^{33}= & {} - n {\tilde{E}} \zeta \frac{2 X^2}{h} = - \frac{n}{1+\phi } \frac{M X^2}{I} , \nonumber \\ G^{13}= & {} 2 \frac{\omega }{h} (\tau -1) \zeta = \frac{\phi (\tau -1)}{1+\phi } \frac{M}{A} . \end{aligned}$$

(A.2)

Turning to the deformed geometry, since $\cosh \eta = 1 + \frac{\eta ^2}{2} + \mathcal{O} \left( \zeta ^4 \right)$, $\sinh \eta = \eta + \mathcal{O} \left( \zeta ^3 \right)$ and $\cosh \zeta = 1 + \frac{\zeta ^2}{2} + \mathcal{O} \left( \zeta ^4 \right)$, from radial position (13) we obtain $\frac{r}{\rho }= 1 + a - \frac{\eta + \mathcal{O} \left( \zeta ^3 \right) + a \left[ 1 + \frac{\eta ^2}{2} + \mathcal{O} \left( \zeta ^4 \right) \right] }{1 + \frac{\zeta ^2}{2} + \mathcal{O} \left( \zeta ^4 \right) } = 1 - \eta + \frac{a}{2} \left( \zeta ^2 - \eta ^2 \right) + \mathcal{O} \left( \zeta ^3 \right)$, while of course, $\theta = \frac{2\zeta }{h} X^1$. In addition, $\cos \xi = 1 - \frac{\xi ^2}{2} + \mathcal{O} \left( \zeta ^4 \right)$ and $\sin \xi = \xi + \mathcal{O} \left( \zeta ^3 \right)$, thus from (15), $X^1 = \rho \xi$ and $\eta = \frac{2 X^2}{h} \zeta$ we have

$$\begin{aligned} x^1&= \rho \left[ 1 - \eta + \frac{a}{2} \left( \zeta ^2 - \eta ^2 \right) + \mathcal{O} \left( \zeta ^3 \right) \right] \left[ \xi + \mathcal{O} \left( \zeta ^3 \right) \right] \\&= \left( 1 - \frac{2 X^2}{h} \zeta \right) X^1 + \mathcal{O} \left( \zeta ^2 \right) \\ x^2&= \rho - \rho \left[ 1 - \eta + \frac{a}{2} \left( \zeta ^2 - \eta ^2 \right) + \mathcal{O} \left( \zeta ^3 \right) \right] \left[ 1 - \frac{\xi ^2}{2} + \mathcal{O} \left( \zeta ^4 \right) \right] \\&= X^2 - \frac{\zeta }{h} \left( a \frac{h^2}{4} - a X^2 X^2 - X^1 X^1 \right) + \mathcal{O} \left( \zeta ^2 \right) . \end{aligned}$$

Using (3), for $\zeta ^2 \rightarrow 0$ the displacements $u^1 = x^1 - x^1_0$ and $u^2 = x^2 - x^2_0$ become

$$\begin{aligned} u^1 = - \frac{2 \zeta }{h} X^1 X^2 \quad \mathrm{and} \quad u^2 = \frac{\zeta }{h} \left( a X^2 X^2 + X^1 X^1 \right) , \end{aligned}$$

since $X^2_e = \frac{h}{4} a \zeta + \mathcal{O} \left( \zeta ^2 \right)$. The last result follows from $r(X^2_e)=\rho$, i.e. as a solution of the quadratic equation $\left( \frac{2 \zeta X^2_e}{h} \right) ^2 + \frac{2}{a} \frac{2 \zeta X^2_e}{h} - \zeta ^2 = \mathcal{O} \left( \zeta ^3 \right)$ coming out of (13), which in this case gives $a \left( \cosh \zeta - \cosh \eta _e \right) = \sinh \eta _e$. Substitutions $\cosh \eta _e = 1 + \frac{1}{2} \eta _e^2 + \mathcal{O} \left( \zeta ^4 \right)$, $\sinh \eta _e = \eta _e + \mathcal{O} \left( \zeta ^3 \right)$ and $\eta _e = \frac{2 X^2_e}{h} \zeta$ are used in the derivation.

Finally, making use of (A.1), the displacement components and the rotation of a material point X are obtained as

$$\begin{aligned} u^1= & {} - \frac{M}{1+\phi } \frac{X^1 X^2}{{\tilde{E}} I} , \\ u^2= & {} \frac{M}{1+\phi } \frac{X^1 X^1 + a X^2 X^2}{2 {\tilde{E}} I} , \quad \theta = \frac{M}{1+\phi } \frac{X^1}{{\tilde{E}} I} , \end{aligned}$$

which correspond to the known linear results derived in Gauthier and Jahsman (1975), Grbčić et al. (2019). For $\phi =0$, these results, as well as those in (A.2), reduce to the classical solution (Timoshenko and Goodier 1951).