Skip to main content
SpringerLink
Log in

Short-Wavelength Diffraction by a Contour with Nonsmooth Curvature. Boundary Layer Approach

  • Published:
Journal of Mathematical Sciences Aims and scope Submit manuscript

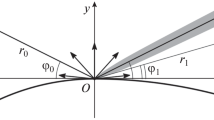
We consider the short-wave length diffraction by a contour with a nonsmooth curvature, whose jth derivative (j = 1, 2, . . .) has a discontinuity at a point. The asymptotic formulas describing the effect of curvature’s nonsmoothness on the wavefield are constructed in the framework of the rigorous boundary layer method. An expression for a cylindrical diffracted wave is derived. A description of the wavefield in a vicinity of the limit ray at a small distance from the contour is given in terms of the parabolic cylinder functions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. V. M. Babič and N. Ya. Kirpičnikova, The Boundary Layer Method in Diffraction Problems, Springer, Berlin (1979).

    Book  Google Scholar 

  2. V. M. Babich, M. A. Lyalinov, and V. E. Grikurov, Sommerfeld–Malyuzhinets Technique in Diffraction Theory, Springer, Berlin (2006).

    Google Scholar 

  3. V. H. Weston, “The effect of a discontinuity in curvature in high-frequency scattering,” IRE Trans. AP, 10, 775–780 (1962).

    Google Scholar 

  4. V. H. Weston, “Effect of a discontinuity of curvature in high-frequency scattering. Part II,” IEEE Trans. AP, 13, 611–613 (1965).

    Article  Google Scholar 

  5. T. B. A. Senior, “The diffraction matrix for a discontinuity in curvature,” IEEE Trans. AP, 20, No. 3, 326–333 (1972).

    Article  Google Scholar 

  6. A. S. Kirpichnikova, N. Ya. Kirpichnikova, and V. B. Philippov, “Diffraction of creeping waves by a line of jump of curvature (a three-dimensional acoustic medium),” J. Math. Sci., 108, No. 5, 689–702 (2002).

    Article  MathSciNet  Google Scholar 

  7. D. Bouche, “Courant sur un obstacle cylindrique parfaitement conducteur présentant une discontinuité de courbure,” Ann. Télécommunic., 47, 391–399 (1992).

    Article  Google Scholar 

  8. A. V. Popov, “Backscattering from a line of jump of curvature,” in: Trudy V Vses. Sympos. Diffr. Raspr. Voln, Nauka, Leningrad (1971), pp. 171–175.

  9. G. L. James, Geometrical theory of diffraction for electromagnetic waves, IEEE Electromagnetic Series 1, London (1986).

  10. A. F. Filippov, “Reflection of a wave from a boundary composed of arcs of variable curvature,” J. Appl. Math. Mech. (PMM), 34, No. 6, 1014–1023 (1970).

    Article  Google Scholar 

  11. L. Kaminetzky and J. B. Keller. “Diffraction coefficients for higher order edges and vertices,” SIAM J. Appl. Math., 22, No. 1, 109–134 (1972).

    Article  MathSciNet  Google Scholar 

  12. Z. M. Rogoff and A. P. Kiselev, “Diffraction at jump of curvature on an impedance boundary,” Wave Motion, 33, No. 2, 183–208 (2001).

    Article  Google Scholar 

  13. A. Michaeli, “Diffraction by a discontinuity in curvature including the effect of creeping wave,” IEEE Trans. AP, 38, No. 6, 929–931 (1990).

    Article  Google Scholar 

  14. V. A. Borovikov and B. E. Kinber, Geometrical Theory of Diffraction, Institute of Electrical Engineers, London (1994).

    Book  Google Scholar 

  15. E. A. Zlobina and A. P. Kiselev, “Boundary-layer approach to high-frequency diffraction by a jump of curvature,” Wave Motion, 96, 102571 (2020).

    Article  MathSciNet  Google Scholar 

  16. E. A. Zlobina and A. P. Kiselev, “Short-wavelength diffraction by a contour with Höldertype singularity in curvature,” St. Petersburg Math. J., 33, No. 2, 207–222 (2022).

    Article  MathSciNet  Google Scholar 

  17. N. V. Tsepelev, “Some special solutions of the Helmholtz equation,” J. Sov. Math., 11, No. 3, 497–501 (1979).

    Article  Google Scholar 

  18. G. E. Shilov and I. M. Gelfand, Generalized Functions: Properties and Operations, Vol. 1, Academic Press, New York & London (1968).

    Google Scholar 

  19. V. I. Smirnov, A Course of Higher Mathematics, Vol. 2, Pergamon Press, Oxford (1964).

    Google Scholar 

  20. V. M. Babich and A. P. Kiselev, Elastic Waves: High-Frequency Theory, Chapman & Hall/CRC, London (2018).

    Book  Google Scholar 

  21. L. M. Brekhovskikh, Waves in Layered Media, Academic Press, New York (1976).

    Google Scholar 

  22. A. Erdélyi, Asymptotic Expansions, Dover Publications, New York (1956).

    Google Scholar 

  23. M. Abramowitz and I. A. Stegun, Handbook of Mathematical Functions, NBS (1964).

Download references

Author information

Authors and Affiliations

  1. St. Petersburg Department of Steklov Institute of Mathematics, St. Petersburg State University, St. Petersburg, Russia

    E. A. Zlobina

Authors
  1. E. A. Zlobina
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to E. A. Zlobina.

Additional information

Dedicated to V. M. Babich’s 90th anniversary

Translated from Zapiski Nauchnykh Seminarov POMI, Vol. 493, 2020, pp. 169–185.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zlobina, E.A. Short-Wavelength Diffraction by a Contour with Nonsmooth Curvature. Boundary Layer Approach. J Math Sci 277, 586–597 (2023). https://doi.org/10.1007/s10958-023-06865-5

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10958-023-06865-5

Search

Navigation