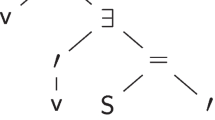This paper is a survey of some topics in computable structure theory, illustrating the power of certain infinitary sentences to describe mathematical structures and classes of structures. It includes classical results such as the Scott isomorphism theorem and the Lopez-Escobar theorem, and work of Friedman and Stanley on comparing classes of structures according to the complexity of their invariants. It also includes more recent results, in particular, on torsion-free Abelian groups.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
D. Scott, “Logic with denumerably long formulas and finite strings of quantifiers,” In: The Theory of Models. Proc. 1963 Int. Symp. Berkeley, pp. 329-341, North-Holland, Amsterdam (1965).
C. J. Ash and J. F. Knight, Computable Structures and the Hyperarithmetical Hierarchy, Elsevier, Amsterdam (2000).
A. Montalbán, Computable Structure Theory, Part II: Beyond the Arithmetic [Submitted for publication in 2022].
M. Vanden Boom, “The effective Borel hierarchy,” Fundam. Math. 195, No. 3, 269–289 (2007).
E. G. K. Lopez-Escobar, “An interpolation theorem for denumerably long formulas,” Fundam. Math. 57, 253–272 (1965).
R. L. Vaught, “Invariant sets in topology and logic,” Fundam. Math. 82, 269–294 (1974).
H. Friedman and L. Stanley, “A Borel reducibility theory for classes of countable structures,” J. Symb. Log. 54, No. 3, 894–914 (1989).
I. S. Lavrov, “Effective inseparability of the set of identically true formulae and finitely refutable formulas for certain elementary theories” [in Russian], Algebra Logic, 2, No. 1, 5–18 (1963).
A. Mekler, “Stability of nilpotent groups of class 2 and prime exponent,” J. Symb. Log. 46, 781–788 (1981).
D. Marker, Model Theory: An Introduction, Springer, New York, NY (2002).
A. Nies, “Undecidable fragments of elementary theories,” Algebra Univers. 35, No. 1, 8–33 (1996).
R. Camerlo and S. Gao, “The completeness of the isomorphism relation for countable Boolean algebras,” Trans. Am. Math. Soc. 353, No. 2, 491–518 (2001).
R. Miller, B. Poonen, H. Schoutens, and A. Shlapentokh, “A computable functor from graphs to fields,” J. Symb. Log. 83, No. 1, 326–348 (2018).
A. I. Mal’tsev, “On a correspondence between rings and groups” [in Russian], Mat. Sb. 50, 257–266 (1960); English transl.: Am. Math. Soc., Transl., II 45, 221–231 (1965).
R. Alvir, W. Calvert, G. Goodman, V. Harizanov, J. F. Knight, A. Morozov, R. Miller, A. Soskova, and R. Weisshaar, “Interpreting a field in its Heisenberg group,” J. Symb. Log. 87, No. 3, 1215–1230 (2022).
J. F. Knight, A. Soskova, and S. Vatev, “Coding in graphs and linear orderings,” J. Symb. Log. 85, No. 2, 673–690 (2020).
M. Harrison-Trainor and A. Montalbán, “The tree of tuples of a structure,” J. Symb. Log. 87, No. 1, 21–46 (2022).
W. Calvert, D. Cummins, J. F. Knight, and S. Miller, “Comparing classes of finite structures,” Algebra Logic 43, No. 6, 374–392 (2004).
J. F. Knight, S. Quinn, and M. Vanden Boom, “Turing computable embeddings,” J. Symb. Log. 72, No. 3, 901–918 (2007).
R. Baer, “Abelian groups without elements of finite order,” Duke Math. J. 3, 68–122 (1937).
G. Hjorth, “Around non-classifiability for countable torsion-free Abelian groups,” In:Abelian Groups and Modules, pp. 269–292., Birkh¨auser, Basel (1999).
S. Thomas, “The classification problem for torsion-free Abelian groups of finite rank,” J. Am. Math. Soc. 16, No. 1, 233–258 (2003).
A. G. Kurosh, “Primitive torsionsfreie abelsche Gruppen vom endlichen Range” [in German], Ann. Math. 38, 175–203 (1937).
L. Fuchs, Infinite Abelian Groups. Vol. II, Academic Press, New York etc. (1973).
S. Thomas and B. Velickovic, “On the complexity of the isomorphism relation for fields of finite transcendence degree,” J. Pure Appl. Algebra 159, No. 2-3, 347–363 (2001).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Knight, J.F. Classes of Algebraic Structures. J Math Sci 275, 16–24 (2023). https://doi.org/10.1007/s10958-023-06656-y
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10958-023-06656-y