Abstract
We study the recently introduced Inverse-Beta (IB) polymer, an exactly solvable, anisotropic finite temperature model of directed polymer on the square lattice, and obtain its stationary measure. In parallel we introduce an anisotropic zero temperature model of directed polymer on the square lattice, the Bernoulli–Geometric polymer, and obtain its stationary measure. This new exactly solvable model is dual to the IB polymer and interpolates between models of first and last passage percolation on the square lattice. Both stationary measures are shown to satisfy detailed balance. We also obtain the asymptotic mean value of (i) the free-energy of the IB polymer; (ii) the optimal energy of the Bernoulli–Geometric polymer. We discuss the convergence of both models to their stationary state. We perform simulations of the Bernoulli–Geometric polymer that confirm our results.











Similar content being viewed by others
Notes
Throughout the paper we will pay attention to emphasize the degree of rigor with which each result is shown, and in particular only fully rigorous results will be stated as Propositions.
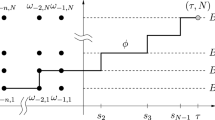
There \(c_\varphi = f_{\mathrm{IB}}(1/2 + \varphi , 1/2 - \varphi )\) for \(\varphi \, \in \, ]-1/2,1/2[\) and the equivalent of \(\lambda ^*\) there is the saddle-point parameter \(k_{\varphi } = \gamma /2 + \lambda ^*\).
Here \(u^{LG} = v^{LG}\) means that the random Boltzmann weights can equally well be interpreted as living on the vertices of the square lattice.
Although we note that this was indeed accomplished in [34] for the case of inhomogeneous last passage percolation models with on site geometric or exponential waiting times.
References
Huse, D.A., Henley, C.L.: Pinning and roughening of domain walls in Ising systems due to random impurities. Phys. Rev. Lett. 54, 27082711 (1985)
Kardar, M., Zhang, Y.-C.: Scaling of directed polymers in random media. Phys. Rev. Lett. 58(20), 1087–2090 (1987)
Kardar, M., Parisi, G., Zhang, Y.C.: Dynamic scaling of growing interfaces. Phys. Rev. Lett. 56, 889 (1986)
Spohn, H.: The Kardar–Parisi–Zhang equation—a statistical physics perspective. arXiv:1601.00499 (2016)
Halpin-Healy, T., Takeuchi, K.A.: A KPZ Cocktail–Shaken, not stirred: toasting 30 years of kinetically roughened surfaces. J. Stat. Phys. 160, 794–814 (2015). arXiv:1505.01910
Corwin, I.: The Kardar–Parisi–Zhang equation and universality class. arXiv:1106.1596 (2011)
Kardar, M.: Replica bethe ansatz studies of two-dimensional interfaces with quenched random impurities. Nucl. Phys. B 290, 582 (1987)
Huse, D., Henley, C.L., Fisher, D.S.: Huse, Henley, and Fisher respond. Phys. Rev. Lett. 55, 2924 (1985)
Forster, D., Nelson, D.R., Stephen, M.J.: Large-distance and long-time properties of a randomly stirred fluid. Phys. Rev. A 16, 732 (1977)
Johansson, K.: Shape fluctuations and random matrices. Commun. Math. Phys. 209, 437 (2000). arXiv:math/9903134
Kriecherbauer, T., Krug, J.: A pedestrians view on interacting particle systems, KPZ universality, and random matrices. J. Phys. A 43, 403001 (2010). arXiv:0803.2796
O’Connell, N., Yor, Marc: Brownian analogues of Burke’s theorem. Stoch. Process. Appl. 96(2), 285–304 (2001)
Seppäläinen, T., Valkó, B.: Bounds for scaling exponents for a 1 + 1 dimensional directed polymer in a Brownian environment. arXiv:1006.4864 (2010)
O’Connell, N.: Directed polymers and the quantum Toda lattice. Ann. Probab. 40(2), 437–458 (2012)
Seppäläinen, T.: Scaling for a one-dimensional directed polymer with boundary. Ann. Probab. 40, 19–73 (2012). arXiv:0911.2446
Corwin, I., O’Connell, N., Seppäläinen, T., Zygouras, N.: Tropical combinatorics and Whittaker functions. arXiv:1110.3489 (2011)
Thiery, T., Le Doussal, P.: Log-Gamma directed polymer with fixed endpoints via the replica Bethe Ansatz. J. Stat. Mech., P10018 (2014). arXiv:1406.5963
Corwin, I., Seppäläinen, T., Shen, H.: The strict-weak lattice polymer. J. Stat. Phys. 160(4), 1027–1053 (2015). arXiv:1409.1794
O’Connell, N., Ortmann, J.: Tracy–Widom asymptotics for a random polymer model with gamma-distributed weights. Electron. J. Probab. 20(25), 1–18 (2015). arXiv:1408.5326
Barraquand, G., Corwin, I.: Random-walk in Beta-distributed random environment. arXiv:1503.04117
Thiery, T., Le Doussal, P.: Exact solution for a random walk in a time-dependent 1D random environment: the point-to-point Beta polymer. arXiv:1605.07538
Thiery, T., Le Doussal, P.: On integrable directed polymer models on the square lattice. J. Phys. A 48, 46 (2015). arXiv:1506.05006
Balázs, M., Rassoul-Agha, F., Seppäläinen, T:. Fluctuation bounds for the stationary beta polymer. Work in preparation (2016)
Borodin, A., Corwin, I., Remenik, D.: Log-Gamma polymer free energy fluctuations via a Fredholm determinant identity. Commun. Math. Phys. 324(1), 215–232 (2013). arXiv:1206.4573
Calabrese, P., Le Doussal, P., Rosso, A.: Free-energy distribution of the directed polymer at high temperature. Eur. Phys. Lett. 90, 20002 (2010). arXiv:1002.4560
Dotsenko, V.: Bethe ansatz derivation of the Tracy–Widom distribution for one-dimensional directed polymers. Eur. Phys. Lett. 90, 20003 (2010)
Dotsenko, V.: Replica Bethe ansatz derivation of the Tracy-Widom distribution of the free energy fluctuations in one-dimensional directed polymers. J. Stat. Mech. P07010 (2010)
Dotsenko, V., Klumov, B.: Bethe ansatz solution for one-dimensional directed polymers in random media. J. Stat. Mech. P03022 (2010)
Comets, F., Nguyen, V.-L.: Localization in Log-Gamma polymers with boundaries. Probab. Theory Relat. Fields, 1–33 (2015). arXiv:1409.5754
Draief, M., Mairesse, J., O’Connell, N.: Queues, stores, and tableaux. J. Appl. Probab. 42(4), 1145–1167 (2005)
Burke, P.: The output of a queueing system. Oper. Res. 4, 699704 (1956)
Martin, J.B.: Batch queues, reversibility and first-passage percolation. Queueing Syst. 62(4), 411–427 (2009). arXiv:0902.2026
Georgiou, N., Seppäläinen, T.: Large deviation rate functions for the partition function in a Log-Gamma distributed random potential. Ann. Probab. 41(6), 4248–4286 (2013). arXiv:1110.3544
Emrah, E.: Limit shapes for inhomogeneous corner growth models with exponential and geometric weights. Electron. Commun. Probab. 21. arXiv:1502.06986 (2016)
Georgiou, N., Rassoul-Agha, F., Seppäläinen, T.: Variational formulas and cocycle solutions for directed polymer and percolation models. Commun. Math. Phys., 1–39 (2016). arXiv:1510.00859
Damron, M., Hanson, J.: Busemann functions and infinite geodesics in two-dimensional first-passage percolation. Commun. Math. Phys. 325(3), 917–963 (2012)
Georgiou, N., Rassoul-Agha, F., Seppäläinen, T., Yilmaz, A.: Ratios of partition functions for the Log-Gamma polymer. Ann. Probab. 43(5), 2282–2331 (2015). arXiv:1303.1229
K. Matveev, K., Petrov, L.: q-randomized Robinson–Schensted–Knuth correspondences and random polymers. arXiv:1504.00666 (2015)
Borodin, A., Petrov, L.: Nearest neighbor Markov dynamics on Macdonald processes. Adv. Math. (2016). doi:10.1016/j.aim.2016.03.016. arXiv:1305.5501
Corwin, I., Petrov, L.: The q-PushASEP: a new integrable model for traffic in 1+1 dimension. J. Stat. Phys. 160(4), 10051026 (2015). arXiv:1308.3124
Borodin, A., Corwin, I.: Discrete time q-TASEPs. Int. Math. Res. Notices (2), 499–537 (2015). arXiv:1305.2972
Povolotsky, A.M.: On the integrability of zero-range chipping models with factorized steady states. J. Phys. 46(46), 465205 (2013). arXiv:1308.3250
Acknowledgments
This paper would have never existed without the numerous discussions I had with Timo Seppäläinen, discussions during which he kindly took the time to explain to me the techniques and results developed and obtained by him and his coworkers for the Log-Gamma polymer. These were a great source of inspiration for this work. He also took an active part during the first stages of research on the stationary measure of the Inverse-Beta polymer and shared with me related new results on the Beta polymer [23]. I warmly thank him for that. I am also grateful to Guillaume Barraquand for many discussions and remarks on the existing mathematical literature, as well as to Francis Comets, Ivan Corwin, Thomas Gueudré, Vivien Lecomte, Jeremy Quastel and Leonid Petrov for interesting discussions. Last but not least, I would like to warmly thank Pierre Le Doussal who introduced me and taught me most of the things I know on this topic through multiple discussions and collaborations on related subjects. I also thank him for useful comments on a first version of this manuscript. I acknowledge the KITP in Santa Barbara for hospitality during the first stages of redaction of this work. This research was supported in part by the National Science Foundation under Grant No. NSF PHY11-25915.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1: Proof of the Properties of the Finite Temperature Reversibility-Stationarity Map
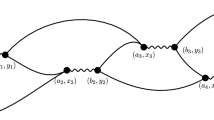
In this appendix we prove Propositions 3.1, 3.2 being trivial. We thus consider three independent random variables (U, V, W) distributed as in (2.7) and (2.3) and consider the RVs \((U',V',W') = \phi (U,V,W)\) as given in (3.3). The Jacobian of the transformation \((U,V,W) \rightarrow (U',V',W')\) is easily computed as, schematically,
The PDF of the triplet \((U',V',W')\) is then directly evaluated as
Where we introduced the PDF of the independent RVs (U, V, W) as noted in (2.7) and (2.3) and used the fact that \(\phi \) is an involution. It is then directly checked that
hence showing that \(U'\), \(V'\) and \(W'\) are independent and distributed as \(U' \sim U\), \(V' \sim V\) and \(W' \sim W\).
Appendix 2: Proof of the Properties of the Zero Temperature Stationarity Map
In this appendix we prove Propositions 4.1 and 4.2. Let us first prove the detailed balance property Proposition 4.2b. We thus consider \(\mathsf{U}\perp \mathsf{V}\perp (\mathsf{u}, \mathsf{v})\) distributed as in (2.12) and (2.20). Let us first compute the conditional probability
where \(k_{\mathsf{U}} \in \mathbb {N}\), \(k_{\mathsf{V}} \in {\mathbb Z}_-\), \(\mathsf{U}' = \mathrm{min}\left( \mathsf{u}, \mathsf{v}+ \mathsf{U}- \mathsf{V}\right) \) and \( \mathsf{V}' = \mathrm{min}\left( \mathsf{u}+\mathsf{V}-\mathsf{U}, \mathsf{v}\right) = \mathsf{U}' + \mathsf{V}- \mathsf{U}\). We have
Using this last expression and the expression of \(\textit{Proba}((\mathsf{U}, \mathsf{V})= (k_{\mathsf{U}} , k_{\mathsf{V}} ) )\) given in (2.22), we obtain
and it is then straightforward (although technically complicated due to the large number of terms) to check the detailed balance property Proposition 4.2. Namely one shows that the equality
holds. Let us emphasize here that this property is rather special: the fact that (7.7) works requires a large number of cancellation between terms that are made possible by the choice of only three parameters \(p_{\mathsf{U}} =\frac{1-q_b q'}{1-qq'}\), \(p_{\mathsf{V}}= \frac{1-q'}{1-q_b q'}\) and \(p_{\mathsf{u}\mathsf{v}}= \frac{1-q'}{1-qq'}\), a characteristic sign of the existence of exact solvability properties for the model. Finally, summing (7.7) on \(k_{\mathsf{U}'}\) and \(k_{\mathsf{V}'}\) gives the stationarity property Proposition 4.1:
Rights and permissions
About this article
Cite this article
Thiery, T. Stationary Measures for Two Dual Families of Finite and Zero Temperature Models of Directed Polymers on the Square Lattice. J Stat Phys 165, 44–85 (2016). https://doi.org/10.1007/s10955-016-1603-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-016-1603-z