Skip to main content
SpringerLink
Log in

Determinantal Martingales and Correlations of Noncolliding Random Walks

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

Abstract

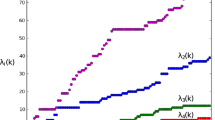
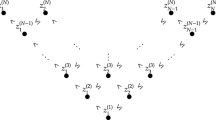
We study the noncolliding random walk (RW), which is a particle system of one-dimensional, simple and symmetric RWs starting from distinct even sites and conditioned never to collide with each other. When the number of particles is finite, \(N < \infty \), this discrete process is constructed as an \(h\)-transform of absorbing RW in the \(N\)-dimensional Weyl chamber. We consider Fujita’s polynomial martingales of RW with time-dependent coefficients and express them by introducing a complex Markov process. It is a complexification of RW, in which independent increments of its imaginary part are in the hyperbolic secant distribution, and it gives a discrete-time conformal martingale. The \(h\)-transform is represented by a determinant of the matrix, whose entries are all polynomial martingales. From this determinantal-martingale representation (DMR) of the process, we prove that the noncolliding RW is determinantal for any initial configuration with \(N < \infty \), and determine the correlation kernel as a function of initial configuration. We show that noncolliding RWs started at infinite-particle configurations having equidistant spacing are well-defined as determinantal processes and give DMRs for them. Tracing the relaxation phenomena shown by these infinite-particle systems, we obtain a family of equilibrium processes parameterized by particle density, which are determinantal with the discrete analogues of the extended sine-kernel of Dyson’s Brownian motion model with \(\beta =2\). Following Donsker’s invariance principle, convergence of noncolliding RWs to the Dyson model is also discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Andrews, G.E., Askey, R., Roy, R.: Special Functions. Cambridge University Press, Cambridge (1999)

    Book  MATH  Google Scholar 

  2. Baik, J.: Random vicious walks and random matrices. Commun. Pure Appl. Math. 53, 1385–1410 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  3. Baik, J., Suidan, T.M.: Random matrix central limit theorems for nonintersecting random walks. Ann. Probab. 35, 1807–1834 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  4. Ben Hough, J., Krishnapur, M., Peres, Y., Virág, B.: Zeros of Gaussian Analytic Functions and Determinantal Point Processes. American Mathematical Society, Providence (2009)

    Book  MATH  Google Scholar 

  5. Billingsley, P.: Convergence of Probability Measures. Wiley, New York (1968)

    MATH  Google Scholar 

  6. Cardy, J., Katori, M.: Families of vicious walkers. J. Phys. A 36, 609–629 (2003)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  7. Dyson, F.J.: A Brownian-motion model for the eigenvalues of a random matrix. J. Math. Phys. 3, 1191–1198 (1962)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  8. Eichelsbacher, P., König, W.: Ordered random walks. Electron. J. Probab. 13(46), 1307–1336 (2008)

    MATH  Google Scholar 

  9. Erdélyi, A. (ed.): Higher Transcendental Functions. Bateman Manuscript Project, vol. 3. McGraw-Hill, New York (1955)

    Google Scholar 

  10. Esaki, S.: Noncolliding system of continuous-time random walks. Pac. J. Math. Ind. 6(1), 1–10 (2014)

    Article  Google Scholar 

  11. Eynard, B., Mehta, M.L.: Matrices coupled in a chain: I. Eigenvalue correlations. J. Phys. A 31, 4449–4456 (1998)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  12. Feierl, T.: The height of watermelons with wall. J. Phys. A 45, 095003 (2012)

    Article  ADS  MathSciNet  Google Scholar 

  13. Feller, W.: An Introduction to Probability Theory and Its Applications, vol. II, 2nd edn. Wiley, New York (1966)

    MATH  Google Scholar 

  14. Fisher, M.E.: Walks, walls, wetting, and melting. J. Stat. Phys. 34, 667–729 (1984)

    Article  ADS  MATH  Google Scholar 

  15. Forrester, P.J.: Log-gases and Random Matrices. London Mathematical Society Monographs. Princeton University Press, Princeton (2010)

    Google Scholar 

  16. Fujita, T.: Stochastic Calculus for Finance (in Japanese). Kodansha, Tokyo (2002)

    Google Scholar 

  17. Fujita, T., Kawanishi, Y.: A proof of Ito’s formula using a discrete Ito’s formula. Stud. Sci. Math. Hungry 45, 125–134 (2008)

    MATH  MathSciNet  Google Scholar 

  18. Grabiner, D.J.: Brownian motion in a Weyl chamber, non-colliding particles, and random matrices. Ann. Inst. Henri Poincaré, Probab. Stat. 35, 177–204 (1999)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  19. Graczyk, P., Małecki, J.: Multidimensional Yamada–Watanabe theorem and its applications to particle systems. J. Math. Phys. 54, 021503/1-15 (2013)

  20. Harkness, W.L., Harkness, M.L.: Generalized hyperbolic secant distributions. J. Am. Stat. Assoc. 63, 329–337 (1968)

    MATH  MathSciNet  Google Scholar 

  21. Johansson, K.: Universality of the local spacing distribution in certain ensembles of Hermitian Wigner matrices. Commun. Math. Phys. 215, 683–705 (2001)

    Article  ADS  MATH  Google Scholar 

  22. Johansson, K.: Non-intersecting paths, random tilings and random matrices. Probab. Theory Relat. Fields 123, 225–280 (2002)

    Article  MATH  Google Scholar 

  23. Johansson, K.: Non-intersecting, simple, symmetric random walks and the extended Hahn kernel. Ann. Inst. Fourier 55, 2129–2145 (2005)

    Article  MATH  Google Scholar 

  24. Katori, M.: Determinantal martingales and noncolliding diffusion processes. Stoch. Process. Appl. 124, 3724–3768 (2014)

    Article  MATH  MathSciNet  Google Scholar 

  25. Katori, M.: Elliptic determinantal process of type A. Probab. Theory Relat. Fields (2014). doi:10.1007/s00440-014-0581-9

  26. Katori, M., Tanemura, H.: Functional central limit theorems for vicious walkers. Stoch. Stoch. Rep. 75, 369–390 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  27. Katori, M., Tanemura, H.: Symmetry of matrix-valued stochastic processes and noncolliding diffusion particle systems. J. Math. Phys. 45, 3058–3085 (2004)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  28. Katori, M., Tanemura, H.: Noncolliding Brownian motion and determinantal processes. J. Stat. Phys. 129, 1233–1277 (2007)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  29. Katori, M., Tanemura, H.: Zeros of Airy function and relaxation process. J. Stat. Phys. 136, 1177–1204 (2009)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  30. Katori, M., Tanemura, H.: Non-equilibrium dynamics of Dyson’s model with an infinite number of particles. Commun. Math. Phys. 293, 469–497 (2010)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  31. Katori, M., Tanemura, H.: Noncolliding squared Bessel processes. J. Stat. Phys. 142, 592–615 (2011)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  32. Katori, M., Tanemura, H.: Noncolliding processes, matrix-valued processes and determinantal processes. Sugaku Expos. (AMS) 24, 263–289 (2011)

    MathSciNet  Google Scholar 

  33. Katori, M., Tanemura, H.: Complex Brownian motion representation of the Dyson model. Electron. Commun. Probab. 18(4), 1–16 (2013)

    MathSciNet  Google Scholar 

  34. König, W.: Orthogonal polynomial ensembles in probability theory. Probab. Surv. 2, 385–447 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  35. König, W., O’Connell, N., Roch, S.: Non-colliding random walks, tandem queues, and discrete orthogonal polynomial ensembles. Electron. J. Probab. 7(1), 1–24 (2002)

    MathSciNet  Google Scholar 

  36. Krattenthaler, C.: Watermelon configurations with wall interaction: exact and asymptotic results. J. Phys. Conf. Ser. 42, 179–212 (2006)

    Article  ADS  Google Scholar 

  37. Levin, B.Y.: Lectures on Entire Functions. Translations of Mathematical Monographs, vol. 150. American Mathematical Society, Providence (1996)

    Google Scholar 

  38. Mehta, M.L.: Random Matrices, 3rd edn. Elsevier, Amsterdam (2004)

    MATH  Google Scholar 

  39. Nagao, T., Forrester, P.: Multilevel dynamical correlation functions for Dyson’s Brownian motion model of random matrices. Phys. Lett. A247, 42–46 (1998)

    Article  ADS  Google Scholar 

  40. Nagao, T., Forrester, P.J.: Vicious random walkers and a discretization of Gaussian random matrix ensembles. Nucl. Phys. B 620(FS), 551–565 (2002)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  41. Osada, H.: Infinite-dimensional stochastic differential equations related to random matrices. Probab. Theory Relat. Fields 153, 471–509 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  42. Osada, H.: Interacting Brownian motions in infinite dimensions with logarithmic potentials. Ann. Probab. 41, 1–49 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  43. Osada, H.: Interacting Brownian motions in infinite dimensions with logarithmic interaction potentials II: Airy random point field. Stoch. Process. Appl. 123, 813–838 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  44. Pérez-Abreu, V., Tudor, C.: Functional limit theorems for trace processes in a Dyson Brownian motion. Commun. Stoch. Anal. 1(3), 415–428 (2007)

    MATH  MathSciNet  Google Scholar 

  45. Privault, N.: Stochastic Analysis in Discrete and Continuous Settings. Lecture Notes in Mathematics, vol. 1982. Springer, Berlin (2009)

  46. Revuz, D., Yor, M.: Continuous Martingales and Brownian Motion, 3rd edn. Springer, New York (2005)

    MATH  Google Scholar 

  47. Sato, K.: Lévy Processes and Infinitely Divisible Distributions. Cambridge University Press, Cambridge (1999)

    MATH  Google Scholar 

  48. Schoutens, W.: Stochastic Processes and Orthogonal Polynomials. Lecture Notes in Statistics, vol. 146. Springer, New York (2000)

    Book  Google Scholar 

  49. Shirai, T., Takahashi, Y.: Random point fields associated with certain Fredholm determinants I: fermion, Poisson and boson point process. J. Funct. Anal. 205, 414–463 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  50. Soshnikov, A.: Determinantal random point fields. Russ. Math. Surv. 55, 923–975 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  51. Spohn, H.: Interacting Brownian particles: a study of Dyson’s model. In: Papanicolaou, G. (ed.) Hydrodynamic Behavior and Interacting Particle Systems, IMA Volumes in Mathematics and its Applications, vol. 9, pp. 151–179. Springer, Berlin (1987)

    Chapter  Google Scholar 

  52. Tao, T.: Topics in Random Matrix Theory. American Mathematical Society, Providence (2012)

    MATH  Google Scholar 

Download references

Acknowledgments

The present author would like to thank T. Shirai, H. Osada, H. Tanemura, and S. Esaki for useful discussions. This work is supported in part by the Grant-in-Aid for Scientific Research (C) (Nos. 21540397 and 26400405) of Japan Society for the Promotion of Science.

Author information

Authors and Affiliations

  1. Department of Physics, Faculty of Science and Engineering, Chuo University, Kasuga, Bunkyo-ku, Tokyo, 112-8551, Japan

    Makoto Katori

Authors
  1. Makoto Katori
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Makoto Katori.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Katori, M. Determinantal Martingales and Correlations of Noncolliding Random Walks. J Stat Phys 159, 21–42 (2015). https://doi.org/10.1007/s10955-014-1179-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-014-1179-4

Keywords

Search

Navigation