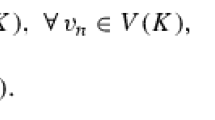Skip to main content
SpringerLink
Log in

The Nonconforming Virtual Element Method with Curved Edges

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

We introduce a nonconforming virtual element method for the Poisson problem on domains with fixed curved boundary and internal interfaces. We prove arbitrary order optimal convergence in the energy and \(L^2\) norms, and assess the theoretical results with numerical experiments. The proposed scheme has the upside that it can be designed and analyzed in any dimension.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Data Availability

The datasets generated during and/or analysed during the current study are available on request.

References

  1. Ahmad, B., Alsaedi, A., Brezzi, F., Marini, L.D., Russo, A.: Equivalent projectors for virtual element methods. Comput. Math. Appl. 66(3), 376–391 (2013)

    Article  MathSciNet  Google Scholar 

  2. Anand, A., Ovall, J.S., Reynolds, S.E., Weißer, S.: Trefftz finite elements on curvilinear polygons. SIAM J. Sci. Comput. 42(2), A1289–A1316 (2020)

    Article  MathSciNet  Google Scholar 

  3. Bertoluzza, S., Pennacchio, M., Prada, D.: High order VEM on curved domains. Atti Accad. Naz. Lincei Rend. Lincei Mat. Appl. 30(2), 391–412 (2019)

    MathSciNet  Google Scholar 

  4. Botti, L., Di Pietro, D.: Assessment of hybrid high-order methods on curved meshes and comparison with discontinuous Galerkin methods. J. Comput. Phys. 370, 58–84 (2018)

    Article  MathSciNet  Google Scholar 

  5. Bramble, J.H., Dupont, T., Thomée, V.: Projection methods for Dirichlet’ problem in approximating polygonal domains with boundary-value corrections. Math. Comput. 26(120), 869–879 (1972)

    MathSciNet  Google Scholar 

  6. Brenner, S.C.: Poincaré-Friedrichs inequalities for piecewise \({H}^1\) functions. SIAM J. Numer. Anal. 41(1), 306–324 (2003)

    Article  MathSciNet  Google Scholar 

  7. Brenner, S.C., Scott, L.: The Mathematical Theory of Finite Element Methods. Springer, Berlin (2008)

    Book  Google Scholar 

  8. Burman, E., Cicuttin, M., Delay, G., Ern, A.: An unfitted hybrid high-order method with cell agglomeration for elliptic interface problems. SIAM J. Sci. Comput. 43(2), A859–A882 (2021)

    Article  MathSciNet  Google Scholar 

  9. Burman, E., Ern, A.: A cut cell hybrid high-order method for elliptic problems with curved boundaries. In: European Conference on Numerical Mathematics and Advanced Applications, pp. 173–181. Springer (2019)

  10. Burman, E., Hansbo, P., Larson, M.: A cut finite element method with boundary value correction. Math. Comput. 87(310), 633–657 (2018)

    Article  MathSciNet  Google Scholar 

  11. Chen, L., Huang, J.: Some error analysis on virtual element methods. Calcolo 55(5), 1–23 (2018)

    MathSciNet  Google Scholar 

  12. Cockburn, B., Di Pietro, D.A., Ern, A.: Bridging the hybrid high-order and hybridizable discontinuous Galerkin methods. ESAIM Math. Model. Numer. Anal. 50(3), 635–650 (2016)

    Article  MathSciNet  Google Scholar 

  13. Cottrell, J.A., Hughes, T.J.R., Bazilevs, Y.: Isogeometric analysis: toward integration of CAD and FEA. Wiley, Hoboken (2009)

    Book  Google Scholar 

  14. Dassi, F., Fumagalli, A., Losapio, D., Scialò, S., Scotti, A., Vacca, G.: The mixed virtual element method on curved edges in two dimensions. Comput. Methods Appl. Mech. Eng. 386, 114098 (2021)

    Article  MathSciNet  Google Scholar 

  15. Dassi, F., Fumagalli, A., Mazzieri, I., Scotti, A., Vacca, G.: A virtual element method for the wave equation on curved edges in two dimensions. J. Sci. Comput. 90(1), 1–25 (2022)

    Article  MathSciNet  Google Scholar 

  16. Dassi, F., Fumagalli, A., Scotti, A., Vacca, G.: Bend 3D mixed virtual element method for Darcy problems. Comput. Math. Appl. 119, 1–12 (2022)

    Article  MathSciNet  Google Scholar 

  17. de Ayuso, B., Lipnikov, K., Manzini, G.: The nonconforming virtual element method. ESAIM Math. Model. Numer. Anal. 50(3), 879–904 (2016)

    Article  MathSciNet  Google Scholar 

  18. Dong, Z., Ern, A.: Hybrid high-order and weak Galerkin methods for the biharmonic problem. SIAM J. Numer. Anal. 60(5), 2626–2656 (2022)

    Article  MathSciNet  Google Scholar 

  19. Ergatoudis, I., Irons, B.M., Zienkiewicz, O.C.: Curved, isoparametric, “quadrilateral’’ elements for finite element analysis. Int. J. Solids Struct. 4(1), 31–42 (1968)

    Article  Google Scholar 

  20. Frittelli, M., Madzvamuse, A., Sgura, I.: Bulk-surface virtual element method for systems of PDEs in two-space dimensions. Numer. Math. 147(2), 305–348 (2021)

    Article  MathSciNet  Google Scholar 

  21. Frittelli, M., Sgura, I.: Virtual element method for the Laplace-Beltrami equation on surfaces. ESAIM Math. Model. Numer. Anal. 52(3), 965–993 (2018)

    Article  MathSciNet  Google Scholar 

  22. Gürkan, C., Sala-Lardies, E., Kronbichler, M., Fernández-Méndez, S.: eXtended Hybridizable Discontinous Galerkin (X-HDG) for void problems. J. Sci. Comput. 66(3), 1313–1333 (2016)

    Article  MathSciNet  Google Scholar 

  23. Lenoir, M.: Optimal isoparametric finite elements and error estimates for domains involving curved boundaries. SIAM J. Numer. Anal. 23(3), 562–580 (1986)

    Article  MathSciNet  Google Scholar 

  24. Mascotto, L., Perugia, I., Pichler, A.: Non-conforming harmonic virtual element method: \(h\)- and \(p\)-versions. J. Sci. Comput. 77(3), 1874–1908 (2018)

    Article  MathSciNet  Google Scholar 

  25. Schwab, C.: \(p\)- and \(hp\)- Finite Element Methods: Theory and Applications in Solid and Fluid Mechanics. Clarendon Press, Oxford (1998)

    Google Scholar 

  26. Stein, E.M.: Singular Integrals and Differentiability Properties of Functions, vol. 2. Princeton University Press, Princeton (1970)

    Google Scholar 

  27. Strang, G., Berger, A.E.: The change in solution due to change in domain. In: Partial differential equations (Proc. Sympos. Pure Math., Vol. XXIII, Univ. California, Berkeley, Calif., 1971), pp. 199–205. Amer. Math. Soc., Providence, R.I. (1973)

  28. Thomée, V.: Polygonal domain approximation in Dirichlet’s problem. IMA J. Appl. Math. 11(1), 33–44 (1973)

    Article  MathSciNet  Google Scholar 

  29. Beirão, L., Brezzi, F., Cangiani, A., Manzini, G., Marini, L.D., Russo, A.: Basic principles of virtual element methods. Math. Models Methods Appl. Sci. 23(01), 199–214 (2013)

    Article  MathSciNet  Google Scholar 

  30. da Beirão, L., Brezzi, F., Marini, L.D.: Virtual elements for linear elasticity problems. SIAM J. Numer. Anal. 51(2), 794–812 (2013)

    Article  MathSciNet  Google Scholar 

  31. da Beirão, L., Brezzi, F., Marini, L.D., Russo, A.: The hitchhiker’s guide to the virtual element method. Math. Models Methods Appl. Sci. 24(08), 1541–1573 (2014)

    Article  MathSciNet  Google Scholar 

  32. da Beirão, L., Brezzi, F., Marini, L.D., Russo, A.: Polynomial preserving virtual elements with curved edges. Mathematical Models and Methods in Applied Sciences 30(08), 1555–1590 (2020)

    Article  MathSciNet  Google Scholar 

  33. da Beirão, L., Lovadina, C., Russo, A.: Stability analysis for the virtual element method. Math. Models Methods Appl. Sci. 27(13), 2557–2594 (2017)

    Article  MathSciNet  Google Scholar 

  34. da Beirão, L., Mascotto, L.: Interpolation and stability properties of low order face and edge virtual element spaces. IMA J. Numer. Anal. 43, 828–851 (2023)

    Article  MathSciNet  Google Scholar 

  35. da Beirão, L., Russo, A., Vacca, G.: The virtual element method with curved edges. ESAIM Math. Model. Numer. Anal. 53(2), 375–404 (2019)

    Article  MathSciNet  Google Scholar 

  36. Yemm, L.: A new approach to handle curved meshes in the hybrid high-order method. Found. Comput. Math. pp. 1–28 (2023)

Download references

Acknowledgements

Y.L. is supported by the NSFC grant 12171244 and China Scholarship Council 202206860034. L. Beirão da Veiga was partially supported by the Italian MIUR through the PRIN Grant No. 905 201744KLJL.

Author information

Authors and Affiliations

  1. Dipartimento di Matematica e Applicazioni, Università degli Studi di Milano-Bicocca, 20125, Milan, Italy

    Lourenço Beirão da Veiga, Yi Liu, Lorenzo Mascotto & Alessandro Russo

  2. IMATI-CNR, 27100, Pavia, Italy

    Lourenço Beirão da Veiga, Lorenzo Mascotto & Alessandro Russo

  3. Nanjing Normal University, Nanjing, 210023, China

    Yi Liu

  4. Fakultät für Mathematik, Universität Wien, 1090, Vienna, Austria

    Lorenzo Mascotto

Authors
  1. Lourenço Beirão da Veiga
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yi Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lorenzo Mascotto
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Alessandro Russo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yi Liu.

Ethics declarations

Conflict of interest

The authors have no relevant financial or non-financial interests to disclose.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Beirão da Veiga, L., Liu, Y., Mascotto, L. et al. The Nonconforming Virtual Element Method with Curved Edges. J Sci Comput 99, 23 (2024). https://doi.org/10.1007/s10915-023-02441-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-023-02441-w

Keywords

Mathematics Subject Classification

Search

Navigation