Optimally Convergent HDG Method for Third-Order Korteweg–de Vries Type Equations

Abstract

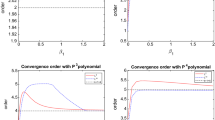
We develop and analyze a new hybridizable discontinuous Galerkin method for solving third-order Korteweg–de Vries type equations. The approximate solutions are defined by a discrete version of a characterization of the exact solution in terms of the solutions to local problems on each element which are patched together through transmission conditions on element interfaces. We prove that the semi-discrete scheme is stable with proper choices of stabilization function in the numerical traces. For the linearized equation, we carry out error analysis and show that the approximations to the exact solution and its derivatives have optimal convergence rates. In numerical experiments, we use an implicit scheme for time discretization and the Newton–Raphson method for solving systems of nonlinear equations, and observe optimal convergence rates for both the linear and the nonlinear third-order equations.

Appendix: Implementation

To implement the HDG method (2.2), we use an implicit scheme for the discretization of the time derivative. One may use high order BDF or an implicit Runge–Kutta method for time discretization. Here, for simplicity we consider the backward Euler method with time-step $\Delta t$. At time-level $t_j$, inserting the definition of the numerical traces (2.2e) into (2.2a)–(2.2e), we obtain the equations

$$\begin{aligned}&({q}_h,{v}) + (u_h,v_x) - \left\langle \widehat{u}_h,{v}n \right\rangle = 0,\\&({p}_h,{z}) + (q_h,z_x) - \left\langle {q}_h+ \tau _{qu} (\widehat{u}_h-u_h)n, z n \right\rangle -\left\langle \tau _{qp} (\widehat{p}_h^{\,-}-p_h), z\right\rangle _{\partial {\mathcal T}_h^-} =0,\\&\quad \frac{1}{\Delta t}({u_h},{w}) -(p_h+F(u_h),w_x) + \left\langle p_h+\tau _{pu}(\widehat{u}_h-u_h)n, w n\right\rangle _{\partial {\mathcal T}_h^+}\quad \quad \\&\quad +\,\left\langle \widehat{p}_h^{\,-}, {w}n \right\rangle _{\partial {\mathcal T}_h^-} +\left\langle F(\widehat{u}_h) -\tau _{F}(\widehat{u}_h, u_h)(\widehat{u}_h -u_h)n, w n\right\rangle = (f,{w}) +\frac{1}{\Delta t}(u_h^{j-1}, w), \end{aligned}$$

from which $(u_h, q_h, p_h)$ can be locally solved in terms of $f,\,\widehat{u}_h$ and $\widehat{p}_h^{\,-}$, and the equations

$$\begin{aligned}&\left\langle q_h+\tau _{qu}(\widehat{u}_h-u_h)n, \mu \, n\right\rangle +\left\langle \tau _{qp} (\widehat{p}_h^{\,-}-p_h), \mu \right\rangle _{\partial {\mathcal T}_h^-}=\left\langle q_N, \mu \,n\right\rangle _{\partial \Omega _N},\\&\quad \left\langle \widehat{p}_h^{\,-}, \chi \, n\right\rangle _{\partial {\mathcal T}_h^-}+\left\langle p_h+\tau _{pu}(\widehat{u}_h-u_h)n, \chi \,n\right\rangle _{\partial {\mathcal T}_h^+}\\&\qquad +\,\left\langle F(\widehat{u}_h)-\tau _F(\widehat{u}_h, u_h)(\widehat{u}_h-u_h)n, \chi \,n\right\rangle =0, \end{aligned}$$

which determine the globally coupled unknowns $(\widehat{u}_h, \widehat{p}_h^{\,-})$.

Next, we apply the Newton–Raphson method to solve the above nonlinear system. Denoting the approximations at the current iteration by $(\bar{u}_h, \bar{q}_h, \bar{p}, \bar{\widehat{u}}_h, \bar{\widehat{p}}_h^{\,-})\in W_h^k\times W_h^k\times W_h^k\times M_h(u_D)\times \tilde{M}_h$, we want to find the increments $(\delta u_h, \delta q_h, \delta p_h, \delta \widehat{u}_h, \delta \widehat{p}_h^{\,-}) \in W_h^k\times W_h^k\times W_h^k\times M_h(0)\times \tilde{M}_h$ such that

$$\begin{aligned}&a_1(\delta q_h, v)+b_1(\delta u_h, v)+d_1(\delta \widehat{u}_h, v)= r_1(v),\\&a_2(\delta p_h, z)-b_1(z, \delta q_h)+c(\delta u_h, z)+d_2(\delta \widehat{u}_h, z)+e_2(\delta \widehat{p}_h^{\,-}, z)=r_2(z),\\&a_3(\delta u_h, w)+b_2(\delta p_h, w)+d_3(\delta \widehat{u}_h, w)+e_3(\delta \widehat{p}_h^{\,-}, w) = r_3(w),\\ \end{aligned}$$

and

$$\begin{aligned}&g_1(\delta p_h, \mu )+g_2(\delta q_h, \mu )+g_3(\delta u_h, \mu )+d_4(\delta \widehat{u}_h, \mu )+e_4(\delta \widehat{p}_h^{\,-}, \mu )= r_4(\mu ),\\&g_4(\delta p_h, \chi )+g_5(\delta u_h, \chi )+d_5(\delta \widehat{u}_h,\chi )+e_5(\delta \widehat{p}_h^{\,-}, \chi ) =r_5(\chi ), \end{aligned}$$

for any $(v, z, w, \mu ,\chi )\,\in \,W_h^{k}\times W_h^{k} \times W_h^{k}\times \tilde{M}_h\times M_h(0)$, where

$$\begin{aligned} a_1(\eta , v)&=(\eta , v), \quad b_1(\sigma , v)=(\sigma , v_x), \quad d_1(\lambda , v)=-\left\langle \lambda , v n\right\rangle ,\\a_2(\rho , z)&= (\rho , z)+\left\langle \tau _{qp} \rho , z\right\rangle _{\partial \mathcal T_h^-}, \quad c(\sigma , z)=\left\langle \tau _{qu} \sigma , z \right\rangle ,\\ d_2(\lambda , z)&= -\left\langle \tau _{qu}\lambda , z\right\rangle ,\quad e_2(\zeta , z)=-\left\langle \tau _{qp} \zeta , z\right\rangle _{\partial \mathcal T_h^-}, \\ a_3(\sigma , w)&=\frac{1}{\Delta t}(\sigma , w)-(F'(\bar{u}_h)\sigma , w_x)-\left\langle \tau _{pu}\sigma , w\right\rangle _{\partial \mathcal T_h^+} +\left\langle (\bar{\tau }_F-\partial _2\bar{\tau }_F(\bar{\widehat{u}}_h-\bar{u}_h))\sigma , w\right\rangle ,\\ b_2(\rho ,w)&= -(\rho , w_x)-\left\langle \rho , w \right\rangle _{\partial \mathcal T_h^+},\quad e_3(\zeta , w)=\left\langle \zeta , w\right\rangle _{\partial \mathcal T_h^-},\\ d_3(\lambda , w)&=\left\langle (F'(\bar{\widehat{u}}_h)n-\partial _1\bar{\tau }_F(\bar{\widehat{u}}_h-\bar{u}_h)-\bar{\tau }_F)\lambda , w\right\rangle +\left\langle \tau _{pu}\lambda ,w\right\rangle _{\partial \mathcal T_h^+},\\ g_1(\rho ,\mu )&=-\left\langle \tau _{qp}\rho ,\mu \right\rangle _{\partial \mathcal T_h^-},\quad g_2(\eta ,\mu )=\left\langle \eta ,\mu n\right\rangle ,\quad g_3(\sigma ,\mu )=-\left\langle \tau _{qu}\sigma , \mu \right\rangle ,\\ g_4(\rho , \chi )&= \left\langle \rho , \chi n\right\rangle _{\partial \mathcal T_h^+},\quad g_5(\sigma , \chi )=-\left\langle \tau _{pu}\sigma ,\chi \right\rangle _{\partial \mathcal T_h^+}+\left\langle (\bar{\tau }_F-\partial _2\bar{\tau }_F (\bar{\widehat{u}}_h-\bar{u}_h)) \sigma , \chi \right\rangle ,\\ d_4(\lambda , \mu )&=\left\langle \tau _{qu}\lambda ,\mu \right\rangle ,\quad e_4(\zeta ,\mu )=\left\langle \tau _{qp}\zeta , \mu \right\rangle _{\partial \mathcal T_h^-},\quad e_5(\zeta , \chi )=\left\langle \zeta ,\chi n\right\rangle _{\partial \mathcal T_h^-},\\ d_5(\lambda ,\chi )&=\left\langle \tau _{pu}\lambda , \chi \right\rangle _{\partial \mathcal T_h^+}+\left\langle (F'(\bar{\widehat{u}}_h)n-\partial _1 \bar{\tau }_F (\bar{\widehat{u}}_h-\bar{u}_h-\bar{\tau }_F))\lambda ,\chi \right\rangle ,\\ r_1(v)&=-(\bar{q}_h, v)-(\bar{u}_h, v_x)+\left\langle \bar{\widehat{u}}_h, vn\right\rangle ,\\ r_2(z)&=-(\bar{p}_h,z)+(\bar{q}_{hx}, z)+\left\langle \tau _{qu}(\bar{\widehat{u}}_h-\bar{u}_h),z\right\rangle +\left\langle \tau _{qp}(\bar{\widehat{p}}_h^{\,-}-\bar{p}_h), z\right\rangle _{\partial \mathcal T_h^-},\\ r_3(w)&=\left( f+\frac{1}{\Delta t}(u_h^{j-1}-\bar{u}_h), w\right) +({\bar{p}_h}+F(\bar{u}_h), w_x) -\left\langle \bar{\widehat{p}}^-_h, w\right\rangle _{\partial \mathcal T_h^-}\\&\quad -\,\left\langle \bar{p}_h n+\tau _{pu}(\bar{\widehat{u}}_h-\bar{u}_h), w \right\rangle _{\partial \mathcal T_h^+} -\left\langle F(\bar{\widehat{u}}_h)n-\bar{\tau }_F(\bar{\widehat{u}}_h-\bar{u}_h), w \right\rangle ,\\ r_4(\mu )&=\left\langle q_N, \mu n \right\rangle _{\partial \Omega _N}-\left\langle \bar{q}_h n+\tau _{qu}(\bar{\widehat{u}}_h-\bar{u}_h), \mu \right\rangle -\left\langle \tau _{qp}(\bar{\widehat{p}}_h^{\,-} -\bar{p}_h), \mu \right\rangle _{\partial \mathcal T_h^-},\\ r_5(\chi )&= -\left\langle \bar{\widehat{p}}_h^{\,-}, \chi n\right\rangle _{\partial \mathcal T_h^-} -\left\langle \bar{p}_h n+\tau _{pu}(\bar{\widehat{u}}_h-\bar{u}_h), \chi \right\rangle _{\partial \mathcal T_h^+}\\&\quad -\,\left\langle F(\bar{\widehat{u}}_h)-\bar{\tau }_F (\bar{\widehat{u}}_h-\bar{u}_h), \chi \right\rangle . \end{aligned}$$

Here we have used the notation $\bar{\tau }_F:=\tau _F(\bar{\widehat{u}}_h, \bar{u}_h)$, and $\partial _1 \bar{\tau }_F$ (respectively, $\partial _2\bar{\tau }_F$) denotes the first-order partial derivative of $\tau _F$ with respect to the first argument (respectively, second argument) evaluated at $(\bar{\widehat{u}}_h, \bar{u}_h)$.

The discretization of the system above gives rise to matrix equations of the form

$$\begin{aligned} \begin{bmatrix} 0&\quad A_1&\quad B_1\\ A_2&\quad -B_1^T&\quad C \\ B_2&\quad 0&\quad A_3 \end{bmatrix} \begin{bmatrix} \delta p_h\\ \delta q_h\\ \delta u_h \end{bmatrix} + \begin{bmatrix} D_1&\quad 0\\ D_2&\quad E_2\\ D_3&\quad E_3 \end{bmatrix} \begin{bmatrix} \delta \widehat{u}_h\\ \delta \widehat{p}_h^{\,-} \end{bmatrix} =\begin{bmatrix} R_1\\ R_2\\ R_3 \end{bmatrix}, \end{aligned}$$

(5.1)

and

$$\begin{aligned} \begin{bmatrix} G_1&\quad G_2&\quad G_3\\ G_4&\quad 0&\quad G_5 \end{bmatrix} \begin{bmatrix} \delta p_h\\ \delta q_h\\ \delta u_h \end{bmatrix} +\begin{bmatrix} D_4&E_4\\ D_5&E_5 \end{bmatrix} \begin{bmatrix} \delta \widehat{u}_h\\ \delta \widehat{p}_h^{\,-} \end{bmatrix} = \begin{bmatrix} R_4\\ R_5 \end{bmatrix}. \end{aligned}$$

(5.2)

From (5.1), we get

$$\begin{aligned} \begin{bmatrix} \delta p_h\\ \delta q_h\\ \delta u_h \end{bmatrix} = \begin{bmatrix} 0&\quad A_1&\quad B_1\\ A_2&\quad -B_1^T&\quad C \\ B_2&\quad 0&\quad A_3 \end{bmatrix}^{-1} \left( \begin{bmatrix} R_1\\ R_2\\ R_3 \end{bmatrix} -\begin{bmatrix} D_1&\quad 0\\ D_2&\quad E_2\\ D_3&\quad E_3 \end{bmatrix} \begin{bmatrix} \delta \widehat{u}_h\\ \delta \widehat{p}_h^{\,-} \end{bmatrix} \right) \end{aligned}$$

(5.3)

We emphasize that the above inverse can be computed on each element independently of each other since the matrices $A_1, A_2,A_3, B_1, B_2$ and C are block-diagonal owing to the discontinuous nature of the approximation spaces. Applying (5.3)–(5.2), we get the global linear system

$$\begin{aligned} \mathbb {K} \begin{bmatrix} \delta \widehat{u}_h\\ \delta \widehat{p}_h^{\,-} \end{bmatrix} =\mathbb {F}, \end{aligned}$$

where

$$\begin{aligned}&\mathbb {K}=\begin{bmatrix} D_4&\quad E_4\\ D_5&\quad E_5 \end{bmatrix} - \begin{bmatrix} G_1&\quad G_2&\quad G_3\\ G_4&\quad 0&\quad G_5 \end{bmatrix} \begin{bmatrix} 0&\quad A_1&\quad B_1\\ A_2&\quad -B_1^T&\quad C \\ B_2&\quad 0&\quad A_3 \end{bmatrix}^{-1} \begin{bmatrix} D_1&\quad 0\\ D_2&\quad E_2\\ D_3&\quad E_3 \end{bmatrix} \end{aligned}$$

and

$$\begin{aligned}&\mathbb {F}= \begin{bmatrix} R_4\\ R_5 \end{bmatrix} -\begin{bmatrix} G_1&\quad G_2&\quad G_3\\ G_4&\quad 0&\quad G_5 \end{bmatrix} \begin{bmatrix} 0&\quad A_1&\quad B_1\\ A_2&\quad -B_1^T&\quad C \\ B_2&\quad 0&\quad A_3 \end{bmatrix}^{-1} \begin{bmatrix} R_1\\ R_2\\ R_3 \end{bmatrix}. \end{aligned}$$

Therefore, the only globally coupled degrees of freedom are those associated with $\delta \widehat{u}_h$ and $\delta \widehat{p}_h^{\,-}$, which live only on element interfaces. Due to the one-dimensional setting of the KdV equation, the size and the bandwidth of the global linear system are independent of the degrees of polynomials used; it only depends on the number of subintervals in the mesh. Once $\delta \widehat{u}_h$ and $\delta \widehat{p}_h^{\,-}$ are obtained, $(\delta p_h, \delta q_h, \delta u_h)$ can be locally computed by using (5.3).