Efficient approximation of solution derivatives for system of singularly perturbed time-dependent convection-diffusion PDEs on Shishkin mesh

Abstract

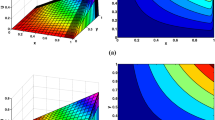
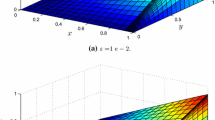
This article deals with a coupled system of singularly perturbed convection-diffusion parabolic partial differential equations (PDEs) possessing overlapping boundary layers. As the thickness of the layer shrinks for small diffusion parameter, efficient capturing of the solution and the diffusive flux (i.e., scaled first-order spatial derivative of the solution) leads to a difficult task. It is well-known that the classical numerical techniques have deficiencies in estimating the solution and the diffusive flux on equidistant mesh unless the mesh-size is adequately large. We aim to generate an efficient numerical approximation to the coupled system of PDEs by employing the implicit-Euler method in time and a classical finite difference scheme in space on a layer-adapted Shishkin mesh. Firstly, we discuss about parameter-uniform convergence of the numerical solution in $C^0$-norm followed by the error analysis for the scaled discrete space derivative and the discrete time derivative. Subsequently, the parameter-uniform error bound is established in weighted $C^1$-norm for global approximation to the solution and the space-time solution derivatives. The theoretical findings are verified by generating the numerical results for two test examples.

Proof of Lemma 4.3

Proof

We derive bound of the truncation error by considering two subregions for $x_i\in (1-{\uptheta }_{\varepsilon _1}^{1}, 1]$ and $x_i\in (1-{\uptheta }_{\varepsilon _2}^{1},1-{\uptheta }_{\varepsilon _1}^{1}]$.

• (i) Let $x_i \in (1-{\uptheta }_{\varepsilon _1}^{1}, 1]$. Then, for $\displaystyle 3N/4< i < N$, by using Lemma 2.2 and the method of [29], Lemma 3.3], and since $\frac{\varepsilon _1}{\varepsilon _2} <1, \text {sinh}\xi \le \xi ,$ we obtain that

  $$\begin{aligned}&\Big |\Big (\delta _{t}^{-}+\texttt{L}_{x,\varepsilon _1}^{N, \Delta t}\Big )(\vec {Q}-\vec {q})(x_i, t_m)\Big |\nonumber \\&\quad \le \text {C} \Bigg [\Delta t+\varepsilon _1^{-1}\mathfrak {B}_{\varepsilon _1}(1-x_{i})(\upbeta \texttt{H}^2/\varepsilon _1)+ \varepsilon _2^{-1}\mathfrak {B}_{\varepsilon _2}(1-x_{i})(\upbeta \texttt{H}^2/\varepsilon _2)\Bigg ]\nonumber \\&\quad \le \text {C} \Bigg [\Delta t+(\varepsilon _1^{-1}\mathfrak {B}_{\varepsilon _1}(1-x_{i})+\varepsilon _2^{-1}\mathfrak {B}_{\varepsilon _2}(1-x_{i}))N^{-1}\ln N\Bigg ], \end{aligned}$$

  and similar bound can be obtained for $\Big (\delta _{t}^{-}+\texttt{L}_{x,\varepsilon _2}^{N, \Delta t}\Big )(\vec {Q}-\vec {q})(x_i, t_m)$.

• (ii) Let $x_i \in (1-{\uptheta }_{\varepsilon _2}^1, 1-{\uptheta }_{\varepsilon _1}^1]$. Since, $\varepsilon _1^{-2}\exp (-\upbeta (1-\xi )/\varepsilon _1) \le \varepsilon _2^{-2}\exp (-\upbeta (1-\xi )/\varepsilon _2)$, for $1-\xi > 2\varepsilon _1/\upbeta$, we deduce for $\displaystyle N/2+\sum _{j=1}^{\Bbbk -1}N_{j} < i \le N/2 + \sum _{j=1}^{\Bbbk }N_{j},$ that

  $$\begin{aligned} \Big |\Big (\delta _{t}^{-}+\texttt{L}_{x,\varepsilon _1}^{N, \Delta t}\Big )(\vec {Q}-\vec {q})(x_i, t_m)\Big |&\le \text {C} \Big [\Delta t+\varepsilon _2^{-1}\mathfrak {B}_{\varepsilon _2}(1-x_{i})(\upbeta \texttt{H}^1/\varepsilon _2)\Big ]\nonumber \\&\le \text {C}\Big [\Delta t+ \varepsilon _2^{-1}\mathfrak {B}_{\varepsilon _2}(1-x_i)N^{-1}\ln N\Big ], \end{aligned}$$

  where $\upbeta \texttt{H}^1 \le \text {C}\varepsilon _2N^{-1}\ln N$; and similarly, one can derive the bound for $\Big (\delta _{t}^{-}+\texttt{L}_{x,\varepsilon _2}^{N, \Delta t}\Big )(\vec {Q}-\vec {q})(x_i, t_m)$.

Now, we consider the following discrete functions:

$$\begin{aligned} \vec {\psi }^\pm (x_i, t_m)=-\vec {\lambda }(x_i,t_m)\pm (\vec {Q}-\vec {q})(x_i,t_m),\quad \text {for } N/2 \le i \le N, \end{aligned}$$

where $\vec {\lambda }=(\lambda _1, \lambda _2)^{T}$ is defined as,

$$\begin{aligned}&\vec {\lambda }(x_i, t_m)\\&\quad = {\left\{ \begin{array}{ll} \displaystyle \displaystyle \Bigg [\vec {\text {C}}(\Delta t+N^{-1}(1+x_{i}))+\text {C}\Uptheta _{2,N}^{-1}\vec {\Uptheta }_{2,i}N^{-1}\ln N\Bigg ], \qquad \text {for}~\displaystyle N/2 \le i \le 3N/4,\\ \displaystyle \Bigg [\vec {\text {C}}(\Delta t+N^{-1}(1+x_{i}))+ \text {C}\Big (\Uptheta _{1,N}^{-1}\vec {\Uptheta }_{1,i}+\Uptheta _{2,N}^{-1}\vec {\Uptheta }_{2,i}\Big ) N^{-1}\ln N \Bigg ],\qquad \\ \quad \text {for} ~\displaystyle 3N/4 < i \le N, \end{array}\right. } \end{aligned}$$

where $\vec {\Uptheta }_{2, i}=(\Uptheta _{2, i}, \Uptheta _{2, i})^{T}, \vec {\Uptheta }_{1, i}=(\Uptheta _{1, i}, -\Uptheta _{1, i})^{T}$. Since, $\texttt{H}^1 =O(\varepsilon _2)$, $\texttt{H}^2=O(\varepsilon _1)$, and $\varepsilon _1 < \varepsilon _2$, from Lemma 3.2, we obtain that

$$\begin{aligned}&\texttt{L}_{x,\varepsilon _1}^{N,\Delta t}\vec {\Uptheta }_{2,i} \ge {\text {C}\varepsilon _2^{-1}\Uptheta _{2,i}},\qquad \texttt{L}_{x,\varepsilon _2}^{N,\Delta t}\vec {\Uptheta }_{2,i} \ge \text {C}\varepsilon _2^{-1}\Uptheta _{2,i},\quad N/2< i \le N,\nonumber \\&\texttt{L}_{x,\varepsilon _1}^{N,\Delta t}\vec {\Uptheta }_{1,i} \ge {\text {C}\varepsilon _1^{-1}\Uptheta _{1,i}},\qquad \texttt{L}_{x,\varepsilon _2}^{N,\Delta t}\vec {\Uptheta }_{1,i} \ge \text {C}\varepsilon _1^{-1}\Uptheta _{1,i}, \quad 3N/4 < i \le N. \end{aligned}$$

(A.1)

Hence, for $N/2< i < N$, using (A.1), since $\mathfrak {B}_{\varepsilon _r}(1-x_i)=e^{-\upbeta (1-x_i)/\varepsilon _r} \le \displaystyle (\Uptheta _{r,i}/\Uptheta _{r,N}),~~ r=1, 2,$ we have

$$\begin{aligned} \Big (\delta _{t}^{-}+\texttt{L}_{x,\vec {\varepsilon }}^{N, \Delta t}\Big )\vec {\lambda }(x_i, t_m) \ge \Big |\Big (\delta _{t}^{-}+\texttt{L}_{x,\vec {\varepsilon }}^{N, \Delta t}\Big )(\vec {Q}-\vec {q})(x_i, t_m)\Big |, \quad \text {for N/2< i < N}. \end{aligned}$$

(A.2)

Now, using Lemma 4.2, we get

$$\begin{aligned} \vec {\lambda }(x_{N/2}, t_m)&=\Bigg [\vec {\text {C}}\Big (\Delta t+N^{-1}(1+x_{N/2})\Big )+\text {C} \Uptheta _{2,N}^{-1} \vec {\Uptheta }_{2, N/2} N^{-1}\ln N\Bigg ],\nonumber \\&\ge \vec {\text {C}}N^{-1} \ge \vec {\text {C}}N^{-2} \ge |(\vec {Q}-\vec {q})(1-{\uptheta }_{\varepsilon _2}^1, t_m)|. \end{aligned}$$

(A.3)

Also, $\vec {\lambda }(x_N, t_m)=\displaystyle \Bigg [\vec {\text {C}}\Big (\Delta t+N^{-1}(1+x_{i})\Big )+ \text {C}\Big ( \Uptheta _{1,N}^{-1}\vec {\Uptheta }_{1,N}+\Uptheta _{2,N}^{-1}\vec {\Uptheta }_{2,N}\Big ) N^{-1}\ln N\Bigg ]\ge \vec {0}.$

Thus,

$$\begin{aligned} \vec {\lambda }(x_N, t_m)\ge \vec {0} =|(\vec {Q}-\vec {q})(1, t_m)|. \end{aligned}$$

(A.4)

Afterwards, we consider $\vec {\lambda }(x_i,0)$, for $N/2\le i\le N$. Clearly, $\lambda _1(x_i, 0) \ge 0,\quad$ for $N/2\le i\le N$. Next, for $\displaystyle N/2 < i \le 3N/4,$ it is obvious that $\lambda _2(x_i, 0) \ge 0$. For $\displaystyle 3N/4 < i \le N,$ we have

$$\begin{aligned} \displaystyle \lambda _2(x_i, 0)= & {} \text {C}\Bigg [\Delta t+N^{-1}(1+x_{i})\\{} & {} \quad \quad + \text {C} \Big (-(\Uptheta _{1,i}/\Uptheta _{1,N})+(\Uptheta _{2, i}/\Uptheta _{2, N})\Big ) N^{-1}\ln N \Bigg ] \ge 0. \end{aligned}$$

Since $0< \Big ((\Uptheta _{2, i}/\Uptheta _{2, N})-(\Uptheta _{1,i}/\Uptheta _{1,N})\Big )<(\Uptheta _{2, i}/\Uptheta _{2, N}) <1.$ Thus, $\lambda _2(x_i, 0) \ge 0, ~\text {for}~N/2 \le i \le N.$ Therefore, we have

$$\begin{aligned} \vec {\lambda }(x_i, 0) \ge \vec {0} =|(\vec {Q}-\vec {q})(x_i, 0)|, \end{aligned}$$

(A.5)

Thus, from (A.2)-(A.5), and by using discrete minimum principle (Lemma 3.1) to $\vec {\psi }^\pm$ over the domain, $\overline{\mathscr {D}}^{N, \Delta t}\cap ([1-{\uptheta }_{\varepsilon _2}^1, 1]\times [0, T]),$ we have

$$\begin{aligned} |(\vec {Q}-\vec {q})(x_i, t_m)| \le \vec {\lambda }(x_i,t_m),\quad (x_i, t_m)\in \overline{\mathscr {D}}_2^{N, \Delta t}\cap ([1-{\uptheta }_{\varepsilon _2}^1, 1]\times [0, T]). \end{aligned}$$

Afterwards, we establish the required estimate for $(\vec {Q}-\vec {q})(x_i, t_m)$ as stated in Lemma 4.3 by deriving the bound for $\vec {\lambda }(x_i, t_m)$.

Let us consider two subregions for $x_i\in (1-{\uptheta }_{\varepsilon _1}^{1}, 1]$ and $x_i\in (1-{\uptheta }_{\varepsilon _2}^{1},1-{\uptheta }_{\varepsilon _1}^{1}]$.

• (i) Let $x_i \in (1-{\uptheta }_{\varepsilon _1}^{1}, 1]$. For $\displaystyle 3N/4 < i \le N,$ we have

  $$\begin{aligned}&\displaystyle \lambda _1(x_i, t_m)\le \text {C}\Big [\Delta t+N^{-1}+ \Big ((\Uptheta _{1,i}/\Uptheta _{1,N})+(\Uptheta _{2, i}/\Uptheta _{2, N}) \Big )N^{-1} \ln N\Big ]\\&\quad \le \text {C}\Big [\Delta t +N^{-1}+N^{-1}\ln N\Big ], \\&\displaystyle \lambda _2(x_i, t_m) \le \text {C}\Big [\Delta t+N^{-1}+ \Big ((\Uptheta _{2, i}/\Uptheta _{2, N})-(\Uptheta _{1, i}/\Uptheta _{1, N})\Big )N^{-1}\ln N \Big ]\\&\quad \le \text {C}\Big [\Delta t + N^{-1}+N^{-1}\ln N\Big ],\! \quad \text {since} \!\quad 0< \Big ((\Uptheta _{2, i}/\Uptheta _{2, N})-(\Uptheta _{1, i}/\Uptheta _{1, N})\Big ) <1. \end{aligned}$$

• (ii) Let $x_i \in (1-{\uptheta }_{\varepsilon _2}^1, 1-{\uptheta }_{\varepsilon _1}^1]$. For $\displaystyle N/2 < i \le 3N/4$, we have

  $$\begin{aligned} \displaystyle \lambda _1(x_i, t_m) \le&\text {C}\Big [\Delta t+N^{-1}+ (\Uptheta _{2, i}/\Uptheta _{2, N}) N^{-1}\ln N\Big ] \\ \le&\text {C}\Big [\Delta t+N^{-1}+N^{-1}\ln N\Big ],\quad \end{aligned}$$

  since $(\Uptheta _{2, i}/\Uptheta _{2, N}) < 1$. Similarly, $\displaystyle \lambda _2(x_i, t_m) \le \text {C}\Big [\Delta t+N^{-1}+N^{-1}\ln N\Big ].$

  Thus, we have

  $$\begin{aligned} \vec {\lambda }(x_i, t_m)\le {\left\{ \begin{array}{ll} \displaystyle \vec {\text {C}}\Big [\Delta t+\text {max}\{N^{-1},N^{-1}\ln N\}\Big ], \quad \text {for}~x_i \in (1-{\uptheta }_{\varepsilon _2}^1, 1-{\uptheta }_{\varepsilon _1}^1], \\ \vec {\text {C}}\Big [\Delta t +N^{-1}+ N^{-1}\ln N \Big ], \quad \text {for}~x_i \in (1-{\uptheta }_{\varepsilon _1}^{1}, 1]. \end{array}\right. } \end{aligned}$$

Hence, the proof is over. $\square$