Skip to main content
SpringerLink
Log in

Algorithm for the development of families of numerical methods based on phase-lag Taylor series

  • Original Paper
  • Published:
Journal of Mathematical Chemistry Aims and scope Submit manuscript

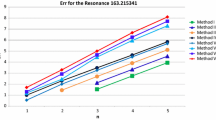
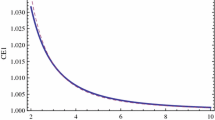
Abstract

In the present research paper we propose a new generator of families of numerical methods with increasing number of internal layers in an attempt to achieve higher order accuracy. The intermediate stages consist of predictor-corrector methods. The final layer is a symmetric two step method with constant coefficients and also free parameters. Those parameters define each family of methods. At first the method is constructed with unknown parameters and subsequently their value is estimated in order to fulfill the requirement of maximum phase-lag order. The stability of the new numerical algorithm is analyzed and the local truncation error is computed. The generator of the new families is applied to well known problems and is found to be more efficient compared to other methods and numerical methods generators with similar characteristics, which attempt to numerically solve such problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Abbreviations

LTE:

Local Truncation Error

References

  1. I. Alolyan, Z.A. Anastassi, T.E. Simos, A new family of symmetric linear four-step methods for the efficient integration of the Schrödinger equation and related oscillatory problems. Appl. Math. Comput. 218(9), 5370–5382 (2012)

    Google Scholar 

  2. I. Alolyan, T. Simos, C. Tsitouras, Interpolants for sixth-order Numerov-type methods. Math. Methods Appl. Sci. (in press) (2019)

  3. I. Alolyan, T.E. Simos, High algebraic order methods with vanished phase-lag and its first derivative for the numerical solution of the Schrödinger equation. J. Math. Chem. 48(4), 925–958 (2010)

    CAS  Google Scholar 

  4. I. Alolyan, T.E. Simos, Mulitstep methods with vanished phase-lag and its first and second derivatives for the numerical integration of the Schrödinger equation. J. Math. Chem. 48(4), 1092–1143 (2010)

    CAS  Google Scholar 

  5. I. Alolyan, T.E. Simos, A family of eight-step methods with vanished phase-lag and its derivatives for the numerical integration of the Schrödinger equation. J. Math. Chem. 49(3), 711–764 (2011)

    CAS  Google Scholar 

  6. I. Alolyan, T.E. Simos, A family of high-order multistep methods with vanished phase-lag and its derivatives for the numerical solution of the Schrödinger equation. Comput. Math. Appl. 62(10), 3756–3774 (2011)

    Google Scholar 

  7. I. Alolyan, T.E. Simos, A family of ten-step methods with vanished phase-lag and its first derivative for the numerical solution of the Schrödinger equation. J. Math. Chem. 49(9), 1843–1888 (2011)

    CAS  Google Scholar 

  8. I. Alolyan, T.E. Simos, On eight-step methods with vanished phase-lag and its derivatives for the numerical solution of the Schrödinger equation. Match Commun. Math. Comput. Chem. 66(2), 473–546 (2011)

    Google Scholar 

  9. I. Alolyan, T.E. Simos, A new high order two-step method with vanished phase-lag and its derivatives for the numerical integration of the Schrödinger equation. J. Math. Chem. 50(9), 2351–2373 (2012)

    CAS  Google Scholar 

  10. I. Alolyan, T.E. Simos, A new hybrid two-step method with vanished phase-lag and its first and second derivatives for the numerical solution of the Schrödinger equation and related problems. J. Math. Chem. 50(7), 1861–1881 (2012)

    CAS  Google Scholar 

  11. I. Alolyan, T.E. Simos, New open modified trigonometrically-fitted Newton-Cotes type multilayer symplectic integrators for the numerical solution of the Schrödinger equation. J. Math. Chem. 50(4), 782–804 (2012)

    CAS  Google Scholar 

  12. I. Alolyan, T.E. Simos, A new four-step hybrid type method with vanished phase-lag and its first derivatives for each level for the approximate integration of the Schrödinger equation. J. Math. Chem. 51(9), 2542–2571 (2013)

    CAS  Google Scholar 

  13. I. Alolyan, T.E. Simos, A new four-step Runge-Kutta type method with vanished phase-lag and its first, second and third derivatives for the numerical solution of the Schrödinger equation. J. Math. Chem. 51(5), 1418–1445 (2013)

    CAS  Google Scholar 

  14. I. Alolyan, T.E. Simos, High order four-step hybrid method with vanished phase-lag and its derivatives for the approximate solution of the Schrödinger equation. J. Math. Chem. 51(2), 532–555 (2013)

    CAS  Google Scholar 

  15. I. Alolyan, T.E. Simos, A family of explicit linear six-step methods with vanished phase-lag and its first derivative. J. Math. Chem. 52(8), 2087–2118 (2014)

    CAS  Google Scholar 

  16. I. Alolyan, T.E. Simos, A hybrid type four-step method with vanished phase-lag and its first, second and third derivatives for each level for the numerical integration of the Schrödinger equation. J. Math. Chem. 52(9), 2334–2379 (2014)

    CAS  Google Scholar 

  17. I. Alolyan, T.E. Simos, A Runge-Kutta type four-step method with vanished phase-lag and its first and second derivatives for each level for the numerical integration of the Schrödinger equation. J. Math. Chem. 52(3), 917–947 (2014)

    CAS  Google Scholar 

  18. I. Alolyan, T.E. Simos, A high algebraic order multistage explicit four-step method with vanished phase-lag and its first, second, third, fourth and fifth derivatives for the numerical solution of the Schrödinger equation. J. Math. Chem. 53(8), 1915–1942 (2015)

    CAS  Google Scholar 

  19. I. Alolyan, T.E. Simos, A high algebraic order predictor-corrector explicit method with vanished phase-lag and its first, second, third and fourth derivatives for the numerical solution of the Schrödinger equation and related problems. J. Math. Chem. 53(7), 1495–1522 (2015)

    CAS  Google Scholar 

  20. I. Alolyan, T.E. Simos, A predictor-corrector explicit four-step method with vanished phase-lag and its first, second and third derivatives for the numerical integration of the Schrödinger equation. J. Math. Chem. 53(2), 685–717 (2015)

    CAS  Google Scholar 

  21. I. Alolyan, T.E. Simos, Efficient low computational cost hybrid explicit four-step method with vanished phase-lag and its first, second, third and fourth derivatives for the numerical integration of the Schrödinger equation. J. Math. Chem. 53(8), 1808–1834 (2015)

    CAS  Google Scholar 

  22. I. Alolyan, T.E. Simos, A family of embedded explicit six-step methods with vanished phase-lag and its derivatives for the numerical integration of the Schrödinger equation: development and theoretical analysis. J. Math. Chem. 54(5), 1159–1186 (2016)

    CAS  Google Scholar 

  23. I. Alolyan, T.E. Simos, A family of two stages tenth algebraic order symmetric six-step methods with vanished phase-lag and its first derivatives for the numerical solution of the radial Schrödinger equation and related problems. J. Math. Chem. 54(9), 1835–1862 (2016)

    CAS  Google Scholar 

  24. I. Alolyan, T.E. Simos, A new eight algebraic order embedded explicit six-step method with vanished phase-lag and its first, second, third and fourth derivatives for the numerical solution of the Schrödinger equation. J. Math. Chem. 54(8), 1696–1727 (2016)

    CAS  Google Scholar 

  25. I. Alolyan, T.E. Simos, An implicit symmetric linear six-step methods with vanished phase-lag and its first, second, third and fourth derivatives for the numerical solution of the radial Schrödinger equation and related problems. J. Math. Chem. 54(4), 1010–1040 (2016)

    CAS  Google Scholar 

  26. I. Alolyan, T.E. Simos, Family of symmetric linear six-step methods with vanished phase-lag and its derivatives and their application to the radial Schrödinger equation and related problems. J. Math. Chem. 54(2), 466–502 (2016)

    CAS  Google Scholar 

  27. I. Alolyan, T.E. Simos, A new two stages tenth algebraic order symmetric six-step method with vanished phase-lag and its first and second derivatives for the solution of the radial Schrödinger equation and related problems. J. Math. Chem. 55(1), 105–131 (2017)

    CAS  Google Scholar 

  28. I. Alolyan, T.E. Simos, New two stages high order symmetric six-step method with vanished phase-lag and its first, second and third derivatives for the numerical solution of the Schrödinger equation. J. Math. Chem. 55(2), 503–531 (2017)

    CAS  Google Scholar 

  29. I. Alolyan, T.E. Simos, New four-stages symmetric six-step method with improved phase properties for second order problems with periodical and/or oscillating solutions. J. Math. Chem. 56(10), 2898–2928 (2018)

    CAS  Google Scholar 

  30. I. Alolyan, T.E. Simos, New three-stages symmetric six-step finite difference method with vanished phase-lag and its derivatives up to sixth derivative for second order initial and/or boundary value problems with periodical and/or oscillating solutions. J. Math. Chem. 56(8), 2267–2301 (2018)

    CAS  Google Scholar 

  31. I. Alolyan, T.E. Simos, A four-stages multistep fraught in phase method for quantum chemistry problems. J. Math. Chem. 57(6), 1627–1651 (2019)

    CAS  Google Scholar 

  32. I. Alolyan, T.E. Simos, New multiple stages multistep method with best possible phase properties for second order initial/boundary value problems. J. Math. Chem. 57(3), 834–857 (2019)

    CAS  Google Scholar 

  33. Z. A. Anastassi, T. E. Simos, A family of two-stage two-step methods for the numerical integration of the Schrödinger equation and related IVPs with oscillating solution. J. Math. Chem., 45(4):1102–1129, APR 2009. Conference on Gene Around the World, Tripolie, GREECE, FEB 29-MAR 01, 2008

    Google Scholar 

  34. Z.A. Anastassi, T.E. Simos, Numerical multistep methods for the efficient solution of quantum mechanics and related problems. Physi. Rep. Rev. Sect. Phys. Lett. 482, 1–240 (2009)

    Google Scholar 

  35. Z. A. Anastassi, T. E. Simos, A parametric symmetric linear four-step method for the efficient integration of the Schrödinger equation and related oscillatory problems. J. Comput. Appl. Math

  36. D.B. Berg, T.E. Simos, An efficient six-step method for the solution of the Schrödinger equation. J. Math. Chem. 55(8), 1521–1547 (2017)

    CAS  Google Scholar 

  37. D.B. Berg, T.E. Simos, High order computationally economical six-step method with vanished phase-lag and its derivatives for the numerical solution of the Schrödinger equation. J. Math. Chem. 55(4), 987–1013 (2017)

    CAS  Google Scholar 

  38. D.B. Berg, T.E. Simos, Three stages symmetric six-step method with eliminated phase-lag and its derivatives for the solution of the Schrödinger equation. J. Math. Chem. 55(5), 1213–1235 (2017)

    CAS  Google Scholar 

  39. D.B. Berg, T.E. Simos, A new multistep finite difference pair for the Schrödinger equation and related problems. J. Math. Chem. 56(3), 656–686 (2018)

    CAS  Google Scholar 

  40. D.B. Berg, T.E. Simos, C. Tsitouras, Trigonometric fitted, eighth-order explicit Numerov-type methods. Math. Methods Appl. Sci. 41(5), 1845–1854 (2018)

    Google Scholar 

  41. L. Brusa, L. Nigro, A one-step method for direct integration of structural dynamic equations. Int. J. Numer. Meth. Eng. 15, 685–699 (1980)

    Google Scholar 

  42. M.M. Chawla, P.S. Rao, A noumerov-type method with minimal phase-lag for the integration of second order periodic initial-value. J. Comput. Appl. Math. 15, 329–337 (1986)

    Google Scholar 

  43. Z. Chen, C. Liu, C.-W. Hsu, T.E. Simos, A new multistage multistep full in phase algorithm with optimized characteristics for problems in chemistry. J. Math. Chem. 57(4), 1112–1139 (2019)

    CAS  Google Scholar 

  44. Z. Chen, C. Liu, T.E. Simos, New three-stages symmetric two step method with improved properties for second order initial/boundary value problems. J. Math. Chem. 56(9), 2591–2616 (2018)

    CAS  Google Scholar 

  45. X. Cheng, T.E. Simos, A new four-stages high algebraic order two-step method with vanished phase-lag and its first, second and third derivatives for the numerical solution of the schrödinger equation. MATCH Commun. Math. Comput. Chem. 77(2), 527–568 (2017)

    Google Scholar 

  46. M. Dong, T.E. Simos, A new high algebraic order efficient finite difference method for the solution of the Schrödinger equation. Filomat 31(15), 4999–5012 (2017)

    Google Scholar 

  47. J. Fang, C. Liu, C.-W. Hsu, T.E. Simos, C. Tsitouras, Explicit hybrid six-step, sixth order, fully symmetric methods for solving y ‘’ = f (x, y). Math. Methods Appl.Sci. 42(9), 3305–3314 (2019)

    Google Scholar 

  48. J. Fang, C. Liu, T.E. Simos, A hybrid finite difference pair with maximum phase and stability properties. J. Math. Chem. 56(2), 423–448 (2018)

    CAS  Google Scholar 

  49. J. Fang, C. Liu, T. E. Simos, I. T. Famelis, Neural network solution of single delay differential equations. Mediterranean J. Math. (to appear) (2019)

  50. R. Hao, T.E. Simos, New Runge-Kutta type symmetric two step finite difference pair with improved properties for second order initial and/or boundary value problems. J. Math. Chem. 56(10), 3014–3044 (2018)

    CAS  Google Scholar 

  51. C.-W. Hsu, C. Lin, C. Liu, T.E. Simos, A new four-stages two-step phase fitted scheme for problems in quantum chemistry. J. Math. Chem. 57(4), 1201–1229 (2019)

    CAS  Google Scholar 

  52. F. Hui, T.E. Simos, A new family of two stage symmetric two-step methods with vanished phase-lag and its derivatives for the numerical integration of the Schrödinger equation. J. Math. Chem. 53(10), 2191–2213 (2015)

    CAS  Google Scholar 

  53. F. Hui, T.E. Simos, Hybrid high algebraic order two-step method with vanished phase-lag and its first and second derivatives. MATCH Commun. Math. Comput. Chem. 73(3), 619–648 (2015)

    Google Scholar 

  54. F. Hui, T.E. Simos, Four stages symmetric two-step p-stable method with vanished phase-lag and its first, second, third and fourth derivatives. Appl. Comput. Math. 15(2), 220–238 (2016)

    Google Scholar 

  55. F. Hui, T.E. Simos, New multistage two-step complete in phase scheme with improved properties for quantum chemistry problems. J. Math. Chem. 57(4), 1088–1111 (2019)

    CAS  Google Scholar 

  56. L. Ixaru, M. Rizea, A numerov-like scheme for the numerical solution of the schrödinger equation in the deep continuum spectrum of energies. Comput. Phys. Commun. 19, 23–27 (1980)

    Google Scholar 

  57. L. G. Ixaru. Numerical methods for differential equations and applications. (1984)

  58. P.J. Van der Houwen, B.P. Someijer, Predictor-corrector methods for periodic second-order initial-value problems. J. Numer. Anal. 7, 407–422 (1987)

    Google Scholar 

  59. Z. Kalogiratou, T. Monovasilis, G. Psihoyios, T.E. Simos, Runge–Kutta type methods with special properties for the numerical integration of ordinary differential equations. Phys. Rep. Rev. Sect. Phys. Lett. 536(3), 75–146 (2014)

    Google Scholar 

  60. Z. Kalogiratou, T. Monovasilis, H. Ramos, T.E. Simos, A new approach on the construction of trigonometrically fitted two step hybrid methods. J. Comput. Appl. Math. 303, 146–155 (2016)

    Google Scholar 

  61. Z. Kalogiratou, T. Monovasilis, T.E. Simos, Computation of the eigenvalues of the Schrödinger equation by exponentially-fitted Runge-Kutta-Nystrom methods. Comput. Phys. Commun. 180(2), 167–176 (2009)

    CAS  Google Scholar 

  62. Z. Kalogiratou, T. Monovasilis, T.E. Simos, New modified Runge-Kutta-Nystrom methods for the numerical integration of the Schrödinger equation. Comput. Math. Appl. 60(6), 1639–1647 (2010)

    Google Scholar 

  63. Z. Kalogiratou, T. Monovasilis, T.E. Simos, A fourth order modified trigonometrically fitted symplectic Runge-Kutta-Nystrom method. Comput. Phys. Commun. 185(12), 3151–3155 (2014)

    CAS  Google Scholar 

  64. Z. Kalogiratou, T. Monovasilis, T.E. Simos, New fifth-order two-derivative Runge-Kutta methods with constant and frequency-dependent coefficients. Math. Methods Appl.Sci. 42(6), 1955–1966 (2019)

    Google Scholar 

  65. A. Konguetsof, A new two-step hybrid method for the numerical solution of the schrödinger equation. J. Math. Chem. 47, 871–890 (2010)

    CAS  Google Scholar 

  66. A. Konguetsof, Two-step high order hybrid explicit method for the numerical solution of the schrödinger equation. J. Math. Chem. 48, 224–252 (2010)

    CAS  Google Scholar 

  67. A. Konguetsof, A hybrid method with phase-lag and derivatives equal to zero for the numerical integration of the schrödinger equation. J. Math. Chem. 49, 1330–1356 (2011)

    CAS  Google Scholar 

  68. A. Konguetsof, A generator of families of two-step numerical methods with free parameters and minimal phase-lag. J. Math. Chem. 55, 1–25 (2017)

    Google Scholar 

  69. A.A. Kosti, Z.A. Anastassi, T.E. Simos, An optimized explicit Runge-Kutta method with increased phase-lag order for the numerical solution of the Schrödinger equation and related problems. J. Math. Chem. 47(1), 315–330 (2010)

    CAS  Google Scholar 

  70. A.A. Kosti, Z.A. Anastassi, T.E. Simos, Construction of an optimized explicit Runge-Kutta-Nystrom method for the numerical solution of oscillatory initial value problems. Comput. Math. Appl. 61(11), 3381–3390 (2011)

    Google Scholar 

  71. A.A. Kosti, Z.A. Anastassi, T.E. Simos, An optimized explicit Runge-Kutta-Nystrom method for the numerical solution of orbital and related periodical initial value problems. Comput. Phys. Commun. 183(3), 470–479 (2012)

    CAS  Google Scholar 

  72. V .N. Kovalnogov, R.V. Fedorov, A .A. Bondarenko, T .E. Simos, New hybrid two-step method with optimized phase and stability characteristics. J. Math. Chem. 56(8), 2302–2340 (2018)

    CAS  Google Scholar 

  73. V.N. Kovalnogov, R.V. Fedorov, V.M. Golovanov, B.M. Kostishko, T.E. Simos, A four stages numerical pair with optimal phase and stability properties. J. Math. Chem. 56(1), 81–102 (2018)

    CAS  Google Scholar 

  74. V.N. Kovalnogov, R.V. Fedorov, T.E. Simos, New hybrid symmetric two step scheme with optimized characteristics for second order problems. J. Math. Chem. 56(9), 2816–2844 (2018)

    CAS  Google Scholar 

  75. V.N. Kovalnogov, R.V. Fedorov, D.V. Suranov, T.E. Simos, New multiple stages scheme with improved properties for second order problems. J. Math. Chem. 57(1), 232–262 (2019)

    CAS  Google Scholar 

  76. J. D. Lambert, I. A. Watson. Symmetric multistip methods for periodic initial value problems. (1976)

    Google Scholar 

  77. T. Lei, T.E. Simos, Four-stages twelfth algebraic order two-step method with vanished phase-lag and its first and second derivatives for the numerical solution of the schrödinger equation. MATCH Commun. Math. Comput. Chem. 76(2), 475–510 (2016)

    Google Scholar 

  78. M. Liang, T.E. Simos, A new four stages symmetric two-step method with vanished phase-lag and its first derivative for the numerical integration of the Schrödinger equation. J. Math. Chem. 54(5), 1187–1211 (2016)

    CAS  Google Scholar 

  79. C. Lin, J. J. Chen, T. E. Simos, C. Tsitouras. Evolutionary Derivation of Sixth-Order P-stable SDIRKN Methods for the Solution of PDEs with the Method of Lines. Mediterranean Journal of Mathematics, 16(3)(Article Number: 69), (JUN 2019)

  80. C. Liu, C.-W. Hsu, T.E. Simos, C. Tsitouras, Phase-fitted, six-step methods for solving x ‘’ = f(t, x). Math. Methods Appl. Sci. 42(11), 3942–3949 (2019)

    Google Scholar 

  81. C. Liu, C.-W. Hsu, C. Tsitouras, T.E. Simos, Hybrid Numerov-type methods with coefficients trained to perform better on classical orbits. Bull. Malays. Math. Sci. Soc. 42(5), 2119–2134 (2019)

    Google Scholar 

  82. C. Liu, T.E. Simos, A five-stages symmetric method with improved phase properties. J. Math. Chem. 56(4), 1313–1338 (2018)

    CAS  Google Scholar 

  83. J. Ma, T.E. Simos, Runge–Kutta type tenth algebraic order two-step method with vanished phase-lag and its first, second and third derivatives. MATCH Commun. Math. Comput. Chem. 74(3), 609–644 (2015)

    Google Scholar 

  84. J. Ma, T.E. Simos, Hybrid high algebraic order two-step method with vanished phase-lag and its first, second, third, fourth and fifth derivatives. International Journal of Modern Physics C 27(5) (2016)

  85. J. Ma, T.E. Simos, An efficient and computational effective method for second order problems. J. Math. Chem. 55(8), 1649–1668 (2017)

    CAS  Google Scholar 

  86. M.A. Medvedev, T.E. Simos, Two stages six-step method with eliminated phase-lag and its first, second, third and fourth derivatives for the approximation of the Schrödinger equation. J. Math. Chem. 55(4), 961–986 (2017)

    CAS  Google Scholar 

  87. M.A. Medvedev, T.E. Simos, A multistep method with optimal properties for second order differential equations. J. Math. Chem. 56(1), 1–29 (2018)

    CAS  Google Scholar 

  88. M.A. Medvedev, T.E. Simos, A new high order method with optimal stability and phase properties. MATCH Commun. Math. Comput. Chem. 79(1), 215–260 (2018)

    Google Scholar 

  89. M.A. Medvedev, T.E. Simos, A new six-step algorithm with improved properties for the numerical solution of second order initial and/or boundary value problems. J. Math. Chem. 56(4), 1206–1233 (2018)

    CAS  Google Scholar 

  90. M.A. Medvedev, T.E. Simos, A new three-stages six-step finite difference pair with optimal phase properties for second order initial and/or boundary value problems with periodical and/or oscillating solutions. J. Math. Chem. 56(4), 1280–1312 (2018)

    CAS  Google Scholar 

  91. M.A. Medvedev, T.E. Simos, New high order p-stable method with optimal phase properties. MATCH Commun. Math. Comput. Chem. 79(1), 175–214 (2018)

    Google Scholar 

  92. M.A. Medvedev, T.E. Simos, A three-stages multistep teeming in phase algorithm for computational problems in chemistry. J. Math. Chem. 57(6), 1598–1617 (2019)

    CAS  Google Scholar 

  93. M. A. Medvedev, T. E. Simos, C. Tsitouras. Low-order, P-stable, two-step methods for use with lax accuracies. Math. Methods Appl. Sci

  94. M.A. Medvedev, T.E. Simos, C. Tsitouras, Explicit, two-stage, sixth-order, hybrid four-step methods for solving (x)=f(x, y). Math. Methods Appl. Sci. 41(16), 6997–7006 (2018)

    Google Scholar 

  95. M.A. Medvedev, T.E. Simos, C. Tsitouras, Fitted modifications of Runge-Kutta pairs of orders 6(5). Math. Methods Appl. Sci. 41(16), 6184–6194 (2018)

    Google Scholar 

  96. M.A. Medvedev, T.E. Simos, C. Tsitouras, Hybrid, phase-fitted, four-step methods of seventh order for solving x ‘’(t) = f(t, x). Math. Methods Appl. Sci. 42(6), 2025–2032 (2019)

    Google Scholar 

  97. M. A. Medvedev, T. E. Simos, C. Tsitouras. Local interpolants for Numerov-type methods and their implementation in variable step schemes. Math. Methods Appl. Sci. (in press) (2019)

  98. M.A. Medvedev, T.E. Simos, C. Tsitouras, Trigonometric-fitted hybrid four-step methods of sixth order for solving y ‘’(x)=f(x, y). Math. Methods Appl. Sci. 42(2), 710–716 (2019)

    Google Scholar 

  99. M. A. Medvedeva, T. Simos, C. Tsitouras. Variable step-size implementation of the sixth-order Numerov-type methods. Math. Methods Appl. Sci. (in press) (2019)

  100. M.A. Medvedeva, T.E. Simos, A new high order finite difference pair with improved properties. MATCH Commun. Math. Comput. Chem. 80(2), 481–536 (2018)

    Google Scholar 

  101. M.A. Medvedeva, T.E. Simos, A high order multistage scheme with improved properties. MATCH Commun. Math. Comput. Chem. 81(3), 639–702 (2019)

    Google Scholar 

  102. T. Monovasilis, Z. Kalogiratou, H. Ramos, T.E. Simos, Modified two-step hybrid methods for the numerical integration of oscillatory problems. Math. Methods Appl. Sci. 40(14), 5286–5294 (2017)

    Google Scholar 

  103. T. Monovasilis, Z. Kalogiratou, T. E. Simos. A family of trigonometrically fitted partitioned Runge-Kutta symplectic methods. APPLIED MATHEMATICS AND COMPUTATION, 209(1):91–96, MAR 1 2009. International Conference on Computational Methods in Sciences and Engineering (ICCMSE 2005), Corinth, GREECE, OCT 21-26 (2005)

  104. T. Monovasilis, Z. Kalogiratou, T.E. Simos, Symplectic Partitioned Runge-Kutta methods with minimal phase-lag. Comput. Phys. Commun. 181(7), 1251–1254 (2010)

    CAS  Google Scholar 

  105. T. Monovasilis, Z. Kalogiratou, T.E. Simos, Two new phase-fitted symplectic partitioned Runge–Kutta methods. Int. J. Mod. Phys. C 22(12), 1343–1355 (2011)

    Google Scholar 

  106. T. Monovasilis, Z. Kalogiratou, T.E. Simos, Exponentially fitted symplectic Runge–Kutta–Nystrom methods. Appl. Math. Inf. Sci. 7(1), 81–85 (2013)

    Google Scholar 

  107. T. Monovasilis, Z. Kalogiratou, T.E. Simos, Construction of exponentially fitted symplectic Runge–Kutta–Nystrom methods from partitioned Runge–Kutta methods. Mediterr. J. Math. 13(4), 2271–2285 (2016)

    Google Scholar 

  108. T. Monovasilis, Z. Kalogiratou, T.E. Simos, Trigonometrical fitting conditions for two derivative Runge–Kutta methods. Numer. Algorithm 79(3), 787–800 (2018)

    Google Scholar 

  109. K. Mu, T.E. Simos, A Runge-Kutta type implicit high algebraic order two-step method with vanished phase-lag and its first, second, third and fourth derivatives for the numerical solution of coupled differential equations arising from the Schrödinger equation. J. Math. Chem. 53(5), 1239–1256 (2015)

    CAS  Google Scholar 

  110. H. Ning, T.E. Simos, A low computational cost eight algebraic order hybrid method with vanished phase-lag and its first, second, third and fourth derivatives for the approximate solution of the Schrödinger equation. J. Math. Chem. 53(6), 1295–1312 (2015)

    CAS  Google Scholar 

  111. H. Ning, T.E. Simos, High algebraic order Runge-Kutta type two-step method with vanished phase-lag and its first, second, third, fourth, fifth and sixth derivatives. Comput. Phys. Commun. 196, 226–235 (2015)

    CAS  Google Scholar 

  112. G.A. Panopoulos, Z.A. Anastassi, T.E. Simos, Two optimized symmetric eight-step implicit methods for initial-value problems with oscillating solutions. J. Math. Chem. 46(2), 604–620 (2009)

    CAS  Google Scholar 

  113. G.A. Panopoulos, Z.A. Anastassi, T.E. Simos, A new symmetric eight-step predictor-corrector method for the numerical solution of the radial schrodinger equation and related orbital problems. Int. J. Mod. Phys. C 22(2), 133–153 (2011)

    Google Scholar 

  114. G.A. Panopoulos, Z.A. Anastassi, T.E. Simos, A symmetric eight-step predictor-corrector method for the numerical solution of the radial Schrödinger equation and related IVPs with oscillating solutions. Comput. Phys. Commun. 182(8), 1626–1637 (2011)

    CAS  Google Scholar 

  115. G.A. Panopoulos, Z.A. Anastassi, T.E. Simos, A new eight-step symmetric embedded predictor-corrector method (EPCM) for orbital problems and related IVPS with oscillatory solutions. Astronomical Journal 145(3) (2013)

    Google Scholar 

  116. G.A. Panopoulos, T.E. Simos, A new optimized symmetric 8-step semi-embedded predictor-corrector method for the numerical solution of the radial Schrödinger equation and related orbital problems. J. Math. Chem. 51(7), 1914–1937 (2013)

    CAS  Google Scholar 

  117. G.A. Panopoulos, T.E. Simos, An optimized symmetric 8-step semi-embedded predictor-corrector method for IVPs with oscillating solutions. Appl. Math. Inf. Sci. 7(1), 73–80 (2013)

    Google Scholar 

  118. G.A. Panopoulos, T.E. Simos, A new optimized symmetric embedded predictor-corrector method (EPCM) for initial-value problems with oscillatory solutions. Applied Mathematics and Information Sciences 8(2), 703–713 (2014)

    Google Scholar 

  119. G.A. Panopoulos, T.E. Simos, A new phase-fitted eight-step symmetric embedded predictor-corrector method (EPCM) for orbital problems and related IVPs with oscillating solutions. Comput. Phys. Commun. 185(2), 512–523 (2014)

    CAS  Google Scholar 

  120. G.A. Panopoulos, T.E. Simos, An eight-step semi-embedded predictor-corrector method for orbital problems and related IVPs with oscillatory solutions for which the frequency is unknown. J. Comput. Appl. Math. 290, 1–15 (2015)

    Google Scholar 

  121. D.F. Papadopoulos, Z.A. Anastassi, T.E. Simos, A phase-fitted Runge-Kutta-Nystrom method for the numerical solution of initial value problems with oscillating solutions. Comput. Phys. Commun. 180(10), 1839–1846 (2009)

    CAS  Google Scholar 

  122. D.F. Papadopoulos, Z.A. Anastassi, T.E. Simos, A modified phase-fitted and amplification-fitted Runge-Kutta-Nystrom method for the numerical solution of the radial Schrödinger equation. J. Mol. Model. 16(8), 1339–1346 (2010)

    CAS  PubMed  Google Scholar 

  123. D.F. Papadopoulos, Z.A. Anastassi, T.E. Simos, An optimized Runge–Kutta–Nystrom method for the numerical solution of the Schrödinger equation and related problems. MATCH Commun. Math. Comput. Chem. 64(2), 551–566 (2010)

    Google Scholar 

  124. D.F. Papadopoulos, T.E. Simos, A new methodology for the construction of optimized Runge–Kutta–Nystrom methods. Int. J. Mod. Phys. C 22(6), 623–634 (2011)

    Google Scholar 

  125. D.F. Papadopoulos, T.E. Simos, A modified Runge–Kutta–Nystrom method by using phase lag properties for the numerical solution of orbital problems. Appl. Math. Inf. Sci. 7(2), 433–437 (2013)

    Google Scholar 

  126. D. F. Papadopoulos, T. E. Simos. The use of phase lag and amplification error derivatives for the construction of a modified Runge–Kutta–Nystrom method. Abstract Appl. Anal. Article Number: 910624 (2013)

  127. G.-H. Qiu, C. Liu, T.E. Simos, A new multistep method with optimized characteristics for initial and/or boundary value problems. J. Math. Chem. 57(1), 119–148 (2019)

    CAS  Google Scholar 

  128. G. D. Quinlan, S. Tremaine. Symmetric multistep methods for the numerical integration of planetary orbits. (1990)

    Google Scholar 

  129. H. Ramos, Z. Kalogiratou, T. Monovasilis, T.E. Simos, An optimized two-step hybrid block method for solving general second order initial-value problems. Numer. Algorithm. 72(4), 1089–1102 (2016)

    Google Scholar 

  130. A.D. Raptis, Two-step methods for the numerical solution of the schrödinger equation. Computing 28, 373–378 (1982)

    Google Scholar 

  131. A.D. Raptis, A.C. Allison, Exponential-fitting methods for the numerical solution of the schrodinger equation. Comput. Phys. Commun. 14, 1–5 (1978)

    Google Scholar 

  132. A.D. Raptis, T.E. Simos, A four-step phase-fitted method for the numerical integration of second order initial-value problems. BIT 31(1), 160–168 (1991)

    Google Scholar 

  133. X. Shi, T.E. Simos, New five-stages finite difference pair with optimized phase properties. J. Math. Chem. 56(4), 982–1010 (2018)

    CAS  Google Scholar 

  134. T. E. Simos, A new Numerov-type method for the numerical solution of the Schrödinger equation. J. Math. Chem., 46(3):981–1007, OCT 2009. International Conference on Computational Methods in Sciences and Engineering (ICCMSE 2005), Corinth, GREECE, OCT 21-26, (2005)

  135. T.E. Simos, Closed Newton–Cotes trigonometrically-fitted formulae of high order for long-time integration of orbital problems. Appl. Math. Lett. 22(10), 1616–1621 (2009)

    Google Scholar 

  136. T. E. Simos, High order closed Newton-Cotes trigonometrically-fitted formulae for the numerical solution of the Schrödinger equation. Appl. Math. Comput. 209(1):137–151, MAR 1 2009. International conference on computational methods in sciences and engineering (ICCMSE 2005), Corinth, GREECE, OCT 21-26 (2005)

  137. T.E. Simos, P-stability, Trigonometric-fitting and the numerical solution of the radial Schrödinger equation. Comput. Phys. Commun. 180(7), 1072–1085 (2009)

    CAS  Google Scholar 

  138. T.E. Simos, Exponentially and trigonometrically fitted methods for the solution of the Schrödinger equation. Acta Appl, Math. 110(3), 1331–1352 (2010)

    Google Scholar 

  139. T. E. Simos, New closed Newton–Cotes type formulae as multilayer symplectic integrators. J. Chem. Phys. 133(10) (2010)

    CAS  PubMed  Google Scholar 

  140. T.E. Simos, A two-step method with vanished phase-lag and its first two derivatives for the numerical solution of the Schrödinger equation. J. Math. Chem. 49(10), 2486–2518 (2011)

    CAS  Google Scholar 

  141. T.E. Simos, Optimizing a class of linear multi-step methods for the approximate solution of the radial Schrödinger equation and related problems with respect to phase-lag. Cent. Eur. J. Phys. 9(6), 1518–1535 (2011)

    Google Scholar 

  142. T.E. Simos, High order closed Newton-Cotes exponentially and trigonometrically fitted formulae as multilayer symplectic integrators and their application to the radial Schrödinger equation. J. Math. Chem. 50(5), 1224–1261 (2012)

    CAS  Google Scholar 

  143. T. E. Simos, New stable closed Newton–Cotes trigonometrically fitted formulae for long-time integration. Abstract Appl. Anal. Article Number: 182536 (2012)

  144. T. E. Simos, Optimizing a hybrid two-step method for the numerical solution of the Schrödinger equation and related problems with respect to phase-lag. J Appl. Math. Article Number: 420387 (2012)

  145. T.E. Simos, Accurately closed Newton–Cotes trigonometrically-fitted formulae for the numerical solution of the schrodinger equation. Int. J. Modern Phys. C 24(3) (2013)

    Google Scholar 

  146. T .E. Simos, New high order multiderivative explicit four-step methods with vanished phase-lag and its derivatives for the approximate solution of the Schrödinger equation. Part I: Construction and theoretical analysis. J. Math. Chem. 51(1), 194–226 (2013)

    CAS  Google Scholar 

  147. T.E. Simos, New open modified Newton Cotes type formulae as multilayer symplectic integrators. Appl. Math. Model. 37(4), 1983–1991 (2013)

    Google Scholar 

  148. T.E. Simos, A new explicit hybrid four-step method with vanished phase-lag and its derivatives. J. Math. Chem. 52(7), 1690–1716 (2014)

    CAS  Google Scholar 

  149. T.E. Simos, An explicit four-step method with vanished phase-lag and its first and second derivatives. J. Math. Chem. 52(3), 833–855 (2014)

    CAS  Google Scholar 

  150. T.E. Simos, An explicit linear six-step method with vanished phase-lag and its first derivative. J. Math. Chem. 52(7), 1895–1920 (2014)

    CAS  Google Scholar 

  151. T.E. Simos, On the explicit four-step methods with vanished phase-lag and its first derivative. Appl. Math. Inf. Sci. 8(2), 447–458 (2014)

    Google Scholar 

  152. T.E. Simos, A new explicit four-step method with vanished phase-lag and its first and second derivatives. J. Math. Chem. 53(1), 402–429 (2015)

    CAS  Google Scholar 

  153. T. E. Simos, Multistage symmetric two-step p-stable method with vanished phase-lag and its first, second and third derivatives. Appl. Comput. Math. 14(3, SI):296–315 (2015)

  154. T.E. Simos, C. Tsitouras, A new family of 7 stages, eighth-order explicit Numerov-type methods. Math. Methods Appl. Sci. 40(18), 7867–7878 (2017)

    Google Scholar 

  155. T.E. Simos, C. Tsitouras, Evolutionary generation of high-order, explicit, two-step methods for second-order linear IVPs. Math. Methods Appl. Sci. 40(18), 6276–6284 (2017)

    Google Scholar 

  156. T.E. Simos, C. Tsitouras, Fitted modifications of classical Runge-Kutta pairs of orders 5(4). Math. Methods Appl. Sci. 41(12), 4549–4559 (2018)

    Google Scholar 

  157. T.E. Simos, C. Tsitouras, High phase-lag order, four-step methods for solving y ‘’ = f (x, y). Appl. Comput. Math. 17(3), 307–316 (2018)

    Google Scholar 

  158. T.E. Simos, C. Tsitouras, I.T. Famelis, Explicit numerov type methods with constant coefficients: a review. Appl. Comput. Math. 16(2), 89–113 (2017)

    Google Scholar 

  159. P.I. Stasinos, T.E. Simos, New 8-step symmetric embedded predictor-corrector (EPCM) method with vanished phase-lag and its first derivative for the numerical integration of the Schrödinger equation. J. Math. Chem. 56(9), 2741–2767 (2018)

    CAS  Google Scholar 

  160. S. Stavroyiannis, T.E. Simos, Optimization as a function of the phase-lag order of nonlinear explicit two-step P-stable method for linear periodic IVPs. Appl. Numer., Math. 59(10), 2467–2474 (2009)

    Google Scholar 

  161. S. Stavroyiannis, T.E. Simos, A nonlinear explicit two-step fourth algebraic order method of order infinity for linear periodic initial value problems. Comput. Phys. Commun. 181(8), 1362–1368 (2010)

    CAS  Google Scholar 

  162. K. Tselios, T.E. Simos, Optimized fifth order symplectic integrators for orbital problems. Rev. Mex. Astron. Astrofis. 49(1), 11–24 (2013)

    Google Scholar 

  163. C. Tsitouras, I.T. Famelis, T.E. Simos, On modified Runge-Kutta trees and methods. Comput. Math. Appl. 62(4), 2101–2111 (2011)

    Google Scholar 

  164. C. Tsitouras, I.T. Famelis, T.E. Simos, Phase-fitted Runge–Kutta pairs of orders 8(7). J. Comput. Appl. Math. 321, 226–231 (2017)

    Google Scholar 

  165. C. Tsitouras, T. E. Simos, On ninth order, explicit numerov-type methods with constant coefficients. Mediterr. J. Math. 15(2)(Article Number: 46) (2018)

  166. C. Tsitouras, T. E. Simos. Trigonometric-fitted explicit numerov-type method with vanishing phase-lag and its first and second derivatives. Mediterr. J. Math. 15(4)(Article Number: 168) (2018)

  167. H. Van deVyver, An explicit numerov-type method for second-order differential equations with oscillating solutions. Comput. Math. Appl. 53:1339–1348, 05 (2007)

  168. G. Wang, T.E. Simos, New multiple stages two-step complete in phase algorithm with improved characteristics for second order initial/boundary value problems. J. Math. Chem. 57(2), 494–515 (2019)

    CAS  Google Scholar 

  169. Z. Wang, T.E. Simos, An economical eighth-order method for the approximation of the solution of the Schrödinger equation. J. Math. Chem. 55(3), 717–733 (2017)

    CAS  Google Scholar 

  170. X. Xi, T.E. Simos, A new high algebraic order four stages symmetric two-step method with vanished phase-lag and its first and second derivatives for the numerical solution of the Schrödinger equation and related problems. J. Math. Chem. 54(7), 1417–1439 (2016)

    CAS  Google Scholar 

  171. X. Xi, T.E. Simos, A new four-stages twelfth algebraic order two-step method with vanished phase-lag and its first, second, third and fourth derivatives for the numerical solution of the Schrödinger equation. MATCH Commun. Math. Comput. Chem. 77(2), 333–392 (2017)

    Google Scholar 

  172. M. Xu, T.E. Simos, A multistage two-step fraught in phase scheme for problems in mathematical chemistry. J. Math. Chem. 57(7), 1710–1731 (2019)

    CAS  Google Scholar 

  173. K. Yan, T.E. Simos, A finite difference pair with improved phase and stability properties. J. Math. Chem. 56(1), 170–192 (2018)

    CAS  Google Scholar 

  174. K. Yan, T.E. Simos, New Runge-Kutta type symmetric two-step method with optimized characteristics. J. Math. Chem. 56(8), 2454–2484 (2018)

    CAS  Google Scholar 

  175. L. Yang, T.E. Simos, An efficient and economical high order method for the numerical approximation of the Schrödinger equation. J. Math. Chem. 55(9), 1755–1778 (2017)

    CAS  Google Scholar 

  176. N. Yang, T.E. Simos, New four stages multistep in phase algorithm with best possible properties for second order problems. J. Math. Chem. 57(3), 895–917 (2019)

    CAS  Google Scholar 

  177. J. Yao, T.E. Simos, New finite difference pair with optimized phase and stability properties. J. Math. Chem. 56(2), 449–476 (2018)

    CAS  Google Scholar 

  178. J. Yao, T.E. Simos, New five-stages two-step method with improved characteristics. J. Math. Chem. 56(6), 1567–1594 (2018)

    CAS  Google Scholar 

  179. L. Zhang, T. E. Simos. An efficient numerical method for the solution of the Schrödinger equation. Adv. Math. Phys. (2016)

  180. W. Zhang, T.E. Simos, A high-order two-step phase-fitted method for the numerical solution of the Schrödinger equation. Mediterr. J. Math. 13(6), 5177–5194 (2016)

    Google Scholar 

  181. J. Zhao, T.E. Simos, A new fourteenth algebraic order finite difference method for the approximate solution of the Schrödinger equation. J. Math. Chem. 55(3), 697–716 (2017)

    CAS  Google Scholar 

  182. J. Zheng, C. Liu, T.E. Simos, A new two-step finite difference pair with optimal properties. J. Math. Chem. 56(3), 770–798 (2018)

    CAS  Google Scholar 

  183. Z. Zhou, T.E. Simos, A new two stage symmetric two-step method with vanished phase-lag and its first, second, third and fourth derivatives for the numerical solution of the radial Schrödinger equation. J. Math. Chem. 54(2), 442–465 (2016)

    CAS  Google Scholar 

  184. Z. Zhou, T.E. Simos, Three-stages tenth algebraic order two-step method with vanished phase-lag and its first, second, third and fourth derivatives. MATCH Commun. Math. Comput. Chem. 75(3), 653–694 (2016)

    Google Scholar 

Download references

Acknowledgements

This work was supported by Special Account for Research Funds(SARF) of Democritus University of Thrace-project no. 81896.

Author information

Authors and Affiliations

  1. Department of Civil Engineering, Polytechnic School of Xanthi, Democritus University of Thrace, Campus Xanthi, Kimmeria, 67100, Xanthi, Greece

    A. Konguetsof

Authors
  1. A. Konguetsof
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. Konguetsof.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

1.1 Phase-Lag Formula Confirmation

The phase-lag formula for \(k=1\) is obtained

$$\begin{aligned} PhL_1=T_0 + T_1 d_1=T_0+(A_1 v^6 + B_1 v^8) d_1 \end{aligned}$$
(66)

and for the value \(k=2\) takes the form

$$\begin{aligned} PhL_2= & {} T_0 + T_1 \prod _{j=2}^{2} d_j + T_2 \prod _{j=1}^{2} d_j \nonumber \\= & {} T_0+(A_1 v^6 + B_1 v^8) d_2 +(A_2 v^8 + B_2 v^{10}) d_1 d_2 \end{aligned}$$
(67)

The phase-lag formula for \(k=n\) and \(i=1, 2...,n\) is as follows

$$\begin{aligned} PhL_n=T_0+\sum _{i=1}^{n} \left( T_i \prod _{j=n+1-i}^{n} d_j \right) \end{aligned}$$
(68)

The phase-lag formula for \(k=n+1\) and consequently \(i=1, 2...,n+1\) is confirmed by the previous mathematical representations (66), (67) and (68)

$$\begin{aligned} PhL_{n+1}= & {} T_0+\sum _{i=1}^{n+1} \left( T_i \prod _{j=n+2-i}^{n+1} d_j \right) \nonumber \\= & {} T_0 + \sum _{i=1}^{n} \left( T_i \prod _{j=n+2-i}^{n+1} d_j \right) + T_{n+1} \prod _{j=1}^{n+1} d_j, \end{aligned}$$
(69)

that can be easily verified after expanding the sum and the product in an analytical way.

1.2 Confirmation of general formula for the system of equation for the solution of the unknown parameters

For a given family of methods, so that the value of k remains unchanged and the value of \(m \in {\mathbb {N}}\) with \(m \ge 3\) is variable, the following procedure describes our general expressions (31) given.

If \(m=3\) we obtain the equation

$$\begin{aligned} F^k(3)=-\frac{5}{3} d_{k-2} d_{k-1} d_{k} - \frac{5}{72} d_{k-1} d_{k} -\frac{1}{B_F}, \end{aligned}$$
(70)

where \(B_F=3628800\).

For \(m=n\) the expression obtained is

$$\begin{aligned}&F^k(n)=\frac{(-1)^{n}}{3} \frac{5}{ 2^{3-n} } \prod _{j=k-n+1}^{k} d_j \nonumber \\&+\frac{(-1)^{n}}{9} \frac{5}{ 2^{6-n} } \prod _{j=k-n+2}^{k} d_j + \frac{(-1)^n}{{B_F {\tilde{F}}^k_j(n)}} \nonumber \\&=\frac{(-1)^{n}}{3} \frac{5}{2^{3-n} } d_{k-n+1} d_{k-n}... d_{k-1} d_{k} \nonumber \\&+\frac{(-1)^{n}}{9} \frac{5}{ 2^{6-n} } d_{k-n+2} d_{k-n+1}... d_{k-1} d_{k}+ \frac{(-1)^n}{B_F {\tilde{F}}^k_j(n)}=0 , \end{aligned}$$
(71)

where \({\tilde{F}}^k_j(n)=\prod _{j=1}^{n-3} \left( 90+38j+4 j^2 \right) \).

For \(m=n+1\) the above expression takes the form

$$\begin{aligned}&F^k(n+1)=\frac{(-1)^{n+1}}{3} \frac{5}{ 2^{2-n} } \prod _{j=k-n}^{k} d_j \nonumber \\&+\frac{(-1)^{n+1}}{9} \frac{5}{ 2^{5-n} } \prod _{j=k-n+1}^{k} d_j + \frac{(-1)^{n+1}}{{B_F {\tilde{F}}^k_j(n+1)}} \nonumber \\&=\frac{(-1)^{n+1}}{3} \frac{5}{2^{2-n} } d_{k-n}... d_{k-1} d_{k} \nonumber \\&+\frac{(-1)^{n+1}}{9} \frac{5}{ 2^{5-n} } d_{k-n+1} ... d_{k-1} d_{k}+ \frac{(-1)^{n+1}}{B_F {\tilde{F}}^k_j(n+1)}=0 , \end{aligned}$$
(72)

where \({\tilde{F}}^k_j(n+1)=\prod _{j=1}^{n-2} \left( 90+38j+4 j^2 \right) \).

If \({\tilde{F}}^k_j(3)=\prod _{j=1}^{m-3} \left( 90+38j+4 j^2 \right) \), then for \(m=3\), we have \({\tilde{F}}^k_j(3)=\prod _{j=1}^{0} \left( 90+38j+4 j^2 \right) \), which is considered equal to one.

Respectively for \(m=n\) the quantity \(F^k_j(n)\) is

$$\begin{aligned} {\tilde{F}}^k_j(n)=\prod _{j=1}^{n-3} s^k(j) \end{aligned}$$
(73)

For \(m=n+1\) it becomes as follows

$$\begin{aligned} {\tilde{F}}^k_j(n+1)=\prod _{j=1}^{n-2} s^k(j), \end{aligned}$$
(74)

with \(s^k(j)=90+38j+4j^2\).

So that the general formula, which forms the system of equations (29)-(31) that leads to the values of the intermediate parameters, is verified.

1.3 Local Truncation Error for some families of the generator

The local truncation error \(LER_k\) is computed for the families with \(k=4,...,10\) and \(k=13\).

$$\begin{aligned} LER_4= & {} - {{7393} \over {32691859200}}\left( {{{{d^{14}}} \over {d{t^{14}}}}y\left( t \right) } \right) {h^{14}} \nonumber \\&+ {1 \over {10461394944000}}\left( {{{{d^{16}}} \over {d{t^{16}}}}y\left( t \right) } \right) {h^{16}} \nonumber \\&+ {1 \over {3201186852864000}}\left( {{{{d^{18}}} \over {d{t^{18}}}}y\left( t \right) } \right) {h^{18}} \nonumber \\&+{1 \over {1216451004088320000}}{\left( {{{{d^{20}}} \over {d{t^{20}}}}y\left( t \right) } \right) }{h^{20}} +... , \end{aligned}$$
(75)
$$\begin{aligned} LER_5= & {} {{{{591443}}} \over {{{31384184832000}}}}{\left( {{{{d^{16}}} \over {d{t^{16}}}}y\left( t \right) } \right) }{h^{16}} \nonumber \\&+ {1 \over {{{3201186852864000}}}}{\left( {{{{d^{18}}} \over {d{t^{18}}}}y\left( t \right) } \right) }{h^{18}} \nonumber \\&+ {1 \over {{ {1216451004088320000}}}}{\left( {{{{d^{20}}} \over {d{t^{20}}}}y\left( t \right) } \right) }{h^{20}} +... , \end{aligned}$$
(76)
$$\begin{aligned} LER_6= & {} - {{{ {10054529}}} \over {{ {6402373705728000}}}}{\left( {{{{d^{18}}} \over {d{t^{18}}}}y\left( t \right) } \right) }{h^{18}} \nonumber \\&+ {1 \over {{ {1216451004088320000}}}}{\left( {{{{d^{20}}} \over {d{t^{20}}}}y\left( t \right) } \right) }{h^{20}} +... , \end{aligned}$$
(77)
$$\begin{aligned} LER_7= & {} {{{ {136454323}}} \over {{ {1042672289218560000}}}}\left( {{{{d^{20}}} \over {d{t^{20}}}}y\left( t \right) } \right) {h^{20}}+... , \end{aligned}$$
(78)
$$\begin{aligned} LER_8= & {} - {{{ {136454323}}} \over {{ {12512067470622720000}}}}{\left( {{{{d^{22}}} \over {d{t^{22}}}}y\left( t \right) } \right) }{h^{22}}+... , \end{aligned}$$
(79)
$$\begin{aligned} LER_9= & {} - {1 \over {{ {562000363888803840000}}}}\left( {{{{d^{22}}} \over {d{t^{22}}}}y\left( t \right) } \right) {h^{22}} \nonumber \\&+ {{{ {14709776017}}} \over {{ {16185610479997550592000}}}}\left( {{{{d^{24}}} \over {d{t^{24}}}}y\left( t \right) } \right) {h^{24}}+... , \end{aligned}$$
(80)
$$\begin{aligned} LER_{10}= & {} - {1 \over {{ {562000363888803840000}}}}\left( {{{{d^{22}}} \over {d{t^{22}}}}y\left( t \right) } \right) {h^{22}} \nonumber \\&- {1 \over {{ {310224200866619719680000}}}}\left( {{{{d^{24}}} \over {d{t^{24}}}}y\left( t \right) } \right) {h^{24}} \nonumber \\&- {{{ {1691624241961}}} \over {{ {22336142462396619816960000}}}}\left( {{{{d^{26}}} \over {d{t^{26}}}}y\left( t \right) } \right) {h^{26}} +... , \end{aligned}$$
(81)
$$\begin{aligned} LER_{13}= & {} - {1 \over {{ {562000363888803840000I}}}} \left( {{{{d^{22}}} \over {d{t^{22}}}}y\left( t \right) } \right) {h^{22}} \nonumber \\&- {1 \over {{ {310224200866619719680000}}}} \left( {{{{d^{24}}} \over {d{t^{24}}}}y\left( t \right) } \right) {h^{24}} \nonumber \\&- {1 \over {20{ {1645730563302817792000000}}}} \left( {{{{d^{26}}} \over {d{t^{26}}}}y\left( t \right) } \right) {h^{26}} \nonumber \\&- {1 \over {{ { 1 52444172305856930250752000000}}}} \left( {{{{d^{28}}} \over {d{t^{28}}}}y\left( t \right) } \right) {h^{28}} \nonumber \\&- {1 \over {{ {132626429906095529318154240000000}}}} \left( {{{{d^{30}}} \over {d{t^{30}}}}y\left( t \right) } \right) {h^{30}} \nonumber \\&+ {{{ {20667575808031441}}} \over {{ {471560639666117437575659520000000}}}} \left( {{{{d^{32}}} \over {d{t^{32}}}}y\left( t \right) } \right) {h^{32}} +... \nonumber \\ \end{aligned}$$
(82)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Konguetsof, A. Algorithm for the development of families of numerical methods based on phase-lag Taylor series. J Math Chem 58, 178–203 (2020). https://doi.org/10.1007/s10910-019-01078-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10910-019-01078-1

Keywords

Search

Navigation