Statistical Properties of the 1D Space Fractional Klein–Gordon Oscillator

Abstract

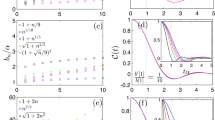
In this paper, we investigate the quantum fractional of the one-dimensional Klein–Gordon oscillator. By using a semiclassical approximation, the energy eigenvalues have been determined for oscillators. The obtained results show a remarkable influence of the fractional parameter $\alpha$ on the energy eigenvalues. By considering a unique energy spectrum, we present a simple numerical computation of the thermal properties of a defined energy spectrum of a system. The Euler–Maclaurin formula has been used to calculate the partition function and therefore the associated thermodynamics quantities. Besides this, we also calculate the eigenfunctions of our problem. The influence of the parameter $\alpha$ on these functions as well as the probability of density has been tested.

Appendix: Review of the Eigensolutions of 1D Harmonic Oscillator Using the Riesz–Feller Fractional Derivative

This appendix is a review of the method proposed by Rosu and Mancas [31]. The method is based on factorization algorithm and uses the Riesz–Feller fractional derivative. At first, its Olivar-Romero and Rosas-Ortiz [30] were first ones to apply the factorization method to a fractional quantum harmonic oscillator. Then Rosu and Mancas [31] apply the factorization algorithm to the fractional quantum harmonic oscillator along the lines previously proposed by Olivar-Romero and Rosas-Ortiz [30] by using the Riesz–Feller fractional derivative.

Starting with the space-fractional Schrödinger equation

$$\begin{aligned} \left[ -D_{\alpha }\hbar \frac{{\mathrm{{d}}}^{\alpha }}{{\text {d}}x^{\alpha }}+V\left( x\right) \right] \psi \left( x\right) =E\psi \left( x\right) . \end{aligned}$$

(56)

In his treatment about Harmonic oscillator, Laskin defined the form of the potential $V\left( x\right)$ with $\left| x\right| ^{\beta }$. The authors [30] fixed the parameter ($\beta =2$), and their arguments are that the arbitrariness of $\beta$ makes no substantial difference in the method. However, they have specialized their study of oscillator potential $V\left( x\right) =x^{2}$. The generalities of the method can be glimpsed the potential $V\left( x\right)$ with the form $\left| x\right| ^{\beta }$. The reason of this is that the effect of the fractional formulation is encoded in the momentum operator ${\hat{p}}^{\alpha }$ which is expressed in terms of the $\alpha$-order spatial derivative.

Now, using that $D_{\alpha }\hbar =1$, Eq. (56) becomes

$$\begin{aligned} H_{\alpha }\psi \left( x\right) =\left[ -\frac{{\mathrm{{d}}}^{\alpha }}{{\text {d}}x^{\alpha }}+x^{2} \right] \psi \left( x\right) =E\psi \left( x\right) ,\,1<\alpha \le 2. \end{aligned}$$

(57)

According to the factorization algorithm, we have the following: consider a pair of operators $A_{\alpha }$ and $B_{\alpha }$ such that

$$\begin{aligned} H_{\alpha }=B_{\alpha }A_{\alpha }+\epsilon _{\alpha } \end{aligned}$$

(58)

where $\epsilon _{\alpha }$ can be a fractional-differential operator. The operators $A_{\alpha }$, $B_{\alpha }$ and $\epsilon _{\alpha }$ are written as

$$\begin{aligned} A_{\alpha }=\frac{1}{\sqrt{\alpha }}\left( \frac{{\mathrm{{d}}}^{\alpha /2}}{{\text {d}}x^{\alpha /2}}+x\right) , \end{aligned}$$

(59)

$$\begin{aligned} B_{\alpha }=\frac{1}{\sqrt{\alpha }}\left( -\frac{{\mathrm{{d}}}^{\alpha /2}}{{\text {d}}x^{\alpha /2}}+x\right) , \end{aligned}$$

(60)

$$\begin{aligned} \epsilon _{\alpha }=\frac{1}{2}\frac{{\mathrm{{d}}}^{\alpha /2-1}}{{\text {d}}x^{\alpha /2-1}}. \end{aligned}$$

(61)

The method consists to solve the kernel equation of

$$\begin{aligned} A_{\alpha }\psi _{0}^{\alpha }=0\rightarrow \left( \frac{{\mathrm{{d}}}^{\alpha /2}}{{\text {d}}x^{\alpha /2}}+x\right) \psi _{0}^{\alpha }=0. \end{aligned}$$

(62)

Then, this fractional derivative equation will solve it in the momentum representation p. In this stage, Rosu and Macans [31] have used the Fourier transform of the Riesz–Feller derivative $d^{\alpha }/{\text {d}}x^{\alpha }$. The authors have been inspired by the works of Berman and Moiseyev [35] for their studies on the exceptional points in the Riesz–Feller Hamiltonian with an impenetrable rectangular potential [35]. As we known, the Fourier transform and its inversion are given by

$$\begin{aligned} f\left( k\right) ={\mathcal {F}}\left[ f\left( x\right) ,k\right] = \int _{-\infty }^{+\infty }f\left( x\right) e^{ikx}{\text {d}}x, \end{aligned}$$

(63)

$$\begin{aligned} f\left( x\right) ={\mathcal {F}}^{-1}\left[ f\left( k\right) ,x\right] = \frac{1}{2\pi }\int _{-\infty }^{+\infty }f\left( k\right) e^{-ikx}{\text {d}}x. \end{aligned}$$

(64)

Now, the Riesz–Feller fractional derivative $_{x}D_{\theta }^{\alpha }$ is defined through a relation [36]

$$\begin{aligned} {\mathcal {F}}\left[ _{x}D_{\theta }^{\alpha }\psi \left( x\right) ,k\right] = -\Psi _{\alpha }^{\theta }\left( k\right) \varPhi \left( k\right) , \end{aligned}$$

(65)

where

$$\begin{aligned} _{x}D_{\theta }^{\alpha }\psi \left( x\right) =-\frac{1}{2\pi } \int _{-\infty }^{+\infty }\Psi _{\alpha }^{\theta }\left( k\right) e^{-ikx}{\text {d}}x \int \psi \left( x'\right) e^{ikx'}{\text {d}}x', \end{aligned}$$

(66)

and

$$\begin{aligned} \Psi _{\alpha }^{\theta }\left( k\right) = \left| k\right| ^{\alpha }e^{i{\text {sign}}\left( k\right) \frac{\theta \pi }{2}},\, 0<\alpha \le 2,\,{\text {and}}\,\left| \theta \right| \le \min \left\{ \alpha ,2-\alpha \right\} . \end{aligned}$$

(67)

The allowed region for the parameters $\alpha$ and $\theta$ turns out to be a diamond in the plane $\left( \alpha ,\theta \right)$ that are called the Feller–Takayasu diamond [31, 35, 37].

Thus, Eqs. (59) and (60) become

$$\begin{aligned} A_{k,\alpha }=\psi _{\alpha /2}^{\theta }+i\frac{{\mathrm{{d}}}}{{\text {d}}k},\,B_{k,\alpha }= \psi _{\alpha /2}^{\theta }-i\frac{{\mathrm{{d}}}}{{\text {d}}k}, \end{aligned}$$

(68)

and the solutions of the kernel $A_{k,\alpha }$ are

$$\begin{aligned} \phi _{0}^{\alpha }=e^{-\frac{\left| k\right| ^{\alpha /2+1}}{\alpha /2+1}}. \end{aligned}$$

(69)

The others solutions are found by the repeated usage of the operator $B_{k,\alpha }$.

Table 2 The first four form of ${\tilde{H}}_{n}$ [31]

Rosu and Mancas have proved one can write

$$\begin{aligned} \phi _{n}\left( k\right) =i^{n}{\tilde{H}}_{n}\phi _{0}^{\alpha } \end{aligned}$$

(70)

where ${\tilde{H}}_{n}$ are the fractionally deformed Hermite polynomials (some form of this function are listed in Table 2). They also call Riesz–Feller Hermite polynomials.