Skip to main content
SpringerLink
Log in

Periodic Motions for Multi-wells Potentials and Layers Dynamic for the Vector Allen–Cahn Equation

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

We consider a nonnegative potential W that vanishes on a finite set and study the existence of periodic orbits of the equation

$$\begin{aligned} \ddot{u}=W_u(u),\;\;t\in {\mathbb {R}}, \end{aligned}$$

that have the property of visiting neighborhoods of zeros of W in a given finite sequence. We give conditions for the existence of such orbits. After introducing the new variable \(x=\epsilon t\), \(\epsilon >0\) small, these orbits correspond to stationary solutions of the parabolic equation

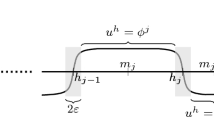
$$\begin{aligned} u_t=u_{xx}-W_u(u),\;\;x\in (0,1),\;t>0, \end{aligned}$$

with periodic boundary conditions. In the second part of the paper we study solutions of this equation that, as the stationary solutions, have a layered structure. We derive a system of ODE that describes the dynamics of the layers and show that their motion is extremely slow.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Alessio, F., Montecchiari, P.: Brake orbits type solutions to some class of semilinear elliptic equations. Calc. Var. Part. Differ. Equ. 30(1), 51–83 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  2. Alessio, F., Montecchiari, P., Zuniga, A.: Prescribed energy connecting orbits for gradient systems. Discrete Contin. Dyn. Syst. 38(8), 4895–4928 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  3. Alikakos, N.D., Bates, P., Fusco, G.: Slow motion for the Cahn–Hilliard equation in one space dimension. J. Differ. Equ. 90, 81–131 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  4. Alikakos, N.D., Betelu, S.I., Chen, X.: Explicit stationary solutions in multiple well dynamics and non-uniqueness of interfacial energy densities. Eur. J. Appl. Math. 17, 525–556 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  5. Alikakos, N.D., Bronsard, L., Fusco, G.: Slow motion in the gradient theory of phase transitions via energy and spectrum. Calc. Var. Part. Differ. Equ. 6(1), 39–66 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  6. Alikakos, N.D., Fusco, G.: On the connection problem for the potentials with several global minima. Indiana Univ. Math. J. 57(4), 1871–1906 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  7. Alikakos, N., Fusco, G.: Slow dynamics for the Cahn–Hilliard equation in higher space dimensions: the motion of bubbles. Arch. Ration. Mech. Anal. 141, 1–61 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  8. Alikakos, N.D., Fusco, G., Kowalczyk, M.: Finite dimensional dynamics and interfaces intersecting the boundary: equilibria and quasi-invariant manifold. Indiana Univ. Math. J. 45(4), 1119–1155 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  9. Antonopoulos, A., Smyrnelis, P.: On minimizers of the Hamiltonian system \(u^{\prime \prime }=\nabla W(u)\) and on the existence of heteroclinic, homoclinic and periodic orbits. Indiana Univ. Math. J. 65(4), 1503–1524 (2016)

    MathSciNet  MATH  Google Scholar 

  10. Bates, P.W., Xun, J.P.: Metastable patterns for the Cahn–Hilliard equation: Part I. J. Differ. Equ. 111(2), 421–457 (1994)

    Article  MATH  Google Scholar 

  11. Bates, P.W., Fusco, G., Karaly, G.: Gradient dynamics: motion near a manifold of quasi-equilibria. SIAM J. Appl. Dyn. Syst. 17(3), 2106–2145 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  12. Bates, P.W., Xun, J.: Metastable patterns for the Cahn–Hilliard equation: part II. Layer dynamics and slow invariant manifold. J. Differ. Equ. 117, 165–216 (1995)

    Article  MATH  Google Scholar 

  13. Bellettini, G., Nayam, A.A., Novaga, M.: \(\Gamma \)-type estimates for the one-dimensional Allen–Cahn’s action. Asymptot. Anal. 94(1–2), 161–185 (2015)

    MathSciNet  MATH  Google Scholar 

  14. Bethuel, F., Orlandi, G., Smets, D.: Slow motion for gradient systems with equal depth multiple-well potentials. J. Differ. Equ. 250(1), 53–94 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  15. Bethuel, F., Smets, D.: Slow motion for equal depth multiple-well gradient systems: the degenerate case. DCDS-A 33(1), 67–87 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  16. Bronsard, L., Kohn, R.V.: On the slowness of phase boundary motion in one space dimension. Commun. Pure Appl. Math. 43, 983–997 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  17. Bronsard, L., Hilhorst, D.: On the slow dynamics for the Cahn–Hilliard equation in one space dimension. Proc. R. Soc. Lond. A 439, 669–682 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  18. Cabré, X., Fontich, E., de la Llave, R.: The parameterization method for invariant manifolds I: manifolds associated to non-resonant subspaces. Indiana Univ. math. J. 52, 283–328 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  19. Chaperon, M.: Invariant manifolds revisited. Proc. Steklov Inst. Math. 236, 415–433 (2002)

    MathSciNet  MATH  Google Scholar 

  20. Carr, J., Pego, R.L.: Metastable patterns in solutions of \(u_t=\epsilon ^2u_{xx}-f(u)\). Commun. Pure Appl. Math. 42, 523–576 (1989)

    Article  MATH  Google Scholar 

  21. Carr, J., Pego, R .L.: Metastable patterns in solutions of \(u_t=\epsilon ^2u_{xx}-f(u)\). Proc. R. Soc. Edinb. 116(A), 133–160 (1990)

    Article  MATH  Google Scholar 

  22. Ei, S.I., Yanagida, E.: Slow dynamics of interfaces in the Allen–Cahn equation on a strip-like domain. SIAM J. Math. Anal. 29(3), 555–595 (1998). (electronic)

    Article  MathSciNet  MATH  Google Scholar 

  23. de la Llave, R.: Invariant manifolds associated to nonresonant spectral subspaces. J. Stat. Phys. 87, 211–249 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  24. Folino, R., Lattanzio, C., Mascia, C.: Slow dynamics for the hyperbolic Cahn–Hilliard equation in one space dimension. Math. Methods Appl. Sci. 42(8), 2492–2512 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  25. Fusco, G.: A geometric approach to the dynamics of \(u_t=\epsilon ^2u_{xx}+f(u)\) for small \(\epsilon \), problems involving change of type K. Kirchgässner Ed. Lect. Notes Phys. 359, 53–73 (1990)

    Article  Google Scholar 

  26. Fusco, G., Gronchi, G.F., Novaga, M.: Existence of periodic orbits near heteroclinic connections. Minimax Theory Appl. 4(1), 113–149 (2019)

    MathSciNet  MATH  Google Scholar 

  27. Fusco, G., Hale, J.K.: Slow motion manifolds, dormant instability and singular perturbations. J. Dyn. Differ. Equ. 1, 75–94 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  28. Grant, C.: Slow motion in one-dimensional Cahn–Morral systems. SIAM. J. Math. Anal. 26, 21–34 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  29. Mascia, C., Strani, M.: Metastability for nonlinear parabolic equations with application to scalar viscous conservation laws. SIAM J. Math. Anal. 45(9), 3084–3113 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  30. Matano, H., Polácik, P.: Dynamics of nonnegative solutions of one-dimensional reaction–diffusion equations with localized initial data. Part I: a general quasiconvergence theorem and its consequences. Commun. Part. Differ. Equ. 41(5), 1–27 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  31. Matano, H., Polácik, P.: Dynamics of nonnegative solutions of one-dimensional reaction–diffusion equations with localized initial data. Part II: generic nonlinearities. Commun. Part. Differ. Equ. 45(6), 483–524 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  32. Monteil, A., Santambrogio, F.: Metric methods for heteroclinic connections. Math. Methods Appl. Sci. 41(3), 1019–1024 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  33. Murray, R., Rinaldi, M.: Slow motion for the nonlocal Allen–Cahn equation in n-dimensions. Calc. Var. 55, 147 (2016). https://doi.org/10.1007/s00526-016-1086-4

    Article  MathSciNet  MATH  Google Scholar 

  34. Neu, J.: Unpublished lecture notes

  35. Otto, F., Reznikoff, M.G.: Slow motion of gradient flows. J. Differ. Equ. 237, 372–420 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  36. Rabinowitz, P.: Homoclinic and heteroclinic orbits for a class of Hamiltonian systems. Calc. Var. PDE Calc. Var. PDE 1, 1–36 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  37. Smyrnelis, P.: Connecting orbits in Hilbert spaces and application to PDE. Commun. Pure Appl. Anal. 19(5), 2797–2818 (2019). https://doi.org/10.3934/cpaa.2020122

  38. Sourdis, C.: The heteroclinic connection problem for general double-well potentials. Mediterr. J. Math. 13, 4693–4710 (2016). https://doi.org/10.1007/s00009-016-0770-0

  39. Ward, M.J.: Metastable patterns, layers collapses and coarsening for a one dimensional Ginzburg-Landau equation. Stud. Appl. Math. 91, 51–93 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  40. Ward, M.J.: Metastable dynamics and exponential asymptotics in multi-dimensional domains, Multiple-Time-Scale Dynamical Systems C.K.R.T. Jones and A.I. Khibnik Edrs. IMA Math. Appl. 122, 233–259 (2001)

    Google Scholar 

  41. Zuniga, A., Sternberg, P.: On the heteroclinic connection problem for multi-well gradient systems. J. Differ. Equ. 261(7), 3987–4007 (2016). https://doi.org/10.1016/j.jde.2016.06.010

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica Pura ed Applicata, Università degli Studi dell’Aquila, Via Vetoio, 67010, Coppito, L’Aquila, Italy

    Giorgio Fusco

Authors
  1. Giorgio Fusco
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Giorgio Fusco.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fusco, G. Periodic Motions for Multi-wells Potentials and Layers Dynamic for the Vector Allen–Cahn Equation. J Dyn Diff Equat 34, 3165–3215 (2022). https://doi.org/10.1007/s10884-021-09949-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10884-021-09949-5

Keywords

Search

Navigation