Abstract
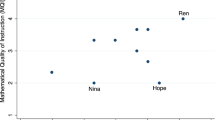
This paper reports findings from a study that establishes empirical support for Harel’s (Zentralblatt für Didaktik der Math 40:893–907 2008b) inclusion of mathematical ways of thinking as a component of teachers’ professional knowledge base. Specifically, we examined the role of quantitative reasoning (Smith and Thompson, in: Kaput, Carraher, Blanton (eds) Algebra in the early grades, Erlbaum, New York 2007; Thompson, in: A theoretical model of quantity-based reasoning in arithmetic and algebra, Center for Research in Mathematics & Science Education: San Diego State University 1990; Thompson, in: Hatfield et al (eds) New perspectives and directions for collaborative research in mathematics education, University of Wyoming, Laramie 2011) on the quality and coherence of an experienced secondary teacher’s instruction of angle measure. We analyzed 37 videos of the teacher’s instruction to characterize the extent to which he attended to supporting students in reasoning about angle measure quantitatively, and to examine the consequences of this attention on the quality and coherence of the meanings the teacher’s instruction supported. Our analysis revealed that the inconsistent meanings the teacher conveyed were occasioned by his lack of awareness of the conceptual affordances of students’ quantitative reasoning on their ability to construct coherent, meaningful understandings of angle measure. Our findings therefore support Harel’s notion that teachers’ mathematical ways of thinking constitute an essential component of their specialized content knowledge.







Similar content being viewed by others
Notes
PCK refers to the character of subject matter knowledge required for effective teaching, which includes an awareness of “the ways of representing and formulating the subject that make it comprehensible to others” (Shulman 1986, p. 9).
We define quantity below, as well as several other constructs within the theory of quantitative reasoning. For now it suffices to say that a quantity is a measurable attribute of an object and that quantitative reasoning involves reasoning about quantities and their relationships.
By “teachers’ attention to quantitative reasoning,” we are referring to teachers’ awareness of the conceptual affordances of students’ quantitative reasoning on their ability to construct coherent, meaningful understandings of specific mathematical ideas. We are also referring to teachers’ instructional actions motivated by this awareness.
It is important to note that iconic assignments of numerical values as measures of particular angles is not an instance of quantification (e.g., assigning 90° to a right angle, 180° to a line, and 360° to a circle) because such assignments are not based on an interiorized measurement process (Moore 2013).
We acknowledge that this definition of meaning necessarily implies a multiplicity of meanings conveyed.
“Incoherence” is often used in a denigratory sense. We stress that by defining coherence as a property of a system of cognitive schemes, our use of “incoherent” is as an explanatory construct, not a pejorative.
For ease of communicating, we henceforth use “students” instead of “our construction of a generalized Algebra II student.”
National Board Certification is a voluntary, peer-reviewed, and performance-based certification program for schoolteachers in the United States (http://www.nbpts.org/national-board-certification).
There are an odd number of classes because in one of David’s afternoon classes, he taught content unrelated to angle measure, sine, and cosine.
We reiterate that this is an important meaning for students to construct since one can deduce from it that any unit of angle measure must be proportional to the circumference of the circle centered at an angle’s vertex. However, this abstraction of the essential criterion that any unit of angle measure must satisfy is only available to those who have coordinated this way of understanding with the meaning of angle measure as the length of a class of subtended arcs.
See Moore et al. (2016) for similar findings about pre-service teachers’ meanings for the unit circle.
A comprehensive discussion of the implications of a quantitative understanding of angle measure for supporting students’ learning of other important trigonometry concepts is beyond the scope of this paper. We refer the interested reader to Thompson et al. (2007) for an analysis of the many ways in which conceptualizing angle measure quantitatively is a precondition to developing a coherent understanding of trigonometric functions.
References
Akkoc, H. (2008). Pre-service mathematics teachers’ concept images of radian. International Journal of Mathematical Education in Science and Technology,39(7), 857–878.
Carlson, M., Jacobs, S., Coe, E., Larsen, S., & Hsu, E. T. (2002). Applying covariational reasoning while modeling dynamic events: A framework and a study. Journal for Research in Mathematics Education,33(5), 352–378.
Carlson, M., O’Bryan, A., & Joyner, K. (2013). Pathways algebra II: Implementing the common core mathematics standards (3rd ed.). Gilbert, AZ: Rational Reasoning LLC.
Castillo-Garsow, C. (2010). Teaching the verhulst model: A teaching experiment in covariational reasoning and exponential growth (unpublished doctoral dissertation). Tempe: Arizona State University.
Confrey, J., & Smith, E. (1995). Splitting, covariation and their role in the development of exponential functions. Journal for Research in Mathematics Education,26(1), 66–86.
Corbin, J., & Strauss, A. (2008). Basics of qualitative research: Techniques and procedures for developing grounded theory (3rd ed.). Los Angeles, CA: Sage Publications Inc.
Dunkin, M. J., & Biddle, B. J. (1974). The study of teaching. New York: Hold, Rinehart, & Winston.
Ellis, A. B. (2007). The influence of reasoning with emergent quantities on students’ generalizations. Cognition and Instruction,25(4), 439–478.
Fennema, E., & Franke, L. M. (1992). Teachers’ knowledge and its impact. In D. A. Grouws (Ed.), Handbook of research on mathematics teaching and learning (pp. 147–164). New York: Macmillan.
Goldin, G. A. (1997). Observing mathematical problem solving through task-based interviews. Journal for Research in Mathematics Education. Monograph,9, 40–177.
Harel, G. (2008a). DNR perspective on mathematics curriculum and instruction part I: Focus on proving. Zentralblatt für Didaktik der Mathematik,40, 487–500.
Harel, G. (2008b). A DNR perspective on mathematics curriculum and instruction, part II: With reference to teacher’s knowledge base. Zentralblatt für Didaktik der Mathematik,40, 893–907.
Harel, G. (2008c). What is mathematics? A pedagogical answer to a philosophical question. In R. B. Gold & R. Simons (Eds.), Proof and other dilemmas: Mathematics and philosophy (pp. 265–290). Washington, DC: Mathematical Association of America.
Hertel, J., & Cullen, C. (2011). Teaching trigonometry: A directed length approach. In L. R. Wiest & T. Lamberg (Eds.), Proceedings of the 33rd annual meeting of the North American chapter of the international group for the psychology of mathematics education. Reno, NV: University of Nevada, Reno.
Hill, H. C., Ball, D. L., & Schilling, S. G. (2008). Unpacking pedagogical content knowledge: Teachers’ topic-specific knowledge of students. Journal for Research in Mathematics Education,39(4), 372–400.
Jonckheere, A., Mandelbrot, B., & Piaget, J. (1958). La lecture de l’expérience. Paris: Presses Universitaires de France.
Ma, L. (1999). Knowing and teaching elementary mathematics. Mahwah, NJ: Lawerence Erlbaum Associates Inc.
Montangero, J., & Maurice-Naville, D. (1997). Piaget: Or, the advance of knowledge. London: Routledge.
Moore, K. C. (2012). Coherence, quantitative reasoning, and the trigonometry of students. In R. Mayes & L. L. Hatfield (Eds.), Quantitative reasoning and mathematical modeling: A driver for STEM integrated education and teaching in context (pp. 75–92). Laramie, WY: University of Wyoming.
Moore, K. C. (2013). Making sense by measuring arcs: A teaching experiment in angle measure. Educational Studies in Mathematics,83(2), 225–245.
Moore, K. C. (2014). Quantitative reasoning and the sine function: The case of Zac. Journal for Research in Mathematics Education,45(1), 102–138.
Moore, K. C., & Carlson, M. P. (2012). Students’ images of problem contexts when solving applied problems. Journal of Mathematical Behavior,31(1), 48–59.
Moore, K. C., LaForest, K. R., & Kim, H. J. (2016). Putting the unit in pre-service secondary teachers’ unit circle. Educational Studies in Mathematics,92(2), 221–241.
Oehrtman, M., Carlson, M. P., & Thompson, P. W. (2008). Foundational reasoning abilities that promote coherence in students’ function understanding. In M. P. Carlson & C. Rasmussen (Eds.), Making the connection: Research and practice in undergraduate mathematics (pp. 150–171). Washington, DC: Mathematical Association of America.
Piaget, J. (1972). Principles of genetic epistemology. New York: Routledge.
Rowland, T., Huckstep, P., & Thwaites, A. (2003). The knowledge quartet. Proceedings of the British Society for Research into Learning Mathematics,23(3), 97–102.
Rowland, T., Huckstep, P., & Thwaites, A. (2005). Elementary teachers’ mathematics subject knowledge: The knowledge quartet and the case of Naomi. Journal of Mathematics Teacher Education,8(3), 255–281.
Saldanha, L., & Thompson, P. W. (1998). Re-thinking covariation from a quantitative perspective: Simultaneous continuous variation. Paper presented at the Annual Meeting of the Psychology of Mathematics Education-North America, Raleigh, NC: North Carolina State University.
Shulman, L. S. (1986). Those who understand: Knowledge growth in teaching. Educational Researcher,15(2), 4–14.
Shulman, L. S., & Quinlan, K. M. (1996). The comparative psychology of school subjects. In D. C. Berliner & R. C. Calfee (Eds.), Handbook of educational psychology (pp. 399–422). New York: Simon & Schuster Macmillan.
Smith, J., & Thompson, P. W. (2007). Quantitative reasoning and the development of algebraic reasoning. In J. J. Kaput, D. W. Carraher, & M. L. Blanton (Eds.), Algebra in the early grades (pp. 95–132). New York: Erlbaum.
Steffe, L. P., & Thompson, P. W. (2000). Interaction or intersubjectivity? A reply to Lerman. Journal for Research in Mathematics Education,31(2), 191–209.
Stigler, J. W., & Hiebert, J. (1999). The teaching gap: Best ideas from the world’s teachers for improving education in the classroom. New York: Free Press.
Strauss, A., & Corbin, J. (1990). Basics of qualitative research: Grounded theory procedures and techniques. London: Sage Publications.
Tallman, M. (2015). An examination of the effect of a secondary teacher’s image of instructional constraints on his enacted subject matter knowledge. Unpublished Ph.D. dissertation, School of Mathematical and Statistical Sciences, Arizona State University.
Thompson, P. W. (1990). A theoretical model of quantity-based reasoning in arithmetic and algebra. Center for Research in Mathematics & Science Education: San Diego State University.
Thompson, P. W. (1994). The development of the concept of speed and its relationship to concepts of rate. In G. Harel & J. Confrey (Eds.), The development of multiplicative reasoning in the learning of mathematics (pp. 181–234). Albany, NY: SUNY Press.
Thompson, P. W. (2000). Radical constructivism: Reflections and directions. In L. P. Steffe & P. W. Thompson (Eds.), Radical constructivism in action: Building on the pioneering work of Ernst von Glasersfeld (pp. 291–315). London: RoutledgeFalmer.
Thompson, P. W. (2008). Conceptual analysis of mathematical ideas: Some spadework at the foundation of mathematics education. Proceedings of the Annual Meeting of the International Group for the Psychology of Mathematics Education,1, 45–64.
Thompson, P. W. (2011). Quantitative reasoning and mathematical modeling. In L. L. Hatfield, S. Chamberlain, & S. Belbase (Eds.), New perspectives and directions for collaborative research in mathematics education (pp. 33–57). Laramie, WY: University of Wyoming.
Thompson, P. W. (2013). In the absence of meaning. In K. Leatham (Ed.), Vital directions for research in mathematics education (pp. 57–90). New York: Springer.
Thompson, P. W. (2016). Researching mathematical meanings for teaching. In L. English & D. Kirshner (Eds.), Third handbook of international research in mathematics education (pp. 435–461). New York: Taylor and Francis.
Thompson, P. W., & Carlson, M. P. (2017). Variation, covariation, and functions: Foundational ways of thinking mathematically. In J. Cai (Ed.), Compendium for research in mathematics education (pp. 421–456). Reston, VA: National Council of Teachers of Mathematics.
Thompson, P. W., Carlson, M. P., Byerley, C., & Hatfield, N. (2014). Schemes for thinking with magnitudes: An hypothesis about foundational reasoning abilities in algebra. In K. C. Moore, L. P. Steffe, & L. L. Hatfield (Eds.), Epistemic algebra students: Emerging models of students’ algebraic knowing (pp. 1–24)., WISDOMe Monographs Laramie, WY: University of Wyoming.
Thompson, P. W., Carlson, M. P., & Silverman, J. (2007). The design of tasks in support of teachers’ development of coherent mathematical meanings. Journal of Mathematics Teacher Education,10, 415–432.
Thompson, A. G., & Thompson, P. W. (1996). Talking about rates conceptually, part II: Mathematical knowledge for teaching. Journal for Research in Mathematics Education,27(1), 2–24.
von Glassersfeld, E. (1995). Radical constructivism: A way of knowing and learning. New York: RoutledgeFalmer.
Weber, K. (2005). Students’ understanding of trigonometric functions. Mathematics Education Research Journal,17(3), 91–112.
Acknowledgements
This manuscript is based on data used in the lead author’s doctoral dissertation. This research was supported by National Science Foundation Grant 943360412. Any conclusions and recommendations stated here are those of the authors and do not necessarily reflect official positions of the NSF. We thank Dr. Marilyn P. Carlson and Dr. Kevin Moore for providing feedback on a previous version of this paper. Finally, we thank SIGMAA on RUME for the opportunity to present a previous version of this paper at their 20th annual conference.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Tallman, M.A., Frank, K.M. Angle measure, quantitative reasoning, and instructional coherence: an examination of the role of mathematical ways of thinking as a component of teachers’ knowledge base. J Math Teacher Educ 23, 69–95 (2020). https://doi.org/10.1007/s10857-018-9409-3
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10857-018-9409-3