Impact of Technical Progress on the Relationship Between Competition and Investment

Abstract

This paper investigates the impact of technical progress on the relationship between competition and investment. Using a model of oligopolistic competition with differentiated products in which firms invest to reduce their marginal cost of production (or to improve quality), I find that technical progress, by increasing the size of innovation, defined as the drop in marginal costs (or the increase in quality) obtained for a given investment amount, increases total investment and decreases the level of competition that maximizes investment in the industry. This feature also holds for consumer surplus and welfare. This means that innovative industries maximize consumer surplus and welfare at a lower level of competitive pressure than do less innovative industries. In the model, competition is measured either by the number of competitors or by the degree of horizontal substitutability between offers. The results hold for both measures, subject to a relatively steady industry specific rate of technological change.

Appendix

1.1 Proof of Lemma 1

The relationship between the number of firms and industry-level investment is decreasing or inverted U-shaped.

Investment at industry level is ${F}_{ind}=\frac{n\tau {q}^2}{2}$.

Let us denote $F{\hbox{'}}_{ind}=\frac{\partial {F}_{ind}}{\partial n},q"=\frac{\partial q\hbox{'}}{\partial n},\mathrm{and}\ F{"}_{ind}=\frac{\partial F{\hbox{'}}_{ind}}{\partial n}$.

We can write $F{\hbox{'}}_{ind}=\tau q\left(\frac{q}{2}+ nq\hbox{'}\right)\ and\ F{"}_{ind}=\tau {q}^{\hbox{'}}\left(\frac{q}{2}+ nq\hbox{'}\right)+\tau q\left(\frac{3q\hbox{'}}{2}+ nq"\right)$.

First-order condition (F_ind = 0) provides the number of firms for which investment of the industry reaches an optimum. This optimum is a maximum if the second order condition (F"_ind < 0) is verified.

I will first show that if there is an optimum, then it is a maximum and it is unique. That is to say if F_ind = 0 and F"_ind < 0.

From Eq. (6),

$$ q"=\frac{2{\gamma}^2\left({\left(1+\gamma \left(n-2\right)\right)}^3+\left(1-\gamma \right)\gamma \left(\tau -1-3\left(1+\gamma \left(n-2\right)\right)\right)\right)\gamma \left(a-{c}_0\right)}{{\left(\left(1-\gamma \right)\left(1+\gamma \left(n-1\right)\right)-\left(1+\gamma \left(n-2\right)\right)\tau +\left(1+\gamma \left(n-1\right)\right)\left(1+\gamma \left(n-2\right)\right)\right)}^3} $$

(11)

Functions F_ind, F'_ind, and F"_ind are continuous for n ∈ ℝ and n ≥ 1 because the denominator of Eqs. (5), (6), and (11) are different from zero since τ < 2.

If there is an optimum, then F^' = 0; therefore, $\frac{q}{2}+n{q}^{\hbox{'}}=0$ or τq = 0. Yet by definition, τ > 0 and q > 0 since a > c₀. As a result, if there is an optimum for n = n^*, then ${n}^{\ast }=-\frac{q}{2q\hbox{'}}$.

At the optimum, the sign of F"_ind is the sign of $\frac{3q\hbox{'}}{2}+ nq"$ because τq is strictly positive.

We can write $\frac{3q\hbox{'}}{2}+{n}^{\ast }q"=\frac{3{q}^{\hbox{'}}- qq"}{2q\hbox{'}}$ using ${n}^{\ast }=-\frac{q}{2q\hbox{'}}$.

From Eq. (6), we can check that for n ≥ 2, q’ < 0; thus, the sign of F"_ind is the sign of qq "  − 3q'.

Using Eqs. (5), (6), and (11), we can write the following:

$$ \kern0.5em qq\hbox{'}\hbox{'}-3q\hbox{'}=-\frac{\left(3{\left(1-\gamma \right)}^2{\gamma}^4+{\gamma}^2{\left(1+\gamma \left(n-2\right)\right)}^4+2{\gamma}^3\left(1-\gamma \right)\left(1+\gamma \left(n-2\right)\right)\left(1-\tau \right)\right){\left(a-{c}_0\right)}^2}{{\left(\left(1-\gamma \right)\left(1+\gamma \left(n-1\right)\right)-\left(1+\gamma \left(n-2\right)\right)\tau +\left(1+\gamma \left(n-1\right)\right)\left(1+\gamma \left(n-2\right)\right)\right)}^4} $$

The denominator of this expression, as those of Eqs. (5), (6), and (11), is strictly positive since τ ∈ ]0, 2[.

If n ≥ 2, then 1 + γ(n − 2) ≥ 1, and thus, 2γ³(1 − γ)(1 + γ(n − 2))(1 − τ) ≥ 2γ³(1 − γ)(1 − τ).

Furthermore, (1 − τ) >  − 1, and as a result, $2{\gamma}^3\left(1-\gamma \right)\left(1-\tau \right)>-\frac{\gamma^2}{2}$, the numerator is positive.

This means that qq "  − 3q' is negative and thus F"_ind ≤ 0. The optimum is a maximum.

In summary, if ∃n^* ≥ 2 such that F ′ (n^*) = 0 then F"_ind(n^*) ≤ 0 then n^* is a maximum.

This maximum is unique because there is no minimum. Indeed, it is not possible to have a second relative maximum without a relative minimum as functions F_ind and F'_ind are continuous.

If there is no optimum, the relationship cannot be monotonically increasing because F_ind(n) = τq²/2, and from equation (5)

$$ \underset{n\to +\infty }{\lim }{F}_{ind}(n)=0 $$

If investment of the industry is maximized for n = 1, then function F_ind(n) is monotonically decreasing because there is no relative minimum.

As a result, the relationship between the number of firms and industry-level investment is inverted U-shaped or monotonically decreasing.

Proof of Proposition 1: From Lemma 1, the relationship between the number of firms and industry-level investment is inverted U-shaped or monotonically decreasing.If the relationship is inverted U-shaped, the number of firms n^* that maximizes industry-level investment is such that F ′ (n^*) = 0, which means n^* =  − q/2q'.

How does n^* move when technical progress, τ, increases?

Output q tends to increase with technical progress, deriving q from Eq. (5) with respect to τ yields:

$$ \frac{\partial q}{\partial \tau }=\frac{q^2}{\left(a-{c}_0\right)} $$

Moreover, deriving q’ from Eq. (6) yields:

$$ \frac{\partial q\hbox{'}}{\partial \tau }=\frac{2 qq\hbox{'}}{\left(a-{c}_0\right)} $$

As a result,

$$ \frac{\partial {n}^{\ast }}{\partial \tau }=\frac{q^2}{2q\hbox{'}\left(a-{c}_0\right)} $$

If n^* ≥ 2 then q^' < 0; therefore, $\frac{\partial {n}^{\ast }}{\partial \tau}\le 0$. This means that if the relationship between competition and investment of the industry is inverted U-shaped, the number of firms that maximizes investment of the industry decreases with technical progress.

If the relationship is monotonically decreasing, this means that n^* = 1.

Investment of the industry is ${F}_{ind}(1)=\frac{\tau {\left(a-{c}_0\right)}^2}{2{\left(2-\tau \right)}^2}$; furthermore, ${F}_{ind}(2)=\frac{\tau {\left(a-{c}_0\right)}^2}{{\left(2-\tau +\gamma \left(1-\gamma \right)\right)}^2}$;

F_ind decreasing means F_ind(1) > F_ind(2), and $\tau >2-\frac{\gamma \left(1-\gamma \right)}{\sqrt{2}-1}$. What happens if τ increases?

$\frac{\partial {F}_{ind}(1)}{\partial \tau }=\frac{\left(4-{\tau}^2\right){\left(a-{c}_0\right)}^2}{2{\left(2-\tau \right)}^4}$ and $\frac{\partial {F}_{ind}(2)}{\partial \tau }=\frac{\left(4-{\tau}^2+\gamma \left(1-\gamma \right)\left(4+\gamma \left(1-\gamma \right)\right)\right){\left(a-{c}_0\right)}^2}{{\left(2-\tau +\gamma \left(1-\gamma \right)\right)}^4}$

We can check that if $\tau >2-\frac{\gamma \left(1-\gamma \right)}{\sqrt{2}-1}$, then $\frac{\partial {F}_{ind}(1)}{\partial \tau }>\frac{\partial {F}_{ind}(2)}{\partial \tau }$.

This means that if the relationship is monotonically decreasing, an increase in technical progress widens the gap and keeps the decreasing shape of the curve. In any case, an increase in technical progress tends to decrease (never increase) the number of firms that maximizes investment of the industry.

1.2 Proof of Lemma 2

Let us denote $C{S}^{\hbox{'}}=\frac{\partial CS}{\partial n}$ and $W\hbox{'}=\frac{\partial W}{\partial n}$

From Eq. (7), it can be written as follows:

$$ C{S}^{\hbox{'}}=\frac{F{\hbox{'}}_{ind}\left(1+\gamma \left(n-1\right)\right)+\gamma {F}_{ind}}{\tau } $$

And from Eq. (8):

$$ {W}^{\hbox{'}}=\left(a-{c}_0\right)\left(q+ nq\hbox{'}\right)+F{\hbox{'}}_{ind}- CS\hbox{'} $$

If $\tau \le \frac{3\left(1-\gamma \right)}{2}$, then CS^' > 0 which means that CS is monotonically increasing.

If $\tau >\frac{3\left(1-\gamma \right)}{2}$, then CS^' may be positive for low values of n, but CS' is negative for high values of n which means that CS is inverted U-shaped or monotonically decreasing.

If τ ≤ (1 − γ), then W ′  > 0 which means that W is monotonically increasing.

If τ > (1 − γ), then W' may be positive for low values of n, but W' is negative for high values of n which means that W is inverted U-shaped or monotonically decreasing.

1.3 Proof of proposition 2:

From Eq. (4), it can be written as follows:

$$ {p}_i={a}_i-{q}_i-\gamma \sum \limits_{i\ne j}{q}_j $$

In symmetric market, this equation becomes p = a − (1 + γ(n − 1))q. The derivative of the price according to n can be written p^' =  − (γq + (1 + γ(n − 1))q^') which yields using Eqs. (5) and (6):

$$ \kern3.5em {p}^{\hbox{'}}=\frac{\gamma \left({\left(1+\gamma \left(n-2\right)\right)}^2\tau -\left(1-\gamma \right){\left(1+\gamma \left(n-1\right)\right)}^2\right)\left(a-{c}_0\right)}{{\left(\left(1-\gamma \right)\left(1+\gamma \left(n-1\right)\right)-\left(1+\gamma \left(n-2\right)\right)\tau +\left(1+\gamma \left(n-1\right)\right)\left(1+\gamma \left(n-2\right)\right)\right)}^2}\kern5.75em $$

(12)

The denominator is positive, thus the sign of p' is the sign of the numerator. p ′  ≥ 0 if $\tau \ge \left(1-\gamma \right){\left(\frac{1+\gamma \left(n-1\right)}{1+\gamma \left(n-2\right)}\right)}^2$. Price is increasing if τ is high enough. $\left(1-\gamma \right){\left(\frac{1+\gamma \left(n-1\right)}{1+\gamma \left(n-2\right)}\right)}^2$ is decreasing with n, as a result, the probability that p^' > 0 increases with n.

For n = 1, price is increasing if $\tau \ge \frac{1}{1-\gamma }$ and

$$ \underset{n\to +\infty }{\lim}\left(1-\gamma \right){\left(\frac{1+\gamma \left(n-1\right)}{1+\gamma \left(n-2\right)}\right)}^2=1-\gamma $$

As a result, if $\tau \ge \frac{1}{1-\gamma }$, then p^' ≥ 0 for all values of n; this means that the number of firms always increases price.

If τ ≤ 1 − γ, then p^' ≤ 0 for all values of n; this means that the number of firms always decreases price.

If $\left(1-\gamma \right)<\tau <\frac{1}{1-\gamma }$, then price is decreasing for low values of n and increasing for high values. The relationship between price and the number of firms is U-shaped.

1.4 Proof of Proposition 4:

From Lemma 2, consumer surplus and welfare are monotonically increasing if τ is under a certain level. An increase in technical progress may cross the threshold and thus decrease the number of firms maximizing consumer surplus or welfare.

The number of firms that maximizes consumer surplus or welfare is denoted n^** and n^* * *, respectively. If consumer surplus or welfare is monotonically decreasing, then the number of firms that maximizes consumer surplus or welfare is n^** = 1 and n^* * * = 1, respectively. In such cases, CS^'(1) < 0 or W^'(1) < 0

and $\frac{\partial {CS}^{\hbox{'}}(1)}{\partial \tau }<0$ or $\frac{\partial {W}^{\hbox{'}}(1)}{\partial \tau }<0$.

If the relationship between the number of firms and consumer surplus or welfare is inverted U-shaped, the number of firms that maximizes consumer surplus is such that CS^'(n^**) = 0 or W^'(n^* * *) = 0. In that case, $\frac{\partial {CS}^{\hbox{'}}\left({n}^{\ast \ast}\right)}{\partial \tau }<0$ or $\frac{\partial {W}^{\hbox{'}}\left({n}^{\ast \ast \ast}\right)}{\partial \tau }<0$. This means that the number of firms that maximizes consumer surplus or welfare decreases with technical progress.

1.4.1 Reallocation of Output Tends to Increase Industry-Level Investment

Investment of firm i is F_i = τq_i/2. Investment of the industry is

$$ {F}_{ind}=\frac{\tau }{2}\sum \limits_{i=1}^n{q_i}^2 $$

Let firm i be the least efficient firm: ∀j ≠ i, q_i < q_j. The reallocation of output decreases the output of firm i which becomes q_i − ε_i and increases the output of the other firms that are more efficient: q_j − ε_j. The reallocation induces ${\varepsilon}_i=\sum \limits_{j\ne i}{\varepsilon}_j$.

Investment of the industry after reallocation is written as follows:

$$ {F}_{indar}=\frac{\tau }{2}\left({\left({q}_i+{\varepsilon}_i\right)}^2+\sum \limits_{j\ne i}{\left({q}_j-{\varepsilon}_j\right)}^2\right) $$

If q_i < q_j, then ${\sum}_{j\ne i}{\varepsilon}_j{q}_i<{\sum}_{j\ne i}{\varepsilon}_j{q}_j$, and thus, ${\varepsilon}_i{q}_i<{\sum}_{j\ne i}{\varepsilon}_j{q}_j$ which leads to ${\sum}_{j\ne i}{\varepsilon}_j{q}_j-{\varepsilon}_i{q}_i>0$. As a result,

$$ {F}_{ind ar}-{F}_{ind}=\frac{\tau }{2}\left(2\left(\sum \limits_{j\ne i}{\varepsilon}_j{q}_j-{\varepsilon}_i{q}_i\right)+{\varepsilon_i}^2+\sum \limits_{j\ne i}{\varepsilon_j}^2\right)>0 $$

1.5 Proof of Lemma 3

The general expression of output of firm i can be written as follows:

$$ {q}_i\left(\gamma \right)=\frac{B\left(\left({B}^2+\left(1-\gamma \right)A- B\tau \right)\left({a}_i-{c}_{0i}\right)-\gamma B{\sum}_{j\ne i}\left({a}_j-{c}_{0j}\right)\right)}{\left(1-\gamma \right)A{B}^2+\left(\left(1-\gamma \right)A- B\tau \right)\left(\left(1-\gamma \right)A+B\left(1+B-\tau \right)\right)} $$

(13)

With A = 1 + γ(n − 1) and B = 1 + γ(n − 2).

γ^*is the value above which the least efficient firm has no output. Let j be the least efficient firm, by definition, q_j(γ^*) = 0.

For γ = 0, $\forall i,\kern0.5em {q}_i(0)=\frac{\left({a}_i-{c}_{0i}\right)}{\left(2-\tau \right)}$ and

$$ {F}_{ind}(0)=\frac{\tau {\sum}_{i=1}^n{\left({a}_i-{c}_{0i}\right)}^2}{2{\left(2-\tau \right)}^2} $$

$$ {F}_{ind}\left({\gamma}^{\ast}\right)=\frac{\tau }{2}\sum \limits_{i\ne j}{q_{i\left({\gamma}^{\ast}\right)}}^2 $$

From Eq. (13), we can check that $\frac{\partial {F}_{ind}(0)}{\partial \tau }>\frac{F_{ind}\left(\gamma \right)}{\partial \tau }$, provided γ ≤ γ^*. As a result, if for a given τ, F_ind(0) > F_ind(γ^*), then, for a higher τ, F_ind(0) > F_ind(γ^*) still holds.

For example, for n = 2,then ${q}_i\left(\gamma \right)=\frac{\left(2-{\gamma}^2-\tau \right)\left({a}_i-{c}_{0i}\right)-\gamma \left({a}_j-{c}_{0j}\right)}{{\left(2-{\gamma}^2-\tau \right)}^2-{\gamma}^2}$. If j is the least efficient firm:

$$ {\gamma}^{\ast }=\frac{\sqrt{{\left({a}_i-{c}_{oi}\right)}^2+4\left(2-\tau \right){\left({a}_j-{c}_{0j}\right)}^2}-\left({a}_i-{c}_{0i}\right)}{2\left({a}_j-{c}_{0j}\right)} $$

(14)

Let τ^* be the value of technical progress for which F_ind(0) = F_ind(γ^*).

$$ {\tau}^{\ast }=\frac{2\left({a}_j-{c}_{0j}\right)-\left(\sqrt{{\left({a}_i-{c}_{oi}\right)}^2+{\left({a}_j-{c}_{0j}\right)}^2}\right){\gamma}^{\ast }}{\left({a}_j-{c}_{0j}\right)} $$

(15)

If τ ≤ τ^*, then F_ind(0) ≤ F_ind(γ^*), and if τ > τ^*, then F_ind(0) > F_ind(γ^*).

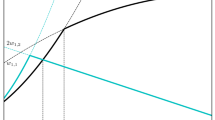
1.5.1 Investment in a Symmetric Market

For this figure, the parameters are the following: (a_i − c_0i) = 0.3 and τ = 0.8